Deck 3: Linear, Quadratic, Polynomial, and Rational Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/166
Play
Full screen (f)
Deck 3: Linear, Quadratic, Polynomial, and Rational Functions
1
Write the domain of the function r(x) as a union of intervals.
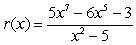
A)
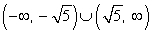
B)
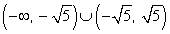
C)
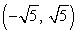
D)
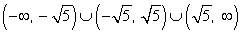
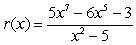
A)
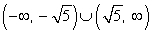
B)
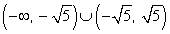
C)
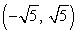
D)
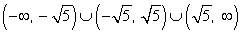
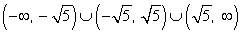
2
Write the domain of the function r(x) as a union of intervals.
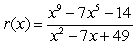
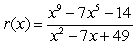
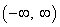
3
Suppose
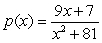 and
and 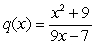 . Write the expression (p + q)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (p + q)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
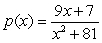 and
and 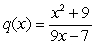 . Write the expression (p + q)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (p + q)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.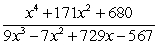
4
Suppose
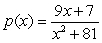 and
and 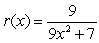 . Write the expression (p + r)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (p + r)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
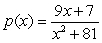 and
and 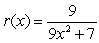 . Write the expression (p + r)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (p + r)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
5
Suppose
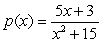 and
and 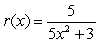 . Write the expression (3p - 15r)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (3p - 15r)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
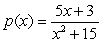 and
and 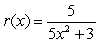 . Write the expression (3p - 15r)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (3p - 15r)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
6
Suppose
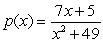 and
and 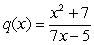 . Write the expression (pq)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (pq)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
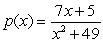 and
and 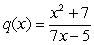 . Write the expression (pq)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (pq)(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
7
Suppose 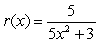 . Write the expression (r(x))2 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (r(x))2 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
A)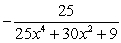
B)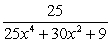
C)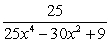
D)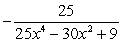
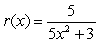 . Write the expression (r(x))2 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (r(x))2 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.A)
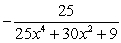
B)
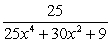
C)
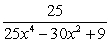
D)
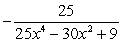

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
8
Suppose
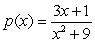 and
and 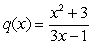 . Write the expression (q(x))2 p(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (q(x))2 p(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
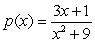 and
and 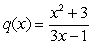 . Write the expression (q(x))2 p(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
. Write the expression (q(x))2 p(x) as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
9
Suppose
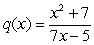 and
and 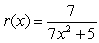 . Write the expression
. Write the expression 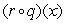 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
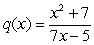 and
and 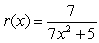 . Write the expression
. Write the expression 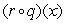 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
10
Suppose
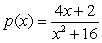 and
and 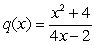 . Write the expression
. Write the expression 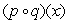 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
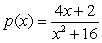 and
and 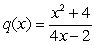 . Write the expression
. Write the expression 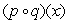 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
11
Suppose
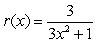 . Write the expression
. Write the expression 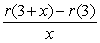 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
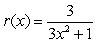 . Write the expression
. Write the expression 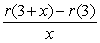 as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
as a ratio, with the numerator and denominator each written as a sum of terms of the form cxm.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
12
What is the domain of 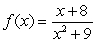 ?
?
A)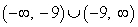
B)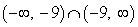
C)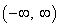
D)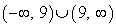
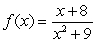 ?
?A)
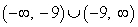
B)
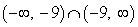
C)
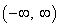
D)
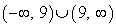

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
13
Find two distinct numbers x such that
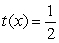 , where
, where 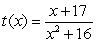
.
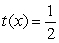 , where
, where 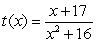
.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
14
What is the range of s, where s is defined by
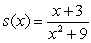 ?
?
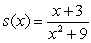 ?
?
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
15
Write the expression
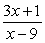 in the form
in the form 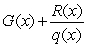 , where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
, where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
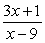 in the form
in the form 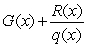 , where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
, where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
16
Write the expression
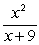 in the form
in the form 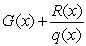 , where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
, where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
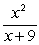 in the form
in the form 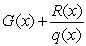 , where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
, where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
17
Write the expression
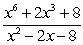 in the form
in the form 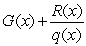 , where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
, where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
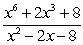 in the form
in the form 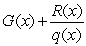 , where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
, where q is the denominator of the given expression and G and R are polynomials with deg R < deg q.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
18
Find a constant c such that 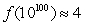 , where
, where 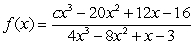 .
.
A) 4
B) 16
C) 0
D) -4
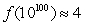 , where
, where 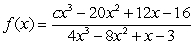 .
.A) 4
B) 16
C) 0
D) -4

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
19
Find the asymptotes of the graph of the function 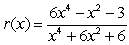
.
A) y = 6
B) y = 0
C) x = 6
D) x = 0
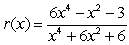
.
A) y = 6
B) y = 0
C) x = 6
D) x = 0

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
20
Find the asymptotes of the graph of the function
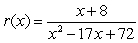
.
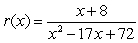
.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
21
Suppose p(x) = x2 + 6x + 4, q(x) = 6x3 - 4x + 1. Write the expression (p + q)(x) as a sum of terms, each of which is a constant times a power of x.
A) 6x3 + x2 + 2x + 5
B) 6x3 + x2 + 2x - 5
C) 6x3 + x2 - 2x + 5
D) 6x3 + x2 - 2x - 5
A) 6x3 + x2 + 2x + 5
B) 6x3 + x2 + 2x - 5
C) 6x3 + x2 - 2x + 5
D) 6x3 + x2 - 2x - 5

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
22
Suppose p(x) = x2 + 9x + 7, r(x) = 18x3 + 9. Write the expression (9p - 7r)(x) as a sum of terms, each of which is a constant times a power of x.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
23
Suppose p(x) = x2 + 3x + 1, r(x) = 6x3 + 3. Write the expression (p(x))2(r(x) - 3) as a sum of terms, each of which is a constant times a power of x.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
24
Suppose q(x) = 5x3 - 3x + 1, r(x) = 10x3 + 5. Write the expression
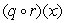 as a sum of terms, each of which is a constant times a power of x.
as a sum of terms, each of which is a constant times a power of x.
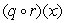 as a sum of terms, each of which is a constant times a power of x.
as a sum of terms, each of which is a constant times a power of x.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
25
Suppose p(x) = x2 + 4x + 2, q(x) = 4x3 - 2x + 1, r(x) = 8x3 + 4. Write the expression 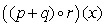 as a sum of terms, each of which is a constant times a power of x.
as a sum of terms, each of which is a constant times a power of x.
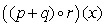 as a sum of terms, each of which is a constant times a power of x.
as a sum of terms, each of which is a constant times a power of x.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
26
Suppose p(x) = x2 + 8x + 6. Write the expression
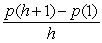 As a sum of terms, each of which is a constant times a power of h.
As a sum of terms, each of which is a constant times a power of h.
A) 10 - h
B) 10 + h
C) -6 + h
D) -6 - h
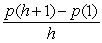 As a sum of terms, each of which is a constant times a power of h.
As a sum of terms, each of which is a constant times a power of h.A) 10 - h
B) 10 + h
C) -6 + h
D) -6 - h

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
27
Find all real numbers x such that
x4 + 36x2 - 2,592 = 0
x4 + 36x2 - 2,592 = 0

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
28
Find all real numbers x such that: 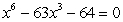
A) 4, 1
B) -4, 1
C) 4, -1
D) -4, -1
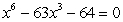
A) 4, 1
B) -4, 1
C) 4, -1
D) -4, -1

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
29
Find all real numbers x such that:
x4 - 53x2 + 196 = 0
x4 - 53x2 + 196 = 0

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
30
Suppose q(x) = . The point (7, b) lies on the graph of q.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
31
is correct?

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
32
Find a number b such that 6 is a zero of the polynomial p defined by p(x) = -36 + bx - 6x2 + x3.
A) 36
B) -36
C) 6
D) -6
A) 36
B) -36
C) 6
D) -6

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
33
Find a number b such that 1 is a zero of the polynomial p defined by p(x) = b - 9x + bx2 + 9x3.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
34
Find a polynomial p of degree 3 such that -6, 6, and 7 are zeros of p and p(0) = 36. Write your answer in descending order by degree.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
35
Find a polynomial p of degree 3 such that -1,
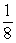 , and 1 are zeros of p and p(0) =
, and 1 are zeros of p and p(0) = 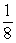 . Write your answer in descending order by degree.
. Write your answer in descending order by degree.
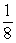 , and 1 are zeros of p and p(0) =
, and 1 are zeros of p and p(0) = 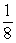 . Write your answer in descending order by degree.
. Write your answer in descending order by degree.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
36
If p(x) = a2x2 + 4x + 5 and q(x) = (1 - 2a)x2 - 8x - 5, find a real number a such that (p + q)(x) has degree 1.
A) 4
B) -4
C) 0
D) 1
A) 4
B) -4
C) 0
D) 1

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
37
Suppose d is a real number. Then if and only if d = 0

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
38
The only possible integer zeros of are 1 and 2.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
39
Find all choices of (b, c, d) such that 4 and 1 are the only zeros of the polynomial p(x) = x3 + bx2 + cx + d.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
40
Find all choices of (b, c, d) such that 9 and -9 are the only zeros of the polynomial p(x) = x3 + bx2 + cx + d.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
41
Factor x24 - y12 as nicely as possible.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
42
Evaluate the expression
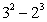
.
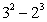
.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
43
Write 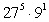 as a power of 3.
as a power of 3.
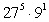 as a power of 3.
as a power of 3.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
44
Simplify the given expression by writing it as a power of a single variable.
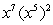
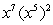

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
45
Simplify the given expression by writing it as a power of a single variable.
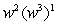
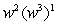

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
46
Simplify the given expression by writing it as a power of a single variable.
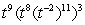
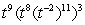

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
47
Write 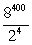 as a power of 2.
as a power of 2.
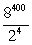 as a power of 2.
as a power of 2.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
48
Find integers m and n such that 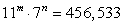
.
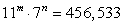
.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
49
Simplify the given expression. 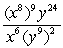
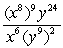

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
50
Simplify the given expression.
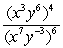
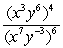

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
51
Simplify the given expression. 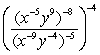
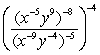

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
52
Find a formula for 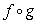 if
if 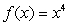 and
and 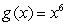
.
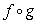 if
if 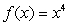 and
and 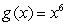
.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
53
Find a formula for 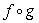 if
if 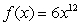 and
and 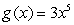
.
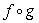 if
if 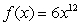 and
and 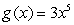
.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
54
Find the function which is graphed below
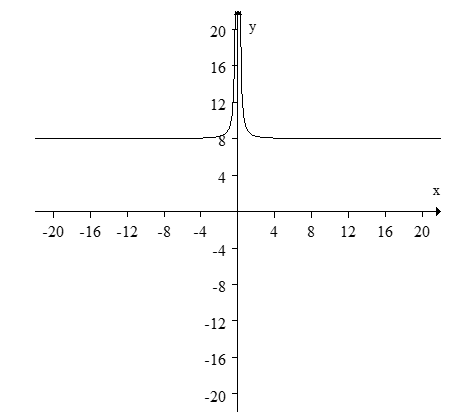
A)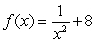
B)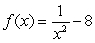
C)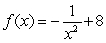
D)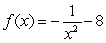

A)
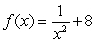
B)
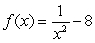
C)
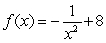
D)
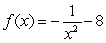

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
55
Find the function which is graphed below.
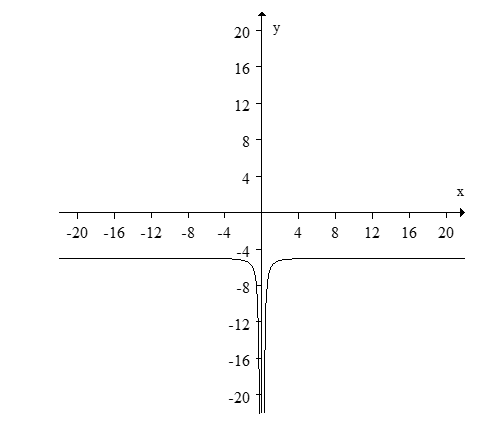
A)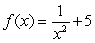
B)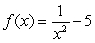
C)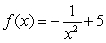
D)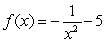

A)
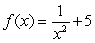
B)
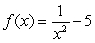
C)
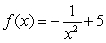
D)
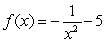

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
56
Find the function which is graphed below.
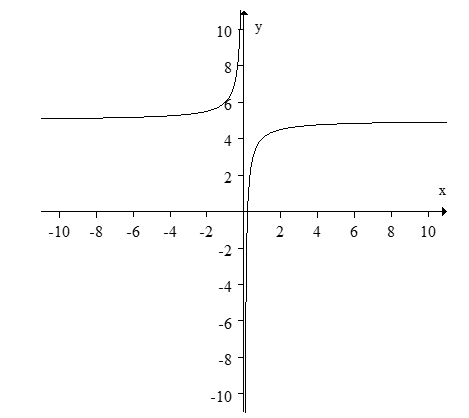
A)
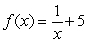
B)
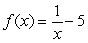
C)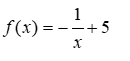 D)
D)
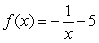

A)
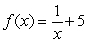
B)
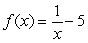
C)
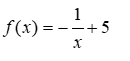 D)
D)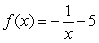

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
57
Find the function which is graphed below.
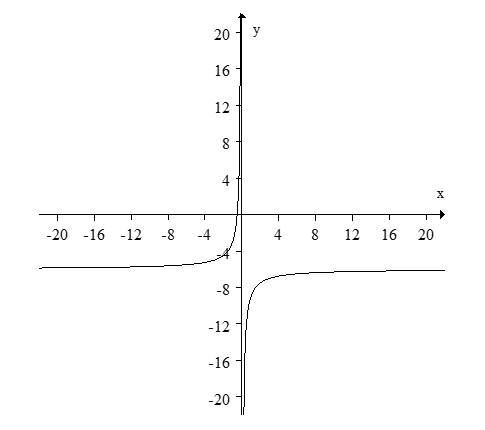
A)
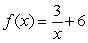
B)
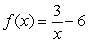
C)
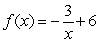
D)
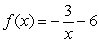

A)
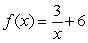
B)
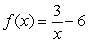
C)
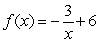
D)
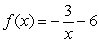

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
58
Find the function which is graphed below.
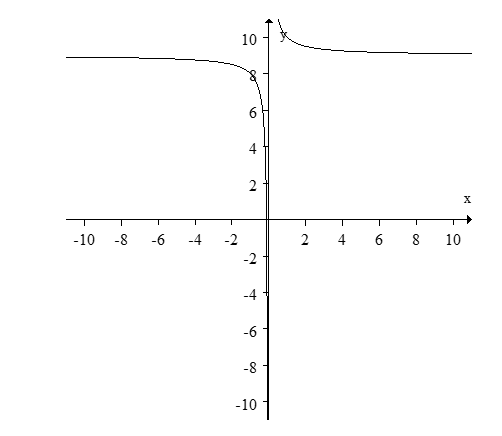
A)
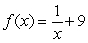
B)
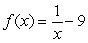
C)
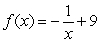
D)
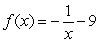

A)
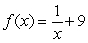
B)
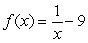
C)
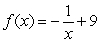
D)
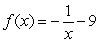

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
59
Evaluate the indicated quantity.253/2
A) 25
B) 5
C) 37.5
D) 125
A) 25
B) 5
C) 37.5
D) 125

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
60
Evaluate the indicated quantity.(-512)5/3
A) 32,768
B) -32,768
C) 512
D) Undefined
A) 32,768
B) -32,768
C) 512
D) Undefined

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
61
Expand the indicated expression. 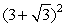
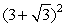

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
62
Expand the indicated expression. 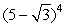
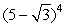

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
63
Expand the indicated expression. 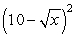
A)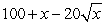
B)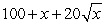
C)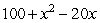
D)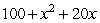
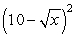
A)
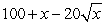
B)
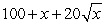
C)
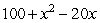
D)
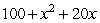

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
64
Expand the indicated expression. 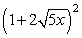
A)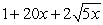
B)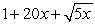
C)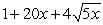
D)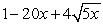
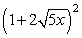
A)
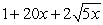
B)
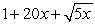
C)
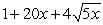
D)
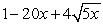

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
65
Find a formula for the inverse function 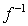 of the indicated function.
of the indicated function. 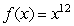
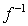 of the indicated function.
of the indicated function. 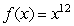

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
66
Find a formula for the inverse function 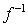 Of the indicated function.
Of the indicated function. 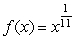
A)
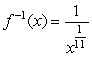
B)
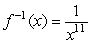
C)
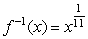
D)
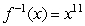
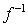 Of the indicated function.
Of the indicated function. 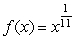
A)
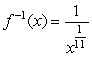
B)
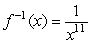
C)
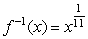
D)
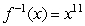

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
67
Find a formula for the inverse function 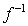 of the indicated function.
of the indicated function. 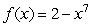
A)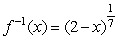
B)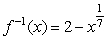
C)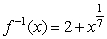
D)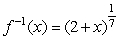
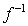 of the indicated function.
of the indicated function. 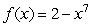
A)
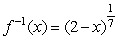
B)
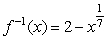
C)
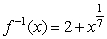
D)
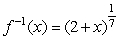

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
68
Find a formula for the inverse function
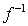 of the indicated function.
of the indicated function. 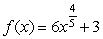
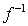 of the indicated function.
of the indicated function. 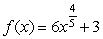

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
69
Find a formula for (f 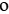 g)(x) assuming that f and g are the indicated functions.
g)(x) assuming that f and g are the indicated functions.
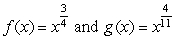 .
.
 g)(x) assuming that f and g are the indicated functions.
g)(x) assuming that f and g are the indicated functions. 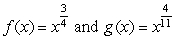 .
.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
70
Find a formula for (f  g)(x) assuming that f and g are the indicated functions.
g)(x) assuming that f and g are the indicated functions. 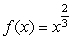 and
and 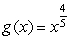 .
.
A)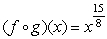
B)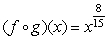
C)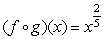
D)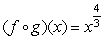
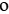 g)(x) assuming that f and g are the indicated functions.
g)(x) assuming that f and g are the indicated functions. 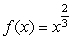 and
and 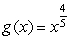 .
.A)
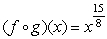
B)
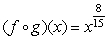
C)
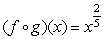
D)
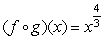

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
71
Find all real numbers x such that satisfy the indicated equation.
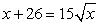
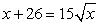

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
72
Find all real numbers x such that satisfy the indicated equation. 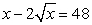
A)
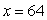 And
And 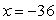
B)
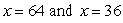
C)
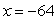
D)
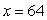
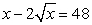
A)
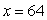 And
And 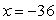
B)
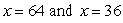
C)
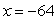
D)
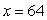

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
73
Find all real numbers x such that satisfy the indicated equation.
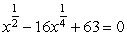
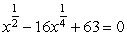

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
74
Suppose x is a number such that 2x = 5. Evaluate 16x.
A) 625
B) 20
C) 80
D) 40
A) 625
B) 20
C) 80
D) 40

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
75
Evaluate the 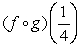 assuming that f and g are the functions defined by f(x) = 3x and
assuming that f and g are the functions defined by f(x) = 3x and 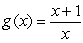
.
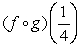 assuming that f and g are the functions defined by f(x) = 3x and
assuming that f and g are the functions defined by f(x) = 3x and 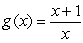
.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
76
What is the domain of the function 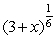 ? Write your answer using set builder notation.
? Write your answer using set builder notation.
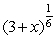 ? Write your answer using set builder notation.
? Write your answer using set builder notation.
Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
77
If x and y are irrational numbers, then xy is always an irrational number.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
78
If x and y are irrational numbers, then x + y may be a rational number.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
79
Find the vertex of the graph of the function
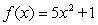
.
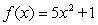
.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck
80
Find the vertex of the graph of the function f(x) = (x - 11)2 + 9.

Unlock Deck
Unlock for access to all 166 flashcards in this deck.
Unlock Deck
k this deck



