Deck 1: Linear Functions, Equations, and Inequalities
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/44
Play
Full screen (f)
Deck 1: Linear Functions, Equations, and Inequalities
1
For each of the functions, determine the
(i) domain
(ii) range
(iii) -intercept(s)
(iv) -intercept(s).
(a)
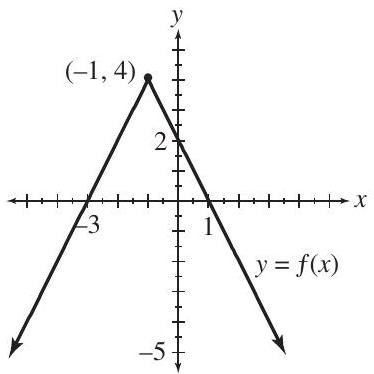
(b)
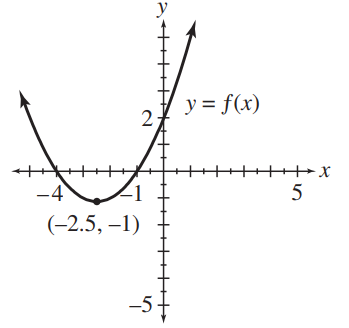
(c)
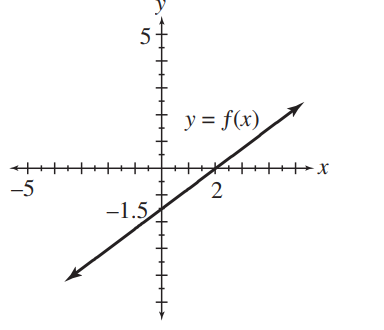
(i) domain
(ii) range
(iii) -intercept(s)
(iv) -intercept(s).
(a)

(b)
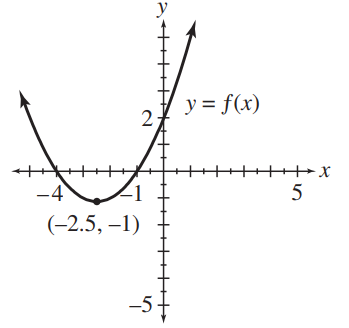
(c)
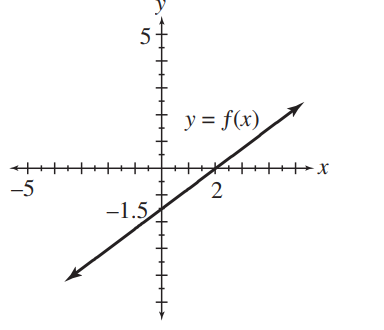
(a) (i)
(ii)
(iii) -3 and 1
(iv) 2
(b) (i)
(ii)
(iii) -4 and -1
(iv) 2
(c) (i)
(ii)
(iii) 2
(iv) -1.5
(ii)
(iii) -3 and 1
(iv) 2
(b) (i)
(ii)
(iii) -4 and -1
(iv) 2
(c) (i)
(ii)
(iii) 2
(iv) -1.5
2
Use the figure to solve each equation or inequality.
(a)
(b)
(c)
(d)
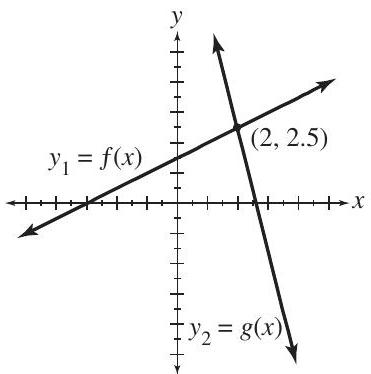
(a)
(b)
(c)
(d)

(a)
(b)
(c)
(d)
(b)
(c)
(d)
3
Use the screen to solve the equation or inequality. Here the function is a linear function defined over the domain of real numbers.
(a)
(b)
(c)
(d)
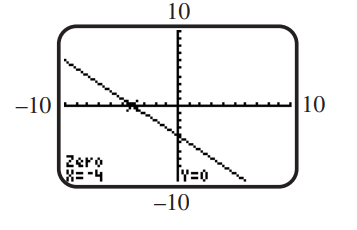
(a)
(b)
(c)
(d)

(a)
(b)
(c)
(d)
(b)
(c)
(d)
4
Consider the linear functions and .
(a) Solve analytically, showing all steps. Also, check analytically.
(b) Graph and and use your result in part (a) to find the solution set of . Explain your answer.
(c) Repeat part (b) for
(a) Solve analytically, showing all steps. Also, check analytically.
(b) Graph and and use your result in part (a) to find the solution set of . Explain your answer.
(c) Repeat part (b) for

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
5
Consider the linear function .
(a) Solve the equation analytically.
(b) Solve the inequality analytically.
(c) Graph in an appropriate viewing window and explain how the graph supports your answers in parts (a) and (b).
(a) Solve the equation analytically.
(b) Solve the inequality analytically.
(c) Graph in an appropriate viewing window and explain how the graph supports your answers in parts (a) and (b).

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
6
During the period from 1996 to 2006 the average annual cost (in dollars) of tuition and fees at private four-year colleges rose in an approximately linear fashion. The graph depicts this growth using a line segment. (Source: The College Board)
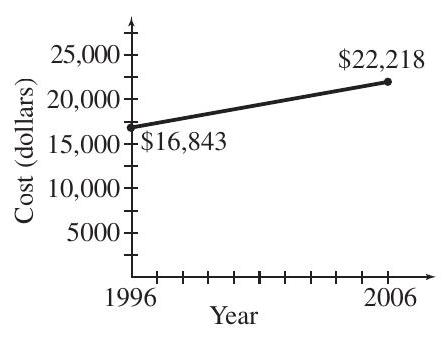
(a) Use the midpoint formula to approximate the cost during the year 2001.
(b) Find the slope of the line and explain its meaning in the context of this situation.
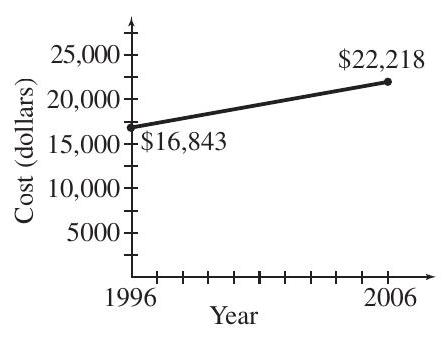
(a) Use the midpoint formula to approximate the cost during the year 2001.
(b) Find the slope of the line and explain its meaning in the context of this situation.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
7
Find the equation of the line passing through the point and
(a) parallel to the line with equation .
(b) perpendicular to the line with equation .
(a) parallel to the line with equation .
(b) perpendicular to the line with equation .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
8
Find the - and -intercepts of the line whose standard form is . What is the slope of this line?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
9
Give the equations of both the horizontal and vertical lines passing through the point .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
10
The table lists the Gross Domestic Product (GDP) from 2004 to 2008 in billions of dollars. (Source: U.S. Department of Commerce)
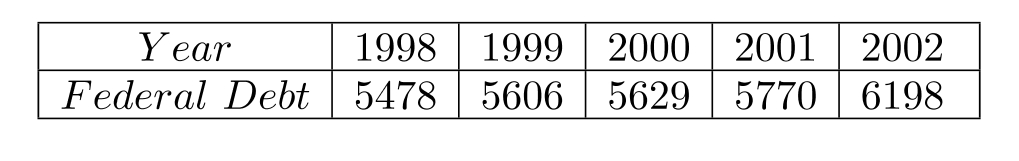
(a) Find the least-squares regression line for the data. Give the correlation coefficient.
(b) Use this line to predict the federal debt in 2009.

(a) Find the least-squares regression line for the data. Give the correlation coefficient.
(b) Use this line to predict the federal debt in 2009.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
11
Suppose that an empty circular wading pool has a radius of 7 feet. During a storm, rain falling at a rate of 1 inch per hour begins to fill the pool. A small drain at the bottom of the pool is capable of draining 35 gallons of water per hour.
(a) Determine the number of cubic inches of water falling into the pool in one hour. (Hint: Each hour a layer of water 1 inch thick falls into the pool.)
(b) One gallon of water equals about 231 cubic inches. Write a formula for a function that computes the gallons of water landing in the pool in hours.
(c) How many gallons of water land in the pool during a 3 hour storm?
(d) Will the drain be able to keep up with the rainfall? If not, how many such drains would be needed?
(a) Determine the number of cubic inches of water falling into the pool in one hour. (Hint: Each hour a layer of water 1 inch thick falls into the pool.)
(b) One gallon of water equals about 231 cubic inches. Write a formula for a function that computes the gallons of water landing in the pool in hours.
(c) How many gallons of water land in the pool during a 3 hour storm?
(d) Will the drain be able to keep up with the rainfall? If not, how many such drains would be needed?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
12
For each of the functions, determine the
(i) domain
(ii) range
(iii) x-intercept(s)
(iv) y-intercept(s).
(a)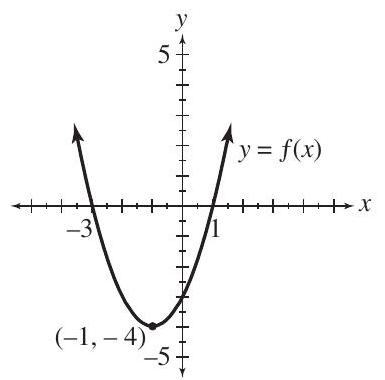
(b)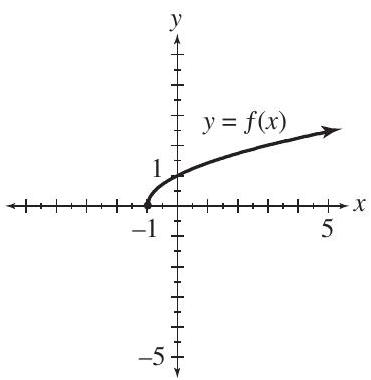
(c)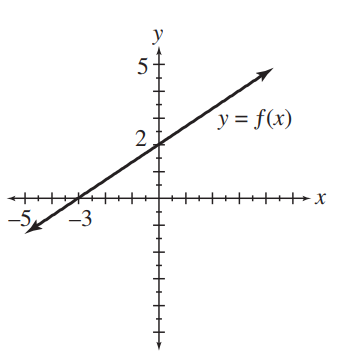
(i) domain
(ii) range
(iii) x-intercept(s)
(iv) y-intercept(s).
(a)

(b)

(c)
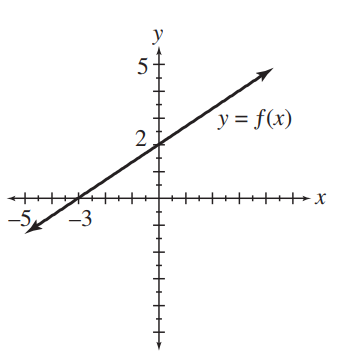

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
13
Use the figure to solve each equation or inequality.
(a)
(b)
(c)
(d)
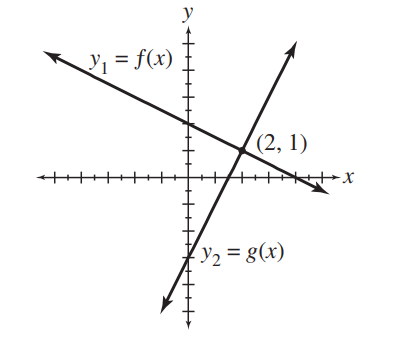
(a)
(b)
(c)
(d)
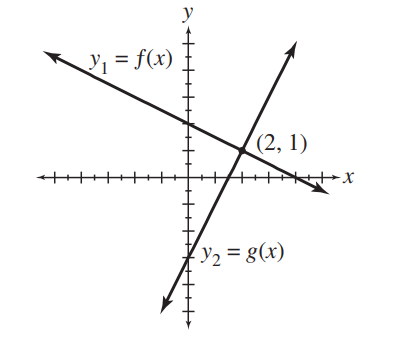

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
14
Use the screen to solve the equation or inequality. Here the function is a linear function defined over the domain of real numbers.
(a)
(b)
(c)
(d)
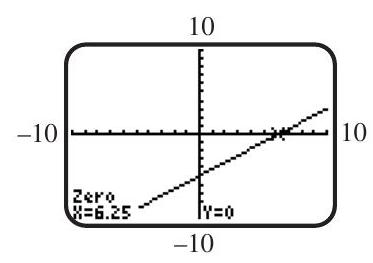
(a)
(b)
(c)
(d)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
15
Consider the linear functions and .
(a) Solve analytically, showing all steps. Also, check analytically.
(b) Graph and and use your result in part (a) to find the solution set of . Explain your answer.
(c) Repeat part (b) for .
(a) Solve analytically, showing all steps. Also, check analytically.
(b) Graph and and use your result in part (a) to find the solution set of . Explain your answer.
(c) Repeat part (b) for .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
16
Consider the linear function .
(a) Solve the equation analytically.
(b) Solve the inequality analytically.
(c) Graph in an appropriate viewing window and explain how the graph supports your answers in parts (a) and (b).
(a) Solve the equation analytically.
(b) Solve the inequality analytically.
(c) Graph in an appropriate viewing window and explain how the graph supports your answers in parts (a) and (b).

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
17
For the years 1996 to 2000, it was estimated that Medicare costs (in billions of dollars) would rise in a linear fashion. See the graph below. (Source: U.S. Office of Management and Budget)
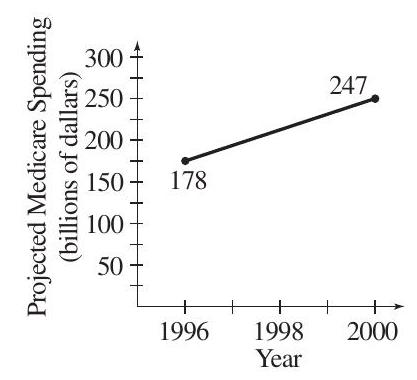
(a) Use the midpoint formula to approximate Medicare costs during the year 1998.
(b) Find the slope of the line and explain its meaning in the context of this situation.

(a) Use the midpoint formula to approximate Medicare costs during the year 1998.
(b) Find the slope of the line and explain its meaning in the context of this situation.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
18
Find the equation of the line passing through the point and
(a) parallel to the line with equation .
(b) perpendicular to the line with equation .
(a) parallel to the line with equation .
(b) perpendicular to the line with equation .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
19
Find the - and -intercepts of the line whose standard form is .
What is the slope of this line?
What is the slope of this line?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
20
Give the equations of both the horizontal and vertical lines passing through the point .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
21
The table lists the average cost (in dollars) of tuition and fees at public four-year colleges for selected years. (Source: The College Board)
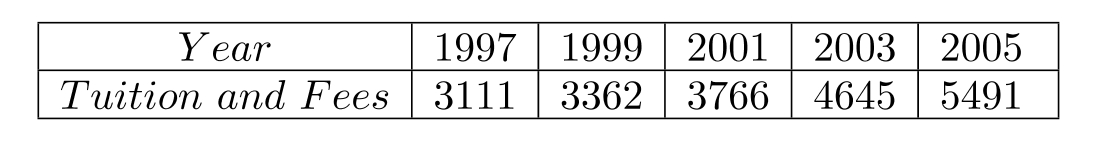
(a) Find the least-squares regression line for the data. Give the correlation coefficient.
(b) Use this line to predict the average annual cost of tuition and fees in 2007.

(a) Find the least-squares regression line for the data. Give the correlation coefficient.
(b) Use this line to predict the average annual cost of tuition and fees in 2007.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
22
Suppose that an empty circular wading pool has a radius of 5 feet. During a storm, rain falling at a rate of 1.5 inches per hour begins to fill the pool. A small drain at the bottom of the pool is capable of draining 30 gallons of water per hour.
(a) Determine the number of cubic inches of water falling into the pool in one hour. (Hint: Each hour a layer of water 1.5 inches thick falls into the pool.)
(b) One gallon of water equals about 231 cubic inches. Write a formula for a function that computes the gallons of water landing in the pool in hours.
(c) How many gallons of water land in the pool during a 2.5 hour storm?
(d) Will the drain be able to keep up with the rainfall? If not, how many such drains would be needed?
(a) Determine the number of cubic inches of water falling into the pool in one hour. (Hint: Each hour a layer of water 1.5 inches thick falls into the pool.)
(b) One gallon of water equals about 231 cubic inches. Write a formula for a function that computes the gallons of water landing in the pool in hours.
(c) How many gallons of water land in the pool during a 2.5 hour storm?
(d) Will the drain be able to keep up with the rainfall? If not, how many such drains would be needed?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
23
For each of the functions, determine the
(i) domain
(ii) range
(iii) -intercept(s)
(iv) -intercept(s).
(a)
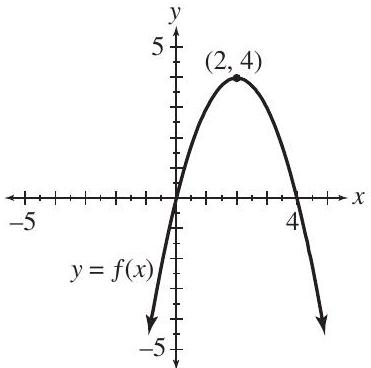
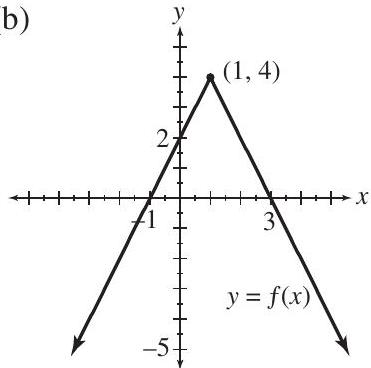
(c)
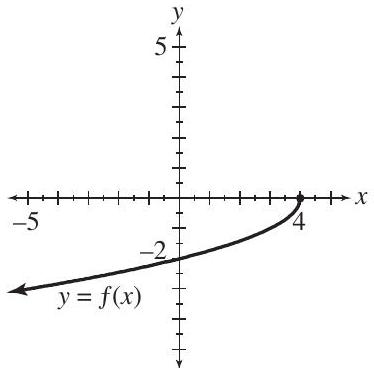
(i) domain
(ii) range
(iii) -intercept(s)
(iv) -intercept(s).
(a)


(c)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
24
Use the figure to solve each equation or inequality.
(a)
(b)
(c)
(d)
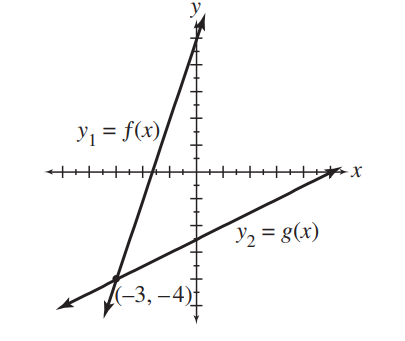
(a)
(b)
(c)
(d)
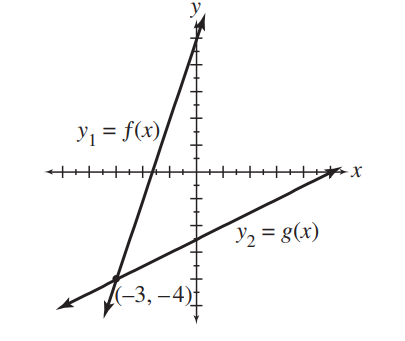

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
25
Use the screen to solve the equation or inequality. Here the function is a linear function defined over the domain of real numbers.
(a)
(b)
(c)
(d)
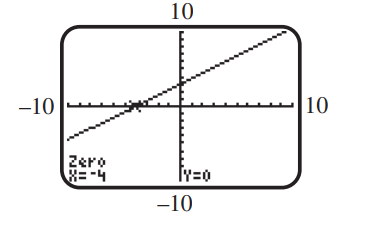
(a)
(b)
(c)
(d)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
26
Consider the linear functions and .
(a) Solve analytically, showing all steps. Also, check analytically.
(b) Graph and and use your result in part (a) to find the solution set of . Explain your answer.
(c) Repeat part (b) for
(a) Solve analytically, showing all steps. Also, check analytically.
(b) Graph and and use your result in part (a) to find the solution set of . Explain your answer.
(c) Repeat part (b) for

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
27
Consider the linear function .
(a) Solve the equation analytically.
(b) Solve the inequality analytically.
(c) Graph in an appropriate viewing window and explain how the graph supports your answers in parts (a) and (b).
(a) Solve the equation analytically.
(b) Solve the inequality analytically.
(c) Graph in an appropriate viewing window and explain how the graph supports your answers in parts (a) and (b).

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
28
The graph below shows a line segment depicting the number of injuries and illnesses resulting in days away from work from 2004 to 2006. (Source: U.S. Department of Labor Statistics)
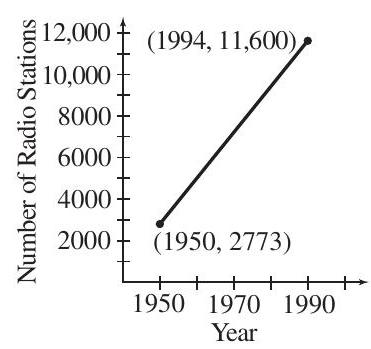
(a) Use the midpoint formula to approximate the number of illnesses and injuries during the year 2005.
(b) Find the slope of the line and explain its meaning in the context of this situation.

(a) Use the midpoint formula to approximate the number of illnesses and injuries during the year 2005.
(b) Find the slope of the line and explain its meaning in the context of this situation.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
29
Find the equation of the line passing through the point and
(a) parallel to the line with equation .
(b) perpendicular to the line with equation .
(a) parallel to the line with equation .
(b) perpendicular to the line with equation .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
30
Find the - and -intercepts of the line whose standard form is .
What is the slope of this line?
What is the slope of this line?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
31
Give the equations of both the horizontal and vertical lines passing through the point .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
32
The table lists the period of revolution (in days) of each planet and the mean distance of the sun (in millions of miles) from each planet.
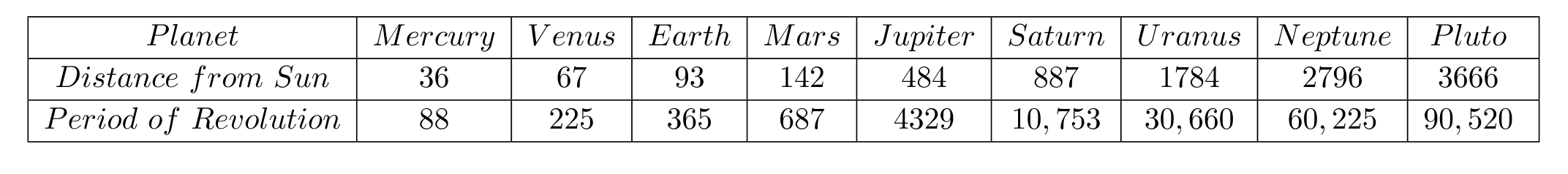
(a) Find the least-squares regression line for the data. Give the correlation coefficient.
(b) Use this line to predict the period of revolution of an asteroid that has a mean distance of 260 million miles from the sun.

(a) Find the least-squares regression line for the data. Give the correlation coefficient.
(b) Use this line to predict the period of revolution of an asteroid that has a mean distance of 260 million miles from the sun.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
33
Suppose that an empty circular wading pool has a radius of 9 feet. During a storm, rain falling at a rate of 0.5 inch per hour begins to fill the pool. A small drain at the bottom of the pool is capable of draining 20 gallons of water per hour.
(a) Determine the number of cubic inches of water falling into the pool in one hour. (Hint: Each hour a layer of water 0.5 inch thick falls into the pool.)
(b) One gallon of water equals about 231 cubic inches. Write a formula for a function that computes the gallons of water landing in the pool in hours.
(c) How many gallons of water land in the pool during a 2.5 hour storm?
(d) Will the drain be able to keep up with the rainfall? If not, how many such drains would be needed?
(a) Determine the number of cubic inches of water falling into the pool in one hour. (Hint: Each hour a layer of water 0.5 inch thick falls into the pool.)
(b) One gallon of water equals about 231 cubic inches. Write a formula for a function that computes the gallons of water landing in the pool in hours.
(c) How many gallons of water land in the pool during a 2.5 hour storm?
(d) Will the drain be able to keep up with the rainfall? If not, how many such drains would be needed?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
34
For each of the functions, determine the
(i) domain
(ii) range
(iii) -intercept(s)
(iv) -intercept(s).
(a)
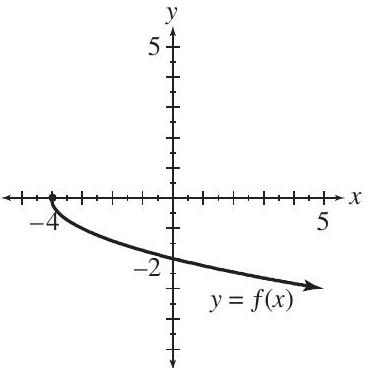
(b)
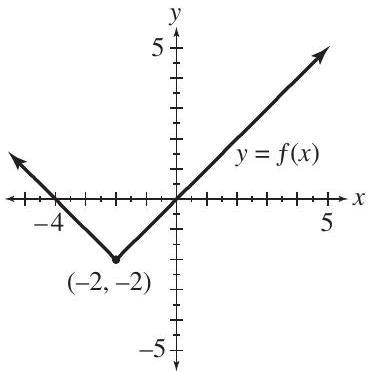
(c)
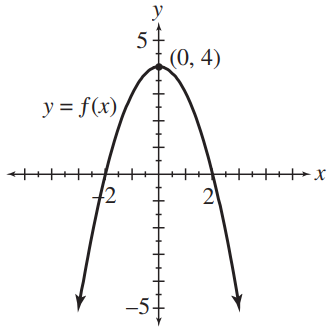
(i) domain
(ii) range
(iii) -intercept(s)
(iv) -intercept(s).
(a)

(b)

(c)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
35
Use the figure to solve each equation or inequality.
(a)
(b)
(c)
(d)
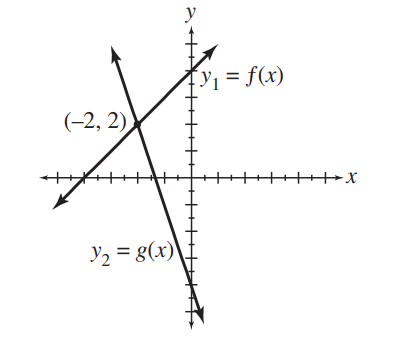
(a)
(b)
(c)
(d)
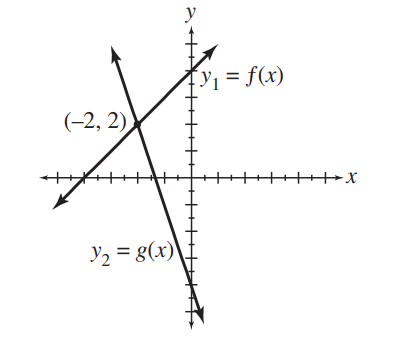

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
36
Use the screen to solve the equation or inequality. Here the function is a linear function defined over the domain of real numbers.
(a)
(b)
(c)
(d)
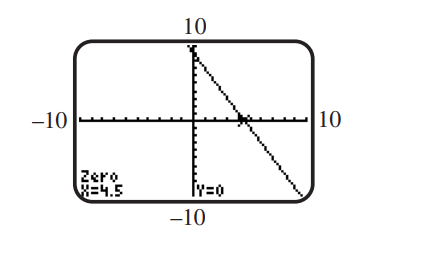
(a)
(b)
(c)
(d)


Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
37
Consider the linear functions and .
(a) Solve analytically, showing all steps. Also, check analytically.
(b) Graph and and use your result in part (a) to find the solution set of . Explain your answer.
(c) Repeat part (b) for .
(a) Solve analytically, showing all steps. Also, check analytically.
(b) Graph and and use your result in part (a) to find the solution set of . Explain your answer.
(c) Repeat part (b) for .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
38
Consider the linear function .
(a) Solve the equation analytically.
(b) Solve the inequality analytically.
(c) Graph in an appropriate viewing window and explain how the graph supports your answers in parts (a) and (b).
(a) Solve the equation analytically.
(b) Solve the inequality analytically.
(c) Graph in an appropriate viewing window and explain how the graph supports your answers in parts (a) and (b).

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
39
From 2004 to 2008 the federal debt (in billion of dollars) rose in approximately linear fashion. See the graph below. (Source: U.S. Office of Management and Budget)
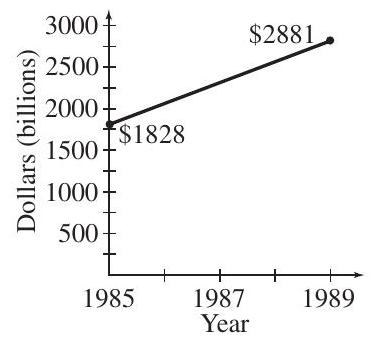
(a) Use the midpoint formula to approximate the debt during the year 2006.
(b) Find the slope of the line and explain its meaning in the context of this situation.

(a) Use the midpoint formula to approximate the debt during the year 2006.
(b) Find the slope of the line and explain its meaning in the context of this situation.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
40
Find the equation of the line passing through the point and
(a) parallel to the line with equation .
(b) perpendicular to the line with equation .
(a) parallel to the line with equation .
(b) perpendicular to the line with equation .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
41
Find the - and -intercepts of the line whose standard form is .
What is the slope of this line?
What is the slope of this line?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
42
Give the equations of both the horizontal and vertical lines passing through the point .

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
43
The table lists the average cost (in dollars) of tuition and fees at private four-year colleges for selected years. (Source: The College Board)
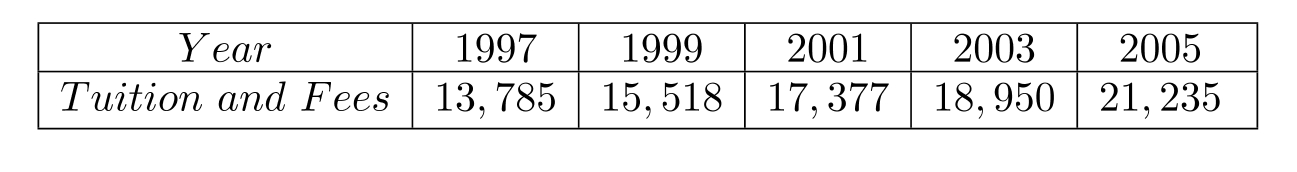
(a) Find the least-squares regression line for the data. Give the correlation coefficient.
(b) Use this line to predict the average annual cost of tuition and fees in 2002.

(a) Find the least-squares regression line for the data. Give the correlation coefficient.
(b) Use this line to predict the average annual cost of tuition and fees in 2002.

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck
44
Suppose that an empty circular wading pool has a radius of 5 feet. During a storm, rain falling at a rate of 0.6 inch per hour begins to fill the pool. A small drain at the bottom of the pool is capable of draining 35 gallons of water per hour.
(a) Determine the number of cubic inches of water falling into the pool in one hour. (Hint: Each hour a layer of water 0.6 inch thick falls into the pool.)
(b) One gallon of water equals about 231 cubic inches. Write a formula for a function that computes the gallons of water landing in the pool in hours.
(c) How many gallons of water land in the pool during a 2 hour storm?
(d) Will the drain be able to keep up with the rainfall? If not, how many such drains would be needed?
(a) Determine the number of cubic inches of water falling into the pool in one hour. (Hint: Each hour a layer of water 0.6 inch thick falls into the pool.)
(b) One gallon of water equals about 231 cubic inches. Write a formula for a function that computes the gallons of water landing in the pool in hours.
(c) How many gallons of water land in the pool during a 2 hour storm?
(d) Will the drain be able to keep up with the rainfall? If not, how many such drains would be needed?

Unlock Deck
Unlock for access to all 44 flashcards in this deck.
Unlock Deck
k this deck



