Deck 6: Systems and Matrices
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/68
Play
Full screen (f)
Deck 6: Systems and Matrices
1
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
Parabola; opens right
2
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
Hyperbola; center
3
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
Circle; center (4, -3 ; radius 6
4
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
5
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
6
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center
-
A) Ellipse; center
B) Parabola; opens right
C) Circle; center (4, -3 ; radius 6
D) Parabola; opens upward
E) Circle; center ; radius 6
F) Hyperbola; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
7
Give the coordinates of the focus and the equation of the directrix for the parabola with equation .

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
8
Graph . Is the graph that of a function? Find the domain and range.

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
9
The screen shown here gives the graph of as generated by a graphing calculator.
What two functions were used to obtain the graph?
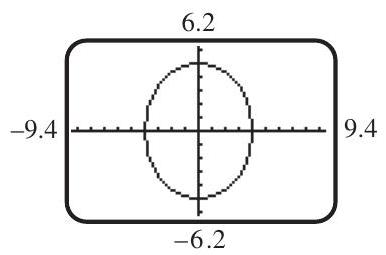
What two functions were used to obtain the graph?


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
10
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
- in
- in

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
11
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
12
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
13
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
14
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
15
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
16
Write an equation for each of the following.
(a) The conic with focus at the origin and vertex at and .
(b) The conic with center at the origin, focus at and .
(a) The conic with focus at the origin and vertex at and .
(b) The conic with center at the origin, focus at and .

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
17
Some comets orbit the sun in elliptical orbits with the sun at one focus. The path of the orbit of Halley's Comet has a major axis of length 35.8 astronomical units (AU) and an eccentricity of .967. (Source: NASA.) Position the center of the ellipse at the origin and determine the equation of the ellipse. What is the minimum distance between Halley's Comet and the sun?

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
18
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
19
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
20
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
21
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
22
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
23
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 5
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 5
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
24
Give the coordinates of the focus and the equation of the directrix for the parabola with equation .

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
25
Graph . Is the graph that of a function? Find the domain and range.

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
26
The screen shown here gives the graph of as generated by a graphing calculator.
What two functions were used to obtain the graph?
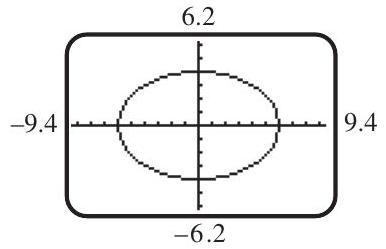
What two functions were used to obtain the graph?


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
27
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
28
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable
- in
- in

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
29
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
30
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
31
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
32
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
33
Write an equation for each of the following.
(a) The conic with center at the origin, focus at and .
(b) The conic centered at the origin with focus at and .
(a) The conic with center at the origin, focus at and .
(b) The conic centered at the origin with focus at and .

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
34
A gallery has the shape of the top half of an ellipse. The cross section is 13 feet high at the center and 40 feet long. Find the equation of the complete ellipse. How far apart are the foci?

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
35
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
36
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
37
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
38
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
39
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
40
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left
-
A) Ellipse; center
B) Hyperbola; center
C) Circle; center ; radius 4
D) Parabola; opens downward
E) Circle; center ; radius 4
F) Parabola; opens left

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
41
Give the coordinates of the focus and the equation of the directrix for the parabola with equation .

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
42
Graph . Is the graph that of a function? Find the domain and range.

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
43
The screen shown here gives the graph of as generated by a graphing calculator.
What two functions were used to obtain the graph?
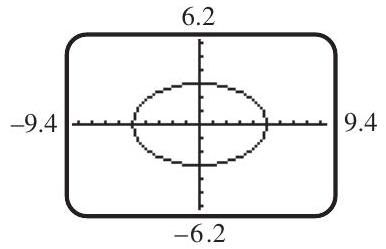
What two functions were used to obtain the graph?


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
44
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
45
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
- in
- in

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
46
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
47
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
48
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
49
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
50
Write an equation for each of the following.
(a) The conic with center at the origin, focus at and .
(b) The conic with focus at the origin, vertex at and .
(a) The conic with center at the origin, focus at and .
(b) The conic with focus at the origin, vertex at and .

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
51
Planets in our solar system orbit the sun in elliptical orbits with the sun at one focus. The path of the orbit of Mercury has a major axis of length 115.8 million miles and an eccentricity of .206. (Source: NASA.) Position the center of the ellipse at the origin and determine the equation of the ellipse. What is the maximum distance between Mercury and the sun?

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
52
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
53
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
54
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
55
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
56
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
57
Match each equation on the left with the appropriate description of its graph on the right.
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center
-
A) Circle; center ; radius 3
B) Hyperbola; center
C) Parabola; opens left
D) Circle; center ; radius 3
E) Parabola; opens downward
F) Ellipse; center

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
58
Give the coordinates of the focus and the equation of the directrix for the parabola with equation .

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
59
Graph . Is the graph that of a function? Find the domain and range.

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
60
The screen shown here gives the graph of as generated by a graphing calculator.
What two functions were used to obtain the graph?
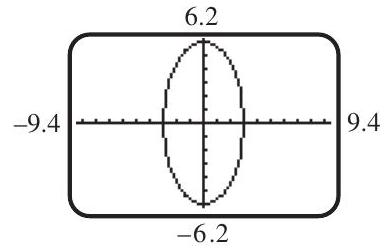
What two functions were used to obtain the graph?


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
61
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
62
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
63
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
- in
- in

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
64
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
65
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
66
Graph the relation. Identify the graph and give the the radius, center, vertices, and foci as applicable.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
67
Write an equation for each of the following.
(a) The conic with center at the origin, focus at and .
(b) The conic centered at the origin with vertical major axis of length 6 and .
(a) The conic with center at the origin, focus at and .
(b) The conic centered at the origin with vertical major axis of length 6 and .

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
68
A comet following a parabolic path with the Earth at the focus came within 8 million miles of the Earth. Position the Earth at the origin and the perihelion (closest) point of the orbit on the positive horizontal axis and determine the equation of the parabola. How far is the comet from Earth when it crosses the vertical axis?

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck



