Deck 5: Inverse, Exponential, and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/84
Play
Full screen (f)
Deck 5: Inverse, Exponential, and Logarithmic Functions
1
Match each equation with its graph.
-
A)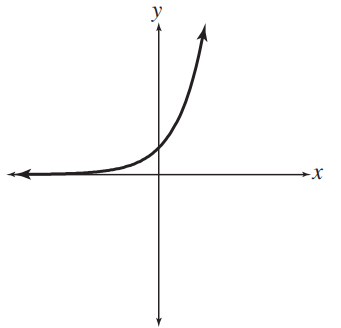
B)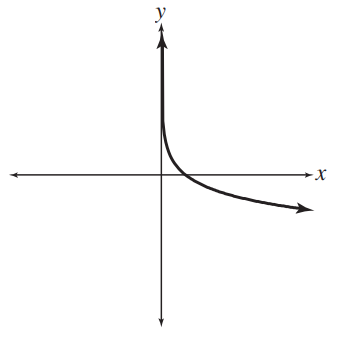
C)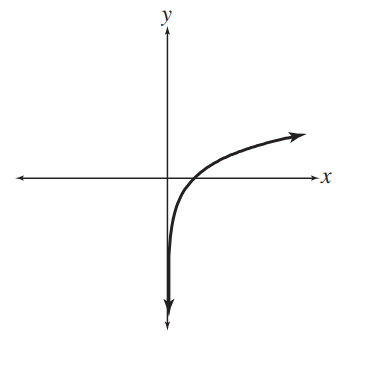
D)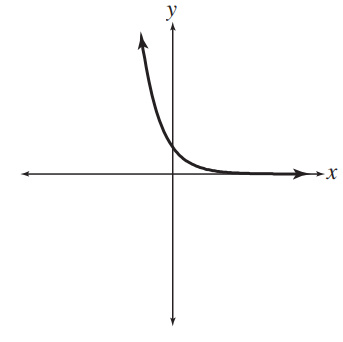
-
A)

B)

C)

D)


2
Match each equation with its graph.
-
A)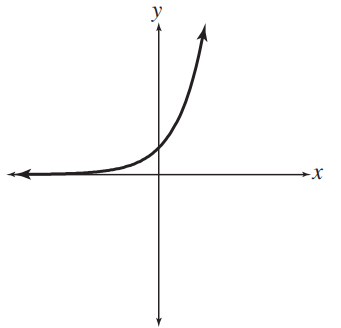
B)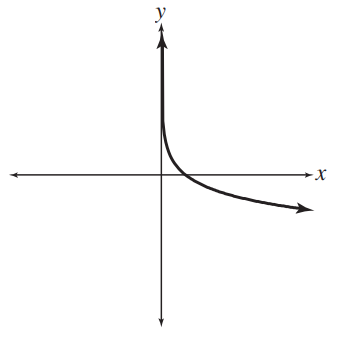
C)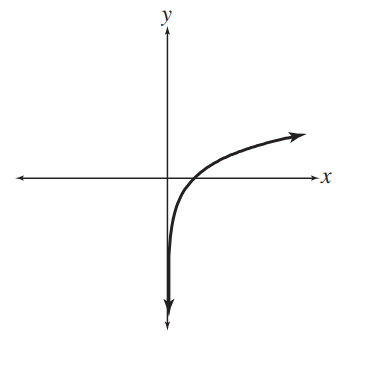
D)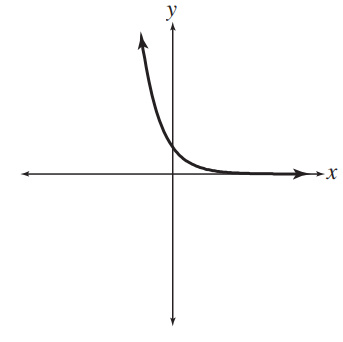
-
A)

B)

C)

D)


3
Match each equation with its graph.
-
A)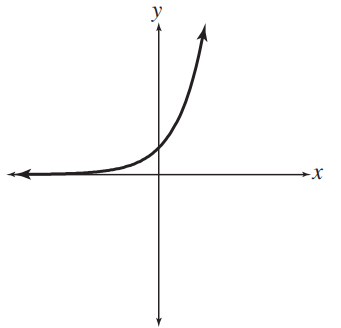
B)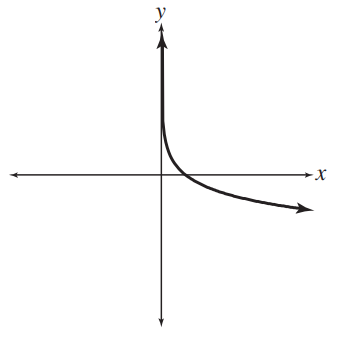
C)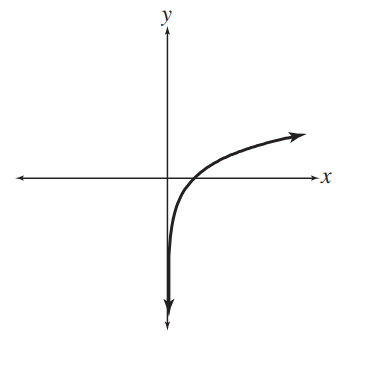
D)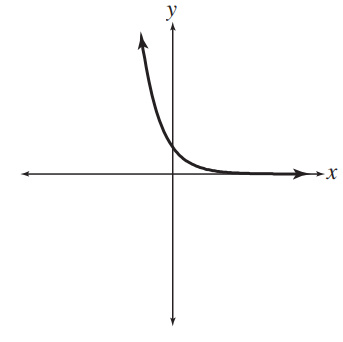
-
A)

B)

C)

D)


4
Match each equation with its graph.
-
A)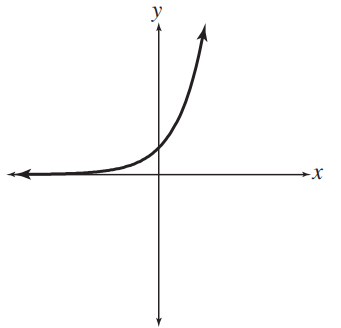
B)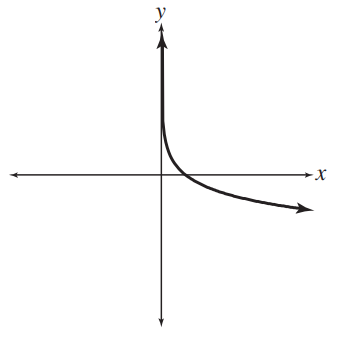
C)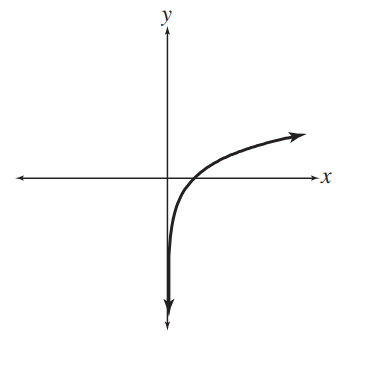
D)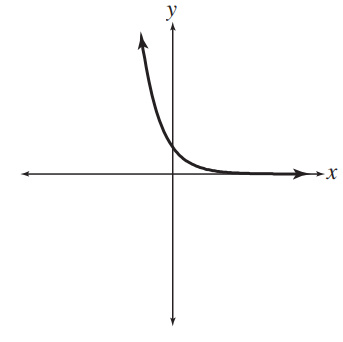
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
5
Consider the function .
(a) Graph it in the standard viewing window of your calculator.
(b) Give the domain and range of .
(c) Does the graph have an asymptote? If so, is it vertical or horizontal, and what is its equation?
(d) Find the - and -intercepts analytically and use the graph from part (a) to support your answers graphically.
(e) Find .
(a) Graph it in the standard viewing window of your calculator.
(b) Give the domain and range of .
(c) Does the graph have an asymptote? If so, is it vertical or horizontal, and what is its equation?
(d) Find the - and -intercepts analytically and use the graph from part (a) to support your answers graphically.
(e) Find .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
6
Solve the equation analytically.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
7
Suppose that is invested at 3.8 % for 20 years. Find the total amount present at the end of this time period if the interest is compounded (a) quarterly and (b) continuously.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
8
One of your friends is taking another mathematics course and tells you, "I have no idea what an expression like really means." Write an explanation of what it means, and tell how you can find an approximation for it with a calculator.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
9
Use a calculator to find an approximation of each logarithm to the nearest thousandth.
(a)
(b)
(c)
(a)
(b)
(c)

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
10
Use the power, quotient, and product properties of logarithms to write as an equivalent expression.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
11
Solve for .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
12
Consider the equation .
(a) Solve the equation analytically. If there is an extraneous value, what is it?
(b) To support the solution in part (a), we may graph and find the -intercept.
Write an expression for using the change-of-base rule with base 10 , and graph the function to support the solution from part (a).
(c) Use the graph to solve the inequality .
(a) Solve the equation analytically. If there is an extraneous value, what is it?
(b) To support the solution in part (a), we may graph and find the -intercept.
Write an expression for using the change-of-base rule with base 10 , and graph the function to support the solution from part (a).
(c) Use the graph to solve the inequality .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
13
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
14
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
15
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
16
An unstable radioactive isotope decays according to the equation where is the number of grams remaining and is the time measured in minutes. Match each question with one of the solutions A, B, C, or D.
-How long will it take for the material to decay to 2 grams?
A) Evaluate
B) Evaluate
C) Solve
D) Solve
-How long will it take for the material to decay to 2 grams?
A) Evaluate
B) Evaluate
C) Solve
D) Solve

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
17
An unstable radioactive isotope decays according to the equation where is the number of grams remaining and is the time measured in minutes. Match each question with one of the solutions A, B, C, or D.
-How many grams are remaining after 2 minutes?
A) Evaluate
B) Evaluate
C) Solve
D) Solve
-How many grams are remaining after 2 minutes?
A) Evaluate
B) Evaluate
C) Solve
D) Solve

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
18
An unstable radioactive isotope decays according to the equation where is the number of grams remaining and is the time measured in minutes. Match each question with one of the solutions A, B, C, or D.
-How many grams are remaining after 30 seconds?
A) Evaluate
B) Evaluate
C) Solve
D) Solve
-How many grams are remaining after 30 seconds?
A) Evaluate
B) Evaluate
C) Solve
D) Solve

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
19
An unstable radioactive isotope decays according to the equation where is the number of grams remaining and is the time measured in minutes. Match each question with one of the solutions A, B, C, or D.
-How long will it take for the material to decay to half its initial amount?
A) Evaluate
B) Evaluate
C) Solve
D) Solve
-How long will it take for the material to decay to half its initial amount?
A) Evaluate
B) Evaluate
C) Solve
D) Solve

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
20
When a loaf of bread is removed from the freezer to thaw, the temperature of the bread increases. Graph each of the following functions on the interval . Use for the range of . Use a graphing calculator to determine the function that best describes the temperature of the bread (in degrees Fahrenheit) minutes after it is removed from the freezer, if the initial temperature of the bread was 30 degrees Fahrenheit.
(a)
(b)
(c)
(d)
(a)
(b)
(c)
(d)

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
21
A sample of radioactive material has a half-life of about 1600 years. An initial sample weighs 18 grams.
(a) Find a formula for the decay function for this material.
(b) Find the amount left after 6400 years
(c) Find the time for the initial amount to decay to 4.5 grams.
(a) Find a formula for the decay function for this material.
(b) Find the amount left after 6400 years
(c) Find the time for the initial amount to decay to 4.5 grams.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
22
Match each equation with its graph
-
A)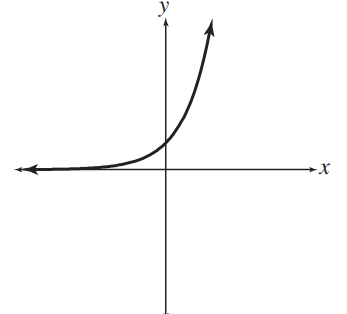
B)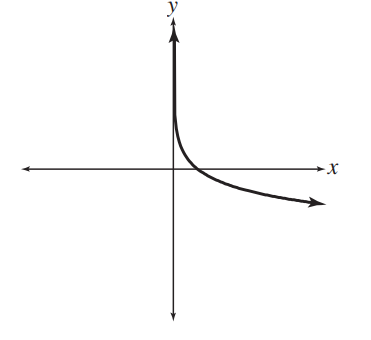
C)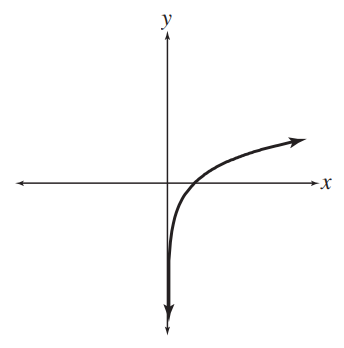
D)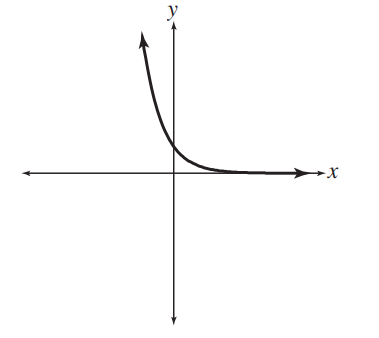
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
23
Match each equation with its graph
-
A)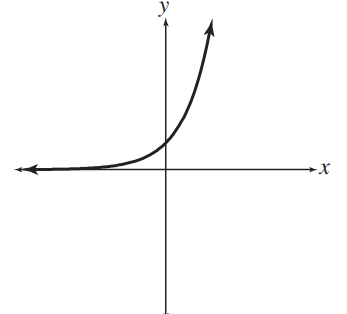
B)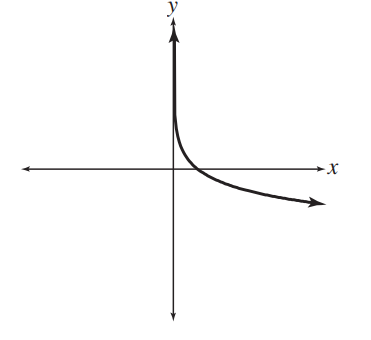
C)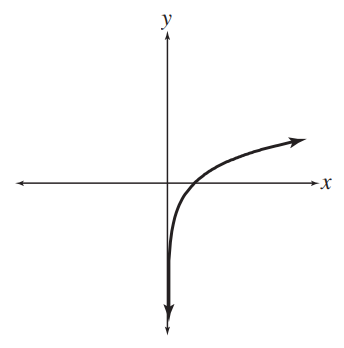
D)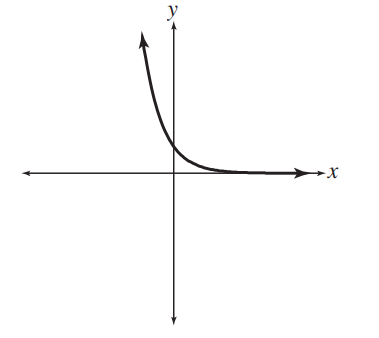
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
24
Match each equation with its graph
-
A)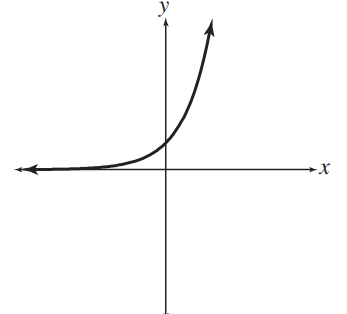
B)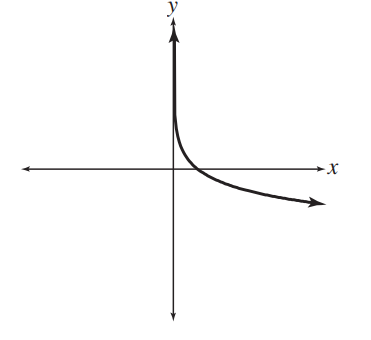
C)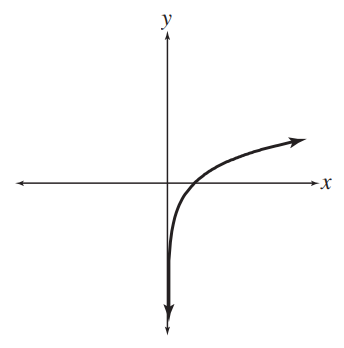
D)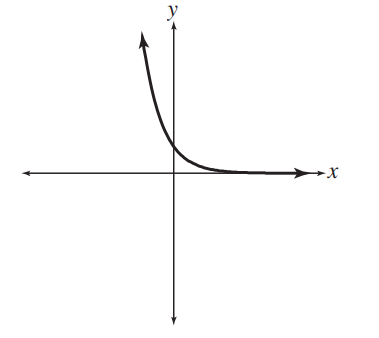
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
25
Match each equation with its graph
-
A)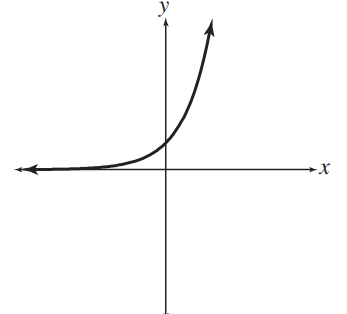
B)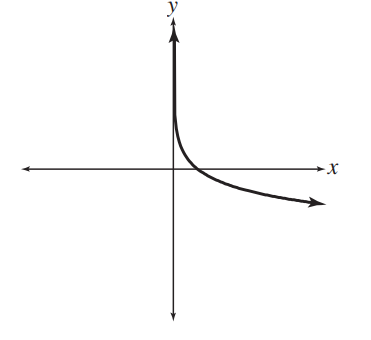
C)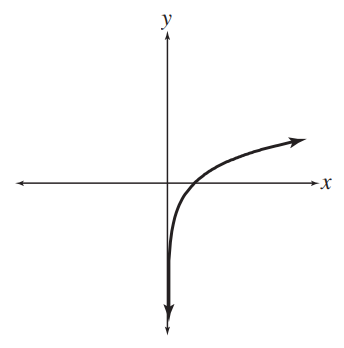
D)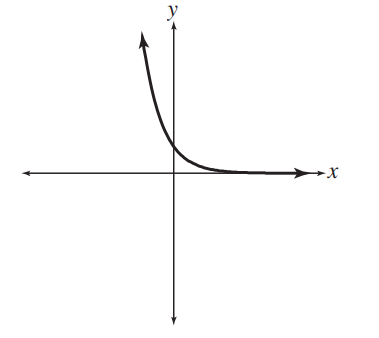
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
26
Consider the function .
(a) Graph it in the standard viewing window of your calculator.
(b) Give the domain and range of .
(c) Does the graph have an asymptote? If so, is it vertical or horizontal, and what is its equation?
(d) Find the - and -intercepts analytically and use the graph from part (a) to support your answers graphically.
(e) Find .
(a) Graph it in the standard viewing window of your calculator.
(b) Give the domain and range of .
(c) Does the graph have an asymptote? If so, is it vertical or horizontal, and what is its equation?
(d) Find the - and -intercepts analytically and use the graph from part (a) to support your answers graphically.
(e) Find .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
27
Solve the equation analytically.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
28
Suppose that is invested at for 6 years. Find the total amount present at the end of this time period if the interest is compounded (a) monthly and (b) continuously.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
29
One of your friends is taking another mathematics course and tells you "I know that the expression is undefined because the definition says that for any expression of the form can't be 0 and it can't be negative, but I don't understand why this is true." Write an explanation for your friend.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
30
Use a calculator to find an approximation of each logarithm to the nearest thousandth.
(a)
(b)
(c)
(a)
(b)
(c)

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
31
Use the power, quotient, and product properties of logarithms to write as an equivalent expression.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
32
Solve for .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
33
Consider the equation .
(a) Solve the equation analytically. If there is an extraneous value, what is it?
(b) To support the solution in part (a), we may graph and find the -intercept.
Write an expression for using the change-of-base rule with base 10, and graph the function to support the solution from part (a).
(c) Use the graph to solve the inequality .
(a) Solve the equation analytically. If there is an extraneous value, what is it?
(b) To support the solution in part (a), we may graph and find the -intercept.
Write an expression for using the change-of-base rule with base 10, and graph the function to support the solution from part (a).
(c) Use the graph to solve the inequality .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
34
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
35
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
36
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
37
A water tank has been contaminated with salt and must be flushed with pure water. The concentration of salt in the tank is given by the equation , where is the concentration of salt in milligrams per gallon and is the time, in hours, since flushing began. Match each question with one of the solutions , or .
-What is the concentration of salt after 2 hours?
A) Evaluate
B) Solve
C) Evaluate
D) Solve
-What is the concentration of salt after 2 hours?
A) Evaluate
B) Solve
C) Evaluate
D) Solve

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
38
A water tank has been contaminated with salt and must be flushed with pure water. The concentration of salt in the tank is given by the equation , where is the concentration of salt in milligrams per gallon and is the time, in hours, since flushing began. Match each question with one of the solutions , or .
-How long will it take for the concentration to reach half its initial value?
A) Evaluate
B) Solve
C) Evaluate
D) Solve
-How long will it take for the concentration to reach half its initial value?
A) Evaluate
B) Solve
C) Evaluate
D) Solve

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
39
A water tank has been contaminated with salt and must be flushed with pure water. The concentration of salt in the tank is given by the equation , where is the concentration of salt in milligrams per gallon and is the time, in hours, since flushing began. Match each question with one of the solutions , or .
-How long will it take for the concentration to reach ?
A) Evaluate
B) Solve
C) Evaluate
D) Solve
-How long will it take for the concentration to reach ?
A) Evaluate
B) Solve
C) Evaluate
D) Solve

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
40
A water tank has been contaminated with salt and must be flushed with pure water. The concentration of salt in the tank is given by the equation , where is the concentration of salt in milligrams per gallon and is the time, in hours, since flushing began. Match each question with one of the solutions , or .
-What is the concentration of salt after 30 minutes?
A) Evaluate
B) Solve
C) Evaluate
D) Solve
-What is the concentration of salt after 30 minutes?
A) Evaluate
B) Solve
C) Evaluate
D) Solve

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
41
A kiln is used to heat materials to high temperatures. Graph each of the following functions on the interval . Use for the range of . Use a graphing calculator to determine the function that best describes the temperature of the material (in degrees Fahrenheit) minutes after it is placed in the kiln, if the initial temperature of the material was 50 degrees Fahrenheit.
(a)
(b)
(c)
(d)
(a)
(b)
(c)
(d)

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
42
A sample of radioactive material has a half-life of about 1200 years. An initial sample weighs 20 grams.
(a) Find a formula for the decay function for this material.
(b) Find the amount left after 6000 years.
(c) Find the time for the initial amount to decay to 2.5 grams.
(a) Find a formula for the decay function for this material.
(b) Find the amount left after 6000 years.
(c) Find the time for the initial amount to decay to 2.5 grams.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
43
Match each equation with its graph.
-
A)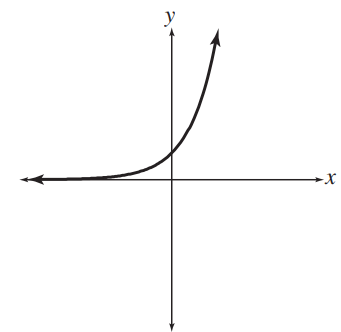
B)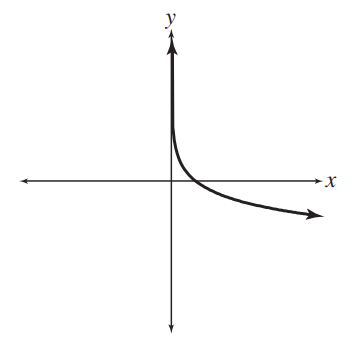
C)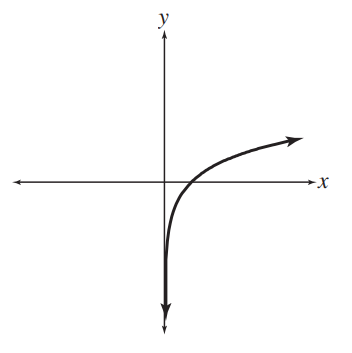
D)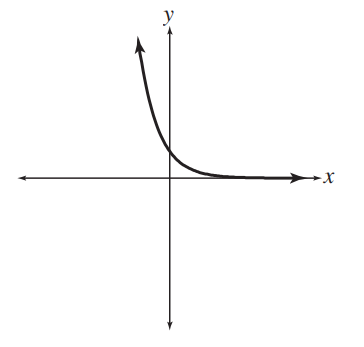
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
44
Match each equation with its graph.
-
A)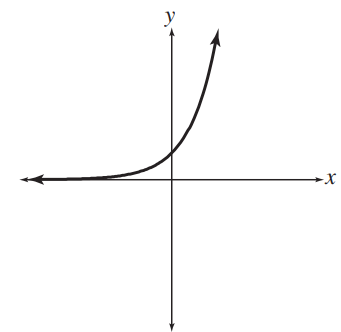
B)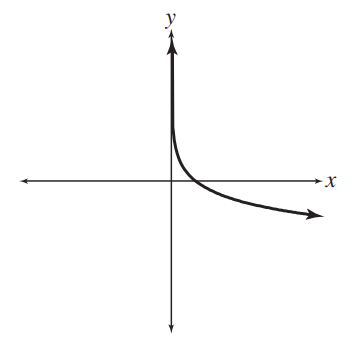
C)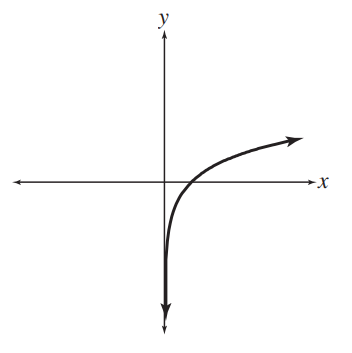
D)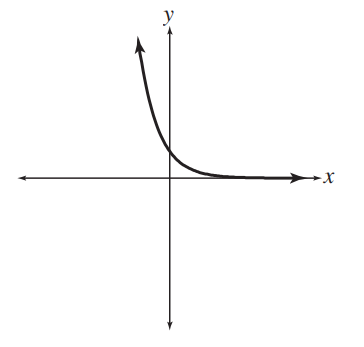
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
45
Match each equation with its graph.
-
A)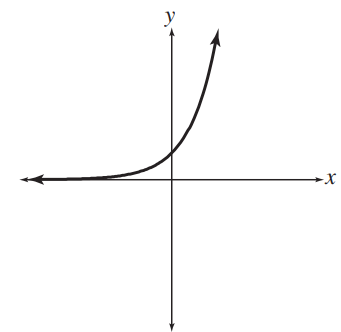
B)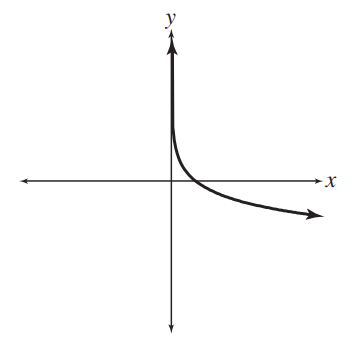
C)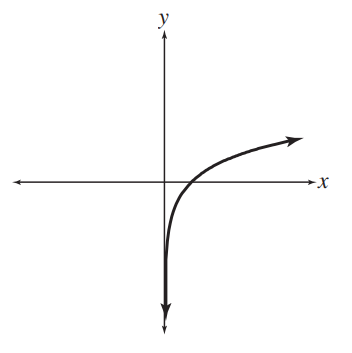
D)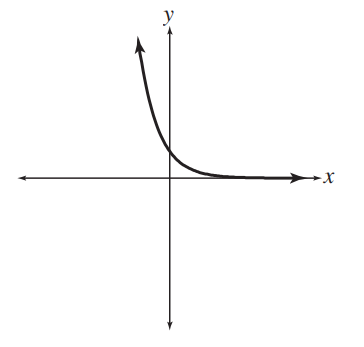
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
46
Match each equation with its graph.
-
A)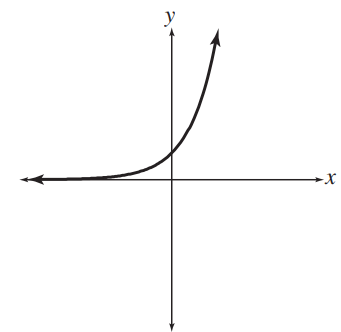
B)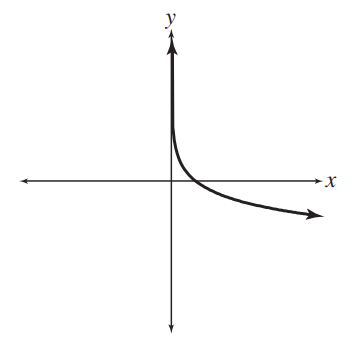
C)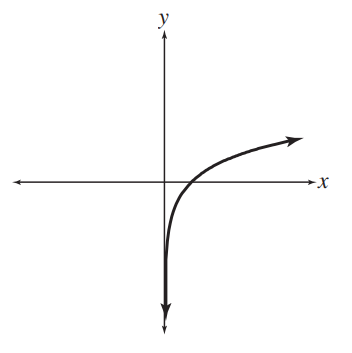
D)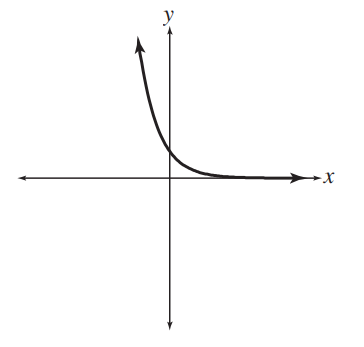
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
47
Consider the function .
(a) Graph it in the standard viewing window of your calculator.
(b) Give the domain and range of .
(c) Does the graph have an asymptote? If so, is it vertical or horizontal, and what is its equation?
(d) Find the - and -intercepts analytically and use the graph from part (a) to support your answers graphically.
(e) Find .
(a) Graph it in the standard viewing window of your calculator.
(b) Give the domain and range of .
(c) Does the graph have an asymptote? If so, is it vertical or horizontal, and what is its equation?
(d) Find the - and -intercepts analytically and use the graph from part (a) to support your answers graphically.
(e) Find .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
48
Solve the equation analytically.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
49
Suppose that is invested at for 15 years. Find the total amount present at the end of this time period if the interest is compounded (a) semiannually and (b) continuously.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
50
One of your friends is taking another mathematics course and tells you, "I have no idea what an expression like really means." Write an explanation of what it means, and tell how you can find an approximation for it with a calculator.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
51
Use a calculator to find an approximation of each logarithm to the nearest thousandth.
(a)
(b)
(c)
(a)
(b)
(c)

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
52
Use the power, quotient, and product properties of logarithms to write as an equivalent expression.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
53
Solve for .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
54
Consider the equation .
(a) Solve the equation analytically. If there is an extraneous value, what is it?
(b) To support the solution in part (a), we may graph and find the -intercept.
Write an expression for using the change-of-base rule with base 10 , and graph the function to support the solution from part (a).
(c) Use the graph to solve the inequality .
(a) Solve the equation analytically. If there is an extraneous value, what is it?
(b) To support the solution in part (a), we may graph and find the -intercept.
Write an expression for using the change-of-base rule with base 10 , and graph the function to support the solution from part (a).
(c) Use the graph to solve the inequality .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
55
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
56
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
57
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
58
The concentration of pollutants in a stream is given by , where is the amount of pollutant in grams per liter and is the distance, in kilometers, downstream from the source of the pollution. Match each question with one of the solutions A, B, C, or D.
-How far downstream is the pollutant level equal to ?
A) Solve
B) Solve
C) Evaluate
D) Evaluate
-How far downstream is the pollutant level equal to ?
A) Solve
B) Solve
C) Evaluate
D) Evaluate

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
59
The concentration of pollutants in a stream is given by , where is the amount of pollutant in grams per liter and is the distance, in kilometers, downstream from the source of the pollution. Match each question with one of the solutions A, B, C, or D.
-What is the pollutant level .02 kilometer from the source?
A) Solve
B) Solve
C) Evaluate
D) Evaluate
-What is the pollutant level .02 kilometer from the source?
A) Solve
B) Solve
C) Evaluate
D) Evaluate

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
60
The concentration of pollutants in a stream is given by , where is the amount of pollutant in grams per liter and is the distance, in kilometers, downstream from the source of the pollution. Match each question with one of the solutions A, B, C, or D.
-What is the pollutant level 500 meters from the source?
A) Solve
B) Solve
C) Evaluate
D) Evaluate
-What is the pollutant level 500 meters from the source?
A) Solve
B) Solve
C) Evaluate
D) Evaluate

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
61
The concentration of pollutants in a stream is given by , where is the amount of pollutant in grams per liter and is the distance, in kilometers, downstream from the source of the pollution. Match each question with one of the solutions A, B, C, or D.
-How far downstream is the pollutant level half the amount at the source?
A) Solve
B) Solve
C) Evaluate
D) Evaluate
-How far downstream is the pollutant level half the amount at the source?
A) Solve
B) Solve
C) Evaluate
D) Evaluate

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
62
When leftover food is placed in a refrigerator following a meal, the temperature of the food decreases. Graph each of the following functions on the interval . Use for the range of . Use a graphing calculator to determine the function that best describes the temperature of the food (in degrees Fahrenheit) minutes after it is placed in the refrigerator, if the initial temperature of the food was 90 degrees Fahrenheit.
(a)
(b)
(c)
(d)
(a)
(b)
(c)
(d)

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
63
A sample of radioactive material has a half-life of about 6000 years. An initial sample weighs 32 grams.
(a) Find a formula for the decay function for this material.
(b) Find the amount left after 30,000 years.
(c) Find the time for the initial amount to decay to .5 gram.
(a) Find a formula for the decay function for this material.
(b) Find the amount left after 30,000 years.
(c) Find the time for the initial amount to decay to .5 gram.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
64
Match each equation with its graph.
-
A)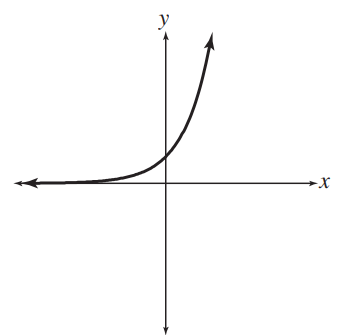
B)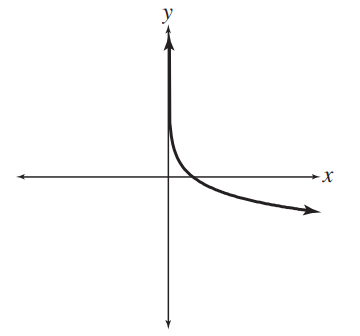
C)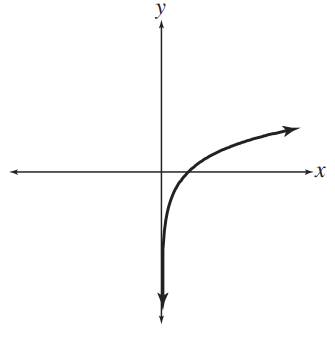
D)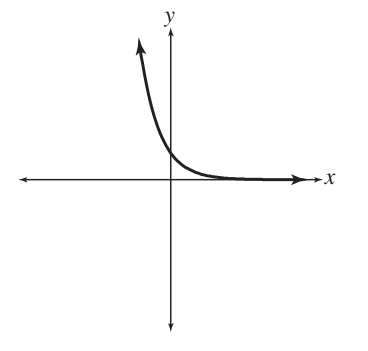
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
65
Match each equation with its graph.
-
A)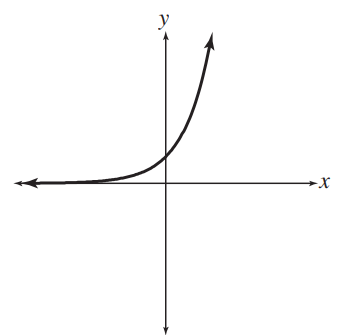
B)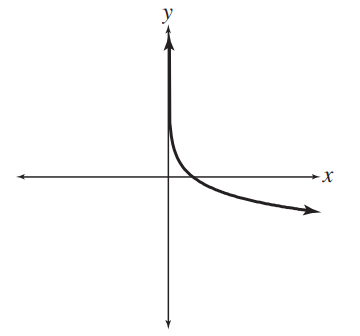
C)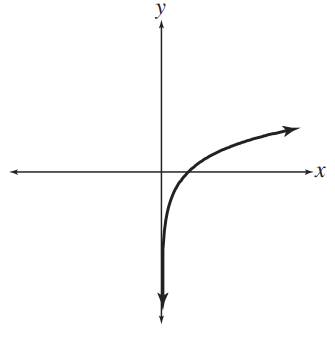
D)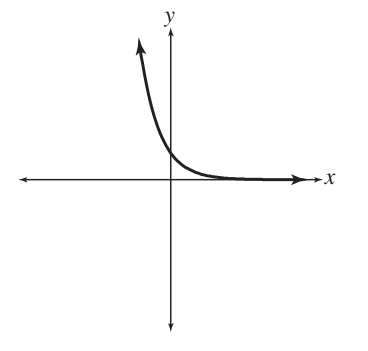
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
66
Match each equation with its graph.
-
A)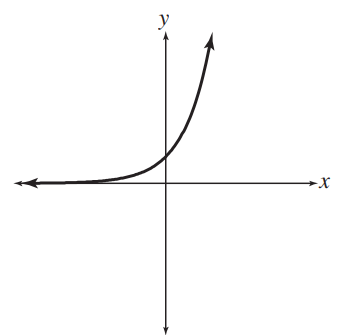
B)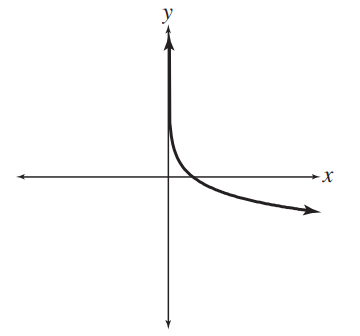
C)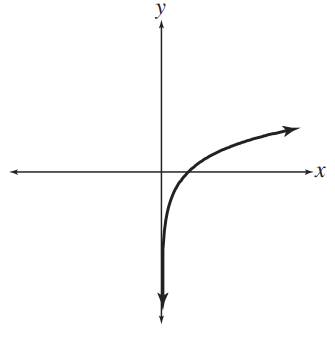
D)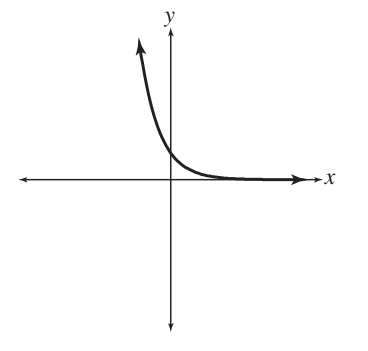
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
67
Match each equation with its graph.
-
A)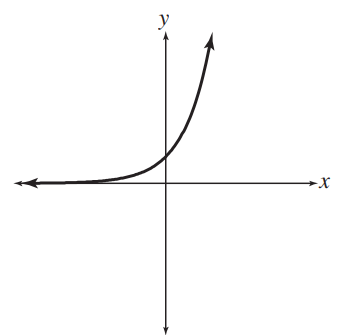
B)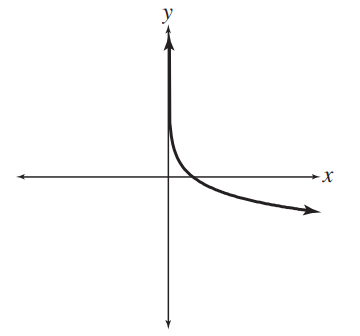
C)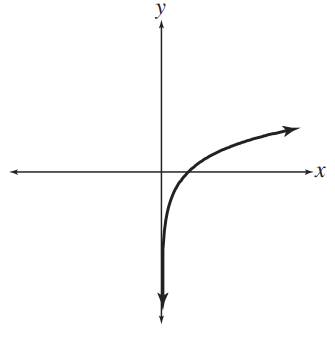
D)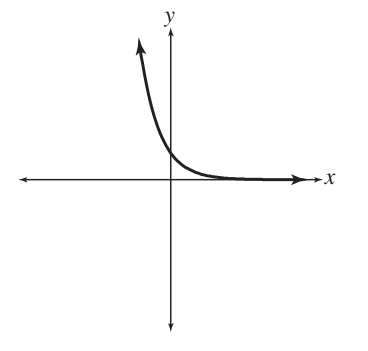
-
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
68
Consider the function .
(a) Graph it in the standard viewing window of your calculator.
(b) Give the domain and range of .
(c) Does the graph have an asymptote? If so, is it vertical or horizontal, and what is its equation?
(d) Find the - and -intercepts analytically and use the graph from part (a) to support your answers graphically.
(e) Find .
(a) Graph it in the standard viewing window of your calculator.
(b) Give the domain and range of .
(c) Does the graph have an asymptote? If so, is it vertical or horizontal, and what is its equation?
(d) Find the - and -intercepts analytically and use the graph from part (a) to support your answers graphically.
(e) Find .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
69
Solve the equation analytically.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
70
Suppose that is invested at for 12 years. Find the total amount present at the end of thime period if the interest is compounded (a) quarterly and (b) continuously.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
71
One of your friends is taking another mathematics course and tells you "I know that the expression is undefined because the definition says that for any expression of the form can't be 0 and it can't be negative, but I don't understand why this is true."' Write an explanation for your friend.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
72
Use a calculator to find an approximation of each logarithm to the nearest thousandth.
(a)
(b)
(c)
(a)
(b)
(c)

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
73
Use the power, quotient, and product properties of logarithms to write as an equivalent expression.

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
74
Solve for .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
75
Consider the equation .
(a) Solve the equation analytically. If there is an extraneous value, what is it?
(b) To support the solution in part (a), we may graph and find the -intercept.
Write an expression for using the change-of-base rule with base 10 , and graph the function to support the solution from part (a).
(c) Use the graph to solve the inequality .
(a) Solve the equation analytically. If there is an extraneous value, what is it?
(b) To support the solution in part (a), we may graph and find the -intercept.
Write an expression for using the change-of-base rule with base 10 , and graph the function to support the solution from part (a).
(c) Use the graph to solve the inequality .

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
76
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
77
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
78
solve each equation. Give the solution set (a) with an exact value and then (b) with an approximation to the nearest thousandth.
-
-

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
79
The atmospheric pressure at a given altitude is given by , where is the atmospheric pressure in pounds per square inch and is the altitude, in feet. Match each question with one of the solutions A, B, C, or D.
-What is the atmospheric pressure at an altitude of 10 feet?
A) Solve
B) Evaluate
C) Solve
D) Evaluate
-What is the atmospheric pressure at an altitude of 10 feet?
A) Solve
B) Evaluate
C) Solve
D) Evaluate

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck
80
The atmospheric pressure at a given altitude is given by , where is the atmospheric pressure in pounds per square inch and is the altitude, in feet. Match each question with one of the solutions A, B, C, or D.
-What is the atmospheric pressure at an altitude of 10 yards?
A) Solve
B) Evaluate
C) Solve
D) Evaluate
-What is the atmospheric pressure at an altitude of 10 yards?
A) Solve
B) Evaluate
C) Solve
D) Evaluate

Unlock Deck
Unlock for access to all 84 flashcards in this deck.
Unlock Deck
k this deck



