Deck 13: Introduction to Prescriptive Analytics
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/49
Play
Full screen (f)
Deck 13: Introduction to Prescriptive Analytics
1
When using Excel to model uncertainty, the RAND function and BINOM.INV functions are used. What key is used to redraw new observations, changing the output?
A) Return key
B) ALT4
C) F4
D) F9
A) Return key
B) ALT4
C) F4
D) F9
F9
2
A CNBC study in 2017 determined only 35% of adults have zero to only a couple of hundred dollars in a savings account. With n = 4 and p = 0.43, using the binomial probability distribution, what is the mean and the standard deviation of the population? Hint: = np(1 - p)
A) 4 and 0.98 respectively
B) 1.72 and 0.9902 respectively
C) 2 and 1.74 respectively
D) 1.8 and 0.9804 respectively
A) 4 and 0.98 respectively
B) 1.72 and 0.9902 respectively
C) 2 and 1.74 respectively
D) 1.8 and 0.9804 respectively
1.72 and 0.9902 respectively
3
A CNBC study in 2017 determined only 35% of adults have zero to only a couple of hundred dollars in a savings account. With n = 5 and p = 0.35, using the binomial probability distribution, what is the mean and the standard deviation of the population? Hint: = 
A) 5 and 1.13 respectively
B) 1.75 and 1.0665 respectively
C) 2.50 and 1.1705 respectively
D) 1.55 and 1.1375 respectively

A) 5 and 1.13 respectively
B) 1.75 and 1.0665 respectively
C) 2.50 and 1.1705 respectively
D) 1.55 and 1.1375 respectively
1.75 and 1.0665 respectively
4
Lane Accessories has a growing demand for custom Apple watch designer bands. The current manufacturing costs are $140 per hour to operate, for each hour of operation, 205 black band designs and 180 multi-color designs are completed. However, Lane found a new larger manufacturing space that will cost $160 per hour and produce 325 black design bands and 289 multi-color bands per hour completed. Lane has newly placed orders to restock other retail outlets nationwide for 7,000 black band designs and 5,000 multi-color bands. Because Lane is out of inventory, she needs to decide how many hours to operate each facility to fulfill orders while minimizing cost. Formulate the minimization function for production costs.
A) Production Costs = 140x1 + 160x2
B) Production Costs = (140x1 + 385) + (160x2 + 614)
C) Production Costs = 385x1 + 614x2
D) Production Costs = (140x1 + 7,000) + (160x2 + 5,000)
A) Production Costs = 140x1 + 160x2
B) Production Costs = (140x1 + 385) + (160x2 + 614)
C) Production Costs = 385x1 + 614x2
D) Production Costs = (140x1 + 7,000) + (160x2 + 5,000)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
5
Lane Accessories has a growing demand for custom Apple watch designer bands. The current manufacturing costs are $120 per hour to operate, for each hour of operation, 210 black band designs and 180 multi-color designs are completed. However, Lane found a new larger manufacturing space that will cost $160 per hour and produce 325 black design bands and 289 multi-color bands per hour completed. Lane has newly placed orders to restock other retail outlets nationwide for 6,000 black band designs and 5,000 multi-color bands. Because Lane is out of inventory, she needs to decide how many hours to operate each facility to fulfill orders while minimizing cost. Formulate the minimization function for production costs.
A) Production Costs = 120x1 + 160x2
B) Production Costs = (120x1 + 390) + (160x2 + 614)
C) Production Costs = 390x1 + 614x2
D) Production Costs = (120x1 + 6,000) + (160x2 + 5,000)
A) Production Costs = 120x1 + 160x2
B) Production Costs = (120x1 + 390) + (160x2 + 614)
C) Production Costs = 390x1 + 614x2
D) Production Costs = (120x1 + 6,000) + (160x2 + 5,000)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
6
Which one of the following is not an essential component of linear programming?
A) random
B) an objective function
C) decision variables
D) constraints
A) random
B) an objective function
C) decision variables
D) constraints

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
7
Lane Accessories has a growing demand for custom Apple watch designer bands. The current manufacturing costs are $130 per hour to operate, for each hour of operation, 245 black band designs and 180 multi-color designs are completed. However, Lane found a new larger manufacturing space that will cost $160 per hour and produce 325 black design bands and 289 multi-color bands per hour completed. Lane has newly placed orders to restock other retail outlets nationwide for 5,000 black band designs and 7,000 multi-color bands. Because Lane is out of inventory, she needs to decide how many hours to operate each facility to fulfill orders while minimizing cost. Formulate the orders for black design bands.
A) 245x1+325x2 5,000x3
B) 245x1 + 325x2 5,000
C) 180x1 + 245x2 5,000
D) 180x1 + 325x2 7,000
A) 245x1+325x2 5,000x3
B) 245x1 + 325x2 5,000
C) 180x1 + 245x2 5,000
D) 180x1 + 325x2 7,000

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
8
Lane Accessories has a growing demand for custom Apple watch designer bands. The current manufacturing costs are $120 per hour to operate, for each hour of operation, 210 black band designs and 180 multi-color designs are completed. However, Lane found a new larger manufacturing space that will cost $160 per hour and produce 325 black design bands and 289 multi-color bands per hour completed. Lane has newly placed orders to restock other retail outlets nationwide for 6,000 black band designs and 5,000 multi-color bands. Because Lane is out of inventory, she needs to decide how many hours to operate each facility to fulfill orders while minimizing cost. Formulate the orders for black design bands.
A) 210x1 + 325x2 6,000x3
B) 210x1 + 325x2 6,000
C) 180x1 + 210x2 6,000
D) 180x1 + 325x2 5,000
A) 210x1 + 325x2 6,000x3
B) 210x1 + 325x2 6,000
C) 180x1 + 210x2 6,000
D) 180x1 + 325x2 5,000

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
9
Sampson Ltd produces two products that can be produced on either of two machines. Each month, only 500 hours of time are available on each machine. The time required to produce each item by hour and machine is:
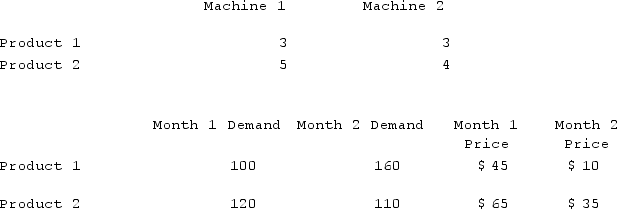
The demand and price point for each product that customers are willing to pay are above. The company goal is to maximize revenue from sales from the next two months. Based on the provided information, how many constraints does this problem have excluding the non-negativity constraints?
A) 2 total constraints
B) 4 total constraints
C) 6 total constraints
D) 8 total constraints

The demand and price point for each product that customers are willing to pay are above. The company goal is to maximize revenue from sales from the next two months. Based on the provided information, how many constraints does this problem have excluding the non-negativity constraints?
A) 2 total constraints
B) 4 total constraints
C) 6 total constraints
D) 8 total constraints

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
10
By viewing the following Excel snapshot, in Excel Solver what would be entered to formulate the Objective Function for E3?
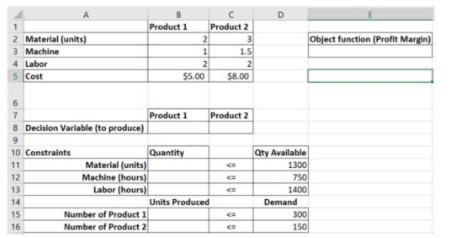
A) =SUM(B5:D11,C8:D11)
B) =SUMPRODUCT(B5*C5) + (B8*C8)
C) =SUMPRODUCT(B5+B8) * (C5+C8)
D) =SUMPRODUCT(B5*B8) + (C5*C8)

A) =SUM(B5:D11,C8:D11)
B) =SUMPRODUCT(B5*C5) + (B8*C8)
C) =SUMPRODUCT(B5+B8) * (C5+C8)
D) =SUMPRODUCT(B5*B8) + (C5*C8)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
11
By viewing the following Excel snapshot, in Excel Solver what would be entered to formulate B11, Material (units) for Quantity?
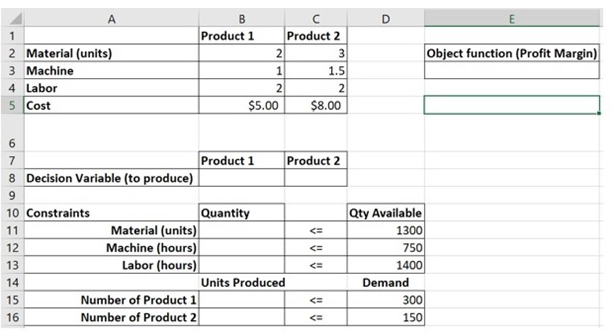
A) =SUMPRODUCT(B2+B8) * (C2+C8)
B) =SUMPRODUCT(B2*B8) + (C2*C8)
C) =SUMPRODUCT(B2*D15) + (C2*D16)
D) =SUMPRODUCT(B2*D15) * (C2*D16)

A) =SUMPRODUCT(B2+B8) * (C2+C8)
B) =SUMPRODUCT(B2*B8) + (C2*C8)
C) =SUMPRODUCT(B2*D15) + (C2*D16)
D) =SUMPRODUCT(B2*D15) * (C2*D16)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
12
Based on the following sensitivity results on constraints, what is the optimal LP solution for Products 1 and 2? 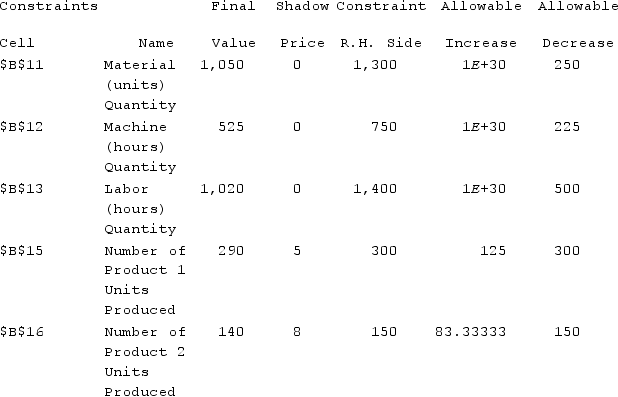
A) Product 1: 290 units and Product 2: 234 units
B) Product 1: 425 units and Product 2: 140 units
C) Product 1: 290 units and Product 2: 140 units
D) Product 1: 125 units and Product 2: 84 units

A) Product 1: 290 units and Product 2: 234 units
B) Product 1: 425 units and Product 2: 140 units
C) Product 1: 290 units and Product 2: 140 units
D) Product 1: 125 units and Product 2: 84 units

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
13
Based on the following sensitivity results on constraints, what is the optimal LP solution for Products 1 and 2? 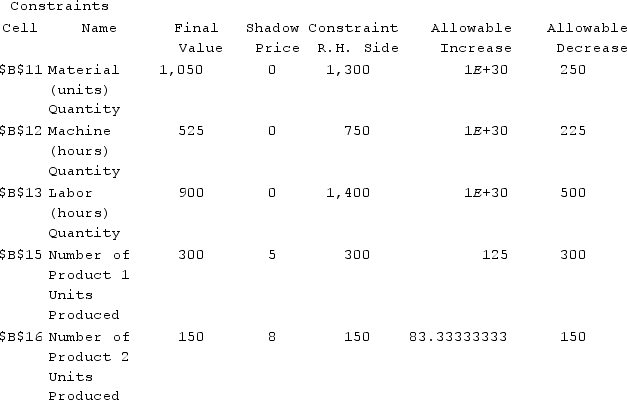
A) Product 1: 300 units and Product 2: 234 units
B) Product 1: 425 units and Product 2: 150 units
C) Product 1: 300 units and Product 2: 150 units
D) Product 1: 125 units and Product 2: 84 units

A) Product 1: 300 units and Product 2: 234 units
B) Product 1: 425 units and Product 2: 150 units
C) Product 1: 300 units and Product 2: 150 units
D) Product 1: 125 units and Product 2: 84 units

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
14
Based on the following sensitivity results on constraints, how would an increase in machine hours impact the LP solution? 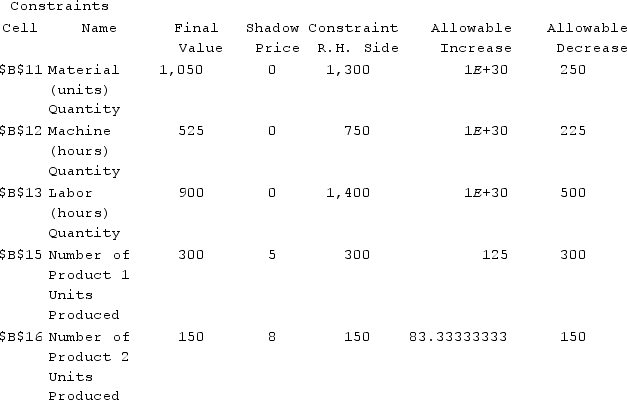
A) The hours can have an infinite increase without altering the solution.
B) The hours can only decrease without altering the solution.
C) The hours can have an increase by 750 units before price decreases.
D) The hours cannot be predicted with the information presented.

A) The hours can have an infinite increase without altering the solution.
B) The hours can only decrease without altering the solution.
C) The hours can have an increase by 750 units before price decreases.
D) The hours cannot be predicted with the information presented.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
15
Based on the following Variable cells segment of a Solver Sensitivity Report, what range does Product 1 per-unit profit fall between at 400 units? 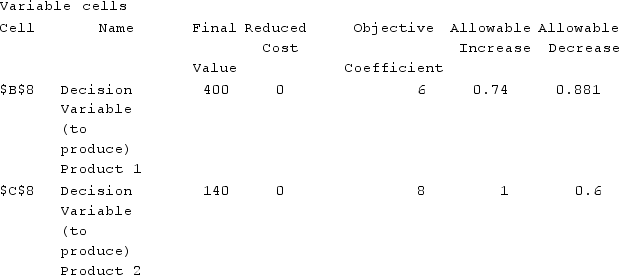
A) The range is between 0.881 and 0.74.
B) The range is between 0.881 and 6.74.
C) The range is between 5.119 and 6.74.
D) The range can only be determined with more details from the sensitivity report.

A) The range is between 0.881 and 0.74.
B) The range is between 0.881 and 6.74.
C) The range is between 5.119 and 6.74.
D) The range can only be determined with more details from the sensitivity report.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
16
Based on the following Variable cells segment of a Solver Sensitivity Report, what range does Product 1 per-unit profit fall between at 300 units? 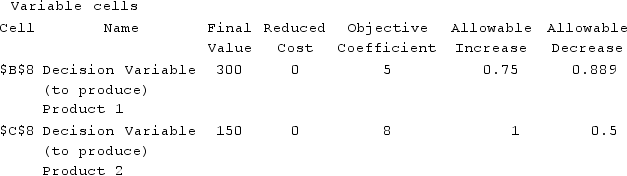
A) The range is between 0.889 and 0.75.
B) The range is between 0.889 and 5.75.
C) The range is between 4.111 and 5.75.
D) The range can only be determined with more details from the sensitivity report.

A) The range is between 0.889 and 0.75.
B) The range is between 0.889 and 5.75.
C) The range is between 4.111 and 5.75.
D) The range can only be determined with more details from the sensitivity report.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
17
The Monte Carlo simulation is also known as
A) stochastic & probabilistic.
B) stochastic & deterministic.
C) deterministic.
D) probabilistic & deterministic.
A) stochastic & probabilistic.
B) stochastic & deterministic.
C) deterministic.
D) probabilistic & deterministic.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
18
In a linear programming model, the parameter values in an objective function are referred to as the
A) objective function coefficients.
B) parameter function.
C) constraint coefficient.
D) quantitative function.
A) objective function coefficients.
B) parameter function.
C) constraint coefficient.
D) quantitative function.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
19
At Taste of Thyme coffee shop a dirty chai latte creates a profit point of $2.83 for a small and $3.36 for a large. In a month, 225 small lattes were sold and 285 large lattes. As a fast growing demand item with fall approaching, the demand is estimated at 400 and 420 per month. The amount of machine time needed to produce the lattes is 5 minutes and 7 minutes each or for a month, 16.47 hours a month for a small and 33.25 hours a month for a large. What is the maximization function for profit?
A) Profit = 400x1 + 420x2
B) Profit = 2.83x1 + 3.36x2
C) Profit = 16.47x1 + 33.25x2
D) Profit = 2.48x1 + 3.06x2
A) Profit = 400x1 + 420x2
B) Profit = 2.83x1 + 3.36x2
C) Profit = 16.47x1 + 33.25x2
D) Profit = 2.48x1 + 3.06x2

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
20
At Taste of Thyme coffee shop a dirty chai latte creates a profit point of $2.85 for a small and $3.80 for a large. In a month, 200 small lattes were sold and 285 large lattes. As a fast growing demand item with fall approaching, the demand is estimated at 400 and 420 per month. The amount of machine time needed to produce the lattes is 5 minutes and 7 minutes each or for a month, 16.67 hours a month for a small and 33.25 hours a month for a large. What is the maximization function for profit?
A) Profit = 400x1 + 420x2
B) Profit = 2.85x1 + 3.80x2
C) Profit = 16.67x1 + 33.25x2
D) Profit = 2.50x1 + 3.50x2
A) Profit = 400x1 + 420x2
B) Profit = 2.85x1 + 3.80x2
C) Profit = 16.67x1 + 33.25x2
D) Profit = 2.50x1 + 3.50x2

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
21
At Taste of Thyme coffee shop a dirty chai latte creates a profit point of $2.79 for a small and $3.33 for a large. In a month, 240 small lattes were sold and 285 large lattes. As a fast growing demand item with fall approaching, the demand is estimated at 400 and 420 per month. The allotted machine time for both lattes is 110 hours. The amount of machine time needed to produce the lattes is 7 minutes and 7 minutes each or for a month, 16.67 hours a month for a small and 34.16 hours a month for a large. What is the corresponding parameters formulation for machine time?
A) 7x1 + 7x2 110
B) 16.67x1 + 34.16x2 110
C) 2.79x1 + 3.38x2 110
D) 16.67x1 + 34.16x2 110
A) 7x1 + 7x2 110
B) 16.67x1 + 34.16x2 110
C) 2.79x1 + 3.38x2 110
D) 16.67x1 + 34.16x2 110

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
22
At Taste of Thyme coffee shop a dirty chai latte creates a profit point of $2.85 for a small and $3.80 for a large. In a month, 200 small lattes were sold and 285 large lattes. As a fast growing demand item with fall approaching, the demand is estimated at 400 and 420 per month. The allotted machine time for both lattes is 90 hours. The amount of machine time needed to produce the lattes is 5 minutes and 7 minutes each or for a month, 16.67 hours a month for a small and 33.25 hours a month for a large. What is the corresponding parameters formulation for machine time?
A) 5x1 + 7x2 90
B) 16.67x1 + 33.25x2 90
C) 2.85x1 + 3.85x2 90
D) 16.67x1 + 33.25x2 90
A) 5x1 + 7x2 90
B) 16.67x1 + 33.25x2 90
C) 2.85x1 + 3.85x2 90
D) 16.67x1 + 33.25x2 90

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
23
Deterministic process is
A) created by random variable selection.
B) created by constraint identification.
C) a Monte Carlo simulation.
D) a precise estimation based on known variables.
A) created by random variable selection.
B) created by constraint identification.
C) a Monte Carlo simulation.
D) a precise estimation based on known variables.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
24
The first step in performing linear programming is
A) to generate random numbers.
B) to formulate a problem into a series of mathematical expressions.
C) to create intervals.
D) to analyze the file for patterns.
A) to generate random numbers.
B) to formulate a problem into a series of mathematical expressions.
C) to create intervals.
D) to analyze the file for patterns.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
25
Martin is evaluating 4 projects for potential capital funding. However, he only has $150,000 for Year one, and $55,000 for the remaining years to invest. Unfortunately, he cannot receive any additional funds unspent from year to year. Based on the following data, what is the constraint for the Year 1 integer programming formulation? 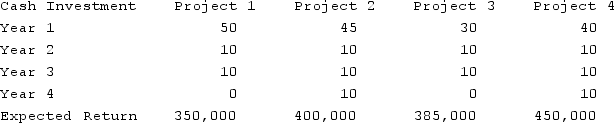
A) = i=14cixi
B) = i=14ai1xi ? 150,000
C) = i=14aixi ? 55,000
D) = i=14ai1xi ? 165,000

A) = i=14cixi
B) = i=14ai1xi ? 150,000
C) = i=14aixi ? 55,000
D) = i=14ai1xi ? 165,000

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
26
Martin is evaluating 4 projects for potential capital funding. However, he only has $90,000 for Year one, and $50,000 for the remaining years to invest. Unfortunately, he cannot receive any additional funds unspent from year to year. Based on the following data, what is the constraint for the Year 1 integer programming formulation? 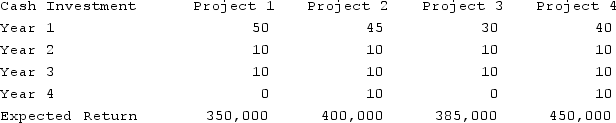
A) <p>=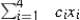
B) <p>=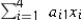 90,000
90,000
C) <p>=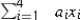 50,000
50,000
D) <p>=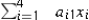 165,000
165,000

A) <p>=
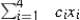
B) <p>=
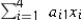 90,000
90,000C) <p>=
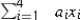 50,000
50,000D) <p>=
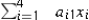 165,000
165,000
Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
27
Martin is evaluating 4 projects for potential capital funding. However, he only has $100,000 for Year one, and $45,000 for the remaining three years to invest. Each of the four projects is projected to generate an expected return of $350,000, $400,000, $385,000, and $450,000 respectively. Based on the summary information provided, what is the Expected Return objective function?
A) Expected Return = i=14cixi
B) Expected Return = 100x1 + 45x2
C) Expected Return = i=x14cixi
D) Expected Return = i=1ai1xi6i
A) Expected Return = i=14cixi
B) Expected Return = 100x1 + 45x2
C) Expected Return = i=x14cixi
D) Expected Return = i=1ai1xi6i

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
28
Martin is evaluating 4 projects for potential capital funding. However, he only has $90,000 for Year one, and $50,000 for the remaining three years to invest. Each of the four projects is projected to generate an expected return of $350,000, $400,000, $385,000, and $450,000 respectively. Based on the summary information provided, what is the Expected Return objective function?
A) <p>Expected Return =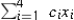
B) Expected Return = 90x1 + 50x2
C) <p>Expected Return =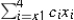
D) <p>Expected Return =
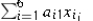
A) <p>Expected Return =
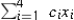
B) Expected Return = 90x1 + 50x2
C) <p>Expected Return =
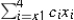
D) <p>Expected Return =
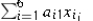

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
29
The following Excel Solver Results show capital projects selected for investment. When constructing the Solver parameters, the "subject to the constraints" need to be set. What two constraints are required?{MISSING IMAGE}
A) $G$8 AND B10:E10 = binary
B) B8:E8 = binary AND F2:F5 <= I2:I5
C) B10:E10 = binary AND F2:F5 => I2:I5
D) B10:E10 = binary AND F2:F5 <= I2:I5
A) $G$8 AND B10:E10 = binary
B) B8:E8 = binary AND F2:F5 <= I2:I5
C) B10:E10 = binary AND F2:F5 => I2:I5
D) B10:E10 = binary AND F2:F5 <= I2:I5

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
30
In Excel, the worksheet needs to be prepared prior to running the Solver. As such, the total (total return), cell G8, shows the total of the expected return for approved projects. What is the formula that must be set in G8 to capture the results accurately?
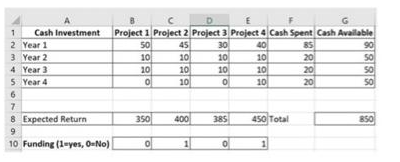
A) =SUM(B8:E8 * B10:E10)
B) =SUMPRODUCT(B8:E8, B10:E10)
C) =SUMPRODUCT(B8:E8 * B10:E10)
D) =SUMPRODUCT(B8:E8, G2:G5)

A) =SUM(B8:E8 * B10:E10)
B) =SUMPRODUCT(B8:E8, B10:E10)
C) =SUMPRODUCT(B8:E8 * B10:E10)
D) =SUMPRODUCT(B8:E8, G2:G5)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
31
Be it a capital project selection or a transportation, a manager's goals are what drives the projects. In IP the manager's goal translates to the ______ function in programming.
A) objective
B) fractional
C) matrix
D) transport
A) objective
B) fractional
C) matrix
D) transport

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
32
Watkins Trucking has two warehouses that service four retail locations for Harmons Hardware. The first warehouse supplies up to 180 pallets a week and the second warehouse supplies 300 pallets a week. The orders received weekly from Harmons are 75 pallets for the 1st location and 50 pallets for locations 2, 3, & 4. Formulate the objective function for the total shipping costs. 
A) <p>Minimize: Total Shipping Cost =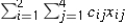
B) <p>Minimize: Total Shipping Cost =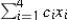
C) <p>Maximize: Total Shipping Cost =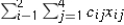
D) <p>Maximize: Total Shipping Cost =
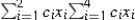

A) <p>Minimize: Total Shipping Cost =
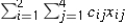
B) <p>Minimize: Total Shipping Cost =
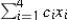
C) <p>Maximize: Total Shipping Cost =
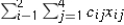
D) <p>Maximize: Total Shipping Cost =
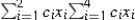

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
33
When using R, how do you store shipping costs? 
A) >unit.costs <- matrix(2.81, 3.32, 2.90, 2.88)
B) >unit.costs <- unit.costs(2.81, 3.32, 2.90, 2.88)
C) >unit.costs <- matrix(c(2.81, 3.32, 2.90, 2.88), nrow=2, byrow=TRUE)
D) >unit.costs <- unit.costs(2.81, 3.32, 2.90, 2.88), nrow=2, byrow=TRUE)

A) >unit.costs <- matrix(2.81, 3.32, 2.90, 2.88)
B) >unit.costs <- unit.costs(2.81, 3.32, 2.90, 2.88)
C) >unit.costs <- matrix(c(2.81, 3.32, 2.90, 2.88), nrow=2, byrow=TRUE)
D) >unit.costs <- unit.costs(2.81, 3.32, 2.90, 2.88), nrow=2, byrow=TRUE)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
34
When using R, how do you store shipping costs? 
A) >unit.costs <- matrix(2.85, 3.32, 3.10, 2.90)
B) >unit.costs <- unit.costs(2.85, 3.32, 3.10, 2.90)
C) >unit.costs <- matrix(c(2.85, 3.32, 3.10, 2.90), nrow=2, byrow=TRUE)
D) >unit.costs <- unit.costs(2.85, 3.32, 3.10, 2.90), nrow=2, byrow=TRUE)

A) >unit.costs <- matrix(2.85, 3.32, 3.10, 2.90)
B) >unit.costs <- unit.costs(2.85, 3.32, 3.10, 2.90)
C) >unit.costs <- matrix(c(2.85, 3.32, 3.10, 2.90), nrow=2, byrow=TRUE)
D) >unit.costs <- unit.costs(2.85, 3.32, 3.10, 2.90), nrow=2, byrow=TRUE)

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
35
In a LP solution, constraints with slack are called ________ constraints.
A) binding
B) nonbinding
C) surplus
D) overage
A) binding
B) nonbinding
C) surplus
D) overage

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
36
If x1 and x2 are outside of the feasibility region, what does that mean?
A) The first constraint is a valid solution to be considered.
B) Solutions outside of the feasibility region can be considered as viable.
C) The farthest outside the feasibility region is the optimal solution.
D) Solutions outside of the feasibility region cannot be considered.
A) The first constraint is a valid solution to be considered.
B) Solutions outside of the feasibility region can be considered as viable.
C) The farthest outside the feasibility region is the optimal solution.
D) Solutions outside of the feasibility region cannot be considered.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
37
As a manager, Mike uses linear programming (LP) to formulate a problem into a series of mathematical expressions. __________ refers to the choices or alternatives Mike selects to minimize or maximize the value of his goals.
A) Objective function
B) Decision variables
C) Optimization
D) Feasible region
A) Objective function
B) Decision variables
C) Optimization
D) Feasible region

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
38
Numerical values that are associated with objective function, decision variables, and constraints are called _________.
A) parameters
B) binary
C) value
D) assumptions
A) parameters
B) binary
C) value
D) assumptions

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
39
An integer programming model which involves selection of investment is classified as what type of problem?
A) clustering problem
B) transportation problem
C) integer problem
D) capital budgeting problem
A) clustering problem
B) transportation problem
C) integer problem
D) capital budgeting problem

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
40
In a transportation model, when constructing the constraints, the points of demand are classified as _________.
A) transportation
B) destinations
C) origin
D) goal
A) transportation
B) destinations
C) origin
D) goal

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
41
Prescriptive analytics is the process of using decision analysis tools to improve decision making.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
42
The Monte Carlo simulation relies solely on average values to capture risk and uncertainty.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
43
Binomial and Poisson distributions are the two most relevant probability distributions for Monte Carlo simulations.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
44
Continuous uniform distribution, also known as the rectangular distribution, captures the time that elapses between occurrences.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
45
Integer Programming, like Linear Programming, requires the analyst to round to the nearest integer for optimization.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
46
In Excel, random observations can be generated, however random seed cannot be set, requiring values to be cut and pasted.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
47
The objective function is a mathematical representation of an objective.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
48
Constraints with slack or surplus in the linear programming solutions are called binding constraints.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck
49
The shadow price, or dual price, of a constraint is an indication of the change in the optimized value of an objective function in a one unit change in a binding constraint.

Unlock Deck
Unlock for access to all 49 flashcards in this deck.
Unlock Deck
k this deck



