Deck 3: Exploring Data: Central Tendency
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/103
Play
Full screen (f)
Deck 3: Exploring Data: Central Tendency
1
A distribution cannot have two means.
True
2
A characteristic of the mean is that is a minimum.
False
3
For continuous data that are positively skewed, the mean is usually larger than the median
True
4
The location of the median is found using the formula, .

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
5
The mean is an appropriate measure of central tendency with open-ended distributions.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
6
If a distribution is skewed, the mean may be a misleading measure of central tendency.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
7
The mode is the midpoint between the largest score and the smallest score.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
8
The overall mean of 2 samples with different Ns is the sum of the two means divided by 2.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
9
Summing the scores and dividing by N produces the median.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
10
A distribution cannot have two medians.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
11
A characteristic of the mean is that = 0.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
12
For continuous data that are positively skewed, the mean is usually smaller than the median.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
13
The location of the median is found using the formula, .

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
14
The mean is an appropriate measure of central tendency for skewed distributions.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
15
The mode can be found for skewed distributions and open-ended distributions.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
16
The median is the midpoint between the two scores that establish the range.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
17
The overall mean of 2 samples with different Ns requires the formula for the weighted mean.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
18
For ordinal data, a median is an appropriate measure of central tendency.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
19
One way to find the median is to sum the scores and divide by N.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
20
A distribution cannot have two modes.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
21
A characteristic of the mean is that is a minimum but greater than 0.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
22
For continuous data that are negatively skewed, the mean is usually smaller than the median.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
23
The location of the median is found using the formula, .

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
24
The central tendency statistic that cannot be calculated on an open-ended distribution is the mean.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
25
The mean is appropriate for all four scales of measurement.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
26
A weighted mean is used when two samples have different Ns.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
27
Data Set 3-1 - CD A student was interested in the structure of the families in the U.S. He sampled 29 of his 330 classmates asking the question, "How many children are there in your family?" He compiled the answers into a simple frequency distribution:
-Refer to Data Set 3-1. The mean is
A) 3.67
B) 2.25
C) 2.35
D) none of the other alternatives are correct.
-Refer to Data Set 3-1. The mean is
A) 3.67
B) 2.25
C) 2.35
D) none of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
28
Data Set 3-1 - CD A student was interested in the structure of the families in the U.S. He sampled 29 of his 330 classmates asking the question, "How many children are there in your family?" He compiled the answers into a simple frequency distribution:
-Refer to Data Set 3-1. A mean calculated for these data should be symbolized
A)
B)
C) either or
D) neither nor
-Refer to Data Set 3-1. A mean calculated for these data should be symbolized
A)
B)
C) either or
D) neither nor

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
29
Data Set 3-1 - CD A student was interested in the structure of the families in the U.S. He sampled 29 of his 330 classmates asking the question, "How many children are there in your family?" He compiled the answers into a simple frequency distribution:
-Refer to Data Set 3-1. The median is
A) 5
B) 3.5
C) 3
D) 2.
-Refer to Data Set 3-1. The median is
A) 5
B) 3.5
C) 3
D) 2.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
30
Data Set 3-1 - CD A student was interested in the structure of the families in the U.S. He sampled 29 of his 330 classmates asking the question, "How many children are there in your family?" He compiled the answers into a simple frequency distribution:
-Refer to Data Set 3-1. The mode is
A) 10
B) 8
C) 7
D) 2.
-Refer to Data Set 3-1. The mode is
A) 10
B) 8
C) 7
D) 2.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
31
Data Set 3-2: 5, 5, 1, 4, 4, 5
-The median of Data Set 3-2 is
A) 5
B) 4.5
C) 4
D) 24.
-The median of Data Set 3-2 is
A) 5
B) 4.5
C) 4
D) 24.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
32
Data Set 3-2: 5, 5, 1, 4, 4, 5
-The mean of Data Set 3-2 is
A) 5
B) 4.5
C) 4
D) 24.
-The mean of Data Set 3-2 is
A) 5
B) 4.5
C) 4
D) 24.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
33
Data Set 3-2: 5, 5, 1, 4, 4, 5
-The mode of Data Set 3-2 is
A) 3
B) 4
C) 5
D) 6.
-The mode of Data Set 3-2 is
A) 3
B) 4
C) 5
D) 6.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
34
Data Set 3-3: These data are representative of a freshman class at a Midwestern university.
-The mean of Data Set 3-3 is
A) 26.0
B) 27.9
C) 57.4
D) 27.0
-The mean of Data Set 3-3 is
A) 26.0
B) 27.9
C) 57.4
D) 27.0

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
35
Data Set 3-3: These data are representative of a freshman class at a Midwestern university.
-The median of Data Set 3-3 is
A) 27.5
B) 27
C) 426
D) 8.5
-The median of Data Set 3-3 is
A) 27.5
B) 27
C) 426
D) 8.5

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
36
Data Set 3-3: These data are representative of a freshman class at a Midwestern university.
-The mode of Data Set 3-3 is
A) 5
B) 16
C) 17
D) 26.
-The mode of Data Set 3-3 is
A) 5
B) 16
C) 17
D) 26.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
37
Data Set 3-4
The descriptive statistics for these data are approximately the same as those of a freshman class at a small private college.
-The mean of Data Set 3-4 is
A) 24.9
B) 58.0
C) 25.4
D) 23.9.
The descriptive statistics for these data are approximately the same as those of a freshman class at a small private college.
-The mean of Data Set 3-4 is
A) 24.9
B) 58.0
C) 25.4
D) 23.9.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
38
Data Set 3-4
The descriptive statistics for these data are approximately the same as those of a freshman class at a small private college.
-The median of Data Set 3-4 is
A) 8.5
B) 17
C) 23
D) 24.
The descriptive statistics for these data are approximately the same as those of a freshman class at a small private college.
-The median of Data Set 3-4 is
A) 8.5
B) 17
C) 23
D) 24.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
39
Data Set 3-4
The descriptive statistics for these data are approximately the same as those of a freshman class at a small private college.
-The mode of Data Set 3-4 is
A) 3
B) 5
C) 22
D) 23.
The descriptive statistics for these data are approximately the same as those of a freshman class at a small private college.
-The mode of Data Set 3-4 is
A) 3
B) 5
C) 22
D) 23.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
40
Data Set 3-5: 5, 1, 2, 1, 1, 5
-The median for Data Set 3-5 is
A) 1
B) 1.5
C) 2
D) 15.
-The median for Data Set 3-5 is
A) 1
B) 1.5
C) 2
D) 15.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
41
Data Set 3-5: 5, 1, 2, 1, 1, 5
-The mean of Data Set 3-5 is
A) 1.33
B) 2.33
C) 2.5
D) 15.
-The mean of Data Set 3-5 is
A) 1.33
B) 2.33
C) 2.5
D) 15.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
42
Data Set 3-5: 5, 1, 2, 1, 1, 5
-The mode for Data Set 3-5 is
A) 1
B) 2
C) 3
D) 5.
-The mode for Data Set 3-5 is
A) 1
B) 2
C) 3
D) 5.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
43
For a negatively skewed curve, the usual case with continuous data is that
A) high scores are more frequent than low scores and the mean is greater than the median
B) low scores are more frequent than high scores and the mean is greater than the median
C) high scores are more frequent than low scores and the median is greater than the mean
D) low scores are more frequent than high scores and the median is greater than the mean.
A) high scores are more frequent than low scores and the mean is greater than the median
B) low scores are more frequent than high scores and the mean is greater than the median
C) high scores are more frequent than low scores and the median is greater than the mean
D) low scores are more frequent than high scores and the median is greater than the mean.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
44
Usually, a frequency distribution with a mean of 100 and a median of 90 is
A) positively skewed
B) negatively skewed
C) symmetrical
D) cannot be determined without knowing the mode.
A) positively skewed
B) negatively skewed
C) symmetrical
D) cannot be determined without knowing the mode.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
45
For a positively skewed curve, the usual case with continuous data is that
A) high scores are more frequent and the mean is larger than the median
B) low scores are more frequent and the mean is larger than the median
C) high scores are more frequent and the mean is smaller than the median
D) low scores are more frequent and the mean is smaller than the median.
A) high scores are more frequent and the mean is larger than the median
B) low scores are more frequent and the mean is larger than the median
C) high scores are more frequent and the mean is smaller than the median
D) low scores are more frequent and the mean is smaller than the median.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
46
When the deviation scores, ( - ), are examined, your text noted two mathematical characteristics of the mean. These two characteristics involved
A) the maximum size of the mean and the minimum size of the mean
B) the size of the deviations when the mean is positive and the size when the mean is negative
C) the sum of the deviations and the size of the sum of the squared deviations
D) the maximum size of the deviations and their minimum size.
A) the maximum size of the mean and the minimum size of the mean
B) the size of the deviations when the mean is positive and the size when the mean is negative
C) the sum of the deviations and the size of the sum of the squared deviations
D) the maximum size of the deviations and their minimum size.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
47
Your text noted which of the following as a characteristic of the mean?
A) The sum of the results of squaring the difference between each score and the mean is zero.
B) The sum of the results of subtracting the mean from each score is a minimum.
C) Both of the descriptive alternatives.
D) Neither of the descriptive alternatives.
A) The sum of the results of squaring the difference between each score and the mean is zero.
B) The sum of the results of subtracting the mean from each score is a minimum.
C) Both of the descriptive alternatives.
D) Neither of the descriptive alternatives.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
48
Your text noted which of the following as a characteristic of the mean of a set of scores?
A) The sum is zero when the mean is subtracted from each score and these differences summed.
B) The sum of the results of scoring the difference between each score and the mean is a minimum.
C) Both of the descriptive alternatives.
D) Neither of the descriptive alternatives.
A) The sum is zero when the mean is subtracted from each score and these differences summed.
B) The sum of the results of scoring the difference between each score and the mean is a minimum.
C) Both of the descriptive alternatives.
D) Neither of the descriptive alternatives.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
49
Your text noted which of the following as a characteristic of the mean?
A) The sum of the results of squaring the difference between each score and the mean is a minimum.
B) The sum of the results of squaring the difference between each score and the mean is zero.
C) Both of the descriptive alternatives.
D) Neither of the descriptive alternatives.
A) The sum of the results of squaring the difference between each score and the mean is a minimum.
B) The sum of the results of squaring the difference between each score and the mean is zero.
C) Both of the descriptive alternatives.
D) Neither of the descriptive alternatives.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
50
Your text noted which of the following as a characteristic of the mean of a set of scores?
A) If each score is squared, the sum will be greater than the mean.
B) If each score is squared, the sum will be less than the mean.
C) The sum of A's is zero, where A is the difference between each score and the mean.
D) None of the other alternatives are correct.
A) If each score is squared, the sum will be greater than the mean.
B) If each score is squared, the sum will be less than the mean.
C) The sum of A's is zero, where A is the difference between each score and the mean.
D) None of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
51
Your text noted which of the following as a characteristic of the mean?
A) The sum of the results of subtracting the mean from each score is a minimum.
B) The sum of the results of subtracting the mean from each score is zero.
C) Both of the descriptive alternatives.
D) Neither of the descriptive alternatives.
A) The sum of the results of subtracting the mean from each score is a minimum.
B) The sum of the results of subtracting the mean from each score is zero.
C) Both of the descriptive alternatives.
D) Neither of the descriptive alternatives.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
52
The median of 4, 3, 4, 2, 3, 4, 4, is
A) 3
B) 3.5
C) 4.5
D) 4
A) 3
B) 3.5
C) 4.5
D) 4

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
53
The median of the numbers 7, 9, 8, 10, 11, 7, 8, is
A) 3.5
B) 8
C) 9
D) 22.5.
A) 3.5
B) 8
C) 9
D) 22.5.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
54
The median of the numbers 3, 1, 2, 2, 3, 2, is
A) 2
B) 2.5
C) 3
D) 3.5.
A) 2
B) 2.5
C) 3
D) 3.5.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
55
For scores of 8, 10, 8, 9, 8, 8, 9, 8, the median is
A) 8
B) 9
C) 4
D) 13.5.
A) 8
B) 9
C) 4
D) 13.5.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
56
For scores of 6, 3, 7, 6, 6, 5, 6, 4, the median is
A) 4
B) 5
C) 6
D) 12.5.
A) 4
B) 5
C) 6
D) 12.5.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
57
For scores of 6, 3, 5, 6, 4, 4, 6, 5, the median is
A) 9
B) 5
C) 4.5
D) 4.
A) 9
B) 5
C) 4.5
D) 4.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
58
For scores of 4, 3, 1, 3, 2, 3, the median is
A) 5
B) 4
C) 3.5
D) 3.
A) 5
B) 4
C) 3.5
D) 3.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
59
In an election Smith received 30 votes, Johnson 26, Williams 29, Jones 19, Brown 17, and Davis 31. (Smith is the most common name in the U.S., followed by Johnson, etc.) What is the mode of the distribution above?
A) 6
B) 31
C) Jones
D) none of the other alternatives are correct.
A) 6
B) 31
C) Jones
D) none of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
60
The mean is the proper descriptive statistic when
A) you have nominal data
B) you have ordinal data
C) you have severely skewed data
D) none of the other alternatives are correct.
A) you have nominal data
B) you have ordinal data
C) you have severely skewed data
D) none of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
61
In which situation would the mean be an appropriate measure of central tendency?
A) Most of the scores are near the minimum, a few are in the middle range, and there are almost none near the maximum
B) We have frequency data on cows, horses, mules, and goats
C) The data categories in the soil analysis are: 0-2 ppm, 3-5 ppm, 6-8 ppm, 9-11 ppm, and over 11 ppm
D) None of the other alternatives are correct.
A) Most of the scores are near the minimum, a few are in the middle range, and there are almost none near the maximum
B) We have frequency data on cows, horses, mules, and goats
C) The data categories in the soil analysis are: 0-2 ppm, 3-5 ppm, 6-8 ppm, 9-11 ppm, and over 11 ppm
D) None of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
62
"For our study of driving habits, we recorded the speed of every fifth vehicle on Drury Lane. Nearly every car traveled right at the speed limit or a little over, but there were some that were 10 mph under, even fewer at 20 mph under, and one car that crept by at just 15 mph. On the basis of the central tendency calculation on our data, we drew conclusions about all drivers on this stretch of road." The proper central tendency value calculated from the data is the
A) population median
B) sample median
C) population mean ( )
D) sample mean ( ).
A) population median
B) sample median
C) population mean ( )
D) sample mean ( ).

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
63
Which of the following was not a consideration in deciding whether to use the mean as the appropriate measure of central tendency?
A) size and number of class intervals
B) degree of skew of the distribution
C) whether any class intervals have no upper limit or a lower limit
D) the scale of measurement used.
A) size and number of class intervals
B) degree of skew of the distribution
C) whether any class intervals have no upper limit or a lower limit
D) the scale of measurement used.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
64
The _______ is appropriate for skewed data with an open-ended category.
A) mean
B) median
C) mode
D) any of the other alternatives are correct.
A) mean
B) median
C) mode
D) any of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
65
The U.S. Department of Agriculture reported the total number of bushels harvested of corn, soybeans, wheat, rice, and oats. This is a frequency distribution of a _______ variable; the proper measure of central tendency is a _______.
A) nominal mode
B) ordinal mode
C) nominal median
D) ordinal median.
A) nominal mode
B) ordinal mode
C) nominal median
D) ordinal median.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
66
If trees in a forest were classified as pine, cedar, oak, hickory and other, the appropriate measure of central tendency would be
A) the mean
B) the median
C) the mode
D) any of the other alternatives are correct.
A) the mean
B) the median
C) the mode
D) any of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
67
Imagine a bar graph with the following categories and frequencies: almonds, 4; betel, 6; cashew, 3; donut, 7. The central tendency statistic that is appropriate and its value:
A) median, 4
B) median, 5
C) mode, 7
D) mode, donut.
A) median, 4
B) median, 5
C) mode, 7
D) mode, donut.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
68
Imagine a bar graph with the following categories and frequencies: Alpha, 2; Beta, 4; Gamma, 1; Delta, 5. The central tendency statistic that is appropriate and its value:
A) median, 5
B) median, 3
C) mode, 5
D) mode, Delta.
A) median, 5
B) median, 3
C) mode, 5
D) mode, Delta.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
69
For which situation below is the mean the appropriate measure of central tendency?
A) the number of hours completed by college graduates, with categories of 120-129, 130-139, 140-149, 150-159, 160 and over.
B) the birds sighted on the annual Audubon bird count, with categories of blue jay, purple finch, scarlet tanager, crow, etc.
C) reaction time in stepping on the brake of a car when danger looms. This distribution is quite skewed
D) none of the other alternatives are correct.
A) the number of hours completed by college graduates, with categories of 120-129, 130-139, 140-149, 150-159, 160 and over.
B) the birds sighted on the annual Audubon bird count, with categories of blue jay, purple finch, scarlet tanager, crow, etc.
C) reaction time in stepping on the brake of a car when danger looms. This distribution is quite skewed
D) none of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
70
Suppose a distribution of scores had a mean of -6 and a median of +6. In the usual case for continuous data, you would conclude
A) the distribution is positively skewed
B) the distribution is negatively skewed
C) nothing about skewness unless additional information is given
D) that such scores are logically impossible.
A) the distribution is positively skewed
B) the distribution is negatively skewed
C) nothing about skewness unless additional information is given
D) that such scores are logically impossible.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
71
A distribution of scores had a mean of 30 and a median of 40. In the usual case of continuous data, the distribution is
A) positively skewed
B) negatively skewed
C) symmetrical
D) need to know the mode to make a decision.
A) positively skewed
B) negatively skewed
C) symmetrical
D) need to know the mode to make a decision.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
72
A frequency distribution had a mean of 3 and a median of -3. In the usual case of continuous data the distribution is
A) positively skewed
B) negatively skewed
C) symmetrical
D) you would have to know the mode in order to answer the question.
A) positively skewed
B) negatively skewed
C) symmetrical
D) you would have to know the mode in order to answer the question.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
73
In a negatively skewed curve the mean is usually
A) larger than the median
B) smaller than the median
C) exactly equal to the median
D) not https://d2lvgg3v3hfg70.cloudfront.net/TB9561/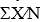 .
.
A) larger than the median
B) smaller than the median
C) exactly equal to the median
D) not https://d2lvgg3v3hfg70.cloudfront.net/TB9561/
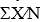 .
.
Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
74
If the mean of 7, 4, 4 is 5.0 and the mean of 14, 3, 7, 4 is 7.0, the weighted mean i
A) 6.0
B) 21.5
C) 4
D) none of the other alternatives are correct.
A) 6.0
B) 21.5
C) 4
D) none of the other alternatives are correct.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
75
The mean of 2, 3, and 4 is 3.0. The mean of 5, 6, 7, 8, and 9 is 7.0. The weighted mean is
A) 5.0
B) 4.5
C) 5.5
A) 5.0
B) 4.5
C) 5.5

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
76
The mean temperature for June was 70 F, for July it was 75 F, and for August it was 80 F. You should conclude that the overall mean for the three months is
A) 75 F
B) more than 75 F
C) less than 75 F.
A) 75 F
B) more than 75 F
C) less than 75 F.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
77
The mean temperature for January was 30 F. In February the mean was 25 F and for March the mean was 35 F. The weighted mean for these three months is
A) 30 F
B) greater than 30 F
C) less than 30 F.
A) 30 F
B) greater than 30 F
C) less than 30 F.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
78
Suppose the mean ACT score for high school seniors in Big City was 21; for Small Town seniors was 20 and for Tiny Berg seniors was 19. The mean of the seniors in the three places is
A) 20
B) less than 20
C) more than 20.
A) 20
B) less than 20
C) more than 20.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
79
The mean salary for all those with a Bachelors degree was $40,000; for all those with Masters degrees, $60,000; and for all those with doctorates, $80,000. The weighted mean salary will be
A) $60,000
B) less than $60,000
C) more than $60,000.
A) $60,000
B) less than $60,000
C) more than $60,000.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck
80
Two investigators tested their friends for memory span. The first tested five people and found a mean of 6.0. The second tested nine people and found a mean of 7.0. The weighted mean for the data is
A) 6.00
B) 6.50
C) 6.64
D) 7.00.
A) 6.00
B) 6.50
C) 6.64
D) 7.00.

Unlock Deck
Unlock for access to all 103 flashcards in this deck.
Unlock Deck
k this deck



