Deck 8: Advanced Project Network Analysis and Scheduling
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/29
Play
Full screen (f)
Deck 8: Advanced Project Network Analysis and Scheduling
1
To reduce the duration of a project using CPM, it is necessary to make a reduction on the activity with:
A) the largest cost slope on the critical path (CP)
B) the smallest cost slope on the CP
C) the largest cost slope not on the CP
D) the smallest cost slope not on the CP
A) the largest cost slope on the critical path (CP)
B) the smallest cost slope on the CP
C) the largest cost slope not on the CP
D) the smallest cost slope not on the CP
the smallest cost slope on the CP
2
When there are multiple critical paths and the project duration must be reduced, it is necessary to make reductions:
A) on whichever critical path affords the smallest cost increase
B) on whichever non-critical path affords the smallest cost increase
C) that will shorten all of the critical paths
D) that will shorten all of the non-critical paths
A) on whichever critical path affords the smallest cost increase
B) on whichever non-critical path affords the smallest cost increase
C) that will shorten all of the critical paths
D) that will shorten all of the non-critical paths
that will shorten all of the critical paths
3
To find the shortest possible project duration for the least cost:
A) crash all activities on the critical path (CP), then relax all non-CP activities
B) crash all activities not on the CP, then relax all critical activities
C) crash all activities in the project, then relax those not on the CP
D) crash all activities in the project, then relax those on the critical path
A) crash all activities on the critical path (CP), then relax all non-CP activities
B) crash all activities not on the CP, then relax all critical activities
C) crash all activities in the project, then relax those not on the CP
D) crash all activities in the project, then relax those on the critical path
crash all activities in the project, then relax those not on the CP
4
Which statement is correct: In the CPM, as the duration of a project is decreased, usually:
A) so are both its direct and indirect costs
B) direct costs increase, but indirect costs decrease
C) both direct and indirect costs increase
D) direct costs decrease, indirect costs increase
A) so are both its direct and indirect costs
B) direct costs increase, but indirect costs decrease
C) both direct and indirect costs increase
D) direct costs decrease, indirect costs increase

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
5
If a = 3, m = 5, and b = 6, then:
A) te = 4.667 and V = 1.5
B) te = 4.833 and V = 0.5
C) neither
A) te = 4.667 and V = 1.5
B) te = 4.833 and V = 0.5
C) neither

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
6
If the project completion has a distribution with Te = 15 weeks and Vp = 9, what is the probability that the project will be completed in less than 18 weeks? To answer this, the correct z value is:
A) 0.333
B) 1
C) -1
D) none of these
A) 0.333
B) 1
C) -1
D) none of these

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
7
Which statement is correct. The critical chain method:
A) helps planners to estimate the duration of activities more accurately.
B) stresses that, in order to finish the project on the project deadline (due date), all critical activities must be finished by their individual deadlines (due dates).
C) forces a person working on an activity to commit to when he/she will finish the work.
D) ensures that sufficient contingency reserve is built into the schedule of each individual activity.
E) None of the above is correct.
A) helps planners to estimate the duration of activities more accurately.
B) stresses that, in order to finish the project on the project deadline (due date), all critical activities must be finished by their individual deadlines (due dates).
C) forces a person working on an activity to commit to when he/she will finish the work.
D) ensures that sufficient contingency reserve is built into the schedule of each individual activity.
E) None of the above is correct.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
8
Which statement is incorrect:
A) Unlike the critical path, the critical chain ensures stability on the project.
B) The critical chain is likely to reduce project duration.
C) The critical chain is, in general, longer than the critical path.
D) The critical chain results in a high probability of finishing the project on the promised date.
E) Unlike the critical path, the critical chain ensures that a resource is scheduled to do only one activity at any specific time.
A) Unlike the critical path, the critical chain ensures stability on the project.
B) The critical chain is likely to reduce project duration.
C) The critical chain is, in general, longer than the critical path.
D) The critical chain results in a high probability of finishing the project on the promised date.
E) Unlike the critical path, the critical chain ensures that a resource is scheduled to do only one activity at any specific time.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
9
A project cannot have more than one ___________buffer.
A) Milestone
B) Feeding
C) Project
D) Resource
A) Milestone
B) Feeding
C) Project
D) Resource

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
10
Contingencies should be built in at the following level:
A) Program
B) Sub-program
C) Project
D) Sub-project
E) Activity
A) Program
B) Sub-program
C) Project
D) Sub-project
E) Activity

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
11
The following type of buffer does not add time to a schedule:
A) Milestone
B) Feeding
C) Project
D) Resource
E) None of the above
A) Milestone
B) Feeding
C) Project
D) Resource
E) None of the above

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
12
The following buffer enables the project manager to focus on critical activities by ensuring that the critical chain does not change during project execution:
A) Milestone
B) Feeding
C) Project
D) Resource
E) The combination of (b) and (d) above
A) Milestone
B) Feeding
C) Project
D) Resource
E) The combination of (b) and (d) above

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
13
Resource buffers are always placed:
A) on the critical chain only
B) on both critical and on non-critical paths
C) where non-critical paths merge into the critical chain
D) at the end of the critical chain
E) at places where a "count down" to the start of a non-critical activity is needed.
A) on the critical chain only
B) on both critical and on non-critical paths
C) where non-critical paths merge into the critical chain
D) at the end of the critical chain
E) at places where a "count down" to the start of a non-critical activity is needed.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
14
An approach to accelerate a project by simply eliminating discretionary dependencies is called:
A) Crashing
B) Concurrent engineering
C) Critical chain scheduling
D) Fast tracking
E) PERT/CPM
A) Crashing
B) Concurrent engineering
C) Critical chain scheduling
D) Fast tracking
E) PERT/CPM

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
15
GERT (circle all that are true):
A) utilizes branching nodes
B) like PERT, cannot repeat activities
C) unlike PERT/CPM, allows for multiple possible outcomes in a project
D) like CPM, activity duration estimates are restricted to a single, deterministic estimate
A) utilizes branching nodes
B) like PERT, cannot repeat activities
C) unlike PERT/CPM, allows for multiple possible outcomes in a project
D) like CPM, activity duration estimates are restricted to a single, deterministic estimate

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
16
Statement: PERT requires three time estimates for every activity.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
17
Statement: Given the assumptions of PERT, it is possible to determine the probability that a project will be completed by a specified date.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
18
Statement: In PERT, the mean (expected) time for each activity is determined by using a formula that gives the greatest weight to the "optimistic" time estimate.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
19
Statement: Although the times used in PERT are "estimates," the resulting probabilities are highly credible, especially in cases where little or no previous experience exists.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
20
Statement: In the critical path method (CPM), the direct cost of an activity is assumed to be proportional to its time, so shortening it to its "crash" time reduces its cost.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
21
Statement: The cost slope is the marginal tradeoff between the cost and time of doing a given activity.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
22
Statement: Indirect costs include administrative, overhead, and any penalty costs.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
23
Statement: A "resource loading" chart shows the amount of a given resource required in a project over a specified period of time.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
24
Statement: In resource loading, the project schedule is altered in an attempt to increase variations in the amount of the resource needed throughout the project.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
25
Statement: It is relatively easy to level many resources in a project at the same time.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
26
Use the following network diagram and table to answer parts (a) through (d).
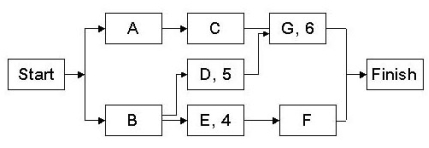
(a) Under "normal" conditions: what is the earliest the project can be completed; what is the critical path; what is the cost?
(b) Suppose you wanted to complete the project one week earlier than under normal conditions. What activity(ies) would you have to speed up. How much would it add to the cost of the project?
(c) Under "crash" conditions: what is the earliest the project can be completed; what is the minimum it will cost to complete it in this time.
(d) The costs in part (a) through (d) are direct costs. Suppose overhead costs are determined by the formula C = 4000 + 2000t, where t is the project duration in weeks. Using this formula, what are the total project costs (direct plus indirect) for the project durations you got in parts (a) and (d)? Based on a cost comparison, should the project be completed under normal conditions, crash conditions, or somewhere in between?

(a) Under "normal" conditions: what is the earliest the project can be completed; what is the critical path; what is the cost?
(b) Suppose you wanted to complete the project one week earlier than under normal conditions. What activity(ies) would you have to speed up. How much would it add to the cost of the project?
(c) Under "crash" conditions: what is the earliest the project can be completed; what is the minimum it will cost to complete it in this time.
(d) The costs in part (a) through (d) are direct costs. Suppose overhead costs are determined by the formula C = 4000 + 2000t, where t is the project duration in weeks. Using this formula, what are the total project costs (direct plus indirect) for the project durations you got in parts (a) and (d)? Based on a cost comparison, should the project be completed under normal conditions, crash conditions, or somewhere in between?

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
27
The Following Questions adapted from tutorial material of H Steyn Ed., Project Management -A Multi-disciplinary Approach (Pretoria: FPM Publishing, 2003). Reprinted with permission.
-Consider the schedule below:
RB = Resource Buffer
FB = Feeding Buffer
PB = Project Buffer
The letters A, B, C, D and E represent the resources needed to do the indicated activities.
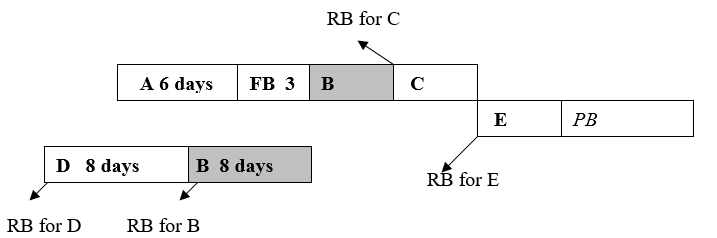
(a) Show the critical chain on the sketch above.
(b) Why is there no resource buffer (RB) before the activity to be performed by A?
(c) Why is there no resource buffer before the second task to be performed by resource B?
(d) Would the 6-day duration shown for the activity to be performed by A be a pessimistic or a realistic value?
-Consider the schedule below:
RB = Resource Buffer
FB = Feeding Buffer
PB = Project Buffer
The letters A, B, C, D and E represent the resources needed to do the indicated activities.

(a) Show the critical chain on the sketch above.
(b) Why is there no resource buffer (RB) before the activity to be performed by A?
(c) Why is there no resource buffer before the second task to be performed by resource B?
(d) Would the 6-day duration shown for the activity to be performed by A be a pessimistic or a realistic value?

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
28
The Following Questions adapted from tutorial material of H Steyn Ed., Project Management -A Multi-disciplinary Approach (Pretoria: FPM Publishing, 2003). Reprinted with permission.
-Put checks in the appropriate boxes in the table below.
-Put checks in the appropriate boxes in the table below.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck
29
The Following Questions adapted from tutorial material of H Steyn Ed., Project Management -A Multi-disciplinary Approach (Pretoria: FPM Publishing, 2003). Reprinted with permission.
-Complete the following table: state how the five steps of constraints management relate to the management of a single project when the critical chain method is used.
-Complete the following table: state how the five steps of constraints management relate to the management of a single project when the critical chain method is used.

Unlock Deck
Unlock for access to all 29 flashcards in this deck.
Unlock Deck
k this deck



