Deck 9: Exponential and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/352
Play
Full screen (f)
Deck 9: Exponential and Logarithmic Functions
1
Let and . Find:
A)
B)
C)
D)
A)
B)
C)
D)
1
Let 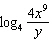 and
and 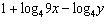 . Find the function
. Find the function 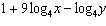 and its domain.
and its domain.
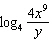 and
and 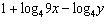 . Find the function
. Find the function 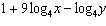 and its domain.
and its domain.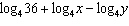 The domain is
The domain is 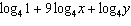 .
. 2
If 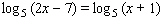 and
and 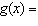 __________, then
__________, then 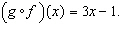
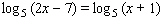 and
and 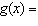 __________, then
__________, then 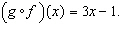
To find the function such that , we need to understand that the composition of functions means applying the function to first, and then applying the function to the result of .
Given , we want to find such that when we apply to , we get .
Let's denote . Then, we want to find such that .
Since , we can square both sides to find :
Now, we substitute for in the equation :
Therefore, the function that satisfies the condition is:
Given , we want to find such that when we apply to , we get .
Let's denote . Then, we want to find such that .
Since , we can square both sides to find :
Now, we substitute for in the equation :
Therefore, the function that satisfies the condition is:
3
If 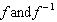 __________, then
__________, then 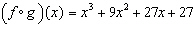
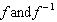 __________, then
__________, then 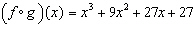
To find the function such that , we need to determine what function when composed with results in the given polynomial.
Given that , the composition means we are taking the output of and plugging it into . In other words, we are cubing whatever is. So, we are looking for a function such that when we cube it, we get .
Let's assume . Then we have:
We need to expand this and match it to the given polynomial . However, we can notice that the given polynomial can be factored as:
This suggests that should be , because when we plug into , we get:
Therefore, the function that satisfies the given condition is:
Given that , the composition means we are taking the output of and plugging it into . In other words, we are cubing whatever is. So, we are looking for a function such that when we cube it, we get .
Let's assume . Then we have:
We need to expand this and match it to the given polynomial . However, we can notice that the given polynomial can be factored as:
This suggests that should be , because when we plug into , we get:
Therefore, the function that satisfies the given condition is:
4
Let . Find:
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
4
The __________ of 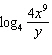 and
and 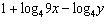 , denoted as
, denoted as 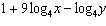 , is defined by
, is defined by 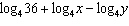 __________.
__________.
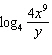 and
and 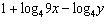 , denoted as
, denoted as  , is defined by
, is defined by  __________.
__________.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
5
Let and . Find:
A) -4
B) 0
C)
D) 48
A) -4
B) 0
C)
D) 48

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
6
Let  and
and  . Find the composition.
. Find the composition. 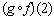
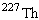 and
and 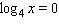 . Find the composition.
. Find the composition. 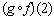

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
7
Let . Find: .
A) 13
B) 7
C) 20
D) 30
A) 13
B) 7
C) 20
D) 30

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
8
Let and . Find: f g
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
9
The __________ of the function 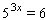 is the set of real numbers x that are in the domain of both f and g .
is the set of real numbers x that are in the domain of both f and g .
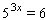 is the set of real numbers x that are in the domain of both f and g .
is the set of real numbers x that are in the domain of both f and g .
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
10
Let and . Find the domain of .
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
10
Let 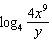 and
and 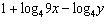 . Find the function
. Find the function 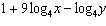 and its domain.
and its domain.
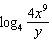 and
and 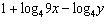 . Find the function
. Find the function 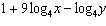 and its domain.
and its domain.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
11
Let 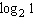 and
and 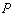 . Find the composition.
. Find the composition. 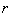
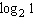 and
and 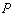 . Find the composition.
. Find the composition. 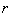

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
12
Let and . Find: f . g
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
13
Let Find:
A) 21
B) -21
C) -16
D) 0
A) 21
B) -21
C) -16
D) 0

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
13
The __________ of 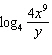 and
and 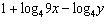 , denoted as
, denoted as 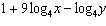 , is defined by
, is defined by 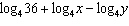 __________.
__________.
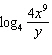 and
and 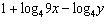 , denoted as
, denoted as 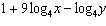 , is defined by
, is defined by 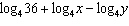 __________.
__________.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
14
Let  and
and  . Find the composition.
. Find the composition. 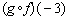
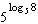 and
and 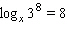 . Find the composition.
. Find the composition. 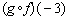

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
15
Let 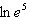 and
and  . Find the composition.
. Find the composition. 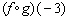
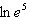 and
and 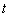 . Find the composition.
. Find the composition. 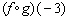

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
16
The __________ function 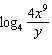 is defined by
is defined by 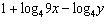 __________.
__________.
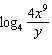 is defined by
is defined by 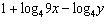 __________.
__________.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
16
Let 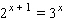 and
and 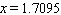 . Find the composition.
. Find the composition. 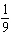
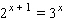 and
and 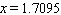 . Find the composition.
. Find the composition. 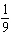

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
17
The __________ function 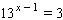 is defined by
is defined by 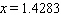 __________.
__________.
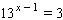 is defined by
is defined by 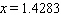 __________.
__________.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
18
The __________ of 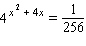 and
and 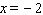 , denoted as
, denoted as  , is defined by
, is defined by 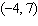 __________ and the __________ of
__________ and the __________ of 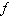 and
and 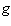 , denoted as
, denoted as 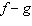 , is defined by
, is defined by 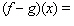 __________.
__________.
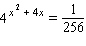 and
and 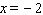 , denoted as
, denoted as 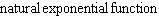 , is defined by
, is defined by 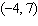 __________ and the __________ of
__________ and the __________ of 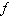 and
and 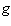 , denoted as
, denoted as 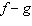 , is defined by
, is defined by 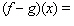 __________.
__________.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
19
The __________ of 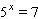 and
and 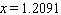 , denoted as
, denoted as 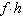 , is defined by
, is defined by 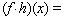 __________ and the __________ of
__________ and the __________ of 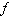 and
and 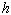 , denoted as
, denoted as 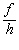 , is defined by
, is defined by 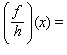 __________.
__________.
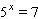 and
and 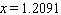 , denoted as
, denoted as  , is defined by
, is defined by 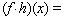 __________ and the __________ of
__________ and the __________ of 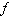 and
and 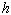 , denoted as
, denoted as 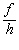 , is defined by
, is defined by 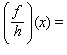 __________.
__________.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
20
Let 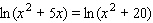 and
and 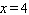 . Find the composition.
. Find the composition. 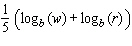
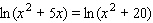 and
and 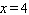 . Find the composition.
. Find the composition. 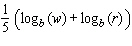

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
21
Let 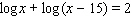 and
and 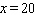 . Find the composition.
. Find the composition. 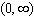
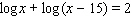 and
and 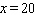 . Find the composition.
. Find the composition. 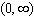

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
22
Determine whether the function is one-to-one.
A) one-to-one
B) not one-to-one
A) one-to-one
B) not one-to-one

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
23
Let 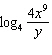 and
and 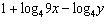 . Find the function
. Find the function 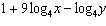 .
.
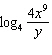 and
and 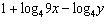 . Find the function
. Find the function 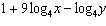 .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
23
The graphs of a function and its inverse are _________ to the line 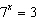

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
24
The __________ line test can be used to decide whether the graph of a function represents a one-to-one function.

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
25
Let 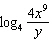 and
and 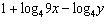 . Find the composition.
. Find the composition. 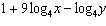
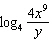 and
and 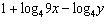 . Find the composition.
. Find the composition. 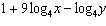

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
25
If the function is one-to-one, find its inverse.
A)
B)
C)
D) not one-to-one
A)
B)
C)
D) not one-to-one

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
26
A function is called a __________ function if different inputs determine different outputs.

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
27
Let 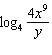 and
and 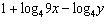 . Find the composition.
. Find the composition. 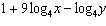
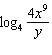 and
and 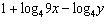 . Find the composition.
. Find the composition. 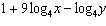

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
27
If the point (- 9, 2) is on the graph of the one-to-one function f , then what point is on the graph of f -1?
A)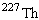
B)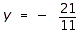
C)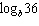
D)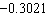
A)
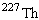
B)
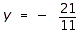
C)
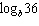
D)
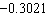

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
28
The __________ line test can be used to decide whether the graph of a function represents a one-to-one function.

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
29
A function is called a __________ function if different inputs determine different outputs.

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
30
Let 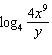 and
and 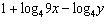 . Find the domain of the function
. Find the domain of the function 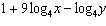 .
.
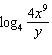 and
and 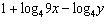 . Find the domain of the function
. Find the domain of the function 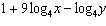 .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
30
Determine the graph of the function is one-to-one. 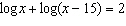
A) not one-to one
B) one-to one
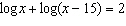
A) not one-to one
B) one-to one

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
31
Let 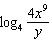 and
and 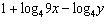 . Find the function
. Find the function 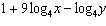 .
.
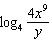 and
and 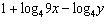 . Find the function
. Find the function 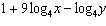 .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
31
Let 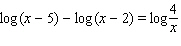 and
and 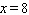 . Find the composition.
. Find the composition. 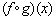
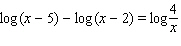 and
and 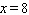 . Find the composition.
. Find the composition. 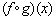

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
32
Let 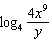 and
and 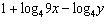 . Find the domain of the function
. Find the domain of the function 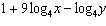 .
.
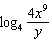 and
and 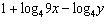 . Find the domain of the function
. Find the domain of the function 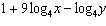 .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
32
If the function is one-to-one, find its inverse.
A)
B)
C)
D) not one-to-one
A)
B)
C)
D) not one-to-one

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
33
Let 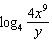 and
and 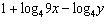 . Find the composition.
. Find the composition. 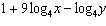
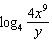 and
and 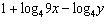 . Find the composition.
. Find the composition. 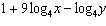

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
33
Select the graph of the function that is one-to-one.
A)
B)
C)
D)
A)
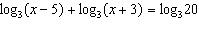
B)
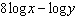
C)
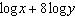
D)
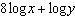

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
34
Let 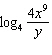 and
and 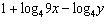 . Find the domain of the function
. Find the domain of the function 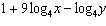 .
.
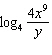 and
and 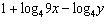 . Find the domain of the function
. Find the domain of the function 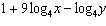 .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
34
The functions 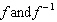 are __________.
are __________.
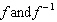 are __________.
are __________.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
35
Let 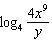 and
and 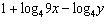 . Find the function
. Find the function 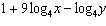 .
.
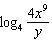 and
and 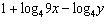 . Find the function
. Find the function 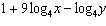 .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
35
Which of the following statements is true for a one-to-one function.
A) A vertical line intersects the graph in more than one point.
B) A horizontal line intersects the graph in more than one point.
C) A horizontal line intersects the graph in at most one point.
D) none of these
A) A vertical line intersects the graph in more than one point.
B) A horizontal line intersects the graph in more than one point.
C) A horizontal line intersects the graph in at most one point.
D) none of these

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
36
Let 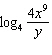 and
and 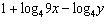 . Find the composition.
. Find the composition. 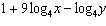
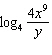 and
and 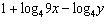 . Find the composition.
. Find the composition. 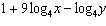

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
36
The graph represents a function. Use the horizontal line test to decide whether the function is one-to-one.  Answer yes or no .
Answer yes or no .
 Answer yes or no .
Answer yes or no .
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
37
Let  and
and  . Find the composition.
. Find the composition. 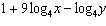
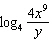 and
and 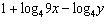 . Find the composition.
. Find the composition. 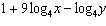

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
37
Determine whether the function is one-to-one. 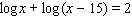 Answer yes or no .
Answer yes or no .
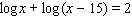 Answer yes or no .
Answer yes or no .
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
38
Let 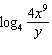 and
and 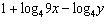 . Find the composition.
. Find the composition. 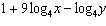
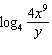 and
and 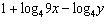 . Find the composition.
. Find the composition. 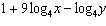

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
38
Find the inverse of the function and express it using 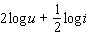 notation.
notation. 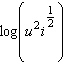
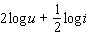 notation.
notation. 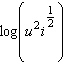

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
39
Let 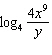 and
and 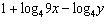 . Find the composition.
. Find the composition. 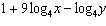
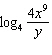 and
and 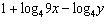 . Find the composition.
. Find the composition. 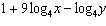

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
39
The graph represents a function. Use the horizontal line test to decide whether the function is one-to-one. 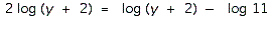 Answer yes or no .
Answer yes or no .
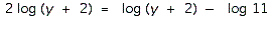 Answer yes or no .
Answer yes or no .
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
40
Let 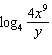 and
and 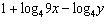 . Find the composition.
. Find the composition. 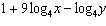
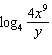 and
and 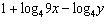 . Find the composition.
. Find the composition. 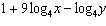

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
40
If the point 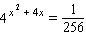 is on the graph of the one-to-one function
is on the graph of the one-to-one function 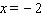 , then the point __________ is on the graph of
, then the point __________ is on the graph of  .
.
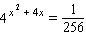 is on the graph of the one-to-one function
is on the graph of the one-to-one function 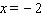 , then the point __________ is on the graph of
, then the point __________ is on the graph of  .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
41
If 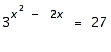 is a one-to-one function, the domain of
is a one-to-one function, the domain of 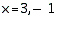 is the __________ of
is the __________ of  , and the range of
, and the range of  is the __________ of
is the __________ of 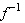 .
.
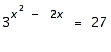 is a one-to-one function, the domain of
is a one-to-one function, the domain of 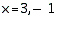 is the __________ of
is the __________ of 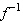 , and the range of
, and the range of  is the __________ of
is the __________ of  .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
42
Determine whether the function is one-to-one. 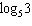
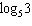

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
42
Determine whether the function is one-to-one. 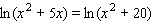 Answer yes or no .
Answer yes or no .
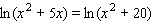 Answer yes or no .
Answer yes or no .
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
43
If 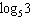 is a one-to-one function, the domain of
is a one-to-one function, the domain of 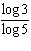 is the __________ of
is the __________ of 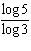 .
.
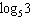 is a one-to-one function, the domain of
is a one-to-one function, the domain of 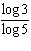 is the __________ of
is the __________ of 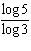 .
.
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
43
Determine whether the function is one-to-one. 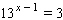 Answer yes or no .
Answer yes or no .
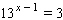 Answer yes or no .
Answer yes or no .
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
44
Determine whether the function is one-to-one. 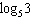
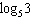

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
44
Determine whether the function is one-to-one.  Answer yes or no .
Answer yes or no .
 Answer yes or no .
Answer yes or no .
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
45
Which of the following statements is true for ?
A) The graph of is an increasing function.
B) The y -axis is a horizontal asymptote of the graph.
C) The range is all real numbers.
D) (1, 0) is a point of the graph of .
A) The graph of is an increasing function.
B) The y -axis is a horizontal asymptote of the graph.
C) The range is all real numbers.
D) (1, 0) is a point of the graph of .

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
46
Graph the inverse of the following one-to-one function. 

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
47
Exponential functions have a constant base and a variable _________.

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
48
Compare the graph of to the graph of .
A) The graph of is shifted 5 units to the left of the graph of .
B) The graph of is shifted 5 units to the right of the graph of .
C) The graph of is shifted up 5 units from the graph of .
D) The graph of is shifted down 5 units from the graph of .
A) The graph of is shifted 5 units to the left of the graph of .
B) The graph of is shifted 5 units to the right of the graph of .
C) The graph of is shifted up 5 units from the graph of .
D) The graph of is shifted down 5 units from the graph of .

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
49
Two exponential functions of the form are graphed below. Which function has the larger base b , the one graphed in red or the one graphed in blue? 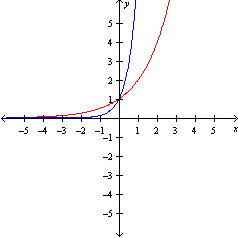
A) red
B) blue

A) red
B) blue

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
50
Determine whether the function is one-to-one. 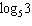
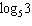

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
50
An initial deposit of $20,000 earns 3% interest, compounded monthly. How much will be in the account after 15 years? Round your answer to the nearest cent.
A) $4,090,067.19
B) $31,348.63
C) $31,159.35
D) $20,763.27
A) $4,090,067.19
B) $31,348.63
C) $31,159.35
D) $20,763.27

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
51
An initial deposit of $20,000 earns 8% interest, compounded quarterly. How much will be in the account after 10 years?
Please give the answer to the nearest cent. $__________
Please give the answer to the nearest cent. $__________

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
52
Determine whether the function is one-to-one. 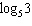
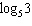

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
52
Find the inverse of the function: 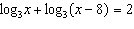
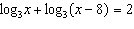

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
53
If the point 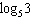 is on the graph of the one-to-one function
is on the graph of the one-to-one function 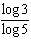 , then what point is on the graph of
, then what point is on the graph of 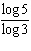 ?
?
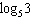 is on the graph of the one-to-one function
is on the graph of the one-to-one function 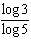 , then what point is on the graph of
, then what point is on the graph of 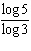 ?
?
Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
53
Use composition to show that the pair of functions are inverses. 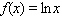
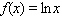

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
54
Find the inverse of the function and express it using 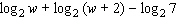 notation.
notation. 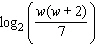
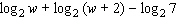 notation.
notation. 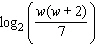

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck
55
Find the inverse of the function: 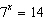
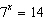

Unlock Deck
Unlock for access to all 352 flashcards in this deck.
Unlock Deck
k this deck



