Deck 16: Developing and Extending Geometric Concepts and Systems
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/47
Play
Full screen (f)
Deck 16: Developing and Extending Geometric Concepts and Systems
1
Classifying squares by the size of their angles is a basic concept associated with:
A)topological geometry.
B)Euclidean geometry.
C)transformational geometry.
D)coordinate geometry.
A)topological geometry.
B)Euclidean geometry.
C)transformational geometry.
D)coordinate geometry.
Euclidean geometry.
2
Which of the following reasons is the least valid for including geometry in the elementary school curriculum?
A)Spatial visualization skills are developed.
B)Geometry connects mathematics to aesthetic aspects of the world.
C)Children learn skills that are applicable in other areas of mathematics.
D)Measurement and fraction concepts are developed as geometric concepts are learned.
A)Spatial visualization skills are developed.
B)Geometry connects mathematics to aesthetic aspects of the world.
C)Children learn skills that are applicable in other areas of mathematics.
D)Measurement and fraction concepts are developed as geometric concepts are learned.
Measurement and fraction concepts are developed as geometric concepts are learned.
3
Identifying whether an object is inside or outside of a fence is a basic concept associated with:
A)topological geometry.
B)Euclidean geometry.
C)transformational geometry.
D)coordinate geometry.
A)topological geometry.
B)Euclidean geometry.
C)transformational geometry.
D)coordinate geometry.
topological geometry.
4
The research of Dina van Hiele-Geldof and Pierre Marie van Hiele suggests that:
A)describing, classifying, and generalizing attributes of geometric figures should be central to the study of geometry in the elementary school curriculum.
B)recognizing and naming geometric figures is the central purpose of elementary school geometry.
C)elementary school students should develop proofs using postulates and axioms.
D)geometry is too difficult for elementary school students and should be delayed until later grades.
A)describing, classifying, and generalizing attributes of geometric figures should be central to the study of geometry in the elementary school curriculum.
B)recognizing and naming geometric figures is the central purpose of elementary school geometry.
C)elementary school students should develop proofs using postulates and axioms.
D)geometry is too difficult for elementary school students and should be delayed until later grades.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
5
If a child can distinguish between the similarities and differences of rectangles and squares, that child is said to have the skill called:
A)figure - ground perception.
B)perception of special relationships.
C)visual discrimination.
D)visual memory.
A)figure - ground perception.
B)perception of special relationships.
C)visual discrimination.
D)visual memory.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
6
The ability to see two or more objects in relation to oneself or in relation to each other is the visual skill known as:
A)perceptual constancy.
B)position-in-space perception.
C)perception of spatial relationships.
D)visual discrimination.
A)perceptual constancy.
B)position-in-space perception.
C)perception of spatial relationships.
D)visual discrimination.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
7
Research about spatial sense has established all of the following except for :
A)Young boys outscore young girls in several areas of spatial sense such as mazes and block designs.
B)Mental rotations are especially difficult for young girls compared to young boys.
C)Practice with spatial sense activities does not appear to improve young girls' spatial sense.
D)Access to early exposure to spatial sense activities gives children a head start on developing spatial sense awareness.
A)Young boys outscore young girls in several areas of spatial sense such as mazes and block designs.
B)Mental rotations are especially difficult for young girls compared to young boys.
C)Practice with spatial sense activities does not appear to improve young girls' spatial sense.
D)Access to early exposure to spatial sense activities gives children a head start on developing spatial sense awareness.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
8
Identifying shapes which have been turned or flipped is a basic concept associated with:
A)topological geometry.
B)Euclidean geometry.
C)transformational geometry.
D)coordinate geometry.
A)topological geometry.
B)Euclidean geometry.
C)transformational geometry.
D)coordinate geometry.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
9
The Pythagorean Theorem is useful for all of the following except for :
A)determining if a given triangle is a right triangle.
B)determining if a set of three numbers constitutes a Pythagorean triple.
C)determining if a triangle is isosceles.
D)determining one side of a right triangle, given the other two sides.
A)determining if a given triangle is a right triangle.
B)determining if a set of three numbers constitutes a Pythagorean triple.
C)determining if a triangle is isosceles.
D)determining one side of a right triangle, given the other two sides.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
10
If children stand in a tight circle, then slowly expand the circle by walking backwards, then reverse their positions by walking forward to the tight circle again, they are practicing with a topological geometry relationship known as:
A)proximity and relative position.
B)separation.
C)order.
D)enclosure.
A)proximity and relative position.
B)separation.
C)order.
D)enclosure.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
11
The ability to recognize figures or objects in space, regardless of size, position, or orientation is the visual skill known as:
A)figure - ground perception.
B)perceptual constancy.
C)position-in-space perception.
D)visual memory.
A)figure - ground perception.
B)perceptual constancy.
C)position-in-space perception.
D)visual memory.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
12
If a fixed figure slides from one position to another, that motion is described as a:
A)rotation.
B)reflection.
C)translation.
D)dilation.
A)rotation.
B)reflection.
C)translation.
D)dilation.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
13
All of the following statements are true about similar figures except :
A)They have the same shape but not the same size.
B)They have congruent corresponding angles.
C)They have corresponding sides in the same ratio.
D)They are dilations in transformational geometry.
A)They have the same shape but not the same size.
B)They have congruent corresponding angles.
C)They have corresponding sides in the same ratio.
D)They are dilations in transformational geometry.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
14
Choosing the highest possible level, a student who can write and understand two-column proofs has reached which van Hiele level?
A)deduction
B)analysis
C)informal deduction
D)rigor
A)deduction
B)analysis
C)informal deduction
D)rigor

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
15
A parallelogram has:
A)rotational symmetry.
B)line symmetry.
C)both rotational and line symmetry.
D)neither rotational nor line symmetry.
A)rotational symmetry.
B)line symmetry.
C)both rotational and line symmetry.
D)neither rotational nor line symmetry.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
16
Describing locations of objects with respect to a two- or three- dimensional grid space is a basic concept associated with:
A)topological geometry.
B)Euclidean geometry.
C)transformational geometry.
D)coordinate geometry.
A)topological geometry.
B)Euclidean geometry.
C)transformational geometry.
D)coordinate geometry.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
17
Choosing the highest possible level, a child who recognizes that all squares are rectangles has reached which van Hiele level?
A)analysis
B)informal deduction
C)visualization
D)deduction
A)analysis
B)informal deduction
C)visualization
D)deduction

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
18
A child who cannot recognize the six parts of a triangle is still operating at which of the following van Hiele levels?
A)analysis
B)visualization
C)informal deduction
D)rigor
A)analysis
B)visualization
C)informal deduction
D)rigor

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
19
A three-dimensional object which has four congruent faces the same shape and size, four vertices, and six edges is:
A)a cube.
B)a prism.
C)a pyramid.
D)a rectangular solid.
A)a cube.
B)a prism.
C)a pyramid.
D)a rectangular solid.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
20
If a child can isolate alternate interior angles in a complex drawing, that child is said to possess the visual skill known as:
A)figure - ground perception.
B)perception of spatial relationships.
C)visual discrimination.
D)visual memory.
A)figure - ground perception.
B)perception of spatial relationships.
C)visual discrimination.
D)visual memory.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
21
Explain the progression of the van Hiele levels of geometric understanding. Give an example to illustrate each level.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
22
Which of the following triangles can also be an equilateral triangle?
A)acute
B)scalene
C)right
D)obtuse
A)acute
B)scalene
C)right
D)obtuse

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
23
TIMSS: Of the following options, which is NOT true for all rectangles?
A)The opposite sides are parallel.
B)The opposite sides are equal.
C)All angles are right angles.
D)The diagonals are equal.
E)The diagonals are perpendicular.
A)The opposite sides are parallel.
B)The opposite sides are equal.
C)All angles are right angles.
D)The diagonals are equal.
E)The diagonals are perpendicular.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
24
Which of the following combinations of regular polygons will tessellate?
A)2 squares, 2 triangles
B)2 pentagons, 1 square
C)2 octagons, 1 triangle
D)3 hexagons
A)2 squares, 2 triangles
B)2 pentagons, 1 square
C)2 octagons, 1 triangle
D)3 hexagons

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
25
An octahedron has 8 faces and 6 vertices. It has ___ edges.
A)14
B)12
C)10
D)8
A)14
B)12
C)10
D)8

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
26
A seven-sided figure called:
A)a hexagon.
B)a heptagon.
C)a decagon.
D)an octagon.
A)a hexagon.
B)a heptagon.
C)a decagon.
D)an octagon.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
27
NAEP: Which of the following cannot be folded into a cube?
A)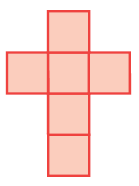
B)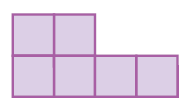
C)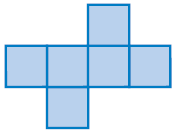
D)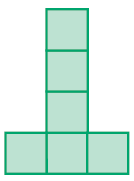
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
28
A parent asks why geometry is taught in the elementary school curriculum when students do not yet know their basic facts and therefore should be spending time on learning them instead. How would you reply to this parent?

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
29
All of the following are true about a parallelogram except:
A)two pairs of parallel sides.
B)two pairs of congruent sides.
C)congruent diagonals.
D)diagonals bisect each other.
A)two pairs of parallel sides.
B)two pairs of congruent sides.
C)congruent diagonals.
D)diagonals bisect each other.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
30
Why is it important for a child to develop spatial sense?

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
31
Explain the differences between congruence and similarity of geometric figures.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
32
Which of the following is true?
A)All squares are rectangles.
B)All rectangles are squares.
C)All parallelograms are rhombuses.
D)All rhombuses are rectangles.
A)All squares are rectangles.
B)All rectangles are squares.
C)All parallelograms are rhombuses.
D)All rhombuses are rectangles.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
33
Teacher Licensing Examination Questions
Praxis: In this circle with center O and radius 2, AP has length 3 and is tangent to the circle at P .
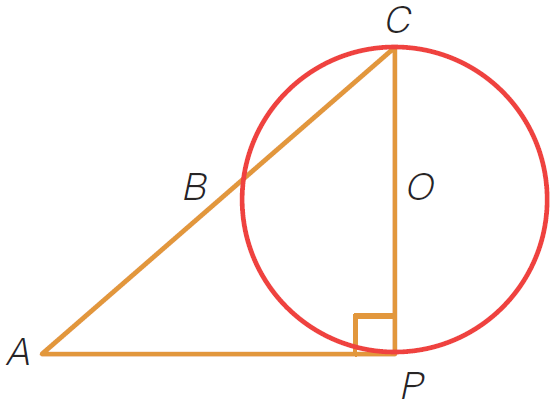
If CP is a diameter of the circle, what is the length of AC ?
A)1.3
B)2.0
C)3.2
D)5.0
Praxis: In this circle with center O and radius 2, AP has length 3 and is tangent to the circle at P .

If CP is a diameter of the circle, what is the length of AC ?
A)1.3
B)2.0
C)3.2
D)5.0

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
34
Give four different types of manipulatives you could use to introduce geometric concepts to elementary children. Explain how these help children to understand these concepts.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
35
What are some activities you could use to introduce children to three-dimensional geometry? Explain how these activities encourage the development of these geometric concepts.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
36
The following translation will move point Q from (3, − 4)to ______.
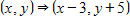
A)(6, 1)
B)(0, 9)
C)(0, 1)
D)(6, 9)
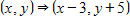
A)(6, 1)
B)(0, 9)
C)(0, 1)
D)(6, 9)

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
37
Explain the differences between the four geometry systems that are taught in the elementary school curriculum (topological, Euclidean, transformational, and coordinate geometry).

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
38
How many lines of symmetry are there in a regular hexagon?
A)3
B)4
C)6
D)8
A)3
B)4
C)6
D)8

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
39
What is the area of a figure on a grid with 12 interior points and 8 boundary points?
A)15
B)19
C)13
D)14
A)15
B)19
C)13
D)14

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
40
The legs of a right triangle are 5 cm and 12 cm. The length of the third side is:
A)17 cm.
B)13 cm.
C)60 cm.
D)119 cm.
A)17 cm.
B)13 cm.
C)60 cm.
D)119 cm.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
41
Use pattern blocks to demonstrate and represent each geometric concept.
A) tessellation
B) line symmetry
C) rotational symmetry
D) similarity
E) congruence

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
42
A student contended that if a line cuts a figure into two congruent parts, that line must be a line of symmetry. Is this a true or false statement? Defend your position.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
43
You are asked to design a new set of coins. All coins will be circular and colored silver, but of different diameters
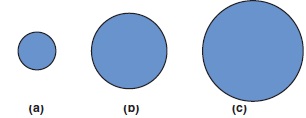
Researchers have found that an ideal coin system meets the following requirements:
Diameters of coins should not be smaller than 15 mm and not be larger than 45 mm.
Given a coin, the diameter of the next coin must be at least 30% larger.
The minting machinery can only produce coins with diameters of a whole number of millimeters (e.g., 17 mm is allowed, but 17.3 mm is not.). You are asked to design a set of coins that satisfy the above requirements. You should start with a 15-mm coin, and your set should contain as any coins as possible. What would be the diameters of the coins in your set?

Researchers have found that an ideal coin system meets the following requirements:
Diameters of coins should not be smaller than 15 mm and not be larger than 45 mm.
Given a coin, the diameter of the next coin must be at least 30% larger.
The minting machinery can only produce coins with diameters of a whole number of millimeters (e.g., 17 mm is allowed, but 17.3 mm is not.). You are asked to design a set of coins that satisfy the above requirements. You should start with a 15-mm coin, and your set should contain as any coins as possible. What would be the diameters of the coins in your set?

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
44
Explain the differences between translations, rotations, reflections, and dilations.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
45
Use paper figures to derive the area formulas for a triangle and a parallelogram from the area formula of a rectangle.

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
46
Illustrate each of the following geometric figures or concepts with a quick sketch. Label the sketches as needed.
Point, ray, line segment
Congruency and similarity
Regular polygon
Irregular Polygon
Open/closed figure
Three kinds of angles
Two tessellations
Three different triangles
Line symmetry
Rotational symmetry
A slide
A flip
A turn
A pantomime shape that will fold into an open box
A pentomino shape that will not fold into an open box
Point, ray, line segment
Congruency and similarity
Regular polygon
Irregular Polygon
Open/closed figure
Three kinds of angles
Two tessellations
Three different triangles
Line symmetry
Rotational symmetry
A slide
A flip
A turn
A pantomime shape that will fold into an open box
A pentomino shape that will not fold into an open box

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck
47
Why is it important to teach children about coordinate geometry?

Unlock Deck
Unlock for access to all 47 flashcards in this deck.
Unlock Deck
k this deck



