Deck 6: Exponential and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/234
Play
Full screen (f)
Deck 6: Exponential and Logarithmic Functions
1
For the given functions f and g, find the requested composite function value.
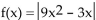 , g(x)= 16x - 3; Find (f ∘ g)(7).
, g(x)= 16x - 3; Find (f ∘ g)(7).
A)106,602
B)6,717
C)99,885
D)45,780
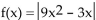 , g(x)= 16x - 3; Find (f ∘ g)(7).
, g(x)= 16x - 3; Find (f ∘ g)(7).A)106,602
B)6,717
C)99,885
D)45,780
106,602
2
For the given functions f and g, find the requested composite function value.
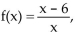 g(x)=
g(x)= 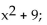 Find (g ∘ f)(-2).
Find (g ∘ f)(-2).
A)25
B)13
C)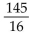
D)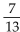
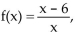 g(x)=
g(x)= 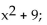 Find (g ∘ f)(-2).
Find (g ∘ f)(-2).A)25
B)13
C)
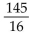
D)
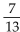
25
3
For the given functions f and g, find the requested composite function value.
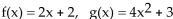 ; Find (g ∘ g)(1).
; Find (g ∘ g)(1).
A)199
B)16
C)67
D)10
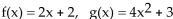 ; Find (g ∘ g)(1).
; Find (g ∘ g)(1).A)199
B)16
C)67
D)10
199
4
For the given functions f and g, find the requested composite function.
f(x)=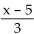 , g(x)= 3x + 5; Find (g ∘ f)(x).
, g(x)= 3x + 5; Find (g ∘ f)(x).
A)x
B)3x + 10
C)x + 10
D)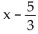
f(x)=
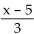 , g(x)= 3x + 5; Find (g ∘ f)(x).
, g(x)= 3x + 5; Find (g ∘ f)(x).A)x
B)3x + 10
C)x + 10
D)
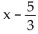

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
5
For the given functions f and g, find the requested composite function value.
f(t)=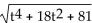 ,
, 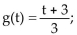 Find (f ∘ g)(9).
Find (f ∘ g)(9).
A)25
B)31
C)625
D)360
f(t)=
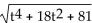 ,
, 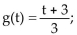 Find (f ∘ g)(9).
Find (f ∘ g)(9).A)25
B)31
C)625
D)360

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
6
For the given functions f and g, find the requested composite function value.
f(x)= 2x + 6, g(x)=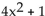 ; Find
; Find 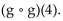
A)16,901
B)136
C)785
D)34
f(x)= 2x + 6, g(x)=
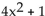 ; Find
; Find 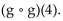
A)16,901
B)136
C)785
D)34

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
7
Evaluate the expression using the values given in the table.
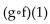
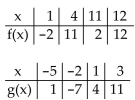
A)-7
B)4
C)-2
D)11
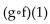
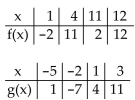
A)-7
B)4
C)-2
D)11

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
8
For the given functions f and g, find the requested composite function.
f(x)=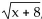 g(x)= 8x - 12; Find (f ∘ g)(x).
g(x)= 8x - 12; Find (f ∘ g)(x).
A)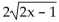
B)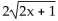
C)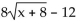
D)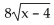
f(x)=
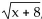 g(x)= 8x - 12; Find (f ∘ g)(x).
g(x)= 8x - 12; Find (f ∘ g)(x).A)
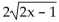
B)
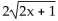
C)
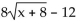
D)
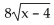

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
9
For the given functions f and g, find the requested composite function.
f(x)=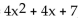 , g(x)= 4x - 3; Find (g ∘ f)(x).
, g(x)= 4x - 3; Find (g ∘ f)(x).
A)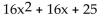
B)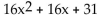
C)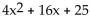
D)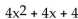
f(x)=
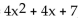 , g(x)= 4x - 3; Find (g ∘ f)(x).
, g(x)= 4x - 3; Find (g ∘ f)(x).A)
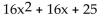
B)
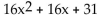
C)
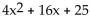
D)
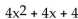

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
10
For the given functions f and g, find the requested composite function.
f(x)= 3x + 8, g(x)= 3x - 1; Find (f ∘ g)(x).
A)9x + 5
B)9x + 11
C)9x + 7
D)9x + 23
f(x)= 3x + 8, g(x)= 3x - 1; Find (f ∘ g)(x).
A)9x + 5
B)9x + 11
C)9x + 7
D)9x + 23

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
11
For the given functions f and g, find the requested composite function.
f(x)=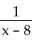 , g(x)=
, g(x)= 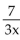 ; Find (f ∘ g)(x).
; Find (f ∘ g)(x).
A)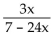
B)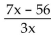
C)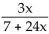
D)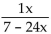
f(x)=
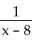 , g(x)=
, g(x)= 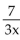 ; Find (f ∘ g)(x).
; Find (f ∘ g)(x).A)
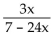
B)
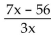
C)
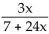
D)
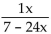

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
12
For the given functions f and g, find the requested composite function.
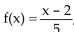 g(x)= 5x + 2; Find (g ∘ f)(x).
g(x)= 5x + 2; Find (g ∘ f)(x).
A)x
B)5x + 8
C)x + 4
D)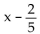
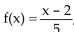 g(x)= 5x + 2; Find (g ∘ f)(x).
g(x)= 5x + 2; Find (g ∘ f)(x).A)x
B)5x + 8
C)x + 4
D)
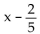

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
13
For the given functions f and g, find the requested composite function value.
f(x)= 7x + 8, g(x)=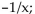 Find (g ∘ f)(3).
Find (g ∘ f)(3).
A)-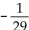
B)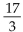
C)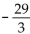
D)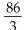
f(x)= 7x + 8, g(x)=
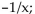 Find (g ∘ f)(3).
Find (g ∘ f)(3).A)-
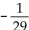
B)
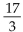
C)
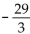
D)
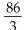

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
14
For the given functions f and g, find the requested composite function.
f(x)= -2x + 5, g(x)= 5x + 3; Find (g ∘ f)(x).
A)-10x + 28
B)-10x + 11
C)10x + 28
D)-10x - 22
f(x)= -2x + 5, g(x)= 5x + 3; Find (g ∘ f)(x).
A)-10x + 28
B)-10x + 11
C)10x + 28
D)-10x - 22

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
15
Evaluate the expression using the values given in the table.

A)1
B)8
C)7
D)Undefined

A)1
B)8
C)7
D)Undefined

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
16
For the given functions f and g, find the requested composite function value.
f(x)= 4x + 6,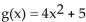 ; Find (f ∘ g)(0).
; Find (f ∘ g)(0).
A)26
B)149
C)30
D)105
f(x)= 4x + 6,
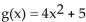 ; Find (f ∘ g)(0).
; Find (f ∘ g)(0).A)26
B)149
C)30
D)105

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
17
Evaluate the expression using the graphs of y = f(x)and y = g(x).
Evaluate (fg)(1).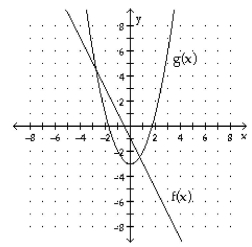
A)6
B)-5
C)3
D)0
Evaluate (fg)(1).

A)6
B)-5
C)3
D)0

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
18
For the given functions f and g, find the requested composite function value.
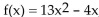 , g(x)= 15x - 8; Find (f ∘ g)(7).
, g(x)= 15x - 8; Find (f ∘ g)(7).
A)121,929
B)9,127
C)112,802
D)59,073
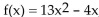 , g(x)= 15x - 8; Find (f ∘ g)(7).
, g(x)= 15x - 8; Find (f ∘ g)(7).A)121,929
B)9,127
C)112,802
D)59,073

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
19
For the given functions f and g, find the requested composite function value.
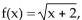 g(x)= 3x; Find (f ∘ g)(3).
g(x)= 3x; Find (f ∘ g)(3).
A)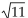
B)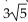
C)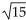
D)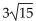
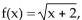 g(x)= 3x; Find (f ∘ g)(3).
g(x)= 3x; Find (f ∘ g)(3).A)
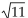
B)
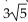
C)
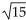
D)
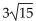

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
20
For the given functions f and g, find the requested composite function.
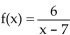 , g(x)=
, g(x)= 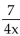 ; Find (f ∘ g)(x).
; Find (f ∘ g)(x).
A)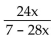
B)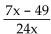
C)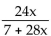
D)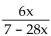
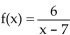 , g(x)=
, g(x)= 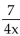 ; Find (f ∘ g)(x).
; Find (f ∘ g)(x).A)
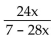
B)
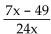
C)
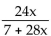
D)
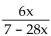

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
21
Find functions f and g so that f ∘ g = H.
H(x)=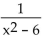
A)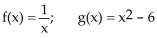
B)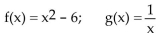
C)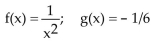
D)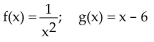
H(x)=
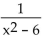
A)
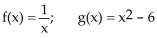
B)
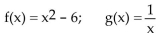
C)
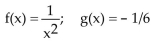
D)
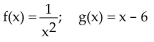

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
22
Solve the problem.
The population P of a predator mammal depends upon the number x of a smaller animal that is itsprimary food source. The population s of the smaller animal depends upon the amount a of a certain plantthat is its primary food source. If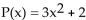 and
and 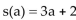 , what is the relationship between thepredator mammal and the plant food source?
, what is the relationship between thepredator mammal and the plant food source?
A)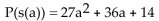
B)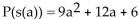
C)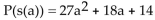
D)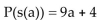
The population P of a predator mammal depends upon the number x of a smaller animal that is itsprimary food source. The population s of the smaller animal depends upon the amount a of a certain plantthat is its primary food source. If
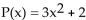 and
and 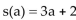 , what is the relationship between thepredator mammal and the plant food source?
, what is the relationship between thepredator mammal and the plant food source?A)
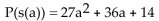
B)
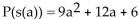
C)
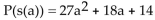
D)
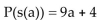

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
23
Find functions f and g so that f ∘ g = H.
H(x)=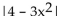
A)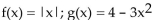
B)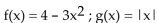
C)f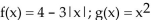
D)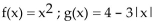
H(x)=
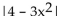
A)
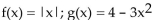
B)
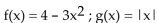
C)f
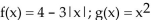
D)
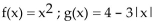

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
24
Solve the problem.
An oil well off the Gulf Coast is leaking, with the leak spreading oil over the surface of the gulf as a circle.At any time t, in minutes, after the beginning of the leak, the radius of the oil slick on the surface isr(t)= 4t ft. Find the area A of the oil slick as a function of time.
A)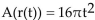
B)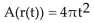
C)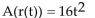
D)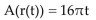
An oil well off the Gulf Coast is leaking, with the leak spreading oil over the surface of the gulf as a circle.At any time t, in minutes, after the beginning of the leak, the radius of the oil slick on the surface isr(t)= 4t ft. Find the area A of the oil slick as a function of time.
A)
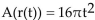
B)
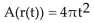
C)
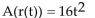
D)
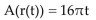

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
25
Find functions f and g so that f ∘ g = H.
H(x)=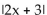
A)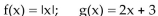
B)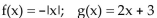
C)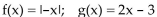
D)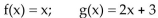
H(x)=
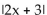
A)
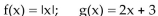
B)
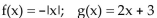
C)
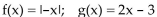
D)
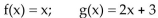

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
26
Find functions f and g so that f ∘ g = H.
H(x)=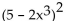
A)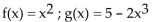
B)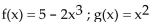
C)
D)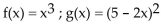
H(x)=
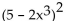
A)
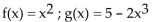
B)
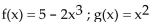
C)

D)
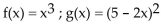

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
27
For the given functions f and g, find the requested composite function.
f(x)= x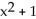 , g(x)=
, g(x)= 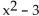 ; Find (f ∘ g)(x).
; Find (f ∘ g)(x).
A)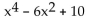
B)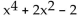
C)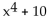
D)
f(x)= x
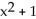 , g(x)=
, g(x)= 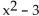 ; Find (f ∘ g)(x).
; Find (f ∘ g)(x).A)
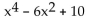
B)
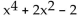
C)
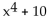
D)


Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
28
Decide whether the composite functions, f ∘ g and g ∘ f, are equal to x.
f(x)=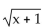 , g(x)=
, g(x)= 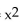
A)No, no
B)No, yes
C)Yes, no
D)Yes, yes
f(x)=
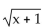 , g(x)=
, g(x)= 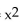
A)No, no
B)No, yes
C)Yes, no
D)Yes, yes

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
29
Find functions f and g so that f ∘ g = H.
H(x)=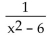
A)f(x)=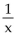 , g(x)=
, g(x)= 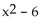
B)f(x)=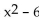 ; g(x)=
; g(x)= 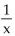
C)f(x)=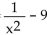 ; g(x)=
; g(x)= 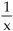
D)f(x)=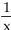 ; g(x)=
; g(x)= 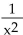 - 9
- 9
H(x)=
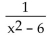
A)f(x)=
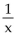 , g(x)=
, g(x)= 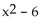
B)f(x)=
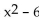 ; g(x)=
; g(x)= 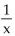
C)f(x)=
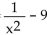 ; g(x)=
; g(x)= 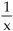
D)f(x)=
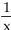 ; g(x)=
; g(x)= 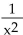 - 9
- 9
Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
30
Decide whether the composite functions, f ∘ g and g ∘ f, are equal to x.
f(x)=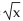 , g(x)=
, g(x)= 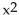
A)Yes, yes
B)No, no
C)No, yes
D)Yes, no
f(x)=
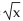 , g(x)=
, g(x)= 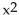
A)Yes, yes
B)No, no
C)No, yes
D)Yes, no

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
31
Find functions f and g so that f ∘ g = H.
H(x)=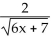
A)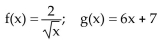
B)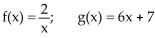
C)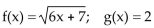
D)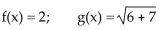
H(x)=
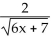
A)
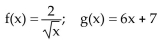
B)
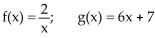
C)
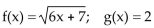
D)
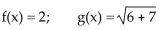

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
32
Decide whether the composite functions, f ∘ g and g ∘ f, are equal to x.
f(x)=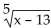 , g(x)=
, g(x)= 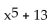
A)Yes, yes
B)No, no
C)No, yes
D)Yes, no
f(x)=
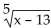 , g(x)=
, g(x)= 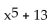
A)Yes, yes
B)No, no
C)No, yes
D)Yes, no

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
33
Decide whether the composite functions, f ∘ g and g ∘ f, are equal to x.
f(x)=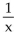 , g(x)= x
, g(x)= x
A)No, no
B)No, yes
C)Yes, no
D)Yes, yes
f(x)=
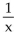 , g(x)= x
, g(x)= xA)No, no
B)No, yes
C)Yes, no
D)Yes, yes

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
34
Decide whether the composite functions, f ∘ g and g ∘ f, are equal to x.
f(x)=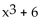 , g(x)=
, g(x)= 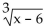
A)Yes, yes
B)No, no
C)No, yes
D)Yes, no
f(x)=
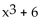 , g(x)=
, g(x)= 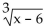
A)Yes, yes
B)No, no
C)No, yes
D)Yes, no

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
35
Find functions f and g so that f ∘ g = H.
H(x)= ∣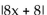
A)f(x)= ∣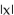 ; g(x)= 8x + 8
; g(x)= 8x + 8
B)f(x)=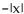 g(x)= 8x + 8
g(x)= 8x + 8
C)f(x)=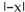 g(x)= 8x - 8
g(x)= 8x - 8
D)f(x)= x; g(x)= 8x + 8
H(x)= ∣
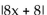
A)f(x)= ∣
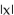 ; g(x)= 8x + 8
; g(x)= 8x + 8B)f(x)=
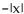 g(x)= 8x + 8
g(x)= 8x + 8C)f(x)=
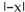 g(x)= 8x - 8
g(x)= 8x - 8D)f(x)= x; g(x)= 8x + 8

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
36
Decide whether the composite functions, f ∘ g and g ∘ f, are equal to x.
f(x)=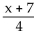 , g(x)= 4x - 7
, g(x)= 4x - 7
A)Yes, yes
B)No, no
C)Yes, no
D)No, yes
f(x)=
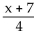 , g(x)= 4x - 7
, g(x)= 4x - 7A)Yes, yes
B)No, no
C)Yes, no
D)No, yes

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
37
Find functions f and g so that f ∘ g = H.
H(x)=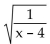
A)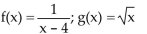
B)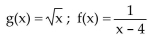
C)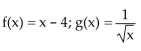
D)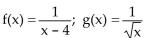
H(x)=
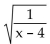
A)
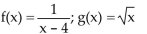
B)
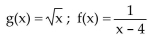
C)
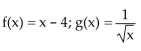
D)
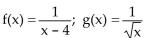

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
38
Decide whether the composite functions, f ∘ g and g ∘ f, are equal to x.
f(x)= 4x, g(x)=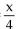
A)Yes, yes
B)No, no
C)Yes, no
D)No, yes
f(x)= 4x, g(x)=
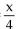
A)Yes, yes
B)No, no
C)Yes, no
D)No, yes

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
39
Find functions f and g so that f ∘ g = H.
H(x)=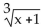
A)f(x)=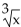 ; g(x)= x + 1
; g(x)= x + 1
B)f(x)= x + 1 ; g(x)=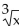
C)f(x)=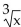 ; g(x)= 1
; g(x)= 1
D)f(x)=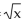 g(x)= x + 1
g(x)= x + 1
H(x)=
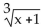
A)f(x)=
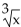 ; g(x)= x + 1
; g(x)= x + 1B)f(x)= x + 1 ; g(x)=
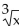
C)f(x)=
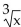 ; g(x)= 1
; g(x)= 1D)f(x)=
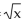 g(x)= x + 1
g(x)= x + 1
Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
40
Decide whether the composite functions, f ∘ g and g ∘ f, are equal to x.
f(x)=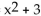 , g(x)=
, g(x)= 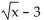
A)No, no
B)No, yes
C)Yes, no
D)Yes, yes
f(x)=
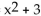 , g(x)=
, g(x)= 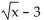
A)No, no
B)No, yes
C)Yes, no
D)Yes, yes

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
41
Find the domain of the composite function f ∘ g.
f(x)=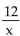 ; g(x)=
; g(x)= 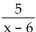
A)
B)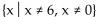
C)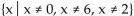
D)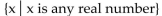
f(x)=
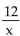 ; g(x)=
; g(x)= 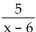
A)

B)
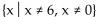
C)
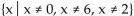
D)
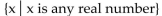

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
42
Write the word or phrase that best completes each statement or answers the question.
If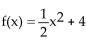 and g(x)= 2x - a, find a so that the graph of f ∘ g crosses the y-axis at 36.
and g(x)= 2x - a, find a so that the graph of f ∘ g crosses the y-axis at 36.
If
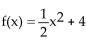 and g(x)= 2x - a, find a so that the graph of f ∘ g crosses the y-axis at 36.
and g(x)= 2x - a, find a so that the graph of f ∘ g crosses the y-axis at 36.
Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
43
Find the domain of the composite function f ∘ g.
f(x)= x + 4; g(x)=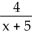
A)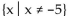
B)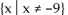
C)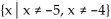
D)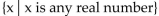
f(x)= x + 4; g(x)=
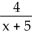
A)
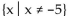
B)
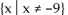
C)
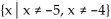
D)
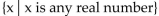

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
44
Indicate whether the function is one-to-one.
{(-10, 4), (-15, 4), (-16, 1)}
A)Yes
B)No
{(-10, 4), (-15, 4), (-16, 1)}
A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
45
Find the domain of the composite function f ∘ g.
f(x)=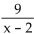 ; g(x)=
; g(x)= 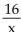
A)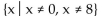
B)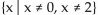
C)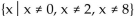
D)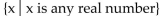
f(x)=
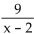 ; g(x)=
; g(x)= 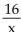
A)
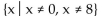
B)
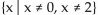
C)
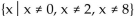
D)
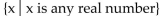

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
46
Find the domain of the composite function f ∘ g.
f(x)=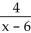 ; g(x)=
; g(x)= 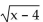
A)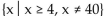
B)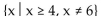
C)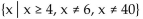
D)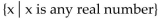
f(x)=
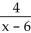 ; g(x)=
; g(x)= 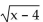
A)
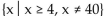
B)
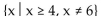
C)
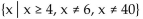
D)
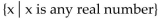

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
47
Write the word or phrase that best completes each statement or answers the question.
Solve the problem.
If f(x)=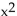 and g(x)= -1 + 5x, find (f ∘g)(x)and find the domain of (f ∘ g)(x).
and g(x)= -1 + 5x, find (f ∘g)(x)and find the domain of (f ∘ g)(x).
Solve the problem.
If f(x)=
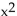 and g(x)= -1 + 5x, find (f ∘g)(x)and find the domain of (f ∘ g)(x).
and g(x)= -1 + 5x, find (f ∘g)(x)and find the domain of (f ∘ g)(x).
Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
48
Find the domain of the composite function f ∘ g.
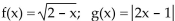
A)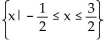
B)all real numbers
C)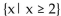
D)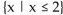
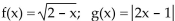
A)
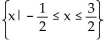
B)all real numbers
C)
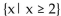
D)
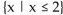

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
49
Find the domain of the composite function f ∘ g.
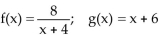
A)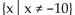
B)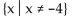
C)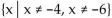
D)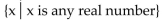
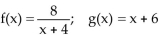
A)
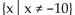
B)
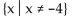
C)
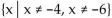
D)
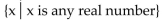

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
50
Find the domain of the composite function f ∘ g.
f(x)=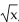 g(x)= 2x + 4
g(x)= 2x + 4
A)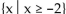
B)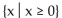
C)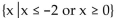
D)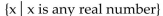
f(x)=
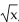 g(x)= 2x + 4
g(x)= 2x + 4A)
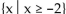
B)
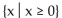
C)
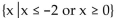
D)
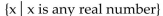

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
51
Write the word or phrase that best completes each statement or answers the question.
The volume V (in cubic inches)of a cylindrical pipe with length 12 inches is given by V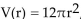 where ris the radius of the piston (in inches). If the radius is increasing with time t (in minutes)according to theformula
where ris the radius of the piston (in inches). If the radius is increasing with time t (in minutes)according to theformula 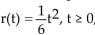 find the volume V of the pipe as a function of the time t.
find the volume V of the pipe as a function of the time t.
The volume V (in cubic inches)of a cylindrical pipe with length 12 inches is given by V
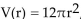 where ris the radius of the piston (in inches). If the radius is increasing with time t (in minutes)according to theformula
where ris the radius of the piston (in inches). If the radius is increasing with time t (in minutes)according to theformula 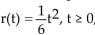 find the volume V of the pipe as a function of the time t.
find the volume V of the pipe as a function of the time t.
Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
52
Solve the problem.
The surface area of a balloon is given by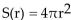 where r is the radius of the balloon. If the radius isincreasing with time t, as the balloon is being blown up, according to the formula
where r is the radius of the balloon. If the radius isincreasing with time t, as the balloon is being blown up, according to the formula 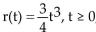 find thesurface area S as a function of the time t.
find thesurface area S as a function of the time t.
A)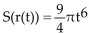
B)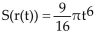
C)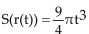
D)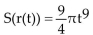
The surface area of a balloon is given by
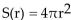 where r is the radius of the balloon. If the radius isincreasing with time t, as the balloon is being blown up, according to the formula
where r is the radius of the balloon. If the radius isincreasing with time t, as the balloon is being blown up, according to the formula 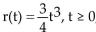 find thesurface area S as a function of the time t.
find thesurface area S as a function of the time t.A)
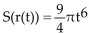
B)
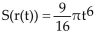
C)
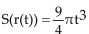
D)
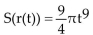

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
53
Write the word or phrase that best completes each statement or answers the question.
The surface area S (in square inches)of a cylindrical pipe with length 12 inches is given by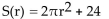 πr, where r is the radius of the piston (in inches). If the radius is increasing with time t (in minutes)according to the formula
πr, where r is the radius of the piston (in inches). If the radius is increasing with time t (in minutes)according to the formula 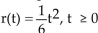 , find the surface area S of the pipe as a function of the time t.
, find the surface area S of the pipe as a function of the time t.
The surface area S (in square inches)of a cylindrical pipe with length 12 inches is given by
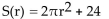 πr, where r is the radius of the piston (in inches). If the radius is increasing with time t (in minutes)according to the formula
πr, where r is the radius of the piston (in inches). If the radius is increasing with time t (in minutes)according to the formula 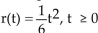 , find the surface area S of the pipe as a function of the time t.
, find the surface area S of the pipe as a function of the time t.
Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
54
Find the domain of the composite function f ∘ g.
f(x)= 7x + 42; g(x)= x + 10
A)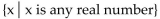
B)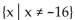
C)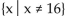
D)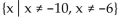
f(x)= 7x + 42; g(x)= x + 10
A)
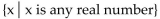
B)
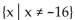
C)
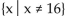
D)
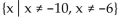

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
55
Indicate whether the function is one-to-one.
{(14, -17), (13, -6), (-16, -2)}
A)Yes
B)No
{(14, -17), (13, -6), (-16, -2)}
A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
56
Write the word or phrase that best completes each statement or answers the question.
The price p of a certain product and the quantity sold x obey the demand equation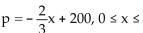 300. Suppose that the cost C of producing x units is
300. Suppose that the cost C of producing x units is 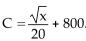 Assuming that all items produced aresold, find the cost C as a function of the price p.
Assuming that all items produced aresold, find the cost C as a function of the price p.
The price p of a certain product and the quantity sold x obey the demand equation
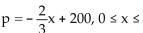 300. Suppose that the cost C of producing x units is
300. Suppose that the cost C of producing x units is 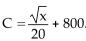 Assuming that all items produced aresold, find the cost C as a function of the price p.
Assuming that all items produced aresold, find the cost C as a function of the price p.
Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
57
Find the domain of the composite function f ∘ g.
f(x)=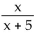 ; g(x)=
; g(x)= 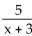
A)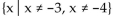
B)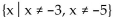
C)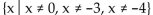
D)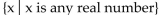
f(x)=
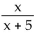 ; g(x)=
; g(x)= 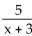
A)
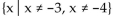
B)
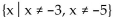
C)
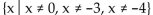
D)
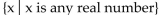

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
58
Solve the problem.
An airline charter service charges a fare per person of $200 plus $20 for each unsold seat. The airplaneholds 200 passengers. Let x represent the number of unsold seats and write an expression for the totalrevenue R for a charter flight.
A)R(x)= (200 - x)(200 + 20x)or 40,000 + 3,800x -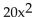
B)R(x)= 200(200 + 20x)or 40,000 + 4,000x
C)R(x)= (200 - x)(200 + 20x)or 40,000 + 4,000x -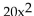
D)R(x)= x(200 + 20x)or 200x +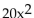
An airline charter service charges a fare per person of $200 plus $20 for each unsold seat. The airplaneholds 200 passengers. Let x represent the number of unsold seats and write an expression for the totalrevenue R for a charter flight.
A)R(x)= (200 - x)(200 + 20x)or 40,000 + 3,800x -
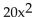
B)R(x)= 200(200 + 20x)or 40,000 + 4,000x
C)R(x)= (200 - x)(200 + 20x)or 40,000 + 4,000x -
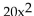
D)R(x)= x(200 + 20x)or 200x +
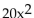

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
59
Find the domain of the composite function f ∘ g.
f(x)= 3x + 3; g(x)=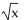
A)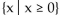
B)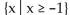
C)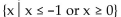
D)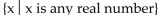
f(x)= 3x + 3; g(x)=
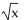
A)
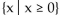
B)
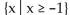
C)
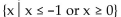
D)
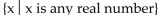

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
60
Find the domain of the composite function f ∘ g.
f(x)=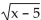 ; g(x)=
; g(x)= 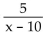
A)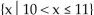
B)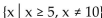
C)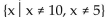
D)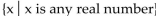
f(x)=
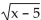 ; g(x)=
; g(x)= 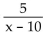
A)
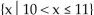
B)
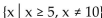
C)
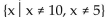
D)
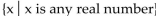

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
61
Use the horizontal line test to determine whether the function is one -to-one.
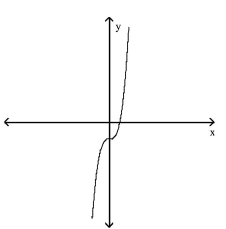
A)Yes
B)No

A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
62
Use the horizontal line test to determine whether the function is one -to-one.
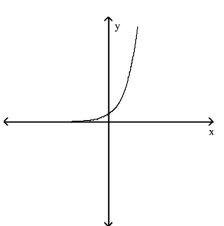
A)Yes
B)No

A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
63
Find the inverse of the function and state its domain and range .
{(6, -2), (2, -6), (8, -8), (-8, 8)}
A){(-2, 6), (-6, 2), (-8, 8), (8, -8)} D = {-2, -6, -8, 8}; R = {6, 2, 8, -8}
B)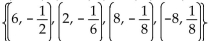 D = { 6, 2, 8, -8}, R =
D = { 6, 2, 8, -8}, R = 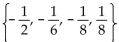
C){(8, 8), (8, 2), (-2, 6), (-8, -8)}; D = {8, 8, -2, -8}; R = {8, 2, 6, -8}
D){(8, 8), (-6, 2), (-2, 2), (-8, -8)}; D = {(8, -6, -2, -8}; R = {8, 2, -8}
{(6, -2), (2, -6), (8, -8), (-8, 8)}
A){(-2, 6), (-6, 2), (-8, 8), (8, -8)} D = {-2, -6, -8, 8}; R = {6, 2, 8, -8}
B)
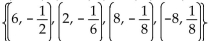 D = { 6, 2, 8, -8}, R =
D = { 6, 2, 8, -8}, R = 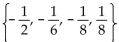
C){(8, 8), (8, 2), (-2, 6), (-8, -8)}; D = {8, 8, -2, -8}; R = {8, 2, 6, -8}
D){(8, 8), (-6, 2), (-2, 2), (-8, -8)}; D = {(8, -6, -2, -8}; R = {8, 2, -8}

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
64
Decide whether or not the functions are inverses of each other.
f(x)= 4x + 16, g(x)=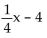
A)Yes
B)No
f(x)= 4x + 16, g(x)=
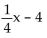
A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
65
The graph of a one-to-one function f is given. Draw the graph of the inverse function f  as a dashed line or curve.
as a dashed line or curve.
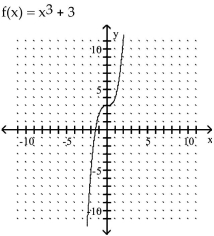
A)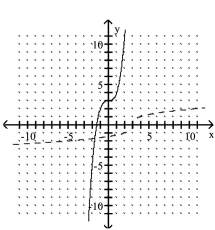
B)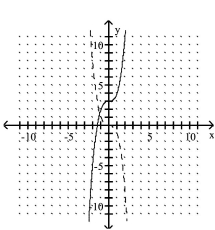
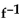 as a dashed line or curve.
as a dashed line or curve.
A)

B)


Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
66
Decide whether or not the functions are inverses of each other.
f(x)= 9x - 6, g(x)=
A)No
B)Yes
f(x)= 9x - 6, g(x)=

A)No
B)Yes

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
67
Indicate whether the function is one-to-one.
{(5, -3), (3, -5), (-4, 9), (4, -9)}
A)Yes
B)No
{(5, -3), (3, -5), (-4, 9), (4, -9)}
A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
68
The graph of a one-to-one function f is given. Draw the graph of the inverse function f  as a dashed line or curve.
as a dashed line or curve.
f(x)= 4x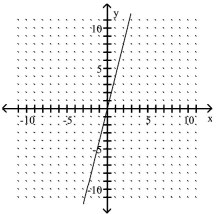
A)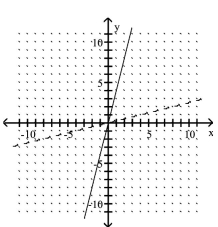
B)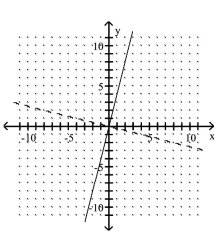
 as a dashed line or curve.
as a dashed line or curve.f(x)= 4x

A)

B)


Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
69
Indicate whether the function is one-to-one.
{(-5, -9), (-4, -9), (-3, 7), (-2, 4)}
A)Yes
B)No
{(-5, -9), (-4, -9), (-3, 7), (-2, 4)}
A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
70
Find the inverse. Determine whether the inverse represents a function.
{(6, -10), (-4, -9), (-6, -8), (-8, -7)}
A){(-10, 6), (-9, -4), (-8, -6), (-7, -8)}; a function
B){(-10, 6), (-9, -4), (-8, -6), (-7, -8)}; not a function
C){(-9, -10), (-10, -6), (6, -4), (-9, -8)}; not a function
D){(-9, -10), (-7, -6), (6, -6), (-9, -8)}; a function
{(6, -10), (-4, -9), (-6, -8), (-8, -7)}
A){(-10, 6), (-9, -4), (-8, -6), (-7, -8)}; a function
B){(-10, 6), (-9, -4), (-8, -6), (-7, -8)}; not a function
C){(-9, -10), (-10, -6), (6, -4), (-9, -8)}; not a function
D){(-9, -10), (-7, -6), (6, -6), (-9, -8)}; a function

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
71
Use the horizontal line test to determine whether the function is one -to-one.
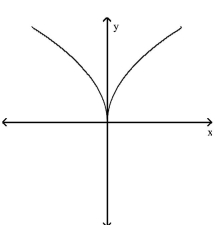
A)Yes
B)No

A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
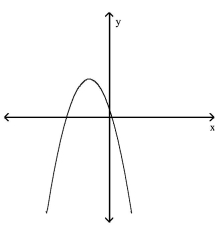
72
Use the graph of the given one-to-one function to sketch the graph of the inverse function. For convenience, the
graph of y = x is also given.
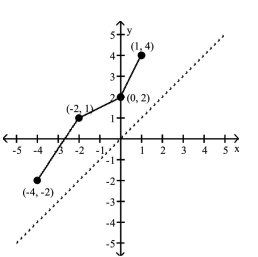
A)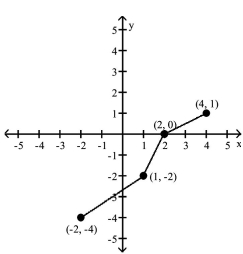
B)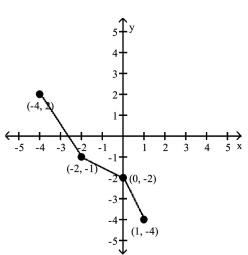
C)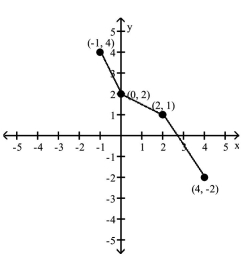
D)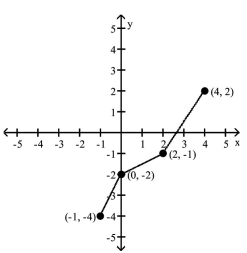
graph of y = x is also given.

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
73
The graph of a one-to-one function f is given. Draw the graph of the inverse function f  as a dashed line or curve.
as a dashed line or curve.
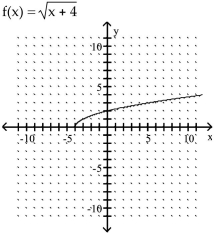
A)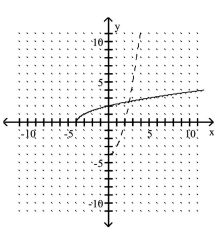
B)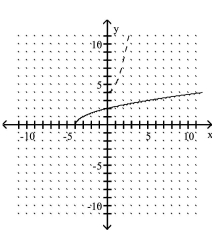
 as a dashed line or curve.
as a dashed line or curve.
A)

B)


Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
74
Use the horizontal line test to determine whether the function is one -to-one.
A)Yes
B)No

A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
75
The graph of a one-to-one function f is given. Draw the graph of the inverse function f  as a dashed line or curve.
as a dashed line or curve.
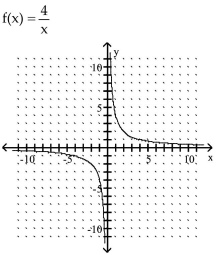
A)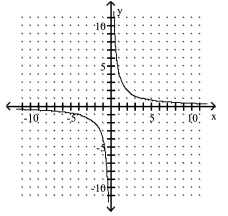 Function is its own inverse
Function is its own inverse
B)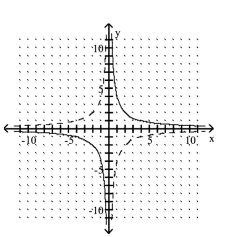
 as a dashed line or curve.
as a dashed line or curve.
A)
 Function is its own inverse
Function is its own inverseB)


Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
76
Indicate whether the function is one-to-one.
{(6, -4), (9, -3), (7, -2), (5, -1)}
A)Yes
B)No
{(6, -4), (9, -3), (7, -2), (5, -1)}
A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
77
Find the inverse of the function and state its domain and range .
{(-3, 4), (-1, 5), (0, 2), (2, 6), (5, 7)}
A){(4, -3), (5, -1), (2, 0), (6, 2), (7, 5)} D = {2, 4, 5, 6, 7}; R = {-3, -1, 0, 2, 5}
B){(3, 4), (1, 5), (0, 2), (-2, 6), (-5, 7)}; D = {3, 1, 0, -2, -5}; R = {2, 4, 5, 6, 7}
C){(-3, -4), (-1, -5), (0, -2), (2, -6), (5, -7)}; D = {-3, -1, 0, 2, 5}; R = {-7, -6, -5, -4, -2}
D){(3, -4), (1, -5), (0, -2), (-2, -6), (-5, -7)}; D = {3, 1, 0, -2, -5}; R = {-7, -6, -5, -4, -2}
{(-3, 4), (-1, 5), (0, 2), (2, 6), (5, 7)}
A){(4, -3), (5, -1), (2, 0), (6, 2), (7, 5)} D = {2, 4, 5, 6, 7}; R = {-3, -1, 0, 2, 5}
B){(3, 4), (1, 5), (0, 2), (-2, 6), (-5, 7)}; D = {3, 1, 0, -2, -5}; R = {2, 4, 5, 6, 7}
C){(-3, -4), (-1, -5), (0, -2), (2, -6), (5, -7)}; D = {-3, -1, 0, 2, 5}; R = {-7, -6, -5, -4, -2}
D){(3, -4), (1, -5), (0, -2), (-2, -6), (-5, -7)}; D = {3, 1, 0, -2, -5}; R = {-7, -6, -5, -4, -2}

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
78
Find the inverse of the function and state its domain and range .
{(7, 2), (5, 3), (3, 4), (1, 5)}
A){(2, 7), (3, 5), (4, 3), (5, 1)}; D = {2, 3, 4, 5 }; R = {7, 5, 3, 1}
B)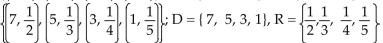
C){(3, 2), (2, 3), (7, 5), (3, 4)}; D = {(3, 2, 7}; R = {(2, 3 5, 4}
D){(3, 2), (5, 3), (7, 3), (3, 4)}; D = {3, 5, 7}; R = {2, 3, 4}
{(7, 2), (5, 3), (3, 4), (1, 5)}
A){(2, 7), (3, 5), (4, 3), (5, 1)}; D = {2, 3, 4, 5 }; R = {7, 5, 3, 1}
B)
C){(3, 2), (2, 3), (7, 5), (3, 4)}; D = {(3, 2, 7}; R = {(2, 3 5, 4}
D){(3, 2), (5, 3), (7, 3), (3, 4)}; D = {3, 5, 7}; R = {2, 3, 4}

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
79
Use the horizontal line test to determine whether the function is one -to-one.
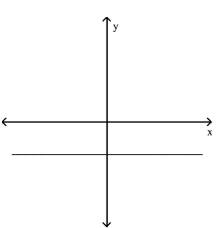
A)Yes
B)No

A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck
80
Use the horizontal line test to determine whether the function is one -to-one.
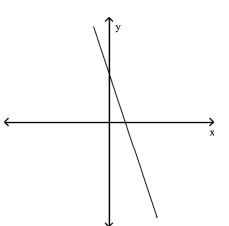
A)Yes
B)No

A)Yes
B)No

Unlock Deck
Unlock for access to all 234 flashcards in this deck.
Unlock Deck
k this deck



