Deck 5: Exponential and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/94
Play
Full screen (f)
Deck 5: Exponential and Logarithmic Functions
1
Use the functions given by and to find the value
A)32
B)35
C)33
D)37
E)30
A)32
B)35
C)33
D)37
E)30
32
2
Show that and are functions by using the definition of inverse functions.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Use the functions given by and to find the composition of functions
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Determine whether the function has an inverse function, If it does, find its inverse function.
A)No, does not have an inverse function.
B)Yes, has an inverse function,
C)Yes, has an inverse function,
D)Yes, has an inverse function,
E)Yes, has an inverse function,
A)No, does not have an inverse function.
B)Yes, has an inverse function,
C)Yes, has an inverse function,
D)Yes, has an inverse function,
E)Yes, has an inverse function,

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
5
Use the functions given by and to find the composition of functions
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
6
Show that and are functions by using the definition of inverse functions.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
7
Find the inverse function of the function f given by the set of ordered pairs.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
8
Sketch the graphs of inverse functions in the same coordinate plane and show that the graphs are reflections of each other in the line
A)![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_1244_eac3_bdc5_c3cad95fdde6_TB8692_11.jpg)
B)![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_1244_eac5_bdc5_abf70ca2f8f0_TB8692_11.jpg)
C)![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_1244_eac7_bdc5_43a04bac2a62_TB8692_11.jpg)
D)![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_1245_11d9_bdc5_f33d2f774948_TB8692_11.jpg)
E)![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11edf8a2_813a_d4a0_9a35_33f7d1f709a5_TB8692_00.jpg)
A)
![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_1244_eac3_bdc5_c3cad95fdde6_TB8692_11.jpg)
B)
![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_1244_eac5_bdc5_abf70ca2f8f0_TB8692_11.jpg)
C)
![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_1244_eac7_bdc5_43a04bac2a62_TB8692_11.jpg)
D)
![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_1245_11d9_bdc5_f33d2f774948_TB8692_11.jpg)
E)
![<strong>Sketch the graphs of inverse functions f ( x ) = x ^ { 5 } - 2 , f ^ { - 1 } ( x ) = \sqrt [ 5 ] { x + 2 } in the same coordinate plane and show that the graphs are reflections of each other in the line y = x </strong> A) \begin{array} { l l r r } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 4 & - 2 & - 1 \\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} B) \begin{array} { l lrr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 2 & 1 \end{array} C) \begin{array} { l r rr } x & 0 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} D) \begin{array} { l l rr } x & - 1 & 0 & 1 \\ f ( x ) & - 3 & - 2 & - 1 \\\\ x & - 3 & - 2 & -1\\ f ^ { - 1 } ( x ) & - 1 & 0 & 1 \end{array} E) \begin{array}{llrr} x & -1 & 0 & -1 \\ f(x) & -3 & -2 & -1 \\\\ x & -3& 2& -1 \\ f^{-1}(x) & -1 & -2 & 1 \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11edf8a2_813a_d4a0_9a35_33f7d1f709a5_TB8692_00.jpg)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
9
Find the inverse function informally . Verify that and
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
10
Use the functions given by and to find the value
A)1
B)0
C)3
D)4
E)2
A)1
B)0
C)3
D)4
E)2

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
11
Find the inverse function of the function f given by the set of ordered pairs.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
12
Find the inverse function informally . Verify that and
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
13
Use the graph of to complete the table and to sketch the graph of 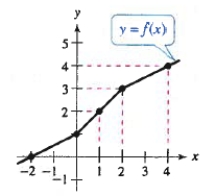
A)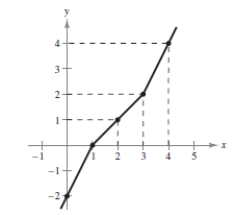
B)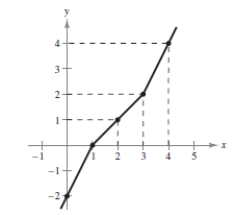
C)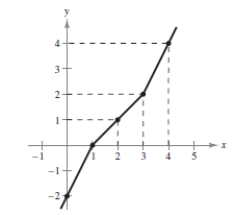
D)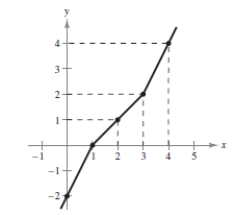
E)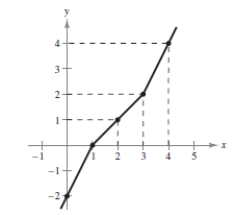

A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
14
Determine whether the function has an inverse function, If it does, find its inverse function.
A)Yes, has an inverse function,
B)No, does not have an inverse function.
C)Yes, has an inverse function,
D)Yes, has an inverse function,
E)Yes, has an inverse function,
A)Yes, has an inverse function,
B)No, does not have an inverse function.
C)Yes, has an inverse function,
D)Yes, has an inverse function,
E)Yes, has an inverse function,

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
15
Use the graph of to determine whether the function has an inverse function. 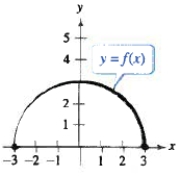
A)Yes, has an inverse function.
B)No, does not have an inverse function.

A)Yes, has an inverse function.
B)No, does not have an inverse function.

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
16
A company's profit for producing units is given by . Find the inverse function and explain what it represents. Describe the domains of and .
A) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
B) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
C) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
D) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
E) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
A) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
B) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
C) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
D) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of
E) , represents the number of units that must be sold to obtain the profit of .Domain of , Domain of

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
17
Sketch the graphs of inverse functions in the same coordinate plane and show that the graphs are reflections of each other in the line
A)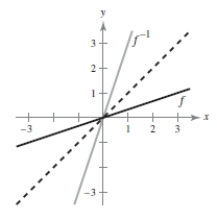
B)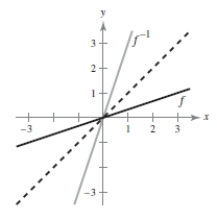
C)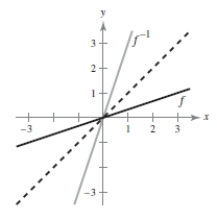
D)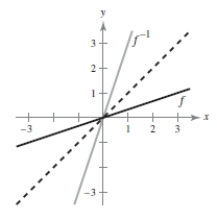
E)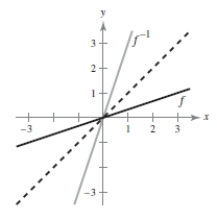
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
18
Determine whether the function has an inverse function, If it does, find its inverse function.
A)No, does not have an inverse function.
B)Yes, has an inverse function,
C)Yes, has an inverse function,
D)Yes, has an inverse function,
E)Yes, has an inverse function,
A)No, does not have an inverse function.
B)Yes, has an inverse function,
C)Yes, has an inverse function,
D)Yes, has an inverse function,
E)Yes, has an inverse function,

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
19
Use the graph of to determine whether the function has an inverse function. 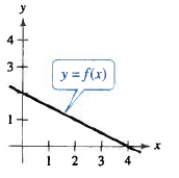
A)No, does not have an inverse function.
B)Yes, has an inverse function.

A)No, does not have an inverse function.
B)Yes, has an inverse function.

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
20
Use the functions given by and to find the value
A)16
B)-16
C)32
D)The value does not exist.
E)-32
A)16
B)-16
C)32
D)The value does not exist.
E)-32

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
21
Use a calculator to evaluate 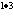 . Round your result to three decimal places.
. Round your result to three decimal places.
A)
B)
C)
D)
E)
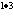 . Round your result to three decimal places.
. Round your result to three decimal places.A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
22
Sketch the graph of the function
A)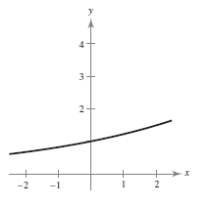
B)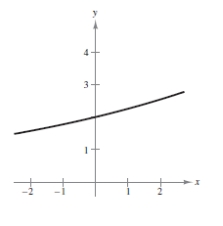
C)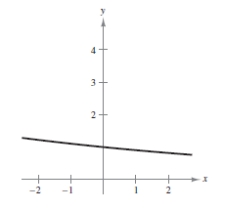
D)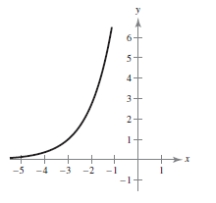
E)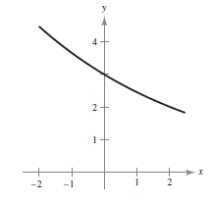
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
23
Use a calculator to evaluate the function for the given value of , . Round your result to three decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
24
You deposit a lump sum in a trust fund on the day your child is born. The fund earns 6.5% interest compounded continuously. Find the amount that will yield the given balance on your child's 25th birthday.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
25
Sketch the graph of the function .
A)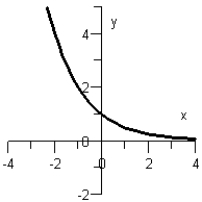
B)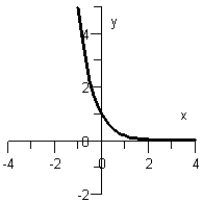
C)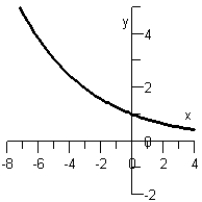
D)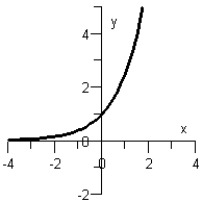
E)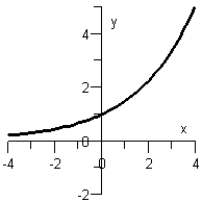
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
26
Evaluate the expression below. Round your results to three decimal places.
A)2.718
B)20.086
C)0.135
D)54.598
E)7.389
A)2.718
B)20.086
C)0.135
D)54.598
E)7.389

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
27
Match the function with its graph.
A)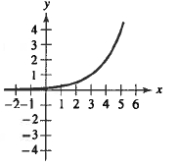
B)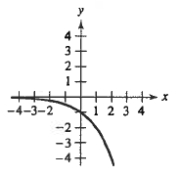
C)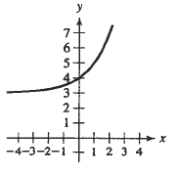
D)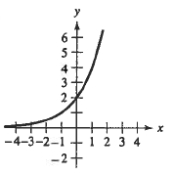
E)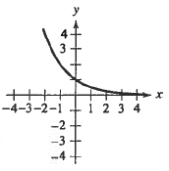
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
28
Strontinum-90 has a half life of 29.1 years.The amount S of 100 kilograms of Strontinum6990 present after t years is given by How much of the 100 kilograms will remain after 50 years?
A)about 31.4 kilograms
B)about 35.4 kilograms
C)about 30.4 kilograms
D)about 37.4 kilograms
E)about 40.4 kilograms
A)about 31.4 kilograms
B)about 35.4 kilograms
C)about 30.4 kilograms
D)about 37.4 kilograms
E)about 40.4 kilograms

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
29
The present value of money is the principal you need to invest today so that it will grow to an amount at the end of specified time. The present value formula is obtained by solving the compound interest formula for . Recall that is the number of years, is the interest rate per year, and is the number of compoundings per year. find the present value of amount invested at rate for years, compounded times per year.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
30
The present value of money is the principal you need to invest today so that it will grow to an amount at the end of specified time. The present value formula is obtained by solving the compound interest formula for . Recall that is the number of years, is the interest rate per year, and is the number of compoundings per year. find the present value of amount invested at rate for years, compounded times per year.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
31
Use a calculator to evaluate . Round your result to three decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
32
The demand function for a limited edition comic book is given by Find the price for a demand of units.
A)$5722.74
B)$277.26
C)$369.89
D)$340.13
E)$6369.89
A)$5722.74
B)$277.26
C)$369.89
D)$340.13
E)$6369.89

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
33
Sketch the graph of the function .
A)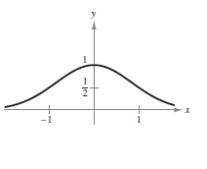
B)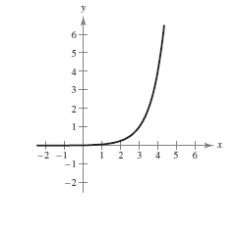
C)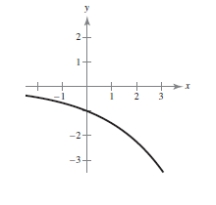
D)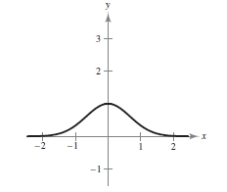
E)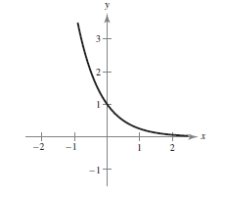
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
34
Sketch the graph of the function .
A)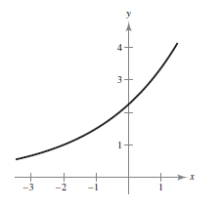
B)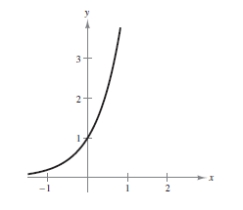
C)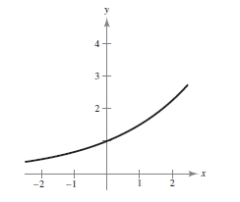
D)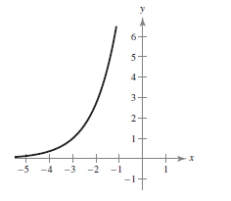
E)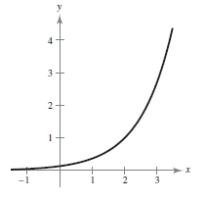
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
35
Sketch the graph of the function
A)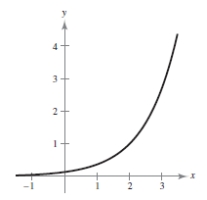
B)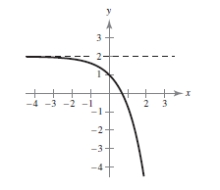
C)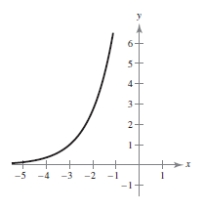
D)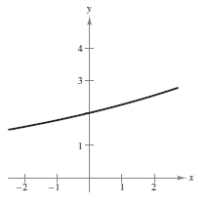
E)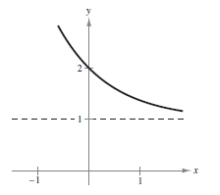
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
36
The number of certain type of bacteria increases according to the model where t is time (in hours)
a)Find P(0).
b)Find P(5).
c)Find P(10).
d)Find P(24).
A)a) b) c) d)
B)a) b) c) d)
C)a) b) c) d)
D)a) b) c) d)
E)a) b) c) d)
a)Find P(0).
b)Find P(5).
c)Find P(10).
d)Find P(24).
A)a) b) c) d)
B)a) b) c) d)
C)a) b) c) d)
D)a) b) c) d)
E)a) b) c) d)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
37
Determine whether . Justify your answer.
A)Yes, because is a rational number.
B)No, because is not a rational number.
A)Yes, because is a rational number.
B)No, because is not a rational number.

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
38
Match the function with its graph.
A)
B)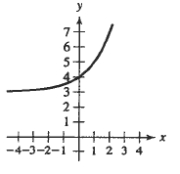
C)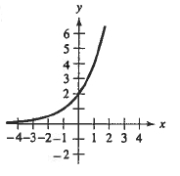
D)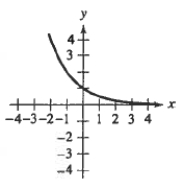
E)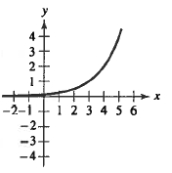
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
39
Determine which of the following functions is graphed below. 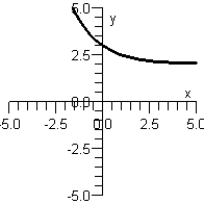
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
40
Use a calculator to evaluate the function for the given value of , . Round your result to three decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
41
Find the domain of the function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
42
Rewrite the exponential equation in logarithmic form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
43
Rewrite the logarithmic equation in exponential form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
44
Expand the given logarithmic expression. Assume all variable expressions represent positive real numbers.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
45
Evaluate the logarithm using the change of base formula. Round to 3 decimal places.
A)6.571
B)0.296
C)3.377
D)12.786
E)2.854
A)6.571
B)0.296
C)3.377
D)12.786
E)2.854

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
46
Expand the logarithmic expression . Assume all variable expressions represent positive real numbers.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
47
Find the exact value of without using a calculator.
A)
B)
C)
D)
E)-1
A)
B)
C)
D)
E)-1

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
48
Write the exponential equation in logarithmic form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
49
Write the expression below as a single logarithm with a coefficient of 1. Assume all variable expressions represent positive real numbers.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
50
Approximate the logarithm below using the properties of logarithms, given and
A)-0.2084
B)-0.2625
C)0.2084
D)0.2625
E)0.4709
A)-0.2084
B)-0.2625
C)0.2084
D)0.2625
E)0.4709

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
51
Condense the expression to the logarithm of a single term.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
52
Write the exponential equation in its logarithmic form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
53
Write the logarithmic equation in its exponential form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
54
Condense the expression to the logarithm of a single term.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
55
Match the function below with its graph. Graph I : 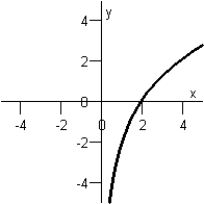 Graph IV:
Graph IV: 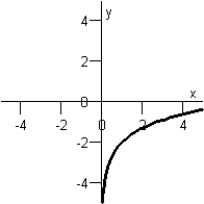 Graph II:
Graph II: 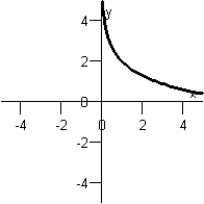 Graph V:
Graph V: 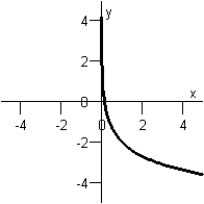 Graph III:
Graph III: 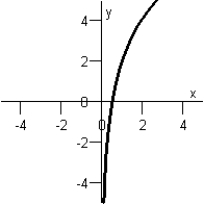
A)Graph III
B)Graph I
C)Graph II
D)Graph V
E)Graph IV
 Graph IV:
Graph IV:  Graph II:
Graph II:  Graph V:
Graph V:  Graph III:
Graph III: 
A)Graph III
B)Graph I
C)Graph II
D)Graph V
E)Graph IV

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
56
Write as a single logarithm with a coefficient of 1. Assume all variable expressions represent positive real numbers.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
57
Find the vertical asymptote of the logarithmic function below.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
58
Use the properties of logarithms to simplify the logarithmic expression below.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
59
Evaluate the logarithm without using a calculator.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
60
Write the logarithmic equation in exponential form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
61
Approximate the solution to . Round to 3 decimal places.
A)1.896
B)4.907
C)4.809
D)-0.446
E)316.979
A)1.896
B)4.907
C)4.809
D)-0.446
E)316.979

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
62
Solve for
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
63
Solve the exponential equation algebraically. Approximate the result to three decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
64
Use algebraic procedures to find the exact solution of the equation .
A)-1, 19
B)
C)2, 9
D)19
E)1
A)-1, 19
B)
C)2, 9
D)19
E)1

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
65
Approximate the solution to . Round to 3 decimal places.
A)-0.632
B)0.250
C)1.746
D)2.718
E)0.333
A)-0.632
B)0.250
C)1.746
D)2.718
E)0.333

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
66
Solve the exponential equation algebraically. Approximate the result to three decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
67
Use algebraic procedures to find the exact solution of the equation .
A)0, -20
B)25
C)3, 5
D)-5, 25
E)5
A)0, -20
B)25
C)3, 5
D)-5, 25
E)5

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
68
Use algebraic procedures to find the exact solution(s) of the equation below.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
69
Solve for x.
A)1
B)
C)
D)
E)no solution
A)1
B)
C)
D)
E)no solution

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
70
The approximate lengths and diameters (in inches) of common nails are shown in the table. Find a logarithmic equation that relates the diameter of a common nail to its length
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
71
Use algebraic procedures to find the exact solution(s) of the equation below.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
72
An initial investment of $4000 grows at an annual interest rate of 4% compounded continuously. How long will it take to double the investment?
A)17.33 years
B)18.33 years
C)18.00 years
D)17.00 years
E)1 year
A)17.33 years
B)18.33 years
C)18.00 years
D)17.00 years
E)1 year

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
73
Solve for x: . Round to 3 decimal places.
A)9.803
B)15.777
C)20.606
D)-20.606
E)-3.268
A)9.803
B)15.777
C)20.606
D)-20.606
E)-3.268

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
74
Solve for x: . Round to 3 decimal places.
A)3.407
B)0.407
C)1.362
D)-1.362
E)no solution
A)3.407
B)0.407
C)1.362
D)-1.362
E)no solution

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
75
The average monthly sales (in billions of dollars) in retail trade in the United States from 1996 to 2005 can be approximated by the model where represents the year, with corresponding to 1996. Estimate the year in which the average monthly sales first exceeded $310 billion.
A)2001
B)2004
C)2002
D)2000
E)1996
A)2001
B)2004
C)2002
D)2000
E)1996

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
76
An industrial psychologist has determined that the average percent score for an employee on a test of the employee's knowledge of the company's product is given by where t is the number of weeks on the job and P is the percent score. Estimate (to the nearest week) the expected number of weeks of employment that are necessary for an employee to earn a 85% score on the test.
A)38 weeks
B)37 weeks
C)42 weeks
D)60 weeks
E)33 weeks
A)38 weeks
B)37 weeks
C)42 weeks
D)60 weeks
E)33 weeks

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
77
Apply the Inverse Property of logarithmic or exponential functions to simplify the expression below.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
78
Approximate the solution of to 3 decimal places. (You may use a graphing utility.)
A)-6.682
B)0.164
C)-1.627
D)2.229
E)0.045
A)-6.682
B)0.164
C)-1.627
D)2.229
E)0.045

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
79
Use a graphing utility to approximate the solution to . Round to 3 decimal places.
A)6.179
B)5.179
C)3.089
D)2.589
E)no solution
A)6.179
B)5.179
C)3.089
D)2.589
E)no solution

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck
80
Solve for x.
A)
B)
C)
D)
E)2
A)
B)
C)
D)
E)2

Unlock Deck
Unlock for access to all 94 flashcards in this deck.
Unlock Deck
k this deck



