Deck 4: Polynomial and Rational Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/105
Play
Full screen (f)
Deck 4: Polynomial and Rational Functions
1
Match the equation with its graph.
A)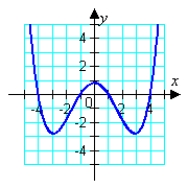
B)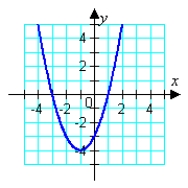
C)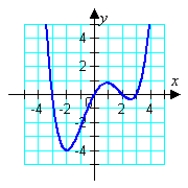
D)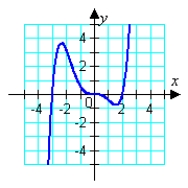
E)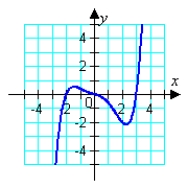
A)

B)

C)

D)

E)


2
Find all real zeros of the polynomial and determine the mutiplicity of each.
A) , multiplicity 2; , multiplicity 1; , multiplicity 1
B) , multiplicity 2; , multiplicity 2
C) , multiplicity 2; , multiplicity 1; , multiplicity 1
D) , multiplicity 2; , multiplicity 2
E) , multiplicity 1; , multiplicity 1; , multiplicity 1; , multiplicity 1
A) , multiplicity 2; , multiplicity 1; , multiplicity 1
B) , multiplicity 2; , multiplicity 2
C) , multiplicity 2; , multiplicity 1; , multiplicity 1
D) , multiplicity 2; , multiplicity 2
E) , multiplicity 1; , multiplicity 1; , multiplicity 1; , multiplicity 1
, multiplicity 2; , multiplicity 1; , multiplicity 1
3
The fuel efficiency, in miles per gallon, for a certain midsize car at various speeds, in miles per hour, is given in the table below. Find a quadratic model for these data.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
The height in feet of a projectile with an initial velocity of 128 feet per second and an initial height of 50 feet is a function of time t, in seconds, given by Find the maximum height of the projectile.
A)409 ft
B)306 ft
C)404 ft
D)162 ft
E)498 ft
A)409 ft
B)306 ft
C)404 ft
D)162 ft
E)498 ft

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
5
Examine the leading term and determine the far-left and far-right behavior of the graph of the polynomial function .
A)up to the far left, up to the far right
B)up to the far left, down to the far right
C)down to the far left, up to the far right
D)down to the far left, down to the far right
E)cannot be determined
A)up to the far left, up to the far right
B)up to the far left, down to the far right
C)down to the far left, up to the far right
D)down to the far left, down to the far right
E)cannot be determined

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
6
Graph the given function. 
A)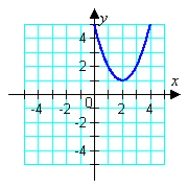
B)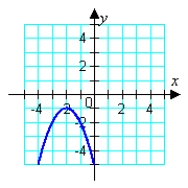
C)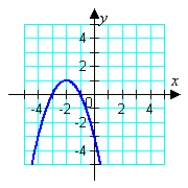
D)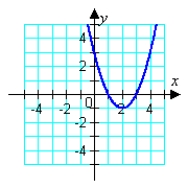
E)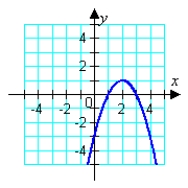

A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
7
Describe the right-hand and the left-hand behavior of the graph of .
A)Because the degree is odd and the leading coefficient is positive, the graph falls to the left and falls to the right.
B)Because the degree is odd and the leading coefficient is positive, the graph rises to the left and falls to the right.
C)Because the degree is odd and the leading coefficient is positive, the graph falls to the left and rises to the right.
D)Because the degree is odd and the leading coefficient is positive, the graph rises to the left and rises to the right.
E)Because the degree is even and the leading coefficient is positive, the graph rises to the left and rises to the right.
A)Because the degree is odd and the leading coefficient is positive, the graph falls to the left and falls to the right.
B)Because the degree is odd and the leading coefficient is positive, the graph rises to the left and falls to the right.
C)Because the degree is odd and the leading coefficient is positive, the graph falls to the left and rises to the right.
D)Because the degree is odd and the leading coefficient is positive, the graph rises to the left and rises to the right.
E)Because the degree is even and the leading coefficient is positive, the graph rises to the left and rises to the right.

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
8
Describe the right-hand and the left-hand behavior of the graph of .
A)Because the degree is even and the leading coefficient is negative, the graph falls to the left and falls to the right.
B)Because the degree is even and the leading coefficient is negative, the graph rises to the left and falls to the right.
C)Because the degree is even and the leading coefficient is negative, the graph falls to the left and rises to the right.
D)Because the degree is even and the leading coefficient is negative, the graph rises to the left and rises to the right.
E)Because the degree is odd and the leading coefficient is negative, the graph rises to the left and rises to the right.
A)Because the degree is even and the leading coefficient is negative, the graph falls to the left and falls to the right.
B)Because the degree is even and the leading coefficient is negative, the graph rises to the left and falls to the right.
C)Because the degree is even and the leading coefficient is negative, the graph falls to the left and rises to the right.
D)Because the degree is even and the leading coefficient is negative, the graph rises to the left and rises to the right.
E)Because the degree is odd and the leading coefficient is negative, the graph rises to the left and rises to the right.

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
9
A farmer has 192 feet of fencing and wants to build two identical pens for his prize-winning pigs. The pens will be arranged as shown. Determine the dimensions of a pen that will maximize its area. 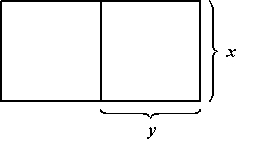
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
10
The path of a diver is given by where is the height (in feet) and is the horizontal distance from the end of the diving board (in feet). Find the maximum height of the diver.
A)13 feet
B)19 feet
C)10 feet
D)11 feet
E)16 feet
A)13 feet
B)19 feet
C)10 feet
D)11 feet
E)16 feet

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
11
Compare the graph of with .
A) shifts right units, shifts downward units, and shrinks by a factor of .
B) shifts right units, shifts upward units, and stretches by a factor of .
C) shifts left units, shifts downward units, and stretches by a factor of .
D) shifts right units, shifts upward units, and shrinks by a factor of .
E) shifts left units, shifts upward units, and stretches by a factor of .
A) shifts right units, shifts downward units, and shrinks by a factor of .
B) shifts right units, shifts upward units, and stretches by a factor of .
C) shifts left units, shifts downward units, and stretches by a factor of .
D) shifts right units, shifts upward units, and shrinks by a factor of .
E) shifts left units, shifts upward units, and stretches by a factor of .

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
12
Determine the vertex of the graph of the quadratic function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
13
Find all real zeros of the polynomial and determine the mutiplicity of each.
A) , multiplicity 2; , multiplicity 2
B) , multiplicity 2; , multiplicity 2
C) , multiplicity 2; , multiplicity 1
D) , multiplicity 2; , multiplicity 2
E) , multiplicity 1; , multiplicity 1; , multiplicity 1; , multiplicity 1
A) , multiplicity 2; , multiplicity 2
B) , multiplicity 2; , multiplicity 2
C) , multiplicity 2; , multiplicity 1
D) , multiplicity 2; , multiplicity 2
E) , multiplicity 1; , multiplicity 1; , multiplicity 1; , multiplicity 1

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
14
The sum of the length l and the width w of a rectangular region is 240 meters. Find the dimensions that produce the greatest area.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
15
Describe the right-hand and the left-hand behavior of the graph of .
A)Because the degree is odd and the leading coefficient is positive, the graph falls to the left and falls to the right.
B)Because the degree is odd and the leading coefficient is negative, the graph rises to the left and falls to the right.
C)Because the degree is odd and the leading coefficient is negative, the graph falls to the left and rises to the right.
D)Because the degree is odd and the leading coefficient is positive, the graph rises to the left and rises to the right.
E)Because the degree is even and the leading coefficient is negative, the graph rises to the left and falls to the right.
A)Because the degree is odd and the leading coefficient is positive, the graph falls to the left and falls to the right.
B)Because the degree is odd and the leading coefficient is negative, the graph rises to the left and falls to the right.
C)Because the degree is odd and the leading coefficient is negative, the graph falls to the left and rises to the right.
D)Because the degree is odd and the leading coefficient is positive, the graph rises to the left and rises to the right.
E)Because the degree is even and the leading coefficient is negative, the graph rises to the left and falls to the right.

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
16
Find an equation of the parabola below. 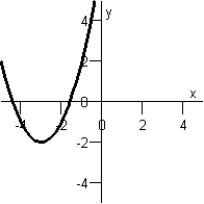
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
17
The sum of the length l and the width w of a rectangular region is 210 meters. Write the area A as a function of w.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
18
Find an equation of the parabola that has a vertex at and whose graph passes through the point
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
19
Determine the vertex of the graph of the quadratic function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
20
The fuel efficiency, in miles per gallon, for a certain midsize car at various speeds, in miles per hour, is given in the table below. Find a quadratic model for these data and use it to predict the fuel efficiency of this car when it is traveling at a speed of 40 mph. Do not round any values in your calculations but round the final answer to the nearest tenth.
A)33.7 mpg
B)35.7 mpg
C)32.7 mpg
D)33.9 mpg
E)34.9 mpg
A)33.7 mpg
B)35.7 mpg
C)32.7 mpg
D)33.9 mpg
E)34.9 mpg

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
21
Write in the form when .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
22
Use synthetic division to divide.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
23
Use synthetic division to divide.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
24
Using the factors and , find the remaining factor(s) of and write the polynomial in fully factored form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
25
Use synthetic division to divide by .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
26
Find the real zeros of the polynomial function and determine the multiplicity of each.
A) , multiplicity 2; , multiplicity 3
B) , multiplicity 2; , multiplicity 1; , multiplicity 2
C) , multiplicity 3; , multiplicity 2
D) , multiplicity 3; , multiplicity 2
E) , multiplicity 2; , multiplicity 2; , multiplicity 1
A) , multiplicity 2; , multiplicity 3
B) , multiplicity 2; , multiplicity 1; , multiplicity 2
C) , multiplicity 3; , multiplicity 2
D) , multiplicity 3; , multiplicity 2
E) , multiplicity 2; , multiplicity 2; , multiplicity 1

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
27
Simplify the rational expression, , by using long division or synthetic division.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
28
Sketch the graph of the polynomial function below.
A)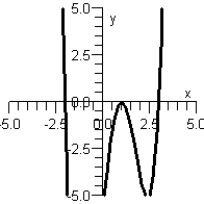
B)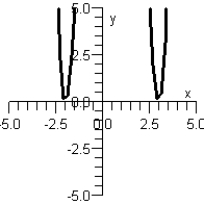
C)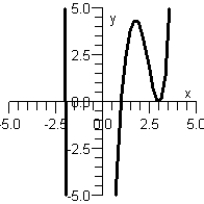
D)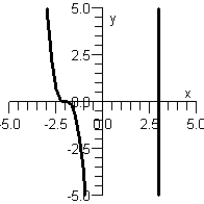
E)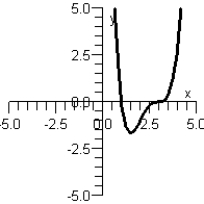
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
29
Find all the real zeros of the polynomial function and determine the multiplicity of each.
A) , multiplicity 2; , multiplicity 1
B) , multiplicity 1; , multiplicity 1; , multiplicity 1
C) , multiplicity 2; , multiplicity 1
D) , multiplicity 1; , multiplicity 1; , multiplicity 1
E) , multiplicity 3
A) , multiplicity 2; , multiplicity 1
B) , multiplicity 1; , multiplicity 1; , multiplicity 1
C) , multiplicity 2; , multiplicity 1
D) , multiplicity 1; , multiplicity 1; , multiplicity 1
E) , multiplicity 3

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
30
Using the factors and , find the remaining factor(s) of and write the polynomial in fully factored form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
31
Match the graph with one of the following functions below.  Function I :
Function I :
Function II :
Function III :
Function IV :
Function
A)Function IV
B)Function II
C)Function III
D)Function V
E)Function I
 Function I :
Function I : Function II :
Function III :
Function IV :
Function
A)Function IV
B)Function II
C)Function III
D)Function V
E)Function I

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
32
Sketch the graph of the polynomial function . Do not use a graphing calculator.
A)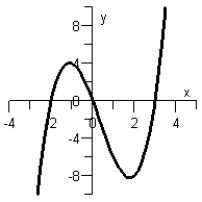
B)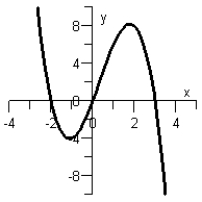
C)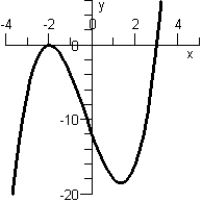
D)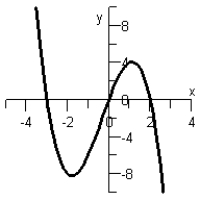
E)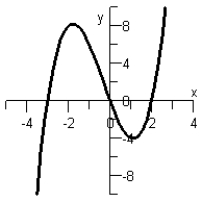
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
33
Use synthetic division to divide.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
34
Use long division to divide.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
35
Use long division to divide.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
36
Use synthetic division and the Factor Theorem to determine whether or not is a factor of .
A)Yes, is a factor.
B)No, is not a factor.
A)Yes, is a factor.
B)No, is not a factor.

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
37
Use long division to divide the first polynomial below by the second.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
38
Use long division to divide.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
39
Use synthetic division and the Factor Theorem to determine whether or not is a factor of .
A)No, is not a factor.
B)Yes, is a factor.
A)No, is not a factor.
B)Yes, is a factor.

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
40
A closed box is to be constructed from a rectangular sheet of cardboard that measures 25 inches by 12 inches. The box is made by cutting rectangles that measure x inches by 2x inches from two of the corners and by cutting two squares that measure x inches by x inches from the top and from the bottom of the rectangle, as shown in the following figure. Use a graphing calculator to determine what value of x (to the nearest thousandth of an inch) will produce a box with maximum volume? 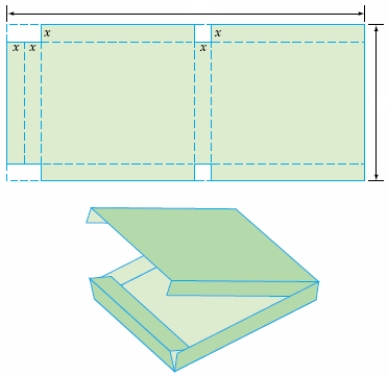
A)2.296
B)9.186
C)1.814
D)7.260
E)1.614

A)2.296
B)9.186
C)1.814
D)7.260
E)1.614

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
41
Find all the real zeros of .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
42
Find all the real zeros of .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
43
The length of a rectangular box is 1 inch more than twice the height of the box, and the width is 3 inches more than the height. If the volume of the box is 440 cubic inches, find the dimensions of the box. 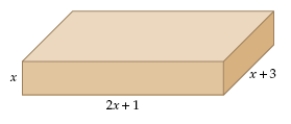
A)5 in. by 11 in. by 8 in.
B)4 in. by 9 in. by 7 in.
C)109 in. by 219 in. by 112 in.
D)5.5 in. by 12 in. by 8.5 in.
E)106 in. by 213 in. by 109 in.

A)5 in. by 11 in. by 8 in.
B)4 in. by 9 in. by 7 in.
C)109 in. by 219 in. by 112 in.
D)5.5 in. by 12 in. by 8.5 in.
E)106 in. by 213 in. by 109 in.

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
44
Simplify the rational expression, , by using long division or synthetic division.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
45
Use the Rational Zero Test to list all possible rational zeros of the polynomial function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
46
Find the zeros of the polynomial function below. If a zero is a multiple zero, state its multiplicity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
47
Evaluate the power of i.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
48
Use the Rational Zero Test to list possible rational zeros for the polynomial function below.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
49
An open box is to be made from a square piece of cardboard, 36 inches on a side, by cutting equal squares with sides of length x from the corners and turning up the sides (see figure below). After determining the function V, in terms of x, that represents the volume of the box, use a graphing utility to estimate the dimensions that will maximize its volume. 
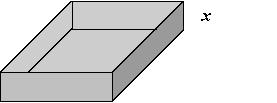
A) × ×
B) × ×
C) × ×
D) × ×
E) × ×


A) × ×
B) × ×
C) × ×
D) × ×
E) × ×

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
50
Simplify and write the following complex number in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
51
Find all real solutions of the polynomial equation .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
52
Use the zero or root feature of a graphing utility to approximate the real zeros of the function accurate to the nearest thousandth.
A) , 1.732, 1.414
B)-1.732, -1.414
C) 1.732, 1.414
D)1.732, 1.414
E) , - 1.732, 1.414
A) , 1.732, 1.414
B)-1.732, -1.414
C) 1.732, 1.414
D)1.732, 1.414
E) , - 1.732, 1.414

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
53
Write the complex number in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
54
Find real numbers a and b such that the equation is true.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
55
A farmer wants to enlarge an existing rectangular pig pen such that the total area of the new pen is twice as large as the original pen. The current pen's dimensions are 21' × 32'. The farmer wants to add 9 feet more to the long side than to the the short side. Find the dimensions of the new pen.
A)7 feet × 16 feet
B)28 feet × 48 feet
C)28 feet × 39 feet
D)28 feet × 37 feet
E)28 feet × 41 feet
A)7 feet × 16 feet
B)28 feet × 48 feet
C)28 feet × 39 feet
D)28 feet × 37 feet
E)28 feet × 41 feet

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
56
Find all real solutions of the polynomial equation .
A)
B)
C)
D)
E)
A)

B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
57
A company that produces video games estimates that the profit (in dollars) for selling a new game is given by where is the advertising expense (in tens of thousands of dollars). Using this model, how much should the company spend on advertising to obtain a profit of $6,100,000? Round to the nearest dollar.
A)$368,914 or $764,017
B)$410,790 or $737,145
C)$344,417 or $778,509
D)$433,844 or $721,190
E)$315,902 or $794,255
A)$368,914 or $764,017
B)$410,790 or $737,145
C)$344,417 or $778,509
D)$433,844 or $721,190
E)$315,902 or $794,255

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
58
Find all the rational zeros of the function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
59
Write the complex number in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
60
Find all the rational zeros of the function .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
61
Simplify and write the answer in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
62
Write as a product of linear factors given that and are zeros of the polynomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
63
Simplify and write the answer in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
64
Write as a product of linear factors.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
65
Use the quadratic formula to solve .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
66
Write the following expression as a complex number in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
67
Plot the complex number below.
A)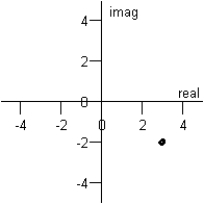
B)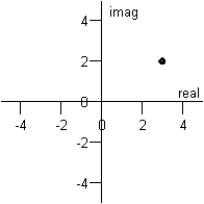
C)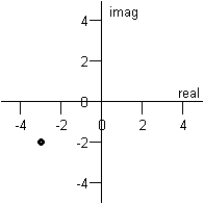
D)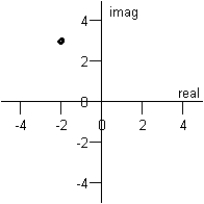
E)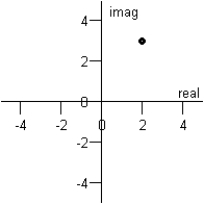
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
68
Combine and write the answer in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
69
Simplify and write the following complex number in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
70
Simplify and write the answer in standard form.
A)
B)
C) .
D)
E) .
A)
B)
C) .
D)
E) .

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
71
The demand and cost equations for a stethoscope are given by
and
where is the unit price (in dollars), is the total cost (in dollars), and is the number of units. The total profit (in dollars) obtained by producing and selling units is given by Determine a price that would yield a profit of $6.9 million.
A)$36.62
B)$48.83
C)$97.65
D)$109.86
E)$73.24
and
where is the unit price (in dollars), is the total cost (in dollars), and is the number of units. The total profit (in dollars) obtained by producing and selling units is given by Determine a price that would yield a profit of $6.9 million.
A)$36.62
B)$48.83
C)$97.65
D)$109.86
E)$73.24

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
72
Determine the number of zeros of the polynomial function below.
A)6
B)8
C)3
D)7
E)5
A)6
B)8
C)3
D)7
E)5

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
73
Simplify and write the answer in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
74
Write the polynomial as the product of factors that are irreducible over the rationals.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
75
Write as a product of linear factors given that and are zeros of the polynomial.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
76
Find a polynomial function with real coefficients that the given zeros.
A)Answers will vary. Sample answer:
B)Answers will vary. Sample answer:
C)Answers will vary. Sample answer:
D)Answers will vary. Sample answer:
E)Answers will vary. Sample answer:
A)Answers will vary. Sample answer:
B)Answers will vary. Sample answer:
C)Answers will vary. Sample answer:
D)Answers will vary. Sample answer:
E)Answers will vary. Sample answer:

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
77
Classify whether the following numbers are in the Mandelbrot Set. I : II : III :
A)Only numbers I and III are in the Mandelbrot Set.
B)Only number II is in the Mandelbrot Set.
C)Only numbers II and III are in the Mandelbrot Set.
D)Only numbers I and II are in the Mandelbrot Set.
E)None are in the Mandelbrot Set.
A)Only numbers I and III are in the Mandelbrot Set.
B)Only number II is in the Mandelbrot Set.
C)Only numbers II and III are in the Mandelbrot Set.
D)Only numbers I and II are in the Mandelbrot Set.
E)None are in the Mandelbrot Set.

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
78
Simplify and write the following complex number in standard form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
79
Simplify and write the following complex number in standard form.
A)
B)
C)
D)
E)0
A)
B)
C)
D)
E)0

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck
80
Find all the zeros of the polynomial function and write the polynomial as a product of its linear factors.
A) , , ;
B) , , ;
C) , , ;
D) , , ;
E) , , ;
A) , , ;
B) , , ;
C) , , ;
D) , , ;
E) , , ;

Unlock Deck
Unlock for access to all 105 flashcards in this deck.
Unlock Deck
k this deck



