Deck 11: Derivatives of Exponential and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/121
Play
Full screen (f)
Deck 11: Derivatives of Exponential and Logarithmic Functions
1
Use a graphing utility to graph the function .
A)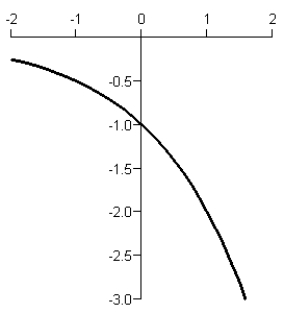
B)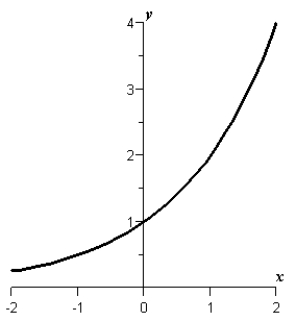
C)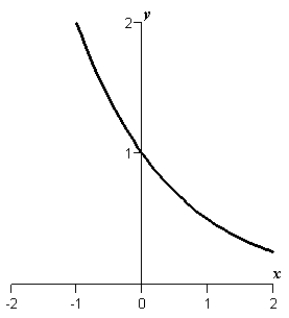
D)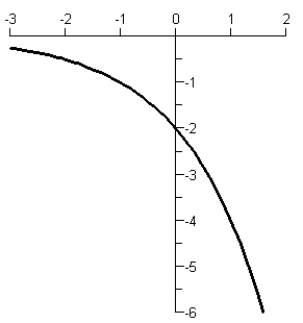
E)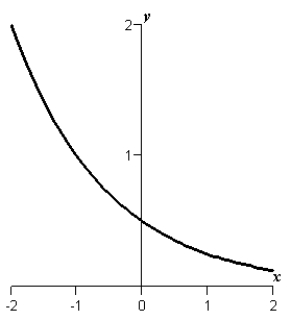
A)

B)

C)

D)

E)


2
Determine the continuity of the function below.
A)discontinuous at x = 0
B)continuous on the entire real number line
C)discontinuous at x = 1
D)discontinuous at x = 2
E)discontinuous at x = 4
A)discontinuous at x = 0
B)continuous on the entire real number line
C)discontinuous at x = 1
D)discontinuous at x = 2
E)discontinuous at x = 4
continuous on the entire real number line
3
With an annual rate of inflation of 4% over the next 10 years, the approximate cost of goods or services during any year in the decade is given by where is the time (in years) and is the present cost. The price of an oil change for a car is presently $24.95.Estimate the price 10 years from now.
A)$37.09
B)$36.93
C)$89.00
D)$63.90
A)$37.09
B)$36.93
C)$89.00
D)$63.90
$36.93
4
How much more interest will be earned if $6000 is invested for 6 years at an annual rate of 9% compounded continuously, instead of at 9% compounded quarterly?
A)$20.72
B)$40.72
C)$61.44
D)$994.60
E)$1035.32
A)$20.72
B)$40.72
C)$61.44
D)$994.60
E)$1035.32

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
5
Sketch the graph of the function .
A)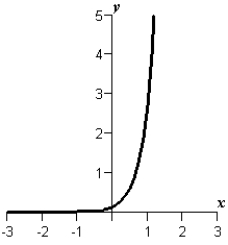
B)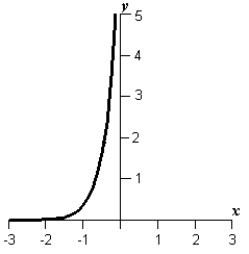
C)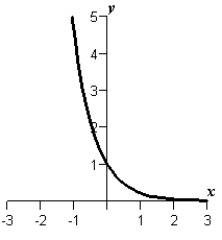
D)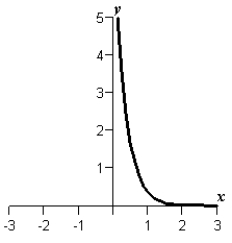
E)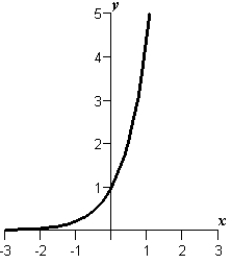
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
6
Use a graphing utility to graph the function . Be sure to choose an appropriate viewing window.
A)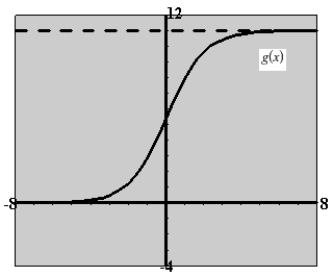
B)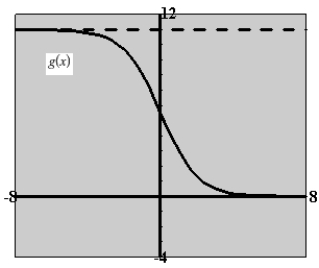
C)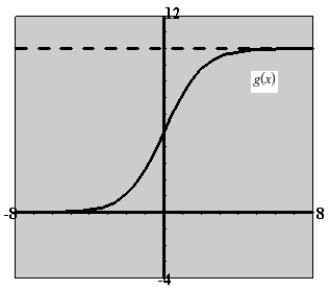
D)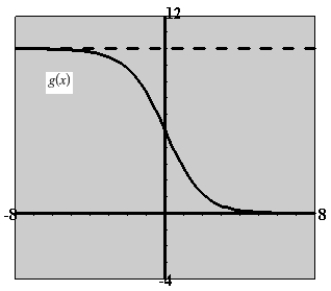
E)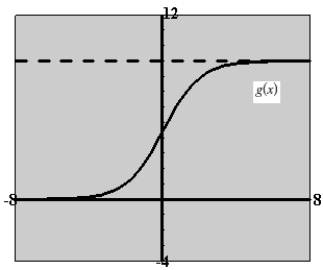
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
7
What lump sum should be deposited in an account that will earn at an annual rate of 12%, compounded quarterly, to grow to $90,000 for retirement in 15 years?
A)$87,176.69
B)$15,010.50
C)$10,975.61
D)$32,142.86
E)$15,275.98
A)$87,176.69
B)$15,010.50
C)$10,975.61
D)$32,142.86
E)$15,275.98

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
8
Assume the population P (in millions) of the United States from 1992 through 2005 can be modeled by the exponential function , where t is the time in years, with t = 2 corresponding to1992. Use the model to estimate the population in the year 2006. Round your answer to the nearest million.
A)4389 million
B)2733 million
C)7049 million
D)660 million
E)4388 million
A)4389 million
B)2733 million
C)7049 million
D)660 million
E)4388 million

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
9
Use a graphing utility to graph the function .
A)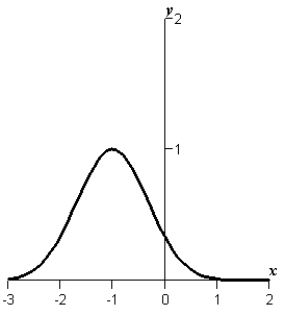
B)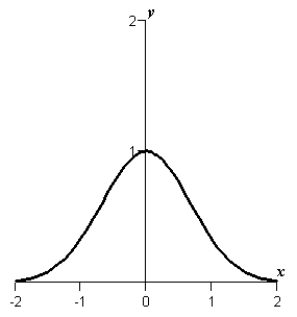
C)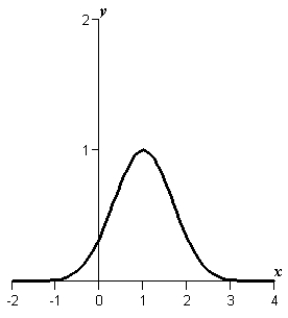
D)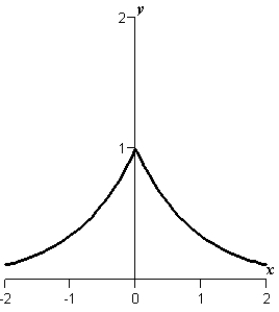
E)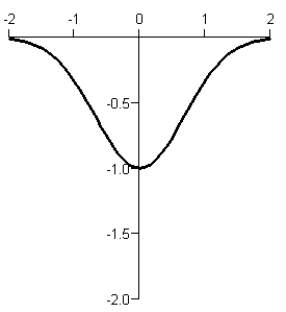
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
10
Sketch the graph of the function .
A)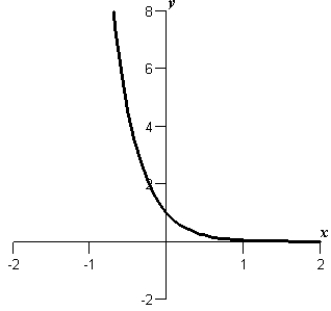
B)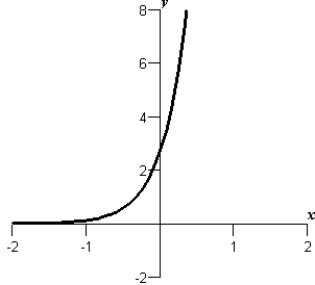
C)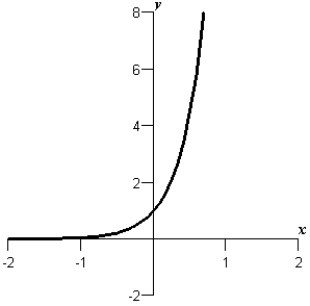
D)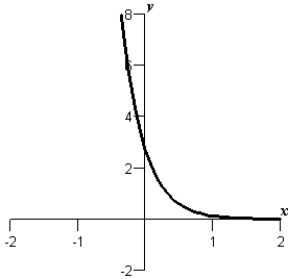
E)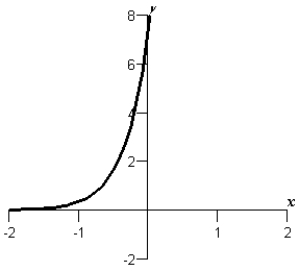
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
11
Determine whether the function below has any horizontal asymptotes.
A)horizontal asymptotes: y = 0 and y = 2
B)no horizontal asymptotes
C)horizontal asymptotes: y = 1
D)horizontal asymptotes: y = 1 and y = 3
E)horizontal asymptotes: y = 3
A)horizontal asymptotes: y = 0 and y = 2
B)no horizontal asymptotes
C)horizontal asymptotes: y = 1
D)horizontal asymptotes: y = 1 and y = 3
E)horizontal asymptotes: y = 3

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
12
After t years, the remaining mass y(in grams) of 16 grams of a radioactive element whose half-life is 32 years is given by , for . How much of the initial mass remains after 96 years? Round your answer to two decimal places.
A)4.00 grams
B)3.20 grams
C)4.30 grams
D)4.90 grams
E)2.00 grams
A)4.00 grams
B)3.20 grams
C)4.30 grams
D)4.90 grams
E)2.00 grams

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
13
Use the properties of exponents to simplify the expression .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
14
Use the properties of exponents to simplify the expression .
A)
B)343
C)
D)
E)49
A)
B)343
C)
D)
E)49

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
15
Sketch the graph of the function .
A)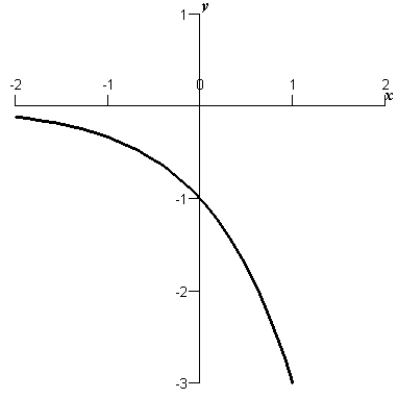
B)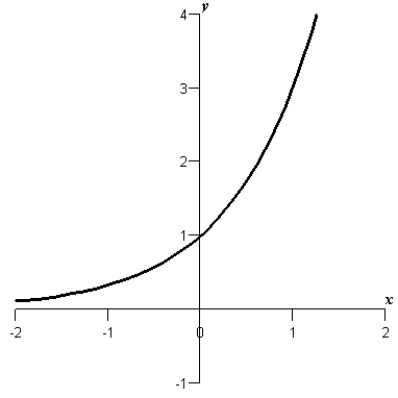
C)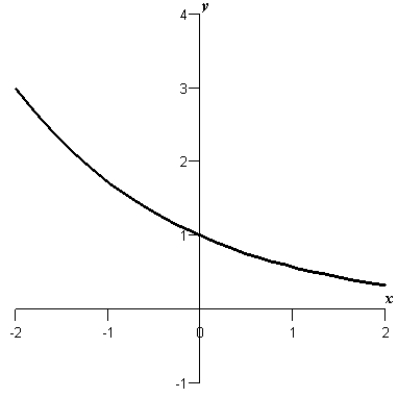
D)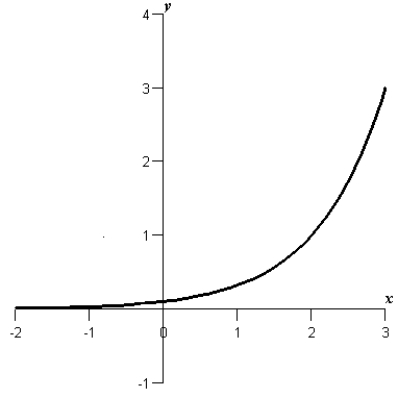
E)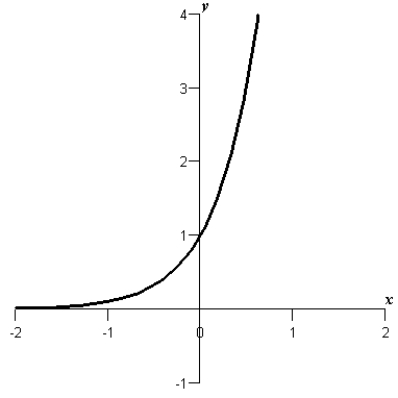
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
16
Suppose that the annual rate of inflation averages 4% over the next 10 years. With this rate of inflation, the approximate cost C of goods or services during any year in that decade will be given by C(t) = P(1.04)t, 0 10 where t is time in years and P is the present cost. If the price of an oil change for your car is presently 24.95, estimate the price 9 years from now. Round your answer to two decimal places.
A) 36.93
B) 37.51
C) 38.93
D) 40.51
E) 35.51
A) 36.93
B) 37.51
C) 38.93
D) 40.51
E) 35.51

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
17
What is the resulting balance if $5700 is invested for 6 years at an annual rate of 7% compounded monthly?
A) 5902.43
B) 5936.86
C) 8094.00
D) 8664.60
E) 11,811.92
A) 5902.43
B) 5936.86
C) 8094.00
D) 8664.60
E) 11,811.92

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
18
After t years, the value of a car that originally cost 17,000 depreciates so that each year it is worth of its value for the previous year. Find a model for V(t), the value of the car after t years.
A)V(t)= 17,000
B)V(t)= 17,000t
C)V(t)= 17,000
D)V(t)= 17,000
E)V(t)= 17,000t
A)V(t)= 17,000
B)V(t)= 17,000t
C)V(t)= 17,000
D)V(t)= 17,000
E)V(t)= 17,000t

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
19
To help their son buy a car on his 19th birthday, a boy's parents invest $1400 on his 10th birthday. If the investment pays an annual rate of 9% compounded continuously, how much is available on his 19th birthday?
A)$3118.94
B)$3147.07
C)$3040.65
D)$2534.00
E)$35,747.21
A)$3118.94
B)$3147.07
C)$3040.65
D)$2534.00
E)$35,747.21

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
20
Evaluate the expression .
A)256
B)4
C)16
D)18
E)20
A)256
B)4
C)16
D)18
E)20

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
21
What is the annual percentage yield (or effective annual rate) for a nominal rate of 9% compounded quarterly?
A)9.00%
B)9.38%
C)9.42%
D)9.31%
E)9.20%
A)9.00%
B)9.38%
C)9.42%
D)9.31%
E)9.20%

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
22
Use implicit differentiation to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
23
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
24
Find the future value if $5000 is invested for 2 years at an annual rate of 10% compounded quarterly.
A) 4000.00
B) 6077.53
C) 5253.12
D) 6050.00
E) 6092.01
A) 4000.00
B) 6077.53
C) 5253.12
D) 6050.00
E) 6092.01

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
25
Find if .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
26
Find the equation of the tangent line to at the point (0,1).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
27
Find the derivative of
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
28
If
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
29
If
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
30
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
31
Find if .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
32
Find the derivative of the following function.
A)
B)
C)
D) .
E)
A)
B)
C)
D) .
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
33
If
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
34
Find an equation of the tangent line to the graph of at the point (0,1) .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
35
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
36
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
37
Write the equation of the line tangent to the graph of at
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
38
The average time between incoming calls at a switchboard is 3 minutes. If a call has just come in, the probability that the next call will come within the next t minutes is . Find the probability that the next call will come within the next minute. Round your answer to two decimal places.
A)15.35%
B)1.54%
C)184.65%
D)17.58%
E)5.08%
A)15.35%
B)1.54%
C)184.65%
D)17.58%
E)5.08%

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
39
The demand function for a product is modeled by . Find the price of the product if the quantity demanded is x = 100. Round your answer to two decimal places where applicable.
A) 422.12
B) 2518.48
C) 491.72
D) 502.02
E) 2508.28
A) 422.12
B) 2518.48
C) 491.72
D) 502.02
E) 2508.28

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
40
The demand function for a product is modeled by . What is the limit of the price as x increases without bound? Round your answer to two decimal places where applicable.
A)The limit of the price as x increases without bound is -1.
B)The limit of the price as x increases without bound is 1.
C)The limit of the price as x increases without bound is 0.
D)The limit of the price as x increases without bound is .
E)The limit of the price as x increases without bound is .
A)The limit of the price as x increases without bound is -1.
B)The limit of the price as x increases without bound is 1.
C)The limit of the price as x increases without bound is 0.
D)The limit of the price as x increases without bound is .
E)The limit of the price as x increases without bound is .

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
41
Use the properties of logarithms to approximate given that and
A)-5.0434
B)-1.8246
C)0.4687
D)5.5267
E)5.0434
A)-5.0434
B)-1.8246
C)0.4687
D)5.5267
E)5.0434

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
42
Find if .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
43
Sketch the graph of the function .
A)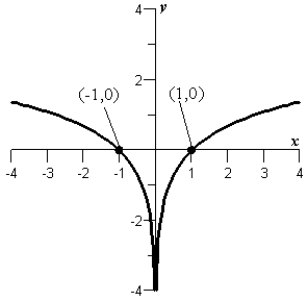
B)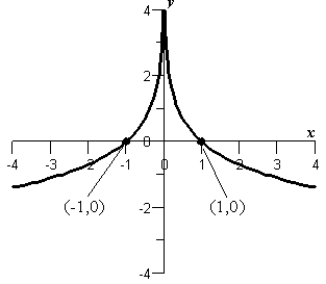
C)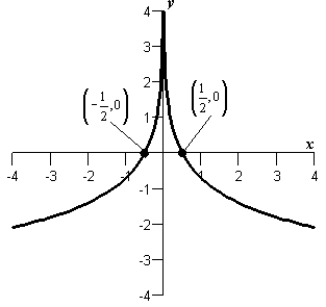
D)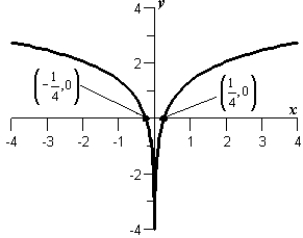
E)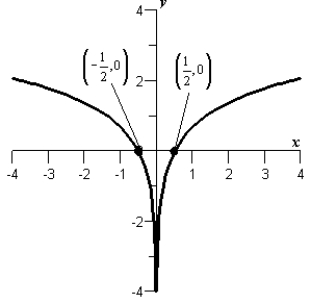
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
44
Use the properties of logarithms to expand
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
45
Find the extrema of the function by analyzing its graph below. 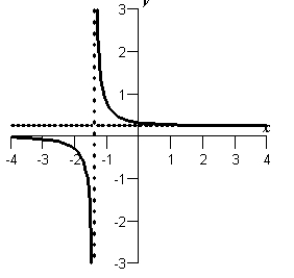
A)(0, 1)
B)no relative extrema
C) , (0, 0)
D)
E)

A)(0, 1)
B)no relative extrema
C) , (0, 0)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
46
Simplify .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
47
Solve for the equation for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
48
Sketch the graph of the function .
A)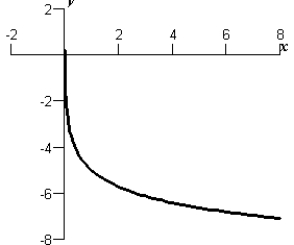
B)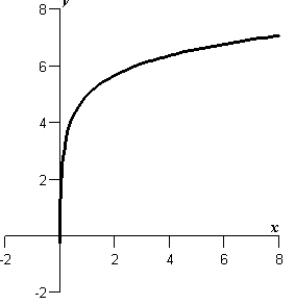
C)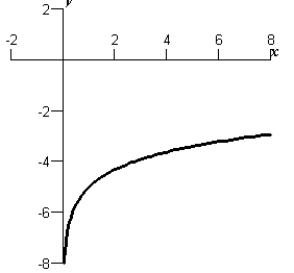
D)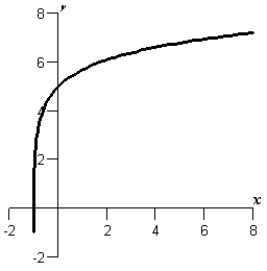
E)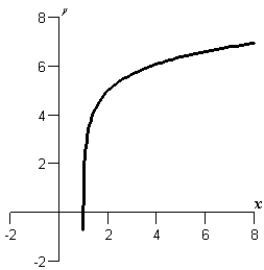
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
49
Use the properties of logarithms to expand .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
50
A survey of high school seniors from a certain school district who took the SAT has determined that the mean score on the mathematics portion was 650 with a standard deviation of 15.5. Assuming the data can be modeled by a normal probability density function, find a model for these data.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
51
Simplify
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
52
The average typing speed N (in words per minute) after t weeks of lessons is modeled by . Find the rate at which the typing speed is changing when t = 20 weeks. Round your answer to two decimal places.
A)2.40 words/min/week
B)2.70 words/min/week
C)3.71 words/min/week
D)4.93 words/min/week
E)6.24 words/min/week
A)2.40 words/min/week
B)2.70 words/min/week
C)3.71 words/min/week
D)4.93 words/min/week
E)6.24 words/min/week

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
53
Sketch the graph of the function .
A)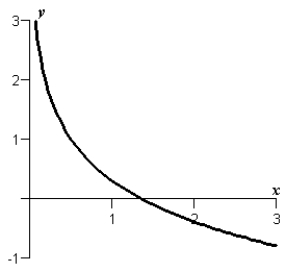
B)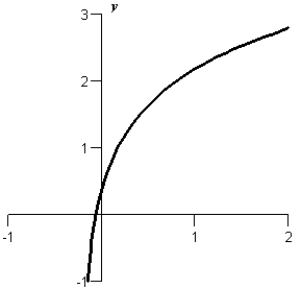
C)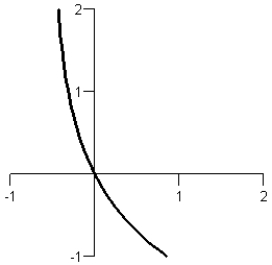
D)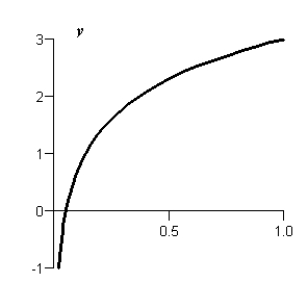
E)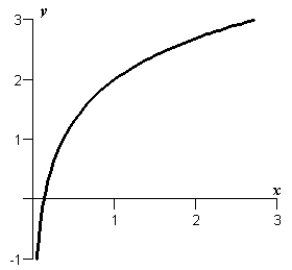
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
54
Write the logarithmic equation as an exponential equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
55
Use the properties of logarithms to write the expression as a single logarithm.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
56
A survey of high school seniors from a certain school district who took the SAT has determined that the mean score on the mathematics portion was 700 with a standard deviation of 13.5. By a normal probability density function the data can be modeled as . Find the derivative of the model.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
57
Use the properties of logarithms to write the expression as a sum, difference, or multiple of logarithms.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
58
Future value. The future value that accrues when $900 is invested at 5%, compounded continuously, is , where t is the number of years. At what rate is the money in this account growing when
A)$14.11 per year
B)$49.24 per year
C)$1411.48 per year
D)$941.43 per year
E)$70.57 per year
A)$14.11 per year
B)$49.24 per year
C)$1411.48 per year
D)$941.43 per year
E)$70.57 per year

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
59
Find the extrema of the function .
A)(0, 1)
B) , (0, 0)
C)
D)no relative extrema
E)
A)(0, 1)
B) , (0, 0)
C)
D)no relative extrema
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
60
Write the exponential equation as a logarithmic equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
61
Write the following expression as a logarithm of a single quantity.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
62
Solve the following equation for accurate to three decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
63
Solve the following equation for accurate to three decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
64
Solve for t. Round your answer to four decimal places.
A)1.4841
B)0.7412
C)3.0151
D)0.7421
E)2.7571
A)1.4841
B)0.7412
C)3.0151
D)0.7421
E)2.7571

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
65
Solve the exponential equation. Give answers correct to 3 decimal places.
A)625
B)0.714
C)1.000
D)0.453
E)313
A)625
B)0.714
C)1.000
D)0.453
E)313

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
66
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
67
Solve the exponential equation. Give the answer correct to 3 decimal places.
A)0.886
B)2.953
C)3.762
D)0.315
E)3.499
A)0.886
B)2.953
C)3.762
D)0.315
E)3.499

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
68
Solve the following equation for accurate to three decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
69
Solve the exponential equation. Give the answer correct to 3 decimal places.
A)-0.274
B)20.387
C)3.921
D)-20.387
E)-3.073
A)-0.274
B)20.387
C)3.921
D)-20.387
E)-3.073

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
70
Find the derivative of
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
71
Write the expression as the logarithm of a single quantity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
72
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
73
Write the following expression as a logarithm of a single quantity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
74
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
75
Write the expression as the logarithm of a single quantity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
76
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
77
Solve the exponential equation. Give the answer correct to 3 decimal places.
A)-2.434
B)-0.877
C)5.315
D)-0.532
E)24.336
A)-2.434
B)-0.877
C)5.315
D)-0.532
E)24.336

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
78
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
79
How long (in years) would $450 have to be invested at an annual rate of 12%, compounded continuously, to amount to $790?
A)6.30 years
B)4.97 years
C)1.13 years
D)5.68 years
E)4.69 years
A)6.30 years
B)4.97 years
C)1.13 years
D)5.68 years
E)4.69 years

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
80
Find the derivative of the following function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck



