Deck 12: Integration and Its Applications
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/74
Play
Full screen (f)
Deck 12: Integration and Its Applications
1
Find the cost function for the marginal cost and fixed cost of (for x = 0).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Use algebra to rewrite the integrand; then integrate and simplify.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Evaluate the integral .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Find the indefinite integral and check the result by differentiation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
5
A ball is thrown vertically upwards from a height of 10 ft with an initial velocity of 70 ft per second. How high will the ball go?
A)239.6875 ft
B)67.4219 ft
C)86.5625 ft
D)219.6875 ft
E)241.6875 ft
A)239.6875 ft
B)67.4219 ft
C)86.5625 ft
D)219.6875 ft
E)241.6875 ft

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
6
Find the indefinite integral and check your result by differentiation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
7
Identify u and for the integral .
A) and
B) and
C) and
D) and
E) and
A) and
B) and
C) and
D) and
E) and

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
8
Find the indefinite integral and check your result by differentiation.
A)
B)
C)4
D)
E)
A)
B)
C)4
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
9
Find the particular solution that satisfies the differential equation and initial condition .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
10
Find the indefinite integral and check the result by differentiation.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
11
Identify and for the integral .
A) and
B) and
C) and
D) and
E) and
A) and
B) and
C) and
D) and
E) and

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
12
Evaluate the integral .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
13
Evaluate the integral .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
14
An evergreen nursery sells a certain shrub after 4 years. The growth rate of the shrub is given by , where t is the time in years and h is the height in centimeters. The seedlings are 10 centimeters tall when planted (t = 0). How tall are the shrubs when they are sold?
A)36 centimeters
B)40 centimeters
C)44 centimeters
D)54 centimeters
E)70 centimeters
A)36 centimeters
B)40 centimeters
C)44 centimeters
D)54 centimeters
E)70 centimeters

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
15
Evaluate the integral .
A)
B)
C)
D)
E)
A)

B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
16
Evaluate the integral
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
17
Find a function that satisfies the conditions .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
18
Evaluate the integral .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
19
The graph of the derivative of a function is given below. Sketch the graphs of two functions that have the given derivative. 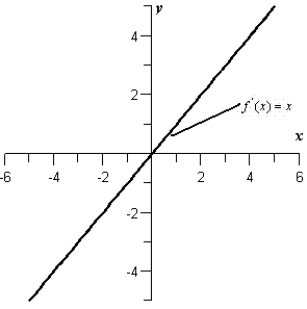
A)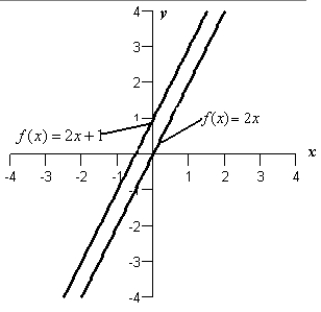
B)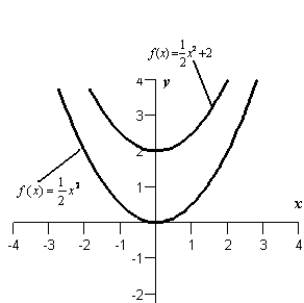
C)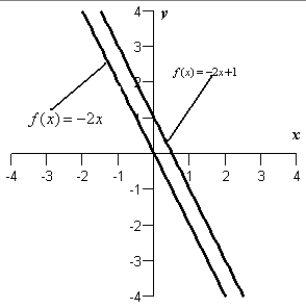
D)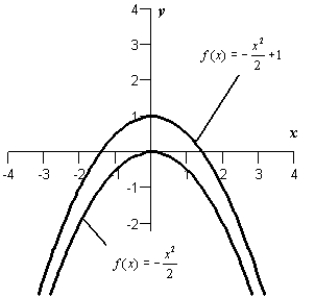
E)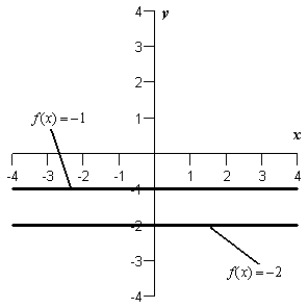

A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
20
Find the indefinite integral of the following function and check the result by differentiation.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
21
Find the equation of the function f whose graph passes through the point and whose derivative is .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
22
Find the indefinite integral of the following function and check the result by differentiation.
A)
B) .
C)
D)
E)
A)
B) .
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
23
Find the indefinite integral.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
24
Evaluate the integral
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
25
Find the indefinite integral.
A)
B)
C)
D)integral does not exist
E)none of the above
A)
B)
C)
D)integral does not exist
E)none of the above

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
26
Find the indefinite integral.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
27
Evaluate the integral
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
28
Use formal substitution to find the indefinite integral .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
29
Find the indefinite integral of the following function and check the result by differentiation.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
30
Use the Log Rule to find the indefinite integral for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
31
Evaluate the integral
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
32
Find the indefinite integral of the following function and check the result by differentiation.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
33
Find the indefinite integral.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
34
Find the supply function that satisfies and the initial condition x = 700 when .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
35
Evaluate the integral
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
36
The marginal cost of a product is modeled by , when x = 8, C = 40. Find the cost function.
A)
B)
C)
D) .
E)
A)
B)
C)
D) .
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
37
Find the indefinite integral.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
38
Find the indefinite integral of the following function and check the result by differentiation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
39
Evaluate the integral
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
40
Find the indefinite integral.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
41
Sketch the region whose area is given by the definite integral and then use a geometric formula to evaluate the integral.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
42
Find the area of the region bounded by the graphs of the algebraic functions.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
43
Use any basic integration formula or formulas to find the indefinite integral .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
44
Find the area between the curve and the x-axis from .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
45
Set up the definite integral that gives the area of the region bounded by the graphs. 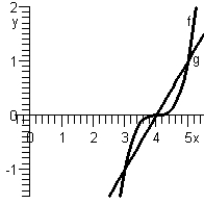
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
46
Find the average value of the function over the given interval. on [0,8]
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
47
Evaluate the following definite integral. Use a graphing utility to check your answer.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
48
Determine the area of the given region. 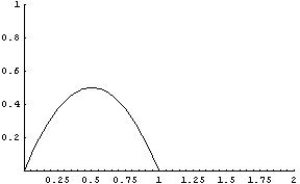
A)
B)
C)
D)
E)None of the above

A)
B)
C)
D)
E)None of the above

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
49
Find the area of the region bounded by the graphs of the algebraic functions.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
50
The rate of depreciation of a building is given by dollars per year, Use the definite integral to find the total depreciation over the first years.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
51
Determine the graph whose area (the shaded region) is represented by the integral.
A)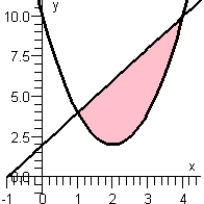
B)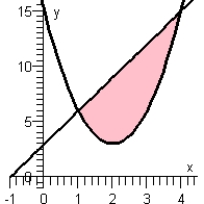
C)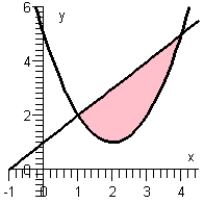
D)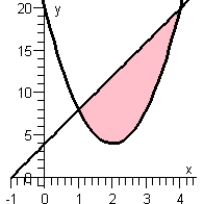
E)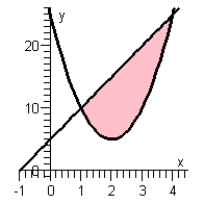
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
52
Find the average value of the function over the given interval. on [-3,3]
A)6
B)21
C)36
D)4
E)20
A)6
B)21
C)36
D)4
E)20

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
53
Sketch the region whose area is given by the definite integral and then use a geometric formula to evaluate the integral.
A)-145
B)290
C)200
D)100
E)10
A)-145
B)290
C)200
D)100
E)10

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
54
The integrand of the following definite integral is a difference of two functions. Sketch the graph of the two functions and shade the region whose area is represented by the integral.
A)![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff82_bdc5_7d5eb135388d_TB8692_11.jpg)
B)![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff83_bdc5_cf1b5317f353_TB8692_11.jpg)
C)![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff84_bdc5_ab9e27f20343_TB8692_11.jpg)
D)![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff85_bdc5_3b8d11bdc61f_TB8692_11.jpg)
E)![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff86_bdc5_9f2dea8cfbb0_TB8692_11.jpg)
A)
![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff82_bdc5_7d5eb135388d_TB8692_11.jpg)
B)
![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff83_bdc5_cf1b5317f353_TB8692_11.jpg)
C)
![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff84_bdc5_ab9e27f20343_TB8692_11.jpg)
D)
![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff85_bdc5_3b8d11bdc61f_TB8692_11.jpg)
E)
![<strong>The integrand of the following definite integral is a difference of two functions. \int _ { 0 } ^ { 4 } \left[ ( x + 1 ) - \frac { 1 } { 2 } x \right] d x Sketch the graph of the two functions and shade the region whose area is represented by the integral.</strong> A) B) C) D) E)](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11f9_ff86_bdc5_9f2dea8cfbb0_TB8692_11.jpg)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
55
Evaluate the definite integral of the algebraic function. Use a graphing utility to verify your results.
A)-12
B)-56
C)-36
D)8
E)-28
A)-12
B)-56
C)-36
D)8
E)-28

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
56
Find the area of the shaded region. 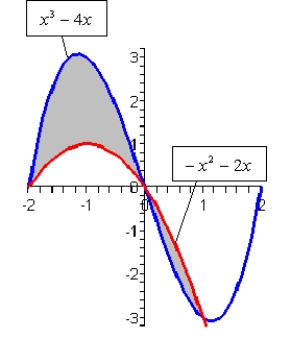
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
57
Use the values and to evaluate the definite integral .
A)14
B)2
C)5
D)11
E)4
A)14
B)2
C)5
D)11
E)4

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
58
Find the equation of the function whose derivative is and whose graph passes through the point .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
59
Evaluate the definite integral .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
60
Evaluate the definite integral of the algebraic function. Use a graphing utility to verify your results.
A)18.4007
B)29.6329
C)59.5941
D)38.9974
E)48.0336
A)18.4007
B)29.6329
C)59.5941
D)38.9974
E)48.0336

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
61
Estimate the surface area of the pond shown in the figure using the Midpoint Rule. 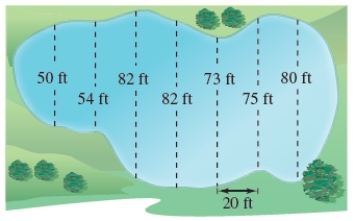
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
62
Use the Midpoint Rule with n = 4 to approximate the area of the following region. ![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the following region. f ( x ) = \frac { 1 } { x } , [ 1,5 ] </strong> A)1.156 B)1.324 C)1.575 D)1.275 E)1.876](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_3734_bdc5_538db0f63874_TB8692_00.jpg)
A)1.156
B)1.324
C)1.575
D)1.275
E)1.876
![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the following region. f ( x ) = \frac { 1 } { x } , [ 1,5 ] </strong> A)1.156 B)1.324 C)1.575 D)1.275 E)1.876](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_3734_bdc5_538db0f63874_TB8692_00.jpg)
A)1.156
B)1.324
C)1.575
D)1.275
E)1.876

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
63
The demand function for a product is , where p is the number of dollars and x is the number of units. If the equilibrium price is , what is the consumer's surplus?
A)$
B)$
C)$
D)$
E)$
A)$
B)$
C)$
D)$
E)$

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
64
Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the x-axis over the interval [ ].
A)11.4421
B)11.0023
C)11.2114
D)11.0000
E)12.0000
A)11.4421
B)11.0023
C)11.2114
D)11.0000
E)12.0000

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
65
The revenue from a manufacturing process (in millions of dollars per year) is projected to follow the model for 10 years. Over the same period of time, the cost (in millions of dollars per year) is projected to follow the model , where t is the time (in years). Approximate the profit over the 10-year period, beginning with t = 0. Round your answer to two decimal places.
A) million
B) million
C) million
D) million
E) million
A) million
B) million
C) million
D) million
E) million

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
66
Use the Midpoint Rule n = 4 to approximate the area of the following region. ![<strong>Use the Midpoint Rule n = 4 to approximate the area of the following region. f ( y ) = \frac { 1 } { 4 } y , [ 2,4 ] </strong> A)2.5 B)1.2 C)1.5 D)1.9 E)2.3](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_ac77_bdc5_eb7034519ba1_TB8692_11.jpg)
A)2.5
B)1.2
C)1.5
D)1.9
E)2.3
![<strong>Use the Midpoint Rule n = 4 to approximate the area of the following region. f ( y ) = \frac { 1 } { 4 } y , [ 2,4 ] </strong> A)2.5 B)1.2 C)1.5 D)1.9 E)2.3](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_ac77_bdc5_eb7034519ba1_TB8692_11.jpg)
A)2.5
B)1.2
C)1.5
D)1.9
E)2.3

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
67
Estimate the surface area of the oil spill shown in the figure using the Midpoint Rule. 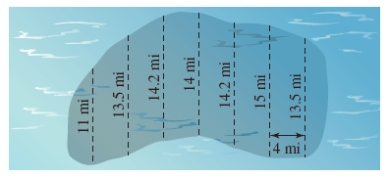
A) 481.6
B) 301.6
C) 311.6
D) 431.6
E) 381.6

A) 481.6
B) 301.6
C) 311.6
D) 431.6
E) 381.6

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
68
Use the Midpoint Rule with to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region.
A)The approximate area is:![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_5e4d_bdc5_89834096f9b0_TB8692_11.jpg)
B)The approximate area is:![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_855f_bdc5_69ca3b70a6bd_TB8692_11.jpg)
C)The approximate area is:![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_8561_bdc5_6779d21b2366_TB8692_11.jpg)
D)The approximate area is:![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_8563_bdc5_bd03e320c0f0_TB8692_11.jpg)
E)The approximate area is:![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_8565_bdc5_875fb75d5669_TB8692_11.jpg)
A)The approximate area is:
![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_5e4d_bdc5_89834096f9b0_TB8692_11.jpg)
B)The approximate area is:
![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_855f_bdc5_69ca3b70a6bd_TB8692_11.jpg)
C)The approximate area is:
![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_8561_bdc5_6779d21b2366_TB8692_11.jpg)
D)The approximate area is:
![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_8563_bdc5_bd03e320c0f0_TB8692_11.jpg)
E)The approximate area is:
![<strong>Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the -axis over the interval. Sketch the region. f ( x ) = \left( x ^ { 2 } - 4 \right) ^ { 2 } , [ - 2,2 ] </strong> A)The approximate area is: \approx 30.25 B)The approximate area is: \approx 24.25 C)The approximate area is: \approx 34.25 D)The approximate area is: \approx 14.25 E)The approximate area is: \approx 34.99](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_8565_bdc5_875fb75d5669_TB8692_11.jpg)

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
69
Use the Midpoint Rule with n = 4 to approximate the area of the region bounded by the graph of and the x-axis over the interval [0,1].
A)1.7524
B)1.3126
C)1.5217
D)2.3103
E)1.3103
A)1.7524
B)1.3126
C)1.5217
D)2.3103
E)1.3103

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
70
Two models, and , are given for revenue (in billions of dollars per year) for a large corporation. Both models are estimates of revenues for 2007 through 2011, with t = 7 corresponding to 2007. Which model is projecting the greater revenue? How much more total revenue does that model project over the five-year period?
A)The model projects greater revenue than . billion
B)The model projects greater revenue than . billion
C)The model projects greater revenue than . billion
D)The model projects greater revenue than . billion
E)The model projects greater revenue than . billion
A)The model projects greater revenue than . billion
B)The model projects greater revenue than . billion
C)The model projects greater revenue than . billion
D)The model projects greater revenue than . billion
E)The model projects greater revenue than . billion

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
71
Find the consumer and producer surpluses by using the demand and supply functions, where p is the price (in dollars) and x is the number of units (in millions). Demand Function Supply Function
A)a. $2587.50b. $3725.00
B)a. $5587.50b. $4725.00
C)a. $2587.50b. $1725.00
D)a. $1587.50b. $4725.00
E)a. $3587.50b. $4725.00
A)a. $2587.50b. $3725.00
B)a. $5587.50b. $4725.00
C)a. $2587.50b. $1725.00
D)a. $1587.50b. $4725.00
E)a. $3587.50b. $4725.00

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
72
Estimate the surface area of the golf green shown in the figure using the midpoint rule. 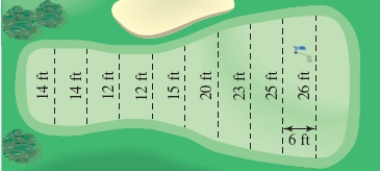
A)966
B)161
C)1449
D)1550
E)234

A)966
B)161
C)1449
D)1550
E)234

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
73
Use the Midpoint Rule with to approximate where . Then use a graphing utility to evaluate the definite integral. Compare your results.
A)a. Midpoint Rule: b. Graphing utility:
B)a. Midpoint Rule: b. Graphing utility:
C)a. Midpoint Rule: b. Graphing utility:
D)a. Midpoint Rule: b. Graphing utility:
E)a. Midpoint Rule: b. Graphing utility:
A)a. Midpoint Rule: b. Graphing utility:
B)a. Midpoint Rule: b. Graphing utility:
C)a. Midpoint Rule: b. Graphing utility:
D)a. Midpoint Rule: b. Graphing utility:
E)a. Midpoint Rule: b. Graphing utility:

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck
74
Use the rectangles to approximate the area of the region. Compare your result with the exact area obtained with a definite integral. ![<strong>Use the rectangles to approximate the area of the region. Compare your result with the exact area obtained with a definite integral. f ( x ) = - 2 x + 3 , [ 0,1 ] </strong> A)a. The approximate area: 3b. The exact area: 2 B)a. The approximate area: 2b. The exact area: 3 C)a. The approximate area: 2b. The exact area: 1 D)a. The approximate area: 2b. The exact area: 2 E)a. The approximate area: 1b. The exact area: 2](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_3736_bdc5_3d508f44058f_TB8692_00.jpg)
A)a. The approximate area: 3b. The exact area: 2
B)a. The approximate area: 2b. The exact area: 3
C)a. The approximate area: 2b. The exact area: 1
D)a. The approximate area: 2b. The exact area: 2
E)a. The approximate area: 1b. The exact area: 2
![<strong>Use the rectangles to approximate the area of the region. Compare your result with the exact area obtained with a definite integral. f ( x ) = - 2 x + 3 , [ 0,1 ] </strong> A)a. The approximate area: 3b. The exact area: 2 B)a. The approximate area: 2b. The exact area: 3 C)a. The approximate area: 2b. The exact area: 1 D)a. The approximate area: 2b. The exact area: 2 E)a. The approximate area: 1b. The exact area: 2](https://d2lvgg3v3hfg70.cloudfront.net/TB8692/11eb99ec_11fb_3736_bdc5_3d508f44058f_TB8692_00.jpg)
A)a. The approximate area: 3b. The exact area: 2
B)a. The approximate area: 2b. The exact area: 3
C)a. The approximate area: 2b. The exact area: 1
D)a. The approximate area: 2b. The exact area: 2
E)a. The approximate area: 1b. The exact area: 2

Unlock Deck
Unlock for access to all 74 flashcards in this deck.
Unlock Deck
k this deck



