Deck 12: Exponential Functions and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/68
Play
Full screen (f)
Deck 12: Exponential Functions and Logarithmic Functions
1
Solve the problem.
-An accountant tabulated a firm's profits for four recent years in the following table:
The accountant then fit both a linear graph and an exponential curve (seen below) to the data, in order to estimat profits. Use the exponential graph to estimate the profits in the year
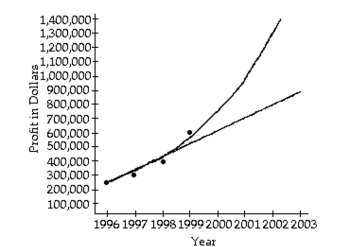
A) About
B) About
C) About
D) About
-An accountant tabulated a firm's profits for four recent years in the following table:
The accountant then fit both a linear graph and an exponential curve (seen below) to the data, in order to estimat profits. Use the exponential graph to estimate the profits in the year

A) About
B) About
C) About
D) About
About
2
Solve the problem.
Suppose that is invested at interest, compounded annually. Find a function A for the amount in the account after years.
A)
B)
C)
D)
Suppose that is invested at interest, compounded annually. Find a function A for the amount in the account after years.
A)
B)
C)
D)
B
3
Solve the problem.
-An accountant tabulated a firm's profits for four recent years in the following table:
The accountant then fit both a linear graph and an exponential curve (seen below) to the data, in order to estimat profits. Use the exponential graph to estimate the profits in the year
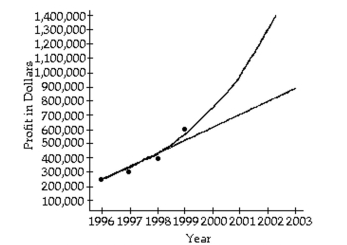
A) About
B) About
C) About
D) About
-An accountant tabulated a firm's profits for four recent years in the following table:
The accountant then fit both a linear graph and an exponential curve (seen below) to the data, in order to estimat profits. Use the exponential graph to estimate the profits in the year

A) About
B) About
C) About
D) About
About
4
Graph.
A)
B)
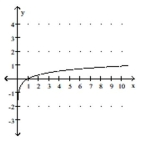
C)
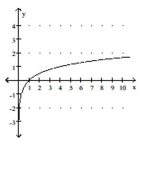
D)
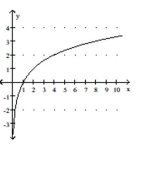

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
5
Solve the problem.
A computer is purchased for . Its value each year is about of the value the preceding year. Its value, in dollars, after years is given by the exponential function . Find the value of the computer after 6 years.
A)
B)
C)
D)
A computer is purchased for . Its value each year is about of the value the preceding year. Its value, in dollars, after years is given by the exponential function . Find the value of the computer after 6 years.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
6
Solve the problem.
The amount of particulate matter left in solution during a filtering process decreases by the equation , where is the number of filtering steps. Find the amounts left for and . (Round to the nearest whole number.)
A)
B)
C)
D)
The amount of particulate matter left in solution during a filtering process decreases by the equation , where is the number of filtering steps. Find the amounts left for and . (Round to the nearest whole number.)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
7
Solve the problem.
-An accountant tabulated a firm's profits for four recent years in the following table:
The accountant then fit both a linear graph and an exponential curve (seen below) to the data, in order to estimat profits. Use the linear graph to estimate the profits in the year
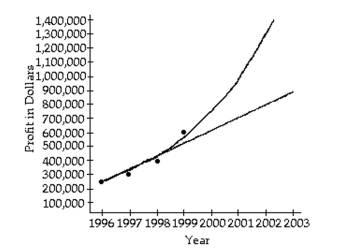
A) About
B) About
C) About
D) About
-An accountant tabulated a firm's profits for four recent years in the following table:
The accountant then fit both a linear graph and an exponential curve (seen below) to the data, in order to estimat profits. Use the linear graph to estimate the profits in the year

A) About
B) About
C) About
D) About

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
8
Graph.
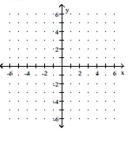
A)
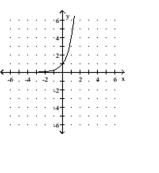
B)
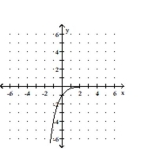
C)
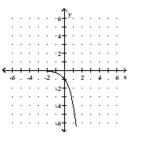
D)
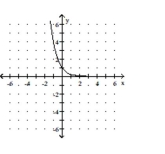

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
9
Graph.
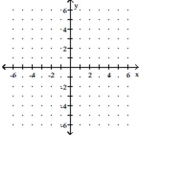
A)
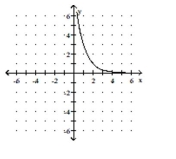
B)
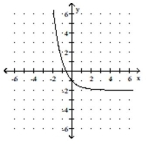
C)
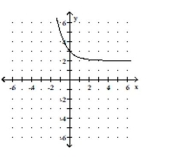
D)
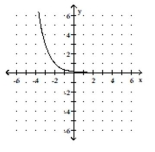

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
10
Solve the problem.
The half-life of a certain radioactive substance is 8 years. Suppose that at time , there are of the substance. Then after t years, the number of grams of the substance remaining will be:
How many grams of the substance will remain after 56 years? Round to the nearest hundredth when necessary.
A)
B)
C)
D)
The half-life of a certain radioactive substance is 8 years. Suppose that at time , there are of the substance. Then after t years, the number of grams of the substance remaining will be:
How many grams of the substance will remain after 56 years? Round to the nearest hundredth when necessary.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
11
Solve the problem.
The half-life of Cesium is hours. If the formula gives the percent (as a decimal) remaining after time (in hours), sketch P versus .
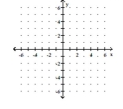
A)
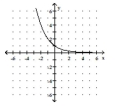
B)
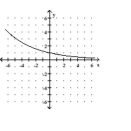
C)
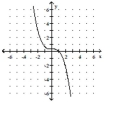
D)
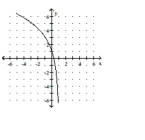
The half-life of Cesium is hours. If the formula gives the percent (as a decimal) remaining after time (in hours), sketch P versus .

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
12
Graph.
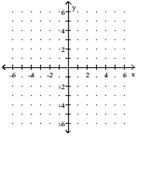
A)
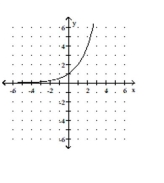
B)
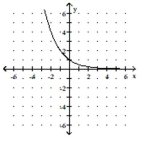
C)
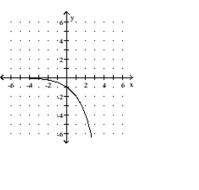
D)
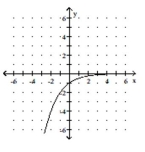

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
13
Solve the problem.
The number of bacteria growing in an incubation culture increases with time according to , where is time in days. Find the number of bacteria when and .
A) 19,
B)
C)
D)
The number of bacteria growing in an incubation culture increases with time according to , where is time in days. Find the number of bacteria when and .
A) 19,
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
14
Graph.
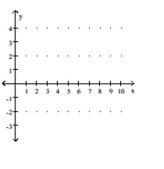
A)
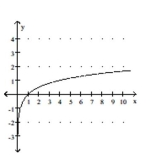
B)
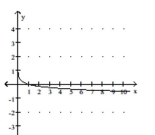
C)
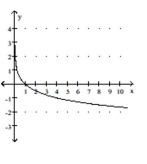
D)
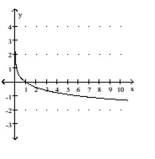

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
15
Solve the problem.
The number of dislocated electric impulses per cubic inch in a transformer increases when lightning strikes by , where is the time in milliseconds of the lightning strike. Find the number of dislocated impulses at and .
A)
B)
C)
D)
The number of dislocated electric impulses per cubic inch in a transformer increases when lightning strikes by , where is the time in milliseconds of the lightning strike. Find the number of dislocated impulses at and .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
16
Graph.
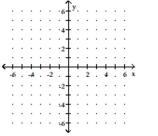
A)
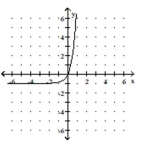
B)
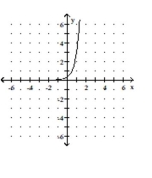
C)
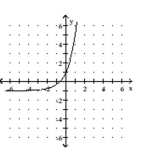
D)
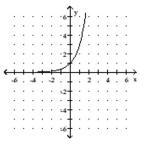

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
17
Graph.
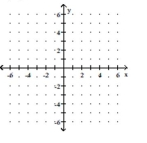
A)
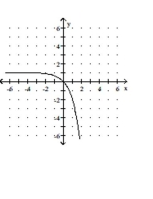
B)
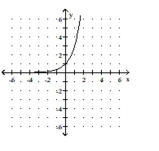
C)
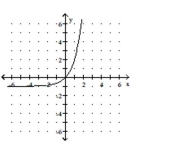
D)
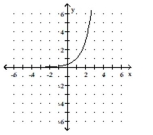

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
18
Solve the problem.
-An accountant tabulated a firm's profits for four recent years in the following table:
The accountant then fit both a linear graph and an exponential curve (seen below) to the data, in order to estimat profits. Use the linear graph to estimate the profits in the year
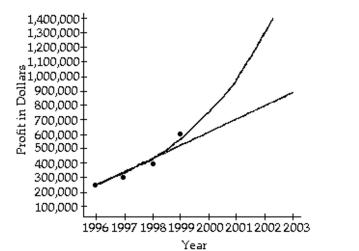
A) About
B) About
C) About
D) About
-An accountant tabulated a firm's profits for four recent years in the following table:
The accountant then fit both a linear graph and an exponential curve (seen below) to the data, in order to estimat profits. Use the linear graph to estimate the profits in the year

A) About
B) About
C) About
D) About

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
19
Graph.
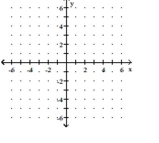
A)
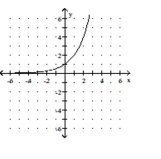
B)
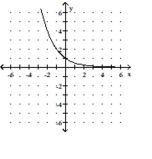
C)
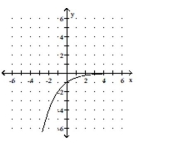
D)
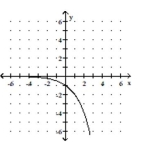

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
20
Graph.
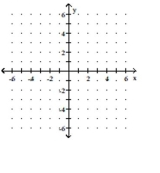
A)
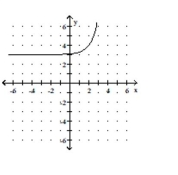
B)
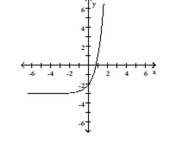
C)
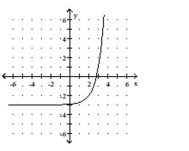
D)


A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
21
Find the requested composition of functions.
Given and , find .
A)
B)
C)
D)
Given and , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
22
Find the inverse of the relation.
A)
B)
C)
A)
B)
C)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
23
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
24
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
25
Find the inverse of the relation.
A)
B)
C)
A)
B)
C)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
26
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
27
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
28
Find the requested composition of functions.
Given and , find
A)
B)
C)
D)
Given and , find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
29
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
30
Find the requested composition of functions.
Given and , find .
A)
B)
C)
D)
Given and , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
31
Find the inverse of the relation.
A)
B)
C)
A)
B)
C)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
32
Find the requested composition of functions.
Given and , find .
A)
B)
C)
D)
Given and , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
33
Find the requested composition of functions.
Given and , find .
A)
B)
C)
D)
Given and , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
34
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
35
Find the requested composition of functions.
Given and , find .
A)
B)
C)
D)
Given and , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
36
Find the inverse of the relation.
A)
B)
C)
A)
B)
C)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
37
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
38
Find the requested composition of functions.
Given and , find .
A)
B)
C)
D)
Given and , find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
39
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
40
Find f(x)and g(x)such that h(x)= (f ° g)(x).
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
41
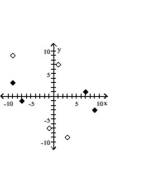
Graph the relation using solid circles and the inverse using open circles.
A)
B)
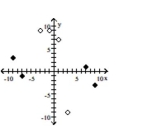
C)
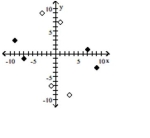
D)
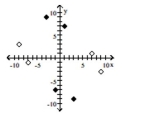

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
42
Graph the relation using solid circles and the inverse using open circles.
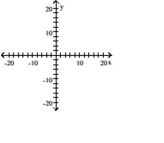
A)
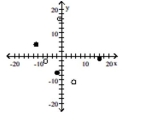
B)
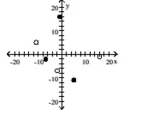
C)
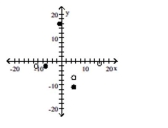
D)
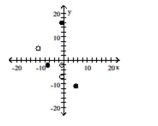

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
43
Graph the relation using solid circles and the inverse using open circles.
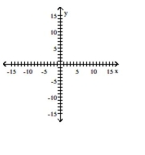
A)
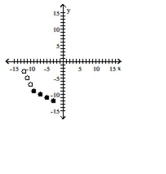
B)
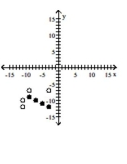
C)
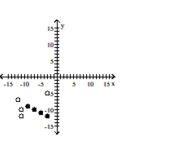
D)
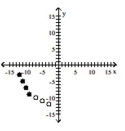

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
44
Graph the equation of the relation using a solid line, and then graph the inverse of the relation using a dashed line.
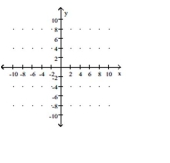
A)
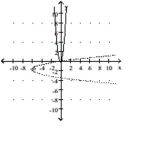
B)
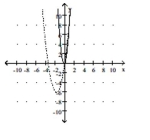
C)
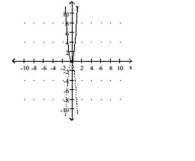
D)
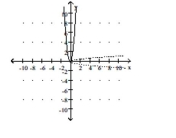

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
45
Find an equation of the inverse of the relation.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
46
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
47
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
48
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
49
Find an equation of the inverse of the relation.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
50
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
51
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
52
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
53
Graph the equation of the relation using a solid line, and then graph the inverse of the relation using a dashed line.
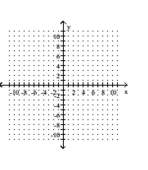
A)
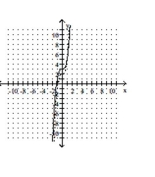
B)
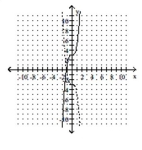
C)
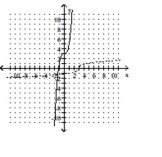
D)
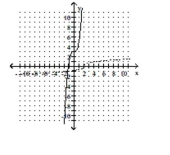

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
54
Find an equation of the inverse of the relation.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
55
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
56
Graph the equation of the relation using a solid line, and then graph the inverse of the relation using a dashed line.

A)
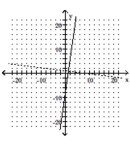
B)
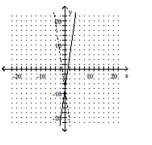
C)
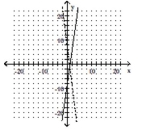
D)
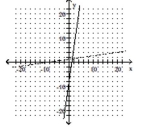

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
57
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
58
Graph the equation of the relation using a solid line, and then graph the inverse of the relation using a dashed line.
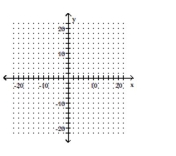
A)
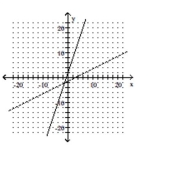
B)
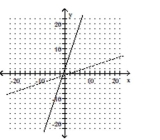
C)
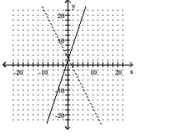
D)
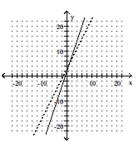

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
59
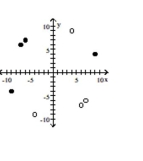
Graph the relation using solid circles and the inverse using open circles.
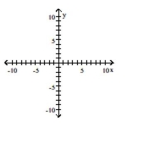
A)
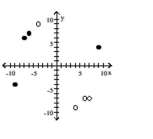
B)
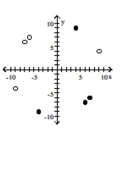
C)
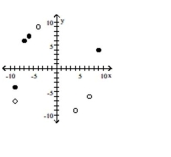
D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
60
Find an equation of the inverse of the relation.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
61
Determine whether the function is one-to-one.
-
-

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
62
Determine whether the given function is one-to-one. If so, find a formula for the inverse.
A)
B)
C) Not a one-to-one function
D)
A)
B)
C) Not a one-to-one function
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
63
Determine whether the given function is one-to-one. If so, find a formula for the inverse.
A)
B)
C) Not a one-to-one function
D)
A)
B)
C) Not a one-to-one function
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
64
Determine whether the given function is one-to-one. If so, find a formula for the inverse.
A)
B)
C)
D) Not a one-to-one function
A)
B)
C)
D) Not a one-to-one function

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
65
Determine whether the given function is one-to-one. If so, find a formula for the inverse.
A)
B)
C) Not a one-to-one function
D)
A)
B)
C) Not a one-to-one function
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
66
Determine whether the given function is one-to-one. If so, find a formula for the inverse.
A)
B)
C) Not a one-to-one function
D)
A)
B)
C) Not a one-to-one function
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
67
Determine whether the given function is one-to-one. If so, find a formula for the inverse.
A)
B) Not a one-to-one function
C)
D)
A)
B) Not a one-to-one function
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck
68
Determine whether the given function is one-to-one. If so, find a formula for the inverse.
A) Not a one-to-one function
B)
C)
D)
A) Not a one-to-one function
B)
C)
D)

Unlock Deck
Unlock for access to all 68 flashcards in this deck.
Unlock Deck
k this deck



