Deck 5: The Trigonometric Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/15
Play
Full screen (f)
Deck 5: The Trigonometric Functions
1
The point is on the terminal side of an angle in standard position. Determine the exact value of .
2
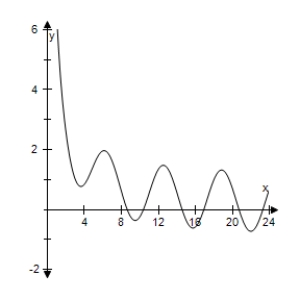
Use a graphing utility to select the graph of the function
3
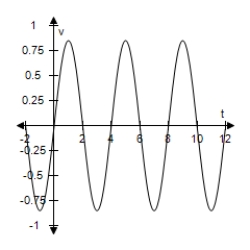
For a person at rest, the velocity v (in liters per second) of airflow during a respiratory cycle (the time from the beginning of one breath to the beginning of the next) is given by
,
where t is the time (in seconds).
(Inhalation occurs when v > 0 and exhalation occurs when v < 0.)
Select the graph of this velocity function.
,
where t is the time (in seconds).
(Inhalation occurs when v > 0 and exhalation occurs when v < 0.)
Select the graph of this velocity function.
4
Given the figure below, determine the value of . 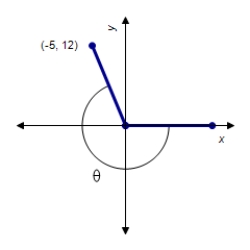


Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
5
Find (if possible) the complement and supplement of the angle.
17°
17°

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
6
Use a graphing utility to select the graph of the function below. Describe the behavior of the function as x approaches 0.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
7
Determine whether the statement is true or false.
The Leaning Tower of Pisa is not vertical, but if you know the angle of elevation θ to the top of the tower when you stand 25 feet away from it, you can find its height h using the formula
The Leaning Tower of Pisa is not vertical, but if you know the angle of elevation θ to the top of the tower when you stand 25 feet away from it, you can find its height h using the formula

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
8
State the period of the function.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
9
Use the unit circle below to estimate to the nearest tenth. 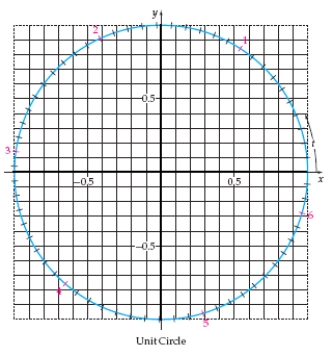


Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
10
Use a graphing utility to select the graph the damping factor and the function below in the same viewing window. Describe the behavior of the function as x increases without bound.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
11
The height of an outdoor basketball backboard is feet, and the backboard casts a shadow feet long.
Find the angle of elevation of the sun. Round your answer to one decimal place.
Find the angle of elevation of the sun. Round your answer to one decimal place.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
12
Find the period and amplitude.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
13
Find the relationship between the graphs of f and g. Consider amplitude, period, and shifts.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
14
Function g is related to a parent function .
Describe the sequence of transformation from f to g.
Describe the sequence of transformation from f to g.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
15
Use a graphing utility to select the graph of the function below and the damping factor of the function in the same viewing window.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck



