Deck 11: Limits of Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/15
Play
Full screen (f)
Deck 11: Limits of Functions
1
Find by direct substitution.
2
Find the limit by direct substitution.
3
Find the limit (if it exists). Use a graphing utility to verify your result graphically.
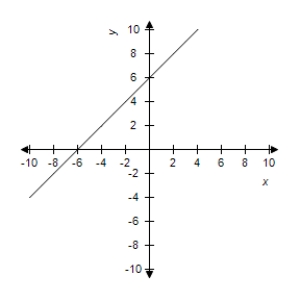

4
Find the limit (if it exists).

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
5
Find the limit.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
6
Select the correct function for the graph using oblique asymptotes as aids. 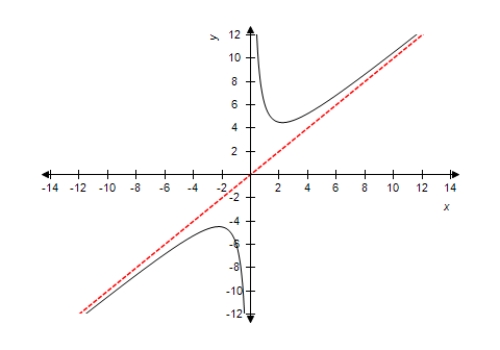


Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
7
Find the limit by direct substitution.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
8
Use the graph to find . 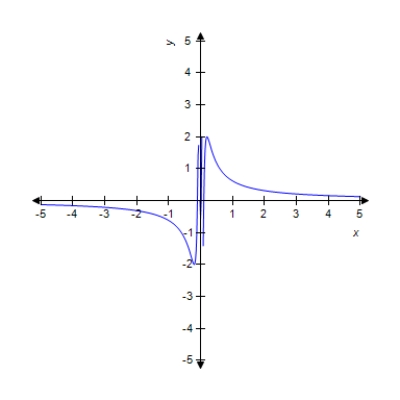


Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
9
Select the correct graph for the following function using a graphing utility. Determine whether the limit exists or not. ,

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
10
Find the limit (if it exists).

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
11
Complete the table and numerically estimate the limit as x approaches infinity for .

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
12
Use asymptotes to match with its graph.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
13
Graph the function. Determine the limit (if it exists) by evaluating the corresponding one-sided limits.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
14
Find for and .

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
15
Find the limit (if it exists). Use a graphing utility to verify your result graphically.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck



