Deck 5: Exponential and Logarithmic Functions

Question 1

A town's population grows at the rate of 10% per year.If this growth rate remains constant, how long will it take the population to double? a. $\quad 4.6$ years
b. $2.3$ years
c. 3 years
d. $6.9$ years
e. 1 years

Answer 1

D

Question 2

Use a calculator to find the value for to four decimal places. $$\begin{array} { l l } \text { a. } & 0.0338 \\\text { b. } & - 0.0338 \\\text { c. } & 0.9661 \\\text { d. } & - 0.0779 \\\text { e. } & - 0.9661\end{array}$$

Answer 2

B

Question 3

If P dollars are invested at the end of each year in an annuity that earns interest at an annual rate r, the amount in the account will be A dollars after n years, where $$n = \frac { \log \left[ \frac { A r } { p } + 1 \right] } { \log ( 1 + r ) }$$ If $S 5,000$ is invested each year in an annuity earning $9 \%$ annual interest, when will the account be worth $\$ 40,000$ ?
a. $\quad 2.9$ years
b. $\quad 6.3$ years
c. $\quad 14.5$ years
$\begin{array} { l l } \text { d. } & - 3.8 \text { years } \\ \text { e. } & 8.0 \text { years } \end{array}$
e. $\quad 8.0$ years

Answer 3

B

Question 4

The percent $P$ of the drug triazolam (a drug for treating insomnia) remaining in a person's bloodstream after t hours is given by $P = e ^ { - 0.3 t }$ . What percent will remain in the bloodstream after 9 hours?
a. $\quad 0.9 \%$
b. $\quad 15.4 \%$
c. $\quad 6.7 \%$
d. $\quad 5.4 \%$
e. $\quad 0.1 \%$

Question 5

Use a calculator to find the value to four decimal places. $6 ^ { \sqrt { 11 } }$
a. $\quad 380.9217$
b. $\quad 355.5336$
c. $1.8091$
d. $\quad 19.8997$
e. $26.9444$

Question 6

The present value of a sum of money is the amount that must be invested now, at a given rate of interest, to produce the desired sum at a later date.Find the present value of $100,000 if interest is paid
At a rate of 5% per year, compounded semiannually, for 8 years.

A)$46,319.35
B)$67,362.49
C)$67,683.94
D)$53,390.82
E)$5,408.79

Question 7

True or False? $$\log _ { c } 6 = \log _ { 6 } c$$

Question 8

Simplify the expression. $10 ^ { \sqrt { 10 } } \cdot 10 ^ { \sqrt { 10 } }$
a. $\quad 100 \sqrt { 20 }$
b. $\quad 100 \sqrt { 10 }$
c. $\quad 10 \sqrt { 20 }$
d. $\quad 20 \sqrt { 20 }$
e. $\quad 10 \sqrt { 10 }$

Question 9

$$\text { Tell whether the statement } \log _ { 2 } 2 ^ { 5 } = 5 \left( 2 ^ { \log 2 ^ { 5 } } \right) \text { is true or false. }$$

Question 10

Find the value of $b$ that would cause the graph of $y = b ^ { x }$ to look like the graph indicated.


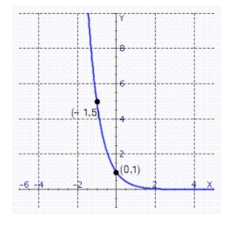
a. $\quad b = \frac { 3 } { 5 }$
b. $\quad b = 5$
c. $\quad b = - \frac { 1 } { 5 }$
d. $b = - 5$
e. $\quad b = \frac { 1 } { 5 }$

Question 11

In one individual, the percent alcohol level $t$ minutes after two shots of whiskey is given by $P = 0.3 ( 1 -$ $\left. e ^ { - 0.05 t } \right)$ . Find the blood alcohol level after 26 minutes.
a. $0.082 \%$
b. $\quad 0.118 \%$
c. $\quad 0.218 \%$
d. $\quad 0.382 \%$
e. $\quad 0.285 \%$

Question 12

Find the db gain of an amplifier whose input voltage is 0.7 volts and whose output voltage is 17 volts. a. $\quad 1.39 \mathrm { db }$
b. $\quad 63.8 \mathrm { db }$
c. $\quad 27.71 \mathrm { db }$
d. $\quad - 63.8 \mathrm { db }$
e. $3 \mathrm { db }$

Question 13

The concentration $x$ of a certain drug in an organ after $t$ minutes is given by $x = 0.06 \left( 1 - e ^ { - 0.1 t } \right)$ . Find the initial concentration of the drug when $t = 0$ .
$\begin{array} { l l } \text { a. } & 0.06 \\ \text { b. } & 1.00 \\ \text { c. } & 0.00 \\ \text { d. } & 0.12 \\ \text { e. } & 0.16 \end{array}$

Question 14

If P dollars are invested at the end of each year in an annuity that earns interest at an annual rate r, the amount in the account will be A dollars after n years, where $$n = \frac { \log \left[ \frac { A r } { P } + 1 \right] } { \log ( 1 + r ) } .$$ If $2,700 is invested each year in an annuity earning 15% annual interest, when will the account be
Worth $15,000?

A)10.0 years
B)5.6 years
C)2.4 years
D)-1.3 years
E)4.3 years

Question 15

Find the $\mathrm { pH }$ of a solution with a hydrogen ion concentration of $1.08 \cdot 10 ^ { - 5 }$ gram-ions per liter.
a. $\quad 4.9$
b. $\quad 0.5$
c. $\quad 4.96$
d. 5
e. $5.01$

Question 16

An account now contains $13,200 and has been accumulating interest at a 7% annual rate, compounded continuously, for 9 years.Find the initial deposit.

A)$13,185.47
B)$7,030.21
C)$7,023.91
D)$13,134.59
E)$13,135.73

Question 17

A bank credit card charges interest at the rate of 15% per year, compounded monthly.If a senior in college charges her last tuition bill of $1,100 and intends to pay it in one year, what will she have to
Pay?

A)$1,277.07
B)$1,325.31
C)$1,276.83
D)$1,113.75
E)$1,239.51

Question 18

Simplify the expression. $7 ^ { \log 76 }$
a. 7
b. 36
c. 6
d. 42
e. none of these

Question 19

A Wisconsin lake is stocked with 10,000 bluegill. The population is expected to grow exponentially according to the model $P = P _ { 0 } 2 ^ { t / 2 }$ . How many bluegill will be in the lake in 6 years?
$\begin{array} { l l } \text { a. } & 120,000 \text { bluegill } \\ \text { b. } & 40,000 \text { bluegill } \\ \text { c. } & 80,000 \text { bluegill } \\ \text { d. } & 60,000 \text { bluegill } \\ \text { e. } & 12,599 \text { bluegill } \end{array}$

Question 20

In a city with a population of 1,200,000, there are currently 1000 cases of infection with the HIV virus. If the spread of the disease is projected by the formula $$p = \frac { 1,200,000 } { 1 + ( 1200 - 1 ) e ^ { - 0.4 t } }$$ how many people will be infected in 8 years?

A)24,100 people
B)211,100 people
C)855,800 people
D)9,100 people
E)24,600 people

Question 21

Solve the equation. $2 \log _ { 2 } x = 1 + \log _ { 2 } ( x + 112 )$
a. $x = 14$
b. $x = - 14$
c. $x = 11$
d. $x = 16$
e. none of these

Question 22

A Wisconsin lake is stocked with 9,500 bluegill. The population is expected to grow exponentially according to the model $P = P _ { \mathrm { o } } 2 ^ { \nu / 2 }$ . How many bluegill will be in the lake in 6 years?
a. $\quad 114,000$ bluegill
b. $\quad 57,000$ bluegill
c. $\quad 11,969$ bluegill
d. $\quad 76,000$ bluegill
e. $\quad 38,000$ bluegill

Question 23

If P dollars are invested at the end of each year in an annuity that earns interest at an annual rate r, the amount in the account will be A dollars after n years, where $$n = \frac { \log \left[ \frac { A r } { p } + 1 \right] } { \log ( 1 + r ) }$$ If $2,600 is invested each year in an annuity earning 14% annual interest, when will the account be
Worth $30,000?

A)2.7 years
B)7.3 years
C)11.5 years
D)3.7 years
E)16.9 years

Question 24

Simplify the expression. $8 ^ { \sqrt { 5 } } \cdot 8 ^ { \sqrt { 5 } }$
a. $\quad 8 \sqrt { 10 }$
b. $\quad 16 ^ { \sqrt { 10 } }$
c. $\quad 5 ^ { \sqrt { 8 } }$
d. $\quad 64 \sqrt { 5 }$
e. $\quad 64 \sqrt { 10 }$

Question 25

Use a calculator to find the value to four decimal places.
$$6 ^ { \sqrt { 15 } }$$
a. $\quad 760.0173$
b. $\quad 1032.2071$
c. $1.5492$
d. $\quad 23.2379$
e. $36.7423$

Question 26

Solve the equation. $\log ( x - 50 ) - \log 9 = \log ( x - 30 ) - \log x$
a. $\quad x = 5$
b. $x = 58$
c. $x = 54$
d. $x = 56$
e. none of these

Question 27

Solve the equation. $\log _ { 3 } x = \log _ { 3 } \frac { 1 } { x } + 6$
a. $\quad x = 30$
b. $\quad x = 16$
c. $\quad x = - 27$
d. $x = 27$
e. $\quad x = - 16$
f. $\quad x = - 30$

Question 28

Find the value of $b$ that would cause the graph of $y = b ^ { x }$ to look like the graph indicated.


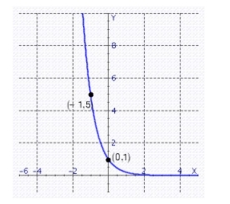
a. $b = - \frac { 1 } { 5 }$
b. $b = \frac { 3 } { 5 }$
c. $b = \frac { 1 } { 5 }$
d. $\quad b = 5$
e. $b = - 5$

Question 29

A town's population grows at the rate of 4% per year.If this growth rate remains constant, how long will it take the population to double? a. $\quad 7.5$ years
b. $0.6$ years
c. $1.4$ years
d. $17.3$ years
e. $2.8$ years

Question 30

Find the db gain of an amplifier whose input voltage is 0.8 volts and whose output voltage is 20 volts. a. $\quad 1.4 \mathrm { db }$
b. $\quad 27.96 \mathrm { db }$
c. $\quad 64.38 \mathrm { db }$
d. $\quad - 64.38 \mathrm { db }$
e. $3 \mathrm { db }$

Question 31

In one individual, the percent alcohol level $t$ minutes after two shots of whiskey is given by $P = 0.3 ( 1$ $e ^ { - 0.05 t }$ ). Find the blood alcohol level after 29 minutes.
a. $\quad 0.118 \%$
b. $\quad 0.230 \%$
c. $\quad 0.285 \%$
d. $\quad 0.070 \%$
e. $\quad 0.370 \%$

Question 32

The concentration $x$ of a certain drug in an organ after $t$ minutes is given by $x = 0.03 \left( 1 - e ^ { - 0.1 t } \right)$ . Find the initial concentration of the drug when $t = 0$ .
a. $\quad 0.00$
b. $\quad 0.06$
c. $\quad 0.08$
d. $\quad 1.00$
e. $\quad 0.03$

Question 33

A cloth fragment is found in an ancient tomb.It contains 63% of the carbon-14 (half-life is 5700 years) that it is assumed to have had initially.How old is the cloth? a. $\quad 10,600$ years
b. $\quad 8,200$ years
c. $\quad 9,000$ years
d. $\quad 8,600$ years
e. none of these

Question 34

The present value of a sum of money is the amount that must be invested now, at a given rate of interest, to produce the desired sum at a later date.Find the present value of $1,000 if interest is paid at
A rate of 7% per year, compounded semiannually, for 9 years.

A)$7.23
B)$452.80
C)$299.25
D)$538.36
E)$543.93

Question 35

In a city with a population of 1,200,000, there are currently 1000 cases of infection with the HIV virus. If the spread of the disease is projected by the formula $$p = \frac { 1,200,000 } { 1 + ( 1200 - 1 ) e ^ { - 0.4 t } }$$ how many people will be infected in 6 years?

A)5,300 people
B)11,000 people
C)302,100 people
D)10,900 people
E)60,800 people

Question 36

The percent $P$ of the drug triazolam (a drug for treating insomnia) remaining in a person's bloodstream after t hours is given by $P = e ^ { - 0.3 t }$ . What percent will remain in the bloodstream after 9 hours?
$\begin{array} { l l } \text { a. } & 0.9 \% \\ \text { b. } & 15.4 \% \\ \text { c. } & 0.1 \% \\ \text { d. } & 6.7 \% \\ \text { e. } & 5.4 \% \end{array}$

Question 37

A bacterial culture grows according to the formula $P = P _ { 0 } a ^ { t }$ . If it takes 4 days for the culture to triple in size, how long will it take to double in size?
a. $\quad - 0.9$ days
b. $\quad 5.0$ days
c. $\quad 2.1$ days
d. $\quad 0.4$ days
e. none of these

Question 38

An account now contains $9,100 and has been accumulating interest at a 14% annual rate, compounded continuously, for 12 years.Find the initial deposit.

A)$1,703.55
B)$8,924.39
C)$8,920.98
D)$1,696.00
E)$9,029.10

Question 39

Use a calculator to find the value for $\log 0.41326$ to four decimal places.
$\begin{array} { l l } \text { a. } & 0.3837 \\ \text { b. } & - 0.6162 \\ \text { c. } & - 0.8836 \\ \text { d. } & - 0.3837 \\ \text { e. } & 0.6162 \end{array}$

Question 40

A bank credit card charges interest at the rate of 15% per year, compounded monthly.If a senior in college charges her last tuition bill of $1,300 and intends to pay it in one year, what will she have to
Pay?

A)$1,316.25
B)$1,566.28
C)$1,509.26
D)$1,508.98
E)$1,464.87

Question 41

Solve the equation. $\log ( x - 46 ) - \log 6 = \log ( x - 32 ) - \log x$
a. $x = 52$
b. $\quad x = 4$
c. none of these
d. $x = 50$
e. $x = 48$

Question 42

True or False? $$\log _ { a } 7 = \log _ { 7 } a$$

Question 43

A bank credit card charges interest at the rate of 16% per year, compounded monthly.If a senior in college charges her last tuition bill of $1,700 and intends to pay it in one year, what will she have to
Pay?

A)$2,101.29
B)$1,722.67
C)$1,915.60
D)$1,993.38
E)$1,992.86

Question 44

Solve the equation. $\log _ { 5 } x = \log _ { 5 } \frac { 1 } { x } + 8$
a. $\quad x = 637$
b. $\quad x = - 625$
c. $\quad x = - 637$
d. $\quad x = 626$
e. $\quad x = - 626$
f. $x = 625$

Question 45

In one individual, the percent alcohol level $t$ minutes after two shots of whiskey is given by $P = 0.3 ( 1 -$ $e ^ { - 0.05 t }$ ). Find the blood alcohol level after 29 minutes.
a. $\quad 0.370 \%$
b. $\quad 0.285 \%$
c. $\quad 0.230 \%$
d. $\quad 0.070 \%$
e. $\quad 0.118 \%$

Question 46

$$\text { Tell whether the statement } \log _ { 7 } 7 ^ { 6 } = 6 \left( 7 ^ { \log 7 ^ { 6 } } \right) \text { is true or false. }$$

Question 47

Simplify the expression. $5 ^ { \log 57 }$
a. 7
b. none of these
c. 49
d. 35
e. 5

Question 48

If P dollars are invested at the end of each year in an annuity that earns interest at an annual rate r, the amount in the account will be A dollars after n years, where $$n = \frac { \log \left[ \frac { A r } { P } + 1 \right] } { \log ( 1 + r ) } .$$ If $\$ 7,000$ is invested each year in an annuity earning $6 \%$ annual interest, when will the account be worth $\$ 55,000 ?$
a. $\quad 15.3$ years
b. $\quad 6.6$ years
c. $\quad 3.2$ years
d. $\quad - 12.9$ years
e. $\quad 7.9$ years

Question 49

Solve the equation. $2 \log _ { 2 } x = 1 + \log _ { 2 } ( x + 40 )$
a. $x = 14$
b. $x = 10$
c. none of these
d. $x = 8$
e. $x = - 8$

Question 50

The concentration $x$ of a certain drug in an organ after $t$ minutes is given by $x = 0.08 \left( 1 - e ^ { - 0.1 t } \right)$ . Find the initial concentration of the drug when $t = 0$ .
$\begin{array} { l l } \text { a. } & 0.16 \\ \text { b. } & 0.00 \\ \text { c. } & 0.08 \\ \text { d. } & 1.00 \\ \text { e. } & 0.22 \end{array}$

Question 51

An account now contains $11,300 and has been accumulating interest at a 9% annual rate, compounded continuously, for 9 years.Find the initial deposit.

A)$5,026.90
B)$11,209.29
C)$11,207.59
D)$11,279.45
E)$5,021.11

Question 52

Find the value of $b$ that would cause the graph of $y = b ^ { x }$ to look like the graph indicated.


a. $b = \frac { 1 } { 5 }$
b. $\quad b = - \frac { 1 } { 5 }$
c. $b = - 5$
d. $b = 5$
e. $b = \frac { 3 } { 5 }$

Question 53

The present value of a sum of money is the amount that must be invested now, at a given rate of interest, to produce the desired sum at a later date.Find the present value of $1,000 if interest is paid at
A rate of 6% per year, compounded semiannually, for 7 years.

A)$617.78
B)$69.34
C)$661.12
D)$444.01
E)$665.06

Question 54

A cloth fragment is found in an ancient tomb.It contains 71% of the carbon-14 (half-life is 5700 years) that it is assumed to have had initially.How old is the cloth?

A)11,800 years
B)none of these
C)13,500 years
D)11,500 years
E)11,700 years

Question 55

Use a calculator to find the value to four decimal places. $4 ^ { \sqrt { 3 } }$
a. $6.0000$
b. $6.9282$
c. $2.3094$
d. $11.0357$
e. $\quad 9.0000$

Question 56

Simplify the expression. $$5 ^ { \sqrt { 10 } } \cdot 5 ^ { \sqrt { 10 } }$$
a. $\quad 25 \sqrt { 10 }$
b. $\quad 5 ^ { \sqrt { 20 } }$
c. $25 \sqrt { 20 }$
d. $10 ^ { \sqrt { 5 } }$
e. $\quad 10 \sqrt { 20 }$

Question 57

The percent $P$ of the drug triazolam (a drug for treating insomnia) remaining in a person's bloodstream after $t$ hours is given by $P = e ^ { - 0.3 t }$ . What percent will remain in the bloodstream after 6 hours?
$\begin{array} { l l } \text { a. } & 0.2 \% \\ \text { b. } & 16.5 \% \\ \text { c. } & 28.7 \% \\ \text { d. } & 0.8 \% \\ \text { e. } & 3.6 \% \end{array}$

Question 58

Find the $\mathrm { pH }$ of a solution with a hydrogen ion concentration of $1.93 \cdot 10 ^ { - 4 }$ gram-ions per liter.
a. $\quad 3.7$
b. $\quad 0.372$
c. $\quad 3.72$
d. $\quad 3.63$
e. $\quad 3.67$

Question 59

A bacterial culture grows according to the formula $P = P _ { \mathrm { o } } a ^ { t }$ . If it takes 4 days for the culture to triple in size, how long will it take to double in size?
a. $\quad - 0.9$ days
b. $\quad 2.1$ days
c. $\quad 0.4$ days
d. $\quad 5.0$ days
e. none of these

Question 60

A Wisconsin lake is stocked with 10,500 bluegill. The population is expected to grow exponentially according to the model $P = P _ { 0 } 2 ^ { t / 2 }$ . How many bluegill will be in the lake in 6 years?
a. $\quad 42,000$ bluegill
b. $\quad 13,229$ bluegill
c. $\quad 63,000$ bluegill
d. $\quad 84,000$ bluegill
e. $\quad 126,000$ bluegill

Question 61

$$\text { Find the db gain of an amplifier whose input voltage is } 1.3 \text { volts and whose output voltage is } 24 \text { volts. }$$ a. $\quad 1.27 \mathrm { db }$
b. $\quad 25.33 \mathrm { db }$
c. $3 \mathrm { db }$
d. $58.31 \mathrm { db }$
e. $\quad - 58.31 \mathrm { db }$

Question 62

$$\text { Find the } \mathrm { pH } \text { of a solution with a hydrogen ion concentration of } 1.13 \cdot 10 ^ { - 6 } \text { gram-ions per liter. }$$

A)5.87
B)0.586
C)5.91
D)5.94
E)5.86

Question 63

Solve the equation. $$\log ( x - 28 ) - \log 8 = \log ( x - 16 ) - \log x$$
a. $\quad x = 32$
b. $x = 34$
c. $x = 4$
d. $x = 36$
e. none of these

Question 64

Solve the equation. $2 \log _ { 2 } x = 1 + \log _ { 2 } ( x + 40 )$
a. none of these
b. $x = - 8$
c. $x = 15$
d. $x = 7$
e. $x = 10$

Question 65

Simplify the expression. $$2 ^ { \log _ { 2 } 4 }$$
a. $\quad 8$
b. 16
c. $\quad 4$
d. 2
e. none of these

Question 66

If P dollars are invested at the end of each year in an annuity that earns interest at an annual rate r, the amount in the account will be A dollars after n years, where $$n = \frac { \log \left[ \frac { A r } { P } + 1 \right] } { \log ( 1 + r ) }$$
If $\$ 6,000$ is invested each year in an annuity earning $10 \%$ annual interest, when will the account be worth $\$ 45,000$ ?
a. $\quad - 3.0$ years
b. $\quad 2.8$ years
c. $\quad 5.9$ years
d. $\quad 13.5$ years
e. $\quad 7.5$ years

Question 67

If P dollars are invested at the end of each year in an annuity that earns interest at an annual rate r, the amount in the account will be A dollars after n years, where $$n = \frac { \log \left[ \frac { A r } { P } + 1 \right] } { \log ( 1 + r ) }$$
If $\$ 2,600$ is invested each year in an annuity earning $12 \%$ annual interest, when will the account be worth $\$ 30,000$ ?
a. $\quad 11.5$ years
b. $\quad 2.8$ years
c. $\quad 2.9$ years
d. $\quad 7.7$ years
e. $\quad 17.7$ years

Question 68

True or False? $$\log _ { f } 4 = \log _ { 4 } f$$

Question 69

A town's population grows at the rate of $4 \%$ per year. If this growth rate remains constant, how long will it take the population to double?
a. $\quad 17.3$ years
b. $\quad 2.8$ years
c. $\quad 7.5$ years
d. $\quad 1.4$ years
e. $\quad 0.6$ years

Question 70

A bacterial culture grows according to the formula $P = P _ { \mathrm { o } } a ^ { t }$ . If it takes 8 days for the culture to triple in size, how long will it take to double in size?
a. $\quad 4.8$ days
b. $\quad - 1.1$ days
c. none of these
d. $\quad 1.9$ days
e. $\quad 0.2$ days

Question 71

$$\text { Tell whether the statement } \log _ { 4 } 4 ^ { 5 } = 5 \left( 4 ^ { \log 4 ^ { 5 } } \right) \text { is true or false. }$$

Question 72

In a city with a population of 1,200,000, there are currently 1000 cases of infection with the HIV virus. If the spread of the disease is projected by the formula $$P = \frac { 1,200,000 } { 1 + ( 1200 - 1 ) e ^ { - 0.4 t } }$$
how many people will be infected in 8 years?
a. $\quad 855,800$ people
b. $\quad 24,600$ people
c. $\quad 24,100$ people
d. $\quad 9,100$ people
e. 211,100 people

Question 73

A cloth fragment is found in an ancient tomb.It contains 78% of the carbon-14 (half-life is 5700 years) that it is assumed to have had initially.How old is the cloth?

A)15,900 years
B)16,400 years
C)18,900 years
D)15,800 years
E)none of these

Question 74

Use a calculator to find the value for to four decimal places. $$\begin{array} { l l } \text { a. } & - 0.8416 \\\text { b. } & 0.8416 \\\text { c. } & 0.1583 \\\text { d. } & - 0.1583 \\\text { e. } & - 1.9379\end{array}$$

Question 75

Solve the equation. $\log _ { 5 } x = \log _ { 5 } \frac { 1 } { x } + 2$
a. $x = 5$
b. $x = 13$
c. $x = - 13$
d. $x = - 2$
e. $x = - 5$
f. $x = 2$