Deck 8: Conic Sections and Quadratic Systems
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/75
Play
Full screen (f)
Deck 8: Conic Sections and Quadratic Systems
1
Find the equation of the hyperbola with center and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.
B
2
Parallel beams of similarly charged particles are shot from two atomic accelerators meters apart, as shown in the figure below. If the particles were not deflected, the beams would be meters apart. However, because the charged particles repel each other, the beams follow the hyperbolic path , for some . Find .
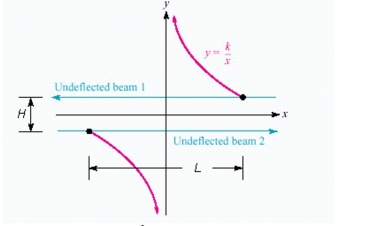
a.
b.
c.
d.
e. none of these

a.
b.
c.
d.
e. none of these
B
3
An arch is a semi-ellipse 16 meters wide and 8 meters high.Write the equation of the ellipse if the ellipse is centered at the origin. a.
b.
c.
d.
e. none of these
b.
c.
d.
e. none of these
A
4
Find the equation of the parabola with vertex at and which passes through and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
5
Find the equation of the curve on which the point lies. The distance between point and the point ( 0 ,
11) is of the distance between point and the line .
a.
b.
c.
d.
e. none of these
11) is of the distance between point and the line .
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
6
Find the equation of the circle with a radius of 9 and its center at the intersection of and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
7
Find the equation of the hyperbola with center and . Transverse axis is horizontal.
a.
b.
c.
d.
a.
b.
c.
d.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
8
Write the equation of the ellipse that has its center at the origin, focus at and is one-half the length of the minor axis.
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
9
Find the graph of the following equation.
a.
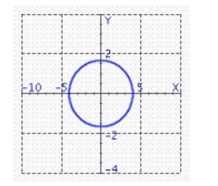
b.
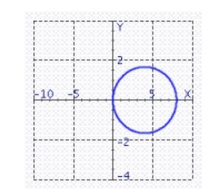
c.
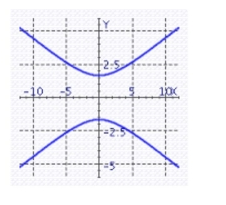
a.

b.

c.


Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
10
Find the equation of the parabola with vertex at and focus at .
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
11
Write the equation of the ellipse with center at and major axis parallel to the x-axis.
a.
b.
c.
d.
a.
b.
c.
d.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
12
Identify the conic as a circle, parabola, ellipse, or hyperbola.
a. parabola
b. hyperbola
c. ellipse
d. circle
e. none of the above
a. parabola
b. hyperbola
c. ellipse
d. circle
e. none of the above

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
13
Find the equation of the ellipse with center at , vertex at , and passing through the intersection of and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
14
Find the equation of the parabola passing through the given points. , and
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
15
Find the equation of the circle graphed below. 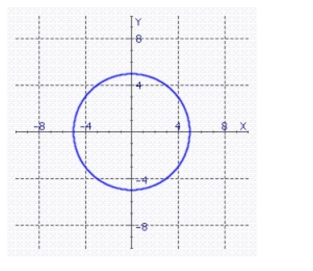
a.
b.
c.
d.
e.

a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
16
Find the equation of the circle with a radius of 2 and its center at the intersection of and .
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
17
Stones dropped into a calm pond at points and create ripples that propagate in widening circles. In the figure below, points and are 26 feet apart, and the radii of the circles differ by 10 feet. The point where the circles intersect moves along a curve. Find the equation of the curve.
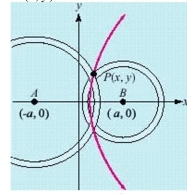
a.
b.
c.
d.

a.
b.
c.
d.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
18
Find the area of the fundamental rectangle of the hyperbola.
a. square units
b. square units
c. square units
d. 108 square units
e. none of these
a. square units
b. square units
c. square units
d. 108 square units
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
19
Graph the following equation.
a.
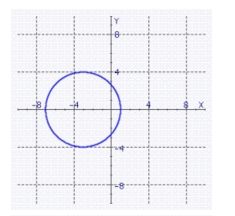
b.
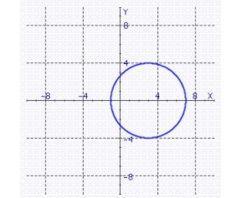
c.
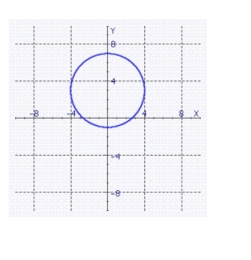
a.

b.

c.


Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
20
Tell whether the parabolic graph of the equation opens up, down, to the left, or to the right.
a. opens down
b. opens up
c. opens to the right
d. opens to the left
a. opens down
b. opens up
c. opens to the right
d. opens to the left

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
21
Graph the following equation.
a.
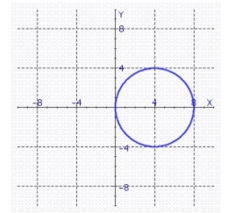
b.
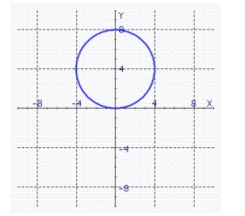
c.
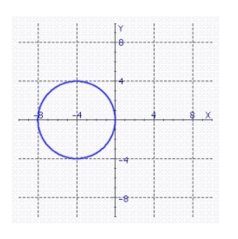
a.

b.

c.


Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
22
Tell whether the parabolic graph of the equation opens up, down, to the left, or to the right.
a. opens down
b. opens up
c. opens to the right
d. opens to the left
a. opens down
b. opens up
c. opens to the right
d. opens to the left

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
23
Find the equation of the hyperbola with center and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
24
Find the equation of the hyperbola with center and . Transverse axis is horizontal.
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
25
Write the equation of the ellipse that has its center at the origin, focus at and is one-half the length of the minor axis.
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
26
Find the equation of the ellipse with center at , vertex at , and passing through the intersection of and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
27
Find the equation of the circle with a radius of 4 and its center at the intersection of and .
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
28
Find the equation of the parabola with vertex at (0, 0) and focus at (0, 5). a.
b.
c.
d.
e. none of these
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
29
Solve the system of equations algebraically for real values of x and y.
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
30
An arch is a semi-ellipse 16 meters wide and 8 meters high. Write the equation of the ellipse if the ellipse is centered at the origin.
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
31
Find the equation of the circle graphed below. 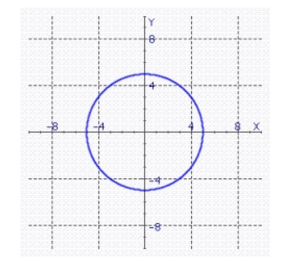
a.
b.
c.
d.
e.

a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
32
Write the equation of the ellipse with center at and major axis parallel to the x-axis.
a.
b.
c.
d.
a.
b.
c.
d.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
33
Find the graph of the following equation.
a.
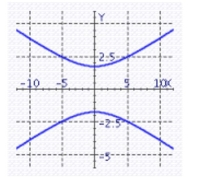
b.
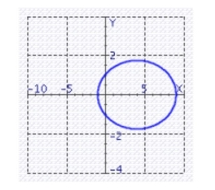
c.
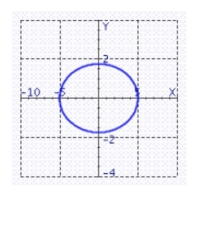
a.

b.

c.


Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
34
Find the equation of the parabola with vertex at (8, 3) and which passes through (7, 5) and (7, 1). a.
b.
c.
d.
e.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
35
Jim drove 252 miles. Jim's brother made the same trip at a speed miles per hour slower than Jim did and required an extra hours. Find Jim's rate and time.
a.
b.
c.
d.
e. not possible
a.
b.
c.
d.
e. not possible

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
36
Solve the system of equations algebraically for real values of x and y.
a.
b.
c.
d.
e. no solution
a.
b.
c.
d.
e. no solution

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
37
Solve the system of equations algebraically for real values of x and y.
a.
b.
c.
d.
e. no solution
a.
b.
c.
d.
e. no solution

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
38
Find the equation of the circle with a radius of 9 and its center at the intersection of and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
39
The area of a rectangle is 30 square centimeters, and its perimeter is 22 centimeters.Find the dimensions of the rectangle.
A)22 cm by 22 cm
B)5 cm by 5 cm
C)5 cm by 6 cm
D)5 cm by 3 cm
E)3 cm by 6 cm
A)22 cm by 22 cm
B)5 cm by 5 cm
C)5 cm by 6 cm
D)5 cm by 3 cm
E)3 cm by 6 cm

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
40
Find the equation of the parabola passing through the given points. , and
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
41
Parallel beams of similarly charged particles are shot from two atomic accelerators meters apart, as shown in the figure below. If the particles were not deflected, the beams would be meters apart. However, because the charged particles repel each other, the beams follow the hyperbolic path , for some . Find .
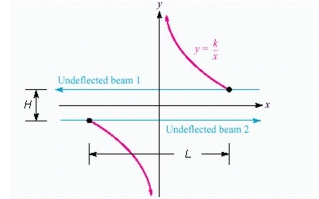
a.
b.
c.
d.
e. none of these

a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
42
Jim drove 196 miles. Jim's brother made the same trip at a speed miles per hour slower than Jim did and required an extra hours. Find Jim's rate and time.
a.
b.
c.
d.
e. not possible
a.
b.
c.
d.
e. not possible

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
43
Identify the conic as a circle, parabola, ellipse, or hyperbola.
a. circle
b. hyperbola
c. parabola
d. ellipse
e. none of the above
a. circle
b. hyperbola
c. parabola
d. ellipse
e. none of the above

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
44
Solve the system of equations algebraically for real values of x and y.
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
45
Graph the following equation.
a.
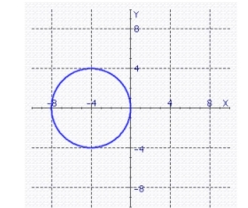
b.
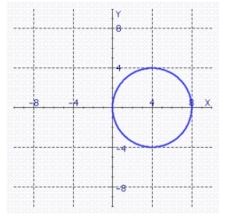
c.
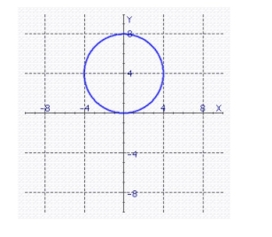
a.

b.

c.


Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
46
Find the area of the fundamental rectangle of the hyperbola.
a. 288 square units
b. 144 square units
c. square units
d. 36 square units
e. none of these
a. 288 square units
b. 144 square units
c. square units
d. 36 square units
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
47
Find the equation of the parabola with vertex at and which passes through and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
48
Write the equation of the ellipse with center at and major axis parallel to the -axis.
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
49
Find the equation of the circle with a radius of 5 and its center at the intersection of and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
50
Find the equation of the curve on which the point lies. The distance between point and the point ( 0 ,
13) is of the distance between point and the line .
a.
b.
c.
d.
e. none of these
13) is of the distance between point and the line .
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
51
Find the equation of the circle graphed below. 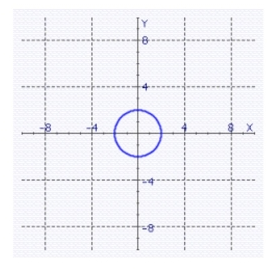
a.
b.
c.
d.
e.

a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
52
Stones dropped into a calm pond at points and create ripples that propagate in widening circles. In the figure below, points and are 12 feet apart, and the radii of the circles differ by 4 feet. The point where the circles intersect moves along a curve. Find the equation of the curve.
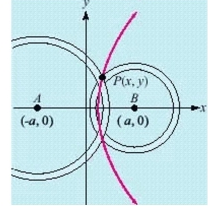
a.
b.
c.
d.
e. none of these

a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
53
Solve the system of equations algebraically for real values of x and y.
a.
b.
c.
d.
e. no solution
a.
b.
c.
d.
e. no solution

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
54
Solve the system of equations algebraically for real values of x and y.
a.
b.
c.
d.
e. no solution
a.
b.
c.
d.
e. no solution

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
55
Tell whether the parabolic graph of the equation opens up, down, to the left, or to the right.
a. opens to the right
b. opens down
c. opens to the left
d. opens up
a. opens to the right
b. opens down
c. opens to the left
d. opens up

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
56
The area of a rectangle is 24 square centimeters, and its perimeter is 20 centimeters.Find the dimensions of the rectangle. a. by
b. by
c. by
d. by
e. by
b. by
c. by
d. by
e. by

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
57
Find the equation of the parabola passing through the given points. , and
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
58
Find the equation of the circle with a radius of 9 and its center at the intersection of and .
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
59
Write the equation of the ellipse that has its center at the origin, focus at and is one-half the length of the minor axis.
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
60
Find the equation of the parabola with vertex at (0, 0) and focus at (0, 3). a.
b.
c.
d.
e. none of these
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
61
Stones dropped into a calm pond at points A and B create ripples that propagate in widening circles.In the figure below, points A and B are 12 feet apart, and the radii of the circles differ by 4 feet.The point
P (x, y) where the circles intersect moves along a curve.Find the equation of the curve.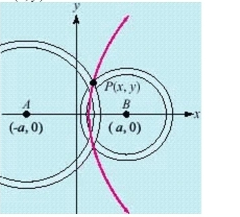
a.
b.
c.
d.
e. none of these
P (x, y) where the circles intersect moves along a curve.Find the equation of the curve.

a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
62
Solve the system of equations algebraically for real values of x and y.
A)(8, 3), (3, 8)
B)(7, 3), (3, 7)
C)(7, 3), (7, 8)
D)(3, 7), (3, 7)
E)no solution
A)(8, 3), (3, 8)
B)(7, 3), (3, 7)
C)(7, 3), (7, 8)
D)(3, 7), (3, 7)
E)no solution

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
63
Solve the system of equations algebraically for real values of x and y.
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
64
Identify the conic as a circle, parabola, ellipse, or hyperbola.
A)circle
B)hyperbola
C)ellipse
D)parabola
E)none of the above
A)circle
B)hyperbola
C)ellipse
D)parabola
E)none of the above

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
65
Find the equation of the hyperbola with center and . Transverse axis is horizontal.
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
66
An arch is a semi-ellipse 6 meters wide and 3 meters high.Write the equation of the ellipse if the ellipse is centered at the origin. a.
b.
c.
d.
e. none of these
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
67
Find the equation of the ellipse with center at , vertex at , and passing through the intersection of and .
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
68
Find the area of the fundamental rectangle of the hyperbola.
a. 192 square units
b. square units
c. square units
d. square units
e. none of these
a. 192 square units
b. square units
c. square units
d. square units
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
69
Parallel beams of similarly charged particles are shot from two atomic accelerators meters apart, as shown in the figure below. If the particles were not deflected, the beams would be meters apart. However, because the charged particles repel each other, the beams follow the hyperbolic path , for some . Find .
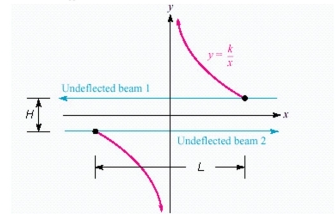
a.
b.
c.
d.
e. none of these

a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
70
The area of a rectangle is 36 square centimeters, and its perimeter is 26 centimeters.Find the dimensions of the rectangle.
A)4 cm by 7 cm
B)7 cm by 9 cm
C)26 cm by 26 cm
D)4 cm by 4 cm
E)4 cm by 9 cm
A)4 cm by 7 cm
B)7 cm by 9 cm
C)26 cm by 26 cm
D)4 cm by 4 cm
E)4 cm by 9 cm

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
71
Solve the system of equations algebraically for real values of x and y.
a.
b.
c.
d.
e. no solution
a.
b.
c.
d.
e. no solution

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
72
Find the graph of the following equation.
a.
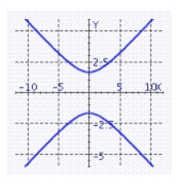
b.
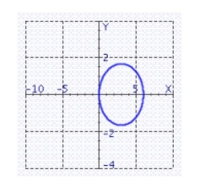
c.
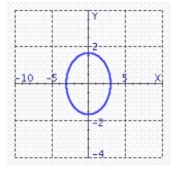
a.

b.

c.


Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
73
Jim drove 252 miles. Jim's brother made the same trip at a speed 14 miles per hour slower than Jim did and required an extra hours. Find Jim's rate and time.
a.
b.
c.
d.
e. not possible
a.
b.
c.
d.
e. not possible

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
74
Find the equation of the hyperbola with center and
a.
b.
c.
d.
e.
a.
b.
c.
d.
e.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
75
Find the equation of the curve on which the point lies. The distance between point and the point , 7) is of the distance between point and the line .
a.
b.
c.
d.
e. none of these
a.
b.
c.
d.
e. none of these

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck



