Deck 9: Natural Number Functions and Probability

Question 1

Select the correct inequality. a. $\quad n > 5 ^ { n }$
b. $n < 5 ^ { n }$
c. $n \geq 5 ^ { n }$
d. $\quad n \leq 5 ^ { n }$

Answer 1

B

Question 2

Use the binomial theorem to expand the binomial. $$( x + d ) ^ { 3 }$$
a. $\quad x ^ { 3 } + 2 x ^ { 2 } d + 2 x d ^ { 2 } + d ^ { 3 }$
b. $\quad x ^ { 3 } + 3 x ^ { 2 } d + 3 x d ^ { 2 } + d ^ { 3 }$
c. $\quad x ^ { 3 } + d ^ { 3 }$
d. $\quad x ^ { 3 } + x ^ { 2 } d + x d ^ { 2 } + d ^ { 3 }$
e. none of the above

Answer 2

B

Question 3

Evaluate the expression. $5 ! \cdot 3 !$
a. $\quad 15$
b. 2,880
c. $\quad 720$
d. $\quad 4,320$
e. none of the above

Answer 3

C

Question 4

Write the first six terms of the sequence defined by the function. $$f ( n ) = 6 n ( n - 1 )$$
$\begin{array} { l l } \text { a. } & 0,12,72,180,336,432 \\ \text { b. } & 12,36,72,120,180,252 \\ \text { c. } & 0,0,12,36,72,120 \\ \text { d. } & 0,12,36,72,120,180 \\ \text { e. } & - 12,0,12,36,72,120 \end{array}$

Question 5

Evaluate the sum. $$\sum _ { k = 2 } ^ { 9 } 4 k$$
a. 2
b. 176
c. 352
d. 9
e. none of these

Question 6

Select the right expression for the following sum and prove that your statement is true for all natural numbers $n$ .
$$P _ { \mathrm { n } } : 8 + 6 + 4 \ldots ( 10 - 2 n )$$
a. $\quad 11 n - 2 n ^ { 2 }$
b. $\quad 9 n - n ^ { 2 }$
c. $\quad 11 n - n ^ { 2 }$
d. $\quad 9 n - 2 n ^ { 2 }$
e. none of the above

Question 7

Evaluate the expression. $$P ( 4,3 ) \cdot C ( 8,7 )$$
a. 1
b. 967,680
c. 161,280
d. 32
e. 192

Question 8

Verify whether $1 + 2 + 3 + \ldots + n = \frac { n } { 2 } ( n + 1 )$ is true for $n = 7$
a. yes
b. no

Question 9

Find the 10th term in the binomial expansion. $$\left( \frac { x } { 2 } - \frac { d } { 2 } \right) ^ { 11 }$$
a. $\quad - \frac { 1 } { 24 } x d ^ { 10 }$
b. $\quad - \frac { 55 } { 2,048 } x ^ { 2 } d ^ { 9 }$
c. $\quad \frac { 55 } { 128 } x ^ { 2 } d ^ { 9 }$
d. $\quad - \frac { 11 } { 2,048 } x ^ { 2 } d ^ { 9 }$
e. none of the above

Question 10

Evaluate the expression. $$\left( \begin{array} { l } 100 \\99\end{array} \right)$$
a. 90
b. 100
c. 1
d. $\quad 99$
e. $- 9$

Question 11

Write the first six terms of the arithmetic sequence with the given properties. 7th term is 22; common difference is 3 a. $\quad 4,10,13,16,19,26$
b. $\quad - 8 , - 3,2,7,12,17$
c. $\quad 4,7,10,13,16,19$
d. $8,11,14,17,20,23$
e. $22,37,34,31,28,25$

Question 12

If you changed the following decimal into a fraction and reduced it to lowest terms, what would be its denominator? $0 . \overline { 5 }$
a. 90
b. 9
c. 999
d. 9999
e. $\quad 99$

Question 13

Select the right expression for the following sum and prove your statement is true for all natural numbers $n$ , where $r$ is a real number, $r \neq 1$ .
$S _ { n } : 1 + r + r ^ { 2 } + \ldots + r ^ { n }$
a. $\quad \frac { 1 - r ^ { n + 1 } } { 1 - r }$
b. $\quad r ^ { n + 1 } - 1$
c. $\quad \frac { r ^ { n + 1 } } { r - 1 }$
d. $\quad \frac { 1 - r ^ { n } } { 1 - r }$
e. $\quad \frac { 1 - r ^ { n } } { 1 - r ^ { n - 1 } }$

Question 14

The first row in a movie theater contains 20 seats.As you move toward the back, each row has 1 additional seat.If there are 18 rows, what is the capacity of the theater? a. 513
b. 570
c. $\quad 333$
d. $\quad 522$
e. 1,083

Question 15

Select the right expression for the following sum and prove that your statement is true for all natural numbers $n$ .
$P _ { \mathrm { n } } : 3 + 6 + 9 \ldots 3 n$
a. $\quad \frac { n ( 3 n + 1 ) } { 2 }$
b. $\quad \frac { 3 n ( n + 1 ) } { 4 }$
c. $\quad \frac { 3 n ( n + 1 ) } { 2 }$
d. $\frac { n ( 3 n + 1 ) } { 4 }$
e. none of the above

Question 16

One bacterium divides into two bacteria every 6 minutes.If two bacteria multiply enough to completely fill a petri dish in 2 hours, how long will it take one bacterium to fill the dish?

A)2 hours
B)2 hours 12 minutes
C)2 hours 7 minutes
D)2 hours 18 minutes
E)2 hours 6 minutes

Question 17

The number of students studying algebra at State College is 511.The Department Chair expects enrollment to increase 11% each year.How many professors will be needed in 8 years to teach algebra
If one professor can handle 50 students?

A)22 professors
B)46 professors
C)26 professors
D)21 professors
E)23 professors

Question 18

To pay for college, a student borrows $5,000 interest-free from his father.If he pays his father back at the rate of $200 per month, how much will he still owe after 11 months? a. $\quad \$ 1,800$
b. $\$ 3,130$
c. $\quad \$ 2,800$
d. $\quad \$ 2,200$
e. none of the above

Question 19

Evaluate the expression. $7 !$
a. $\quad 40320$
b. 7
c. 42
d. 720
e. 5,040

Question 20

Find the r th term in the binomial expansion. $$( z + d ) ^ { n }$$
a. $\frac { n ! } { ( n - r ) ! r ! } z ^ { n - r } d ^ { r }$
b. $\frac { n ! } { ( n + r - 1 ) ! ( r + 1 ) ! } z ^ { n + r - 1 } d ^ { r + 1 }$
c. $\frac { n ! } { ( n - r + 1 ) ! ( r - 1 ) ! } z ^ { n - r } d ^ { r - 1 }$
d. $\quad \frac { n ! } { ( n - r ) ! r ! } z ^ { n - r + 1 } d ^ { r - 1 }$
e. $\frac { n ! } { ( n - r + 1 ) ! ( r - 1 ) ! } z ^ { n - r + 1 } d ^ { r - 1 }$

Question 21

One bacterium divides into two bacteria every 5 minutes.If two bacteria multiply enough to completely fill a petri dish in 1 hour, how long will it take one bacterium to fill the dish?

A)1 hour 10 minutes
B)1 hour 5 minutes
C)1 hour 15 minutes
D)1 hour 6 minutes
E)1 hour

Question 22

Assume that the probability that an airplane engine will fail during a torture test is and that the aircraft in question has 6 engines.Find the probability that no engines will survive. a. $\frac { 1 } { 3 }$
b. $\frac { 1 } { 730 }$
c. $\frac { 1 } { 729 }$
d. $\frac { 1 } { 6 }$
e. $\frac { 1 } { 1,458 }$

Question 23

How many lines are determined by 9 points if no 3 points lie on a straight line? a. 72
b. 32
c. 25
d. 29
e. 36

Question 24

The first row in a movie theater contains 30 seats.As you move toward the back, each row has 3 additional seats.If there are 22 rows, what is the capacity of the theater? a. $\quad 1,023$
b. $\quad 2,829$
c. $\quad 1,386$
d. $\quad 1,476$
e. $\quad 1,353$

Question 25

The number of students studying algebra at State College is 543.The Department Chair expects enrollment to increase 13% each year.How many professors will be needed in 5 years to teach algebra
If one professor can handle 70 students?

A)12 professors
B)17 professors
C)14 professors
D)28 professors
E)13 professors

Question 26

Evaluate the sum. $$\sum _ { k = 3 } ^ { 8 } 4 k$$
a. 264
b. 132
c. 8
d. 3
e. none of these

Question 27

Evaluate the expression. $5 !$
a. 20
b. 5
c. $\quad 720$
d. 24
e. $\quad 120$

Question 28

Use the binomial theorem to expand the binomial. $$( z + t ) ^ { 3 }$$
a. $\quad z ^ { 3 } + 3 z ^ { 2 } t + 3 z t ^ { 2 } + t ^ { 3 }$
b. $\quad z ^ { 3 } + z ^ { 2 } t + z t ^ { 2 } + t ^ { 3 }$
c. $\quad z ^ { 3 } + t ^ { 3 }$
d. $\quad z ^ { 3 } + 2 z ^ { 2 } t + 2 z t ^ { 2 } + t ^ { 3 }$
e. none of the above

Question 29

Evaluate the expression. $4 ! \cdot 6 !$
a. $\quad 17,280$
b. $\quad 24$
c. 120,960
d. $\quad 86,400$
e. none of the above

Question 30

Find the r th term in the binomial expansion. $$( z + y ) ^ { n }$$
a. $\frac { n ! } { ( n - r ) ! r ! } z ^ { n - r + 1 } y ^ { r - 1 }$
b. $\frac { n ! } { ( n - r ) ! r ! } z ^ { n - r } y ^ { r }$
c. $\frac { n ! } { ( n + r - 1 ) ! ( r + 1 ) ! } z ^ { n + r - 1 } y ^ { r + 1 }$
d.
e.
$\frac { n ! } { ( n - r + 1 ) ! ( r - 1 ) ! } z ^ { n - r } y ^ { r - 1 }$
$\frac { n ! } { ( n - r + 1 ) ! ( r - 1 ) ! } z ^ { n - r + 1 } y ^ { r - 1 }$

Question 31

To pay for college, a student borrows $4,600 interest-free from his father.If he pays his father back at the rate of $350 per month, how much will he still owe after 10 months? $$\begin{array} { l l } \text { a. } & \$ 750 \\\text { b. } & \$ 1,100 \\\text { c. } & \$ 3,500 \\\text { d. } & \$ 1,900 \\\text { e. } & \text { none of the above }\end{array}$$

Question 32

If you changed the following decimal into a fraction and reduced it to lowest terms, what would be its denominator? $0 . \overline { 2 }$
a. 9999
b. 99
c. $\quad 90$
d. 999
e. 9

Question 33

Find the 10th term in the binomial expansion. $$\left( \frac { z } { 3 } - \frac { d } { 3 } \right) ^ { 11 }$$
a. $\quad - \frac { 11 } { 177,147 } z ^ { 2 } d ^ { 9 }$
b. $\quad - \frac { 55 } { 177,147 } z ^ { 2 } d ^ { 9 }$
c. $\quad - \frac { 1 } { 24 } z d ^ { 10 }$
d. $\quad \frac { 55 } { 128 } z ^ { 2 } d ^ { 9 }$
e. none of the above

Question 34

If there are 7 class periods in a school day, and a typical student takes 4 classes, how many different time patterns are possible for the student?

A)70
B)105
C)33
D)32
E)35

Question 35

5 words are to be paired with the correct 5 out of 10 possible definitions.How many ways are there of guessing? a. $\quad 176$
b. 227
c. 202
d. $\quad 504$
e. 252

Question 36

Write the first six terms of the sequence defined by the function. $$f ( n ) = 5 n ( n - 1 )$$
a. $\quad 0,10,60,150,280,360$
b. $\quad 10,30,60,100,150,210$
c. $\quad - 10,0,10,30,60,100$
d. $\quad 0,0,10,30,60,100$
e. $\quad 0,10,30,60,100,150$

Question 37

Assume that a survey of 258 people is taken to determine the opinions of doctors, teachers, and lawyers on a proposed piece of legislation, with the results as shown below.A person is chosen at random from
Those surveyed.Refer to the table to find the probability the person favors the legislation.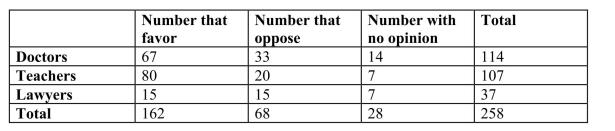

a. $\quad 0.5$
b. $\quad 0.258$
c. $\quad 0.67$
d. $\quad 0.63$
e. $\quad 0.107$

Question 38

Select the right expression for the following sum and prove that your statement is true for all natural numbers n. $$P _ { \mathrm { n } } : 8 + 6 + 4 \ldots ( 10 - 2 n )$$
a. $\quad 9 n - n ^ { 2 }$
b. $\quad 9 n - 2 n ^ { 2 }$
c. $\quad 11 n - 2 n ^ { 2 }$
d. $\quad 11 n - n ^ { 2 }$
e. none of the above

Question 39

Select the right expression for the following sum and prove that your statement is true for all natural numbers $n$ .
$$P _ { \mathrm { n } } : 3 + 6 + 9 \ldots 3 n$$
a. $\quad \frac { 3 n ( n + 1 ) } { 4 }$
b. $\frac { n ( 3 n + 1 ) } { 2 }$
c. $\quad \frac { 3 n ( n + 1 ) } { 2 }$
d. $\frac { n ( 3 n + 1 ) } { 4 }$
e. none of the above

Question 40

Write the first six terms of the arithmetic sequence with the given properties. 7th term is 23; common difference is 3 a. $\quad 5,8,11,14,17,20$
b. $23,38,35,32,29,26$
c. $\quad - 1,3,7,11,15,19$
d. $5,11,14,17,20,28$
e. $\quad 6,9,12,15,18,21$

Question 41

Select the right expression for the following sum and prove your statement is true for all natural numbers $n$ , where $r$ is a real number, $r \neq 1$ . $S _ { n } : 1 + r + r ^ { 2 } + \ldots + r ^ { n }$
a. $\quad \frac { 1 - r ^ { n + 1 } } { 1 - r }$
b. $\quad \mathrm { r } ^ { n + 1 } - 1$
c. $\quad \frac { r ^ { n + 1 } } { r - 1 }$
d. $\quad \frac { 1 - r ^ { n } } { 1 - r }$
e. $\quad \frac { 1 - r ^ { n } } { 1 - r ^ { n - 1 } }$

Question 42

Write the first six terms of the sequence defined by the function. $$f ( n ) = 7 n ( n - 1 )$$
a. $0,0,14,42,84,140$
b. $\quad 0,14,84,210,392,504$
c. $\quad 0,14,42,84,140,210$
d. $\quad - 14,0,14,42,84,140$
e. $14,42,84,140,210,294$

Question 43

13 words are to be paired with the correct 13 out of 15 possible definitions.How many ways are there of guessing?

A)105
B)84
C)210
D)74
E)95

Question 44

The first row in a movie theater contains 22 seats.As you move toward the back, each row has 2 additional seats.If there are 34 rows, what is the capacity of the theater?

A)1,904
B)1,980
C)1,496
D)1,870
E)3,850

Question 45

Assume that a survey of 263 people is taken to determine the opinions of doctors, teachers, and lawyers on a proposed piece of legislation, with the results as shown below.A person is chosen at random from
Those surveyed.Refer to the table to find the probability the person favors the legislation. $$\begin{array}{l}\begin{array} { | l | l | l | l | l | } \hline & \begin{array} { l } \text { Number that } \\\text { favor }\end{array} & \begin{array} { l } \text { Number that } \\\text { oppose }\end{array} & \begin{array} { l } \text { Number with } \\\text { no opinion }\end{array} & \text { Total } \\\hline \text { Doctors } & 74 & 33 & 16 & 123 \\\hline \text { Teachers } & 80 & 17 & 5 & 102 \\\hline \text { Lawyers } & 22 & 13 & 3 & 38 \\\hline \text { Total } & 176 & 63 & 24 & 263 \\\hline\end{array}\\\begin{array} { l l } \text { a. } & 0.74 \\\text { b. } & 0.263 \\\text { c. } & 0.102 \\\text { d. } & 0.5 \\\text { e. } & 0.67\end{array}\end{array}$$

Question 46

Evaluate the expression. $$\left( \begin{array} { l } 81 \\80\end{array} \right)$$
a. 81
b. $- 7$
c. 73
d. 80
e. 1

Question 47

Evaluate the expression. $2 ! \cdot 3 !$
a. 36
b. 6
c. 48
d. 12
e. none of the above

Question 48

Evaluate the expression. $6 !$
a. 5040
b. 720
c. 30
d. 120
e. 6

Question 49

How many lines are determined by 9 points if no 3 points lie on a straight line?

A)29
B)25
C)32
D)36
E)72

Question 50

Assume that the probability that an airplane engine will fail during a torture test is and that the aircraft in question has 6 engines.Find the probability that no engines will survive. a. $\quad \frac { 1 } { 4,096 }$
b. $\frac { 1 } { 6 }$
c. $\frac { 1 } { 4 }$
d. $\frac { 1 } { 4,097 }$
e. $\frac { 1 } { 8,192 }$

Question 51

Select the correct inequality. a. $\quad n \geq 7 ^ { n }$
b. $n \leq 7 ^ { n }$
c. $n < 7 ^ { n }$
d. $\quad n > 7 ^ { n }$

Question 52

Find the 10th term in the binomial expansion. $$\left( \frac { a } { 3 } - \frac { y } { 3 } \right) ^ { 11 }$$
a. $\quad - \frac { 11 } { 177,147 } a ^ { 2 } y ^ { 9 }$
b. $\quad \frac { 55 } { 128 } a ^ { 2 } y ^ { 9 }$
c. $\quad - \frac { 1 } { 24 } a y ^ { 10 }$
d. $\quad - \frac { 55 } { 177,147 } a ^ { 2 } y ^ { 9 }$
e. none of the above

Question 53

To pay for college, a student borrows $4,200 interest-free from his father.If he pays his father back at the rate of $190 per month, how much will he still owe after 15 months?

A)$1,050
B)$780
C)$2,850
D)$1,350
E)none of the above

Question 54

Evaluate the sum. $$\sum _ { k = 2 } ^ { 5 } 2 k$$
a. 2
b. 56
c. 28
d. 5
e. none of these

Question 55

Find the r th term in the binomial expansion. $( c + y ) ^ { n }$
a. $\quad \frac { n ! } { ( n - r ) ! r ! } c ^ { n - r + 1 } y ^ { r - 1 }$

b. $\frac { n ! } { ( n - r ) ! r ! } c ^ { n - r } y ^ { r }$

c. $\frac { n ! } { ( n - r + 1 ) ! ( r - 1 ) ! } c ^ { n - r } y ^ { r - 1 }$

d. $\frac { n ! } { ( n - r + 1 ) ! ( r - 1 ) ! } c ^ { n - r + 1 } y ^ { r - 1 }$
e.
$\frac { n ! } { ( n + r - 1 ) ! ( r + 1 ) ! } c ^ { n + r - 1 } y ^ { r + 1 }$

Question 56

Verify whether $1 + 2 + 3 + \ldots + n = \frac { n } { 2 } ( n + 1 )$ is true for $n = 3$ .
a. yes
b. no

Question 57

If there are 5 class periods in a school day, and a typical student takes 3 classes, how many different time patterns are possible for the student?

A)30
B)10
C)7
D)20
E)8

Question 58

Write the first six terms of the arithmetic sequence with the given properties. 7th term is 10; common difference is 1 a. $\quad 4,6,7,8,9,14$
b. $\quad 4,5,6,7,8,9$
c. $\quad 10,15,14,13,12,11$
d. $\quad - 2,0,2,4,6,8$
e. $\quad 2,3,4,5,6,7$

Question 59

Use the binomial theorem to expand the binomial. $$( a + b ) ^ { 3 }$$
a. $\quad a ^ { 3 } + 3 a ^ { 2 } b + 3 a b ^ { 2 } + b ^ { 3 }$
b. $\quad a ^ { 3 } + b ^ { 3 }$
c. $\quad a ^ { 3 } + 2 a ^ { 2 } b + 2 a b ^ { 2 } + b ^ { 3 }$
d. $\quad a ^ { 3 } + a ^ { 2 } b + a b ^ { 2 } + b ^ { 3 }$
e. none of the above

Question 60

Evaluate the expression. $$\begin{array}{l}P ( 9,6 ) \cdot C ( 9,7 )\\\begin{array} { l l } \text { a. } & 36,288 \\\text { b. } & 2,177,280 \\\text { c. } & 10,973,491,200 \\\text { d. } & 15,240,960 \\\text { e. } & 3\end{array}\end{array}$$

Question 61

Assume that a survey of 253 people is taken to determine the opinions of doctors, teachers, and lawyers on a proposed piece of legislation, with the results as shown below.A person is chosen at random from
Those surveyed.Refer to the table to find the probability the person favors the legislation. $$\begin{array}{l}\begin{array} { | l | l | l | l | l | } \hline & \begin{array} { l } \text { Number that } \\\text { favor }\end{array} & \begin{array} { l } \text { Number that } \\\text { oppose }\end{array} & \begin{array} { l } \text { Number with } \\\text { no opinion }\end{array} & \text { Total } \\\hline \text { Doctors } & 70 & 27 & 15 & 112 \\\hline \text { Teachers } & 75 & 15 & 11 & 101 \\\hline \text { Lawyers } & 16 & 16 & 8 & 40 \\\hline \text { Total } & 161 & 58 & 34 & 253 \\\hline\end{array}\\\begin{array} { l l } \text { a. } & 0.64 \\\text { b. } & 0.5 \\\text { c. } & 0.101 \\\text { d. } & 0.253 \\\text { e. } & 0.70\end{array}\end{array}$$

Question 62

5 words are to be paired with the correct 5 out of 15 possible definitions.How many ways are there of guessing?

A)3,003
B)6,006
C)2,703
D)2,102
E)2,402

Question 63

Find the 3rd term in the binomial expansion. $$\left( c + \frac { t } { 2 } \right) ^ { 8 }$$
a. $\quad 7 c ^ { 2 } t ^ { 6 }$
b. $\quad 7 c ^ { 6 } t ^ { 2 }$
c. $4 c ^ { 6 } t ^ { 2 }$
d. $\quad 28 c ^ { 6 } t ^ { 2 }$
e. $\quad c ^ { 6 } t ^ { 2 }$

Question 64

One bacterium divides into two bacteria every 6 minutes.If two bacteria multiply enough to completely fill a petri dish in 3 hours, how long will it take one bacterium to fill the dish?

A)3 hours 18 minutes
B)3 hours 12 minutes
C)3 hours 7 minutes
D)3 hours 6 minutes
E)3 hours

Question 65

Select the right expression for the following sum and prove that your statement is true for all natural numbers n. $P _ { n } : 3 + 6 + 9 \ldots 3 n$
a. $\quad \frac { n ( 3 n + 1 ) } { 2 }$
b. $\frac { 3 n ( n + 1 ) } { 4 }$
c. $\quad \frac { n ( 3 n + 1 ) } { 4 }$
d. $\frac { 3 n ( n + 1 ) } { 2 }$
e. none of the above

Question 66

Use the binomial theorem to expand the binomial. $$\left( \frac { a } { 2 } + t \right) ^ { 4 }$$
a. $\quad a ^ { 4 } + a ^ { 3 } t + a ^ { 2 } t ^ { 2 } + a t ^ { 3 } + t ^ { 4 }$
b. $\quad \frac { 1 } { 8 } a ^ { 4 } + \frac { 1 } { 2 } a ^ { 3 } t + \frac { 3 } { 2 } a ^ { 2 } t ^ { 2 } + 2 a t ^ { 3 } + t ^ { 4 }$
c. $\quad \frac { 1 } { 2 } a ^ { 4 } + t ^ { 4 }$
d. $\quad \frac { 1 } { 16 } a ^ { 4 } + \frac { 1 } { 2 } a ^ { 3 } t + \frac { 3 } { 2 } a ^ { 2 } t ^ { 2 } + 2 a t ^ { 3 } + t ^ { 4 }$
e. $\quad \frac { 1 } { 16 } a ^ { 4 } + \frac { 1 } { 8 } a ^ { 3 } t + \frac { 1 } { 4 } a ^ { 2 } t ^ { 2 } + \frac { 1 } { 2 } a t ^ { 3 } + t ^ { 4 }$

Question 67

Find the 5th term in the binomial expansion. $$( a + \sqrt { 6 } d ) ^ { 9 }$$
a. $\quad 504 a ^ { 5 } d ^ { 4 }$
b. $\quad 6,340 a ^ { 6 } d ^ { 3 }$
c. $\quad 4,536 a ^ { 5 } d ^ { 4 }$
d. $4,536 a ^ { 4 } d ^ { 5 }$
e. $\quad 6,340 a ^ { 5 } d ^ { 4 }$

Question 68

Evaluate the expression. $$\left( \begin{array} { l } 93 \\92\end{array} \right)$$
a. $\quad 83$
b. $- 9$
c. $\quad 93$
d. $\quad 92$
e. 1

Question 69

Verify whether $1 + 2 + 3 + \ldots + n = \frac { n } { 2 } ( n + 1 ) + 1$ is true for $n = 1$ .
a. yes
b. no

Question 70

Assume that the probability that an airplane engine will fail during a torture test is $\frac { 1 } { 3 }$ and that the aircraft in question has 5 engines. Find the probability that no engines will survive.
a. $\frac { 1 } { 243 }$
b. $\frac { 1 } { 3 }$
c. $\frac { 1 } { 5 }$
d. $\frac { 1 } { 486 }$
e. $\frac { 1 } { 244 }$

Question 71

The number of students studying algebra at State College is 593.The Department Chair expects enrollment to increase 11% each year.How many professors will be needed in 7 years to teach algebra
If one professor can handle 65 students?

A)17 professors
B)36 professors
C)16 professors
D)18 professors
E)21 professors

Question 72

Select the correct inequality. a. $n > 4 ^ { n }$
b. $n < 4 ^ { n }$
c. $n \leq 4 ^ { n }$
d. $\quad n \geq 4 ^ { n }$

Question 73

Select the right expression for the following sum and prove your statement is true for all natural numbers $n$ , where $p$ is a real number, $p \neq 1$ .
$S _ { n } : 1 + p + p ^ { 2 } + \ldots + p ^ { n }$
a. $\quad \frac { 1 - p ^ { n } } { 1 - p ^ { n - 1 } }$
b. $\quad \frac { 1 - p ^ { n + 1 } } { 1 - p }$
c. $\frac { p ^ { n + 1 } } { p - 1 }$
d. $\quad \mathrm { p } ^ { n + 1 } - 1$
e. $\frac { 1 - p ^ { n } } { 1 - p }$

Question 74

If you changed the following decimal into a fraction and reduced it to lowest terms, what would be its denominator? $0 . \overline { 4 }$
a. 99
b. 9
c. 9999
d. 90
e. $\quad 999$

Question 75

Find the 4th term in the binomial expansion. $$( z + y ) ^ { n }$$
a. $\frac { ( n - 3 ) ! } { ( n - 3 ) ! 3 ! } z ^ { n - 3 } y ^ { 3 }$
b. $\frac { n ! } { ( n - 4 ) ! } z ^ { n - 4 } y ^ { 4 }$
c. $\quad \frac { n ! } { ( n - 3 ) ! 3 ! } z ^ { n - 3 } y ^ { 3 }$
d. $\frac { n ! } { ( n - 4 ) ! 4 ! } z ^ { n - 4 } y ^ { 4 }$
e. $\frac { n ! } { ( n - 3 ) ! 3 ! } z ^ { n - 3 } y ^ { n }$

Question 76

Select the right expression for the following sum and prove that your statement is true for all natural numbers n. $$p _ { n } : 8 + 6 + 4 \ldots ( 10 - 2 n )$$
a. $9 n - 2 n ^ { 2 }$
b. $11 n - n ^ { 2 }$
c. $\quad 9 n - n ^ { 2 }$
d. $\quad 11 n - 2 n ^ { 2 }$
e. none of the above

Question 77

If there are 5 class periods in a school day, and a typical student takes 3 classes, how many different time patterns are possible for the student?

A)8
B)20
C)30
D)7
E)10

Question 78

Evaluate the expression. $$P ( 9,4 ) \cdot C ( 9,9 )$$
$\begin{array} { l l } \text { a. } & 15,120 \\ \text { b. } & 45,722,880 \\ \text { c. } & 5 \\ \text { d. } & 1,097,349,120 \\ \text { e. } & 3,024 \end{array}$

Question 79

How many lines are determined by 10 points if no 3 points lie on a straight line?

A)41
B)45
C)32
D)90
E)36

Question 80

Find the 5th term in the binomial expansion. $$( c - t ) ^ { n }$$
a. $\frac { n ! } { ( n - 4 ) ! 4 ! } c ^ { n - 4 } t ^ { 4 }$
b. $\quad \frac { ( n - 4 ) ! } { ( n - 4 ) ! 4 ! } c ^ { n - 4 } t ^ { 4 }$
c. $\quad \frac { n ! } { ( n - 5 ) ! 5 ! } c ^ { n - 5 } t ^ { n }$
d. $\frac { n ! } { ( n - 4 ) ! } c ^ { n - 4 } t ^ { 4 }$
e. $\frac { n ! } { ( n - 5 ) ! 5 ! } c ^ { n - 5 } t ^ { 5 }$