Deck 4: Polynomial and Rational Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/147
Play
Full screen (f)
Deck 4: Polynomial and Rational Functions
1
For the following function, find a number 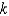 such that the graph of
such that the graph of 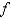 contains the point
contains the point 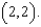
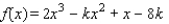
A)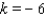
B)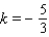
C)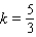
D)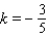
E)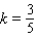
 such that the graph of
such that the graph of 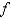 contains the point
contains the point 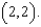
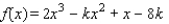
A)
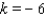
B)
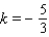
C)
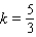
D)
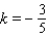
E)
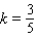
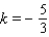
2
Find all solutions of the equation. 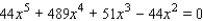
A)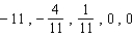
B)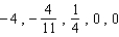
C)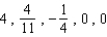
D)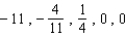
E)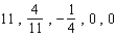
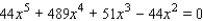
A)
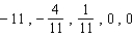
B)
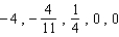
C)
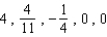
D)
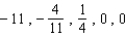
E)
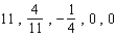
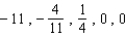
3
Sketch the graph of 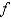 .
. 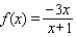
A)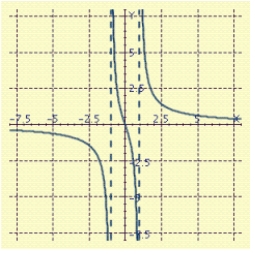
B)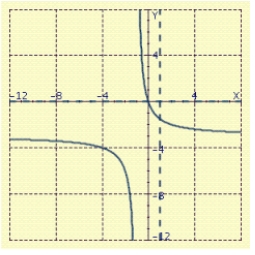
C)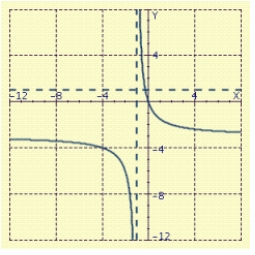
D)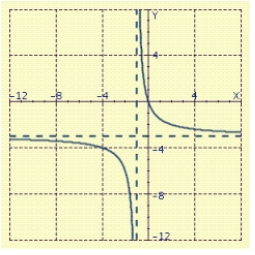
E)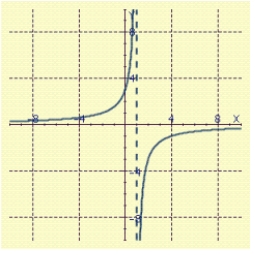
 .
. 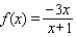
A)

B)

C)

D)

E)


4
Find all values of 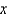 such that
such that 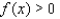
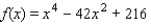
A)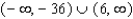
B)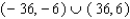
C)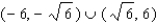
D)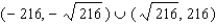
E)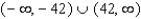
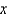 such that
such that 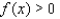
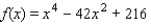
A)
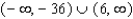
B)
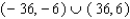
C)
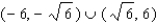
D)
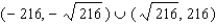
E)
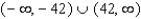

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
5
A meteorologist determines that the temperature T  for a certain 24-hour period in winter was given by the following formula.
for a certain 24-hour period in winter was given by the following formula. 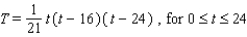
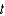 is time in hours and
is time in hours and 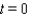 corresponds to 6 A.M.
corresponds to 6 A.M.
A)-16 < t < -24
B)0 < t < 8
C)0 < t < 24
D)16 < t < 24
E)0 < t < 16
 for a certain 24-hour period in winter was given by the following formula.
for a certain 24-hour period in winter was given by the following formula. 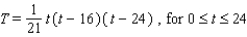
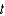 is time in hours and
is time in hours and 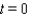 corresponds to 6 A.M.
corresponds to 6 A.M.A)-16 < t < -24
B)0 < t < 8
C)0 < t < 24
D)16 < t < 24
E)0 < t < 16

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
6
Show that the number is a zero of 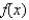 of the given multiplicity, and express
of the given multiplicity, and express 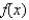 as a product of linear factors.
as a product of linear factors. 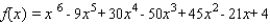
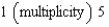
A)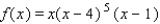
B)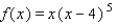
C)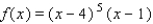
D)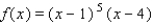
E)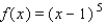
 of the given multiplicity, and express
of the given multiplicity, and express  as a product of linear factors.
as a product of linear factors. 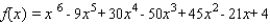
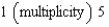
A)
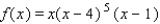
B)
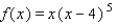
C)
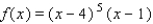
D)
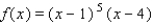
E)
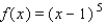

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
7
Use the remainder theorem to find 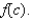
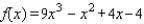 ,
, 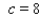
A)f ( 8 ) = 4,576
B)f ( 8 ) = 4,620
C)f ( 8 ) = 4,572
D)f ( 8 ) = 4,580
E)f ( 8 ) = 4,548
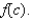
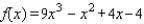 ,
, 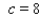
A)f ( 8 ) = 4,576
B)f ( 8 ) = 4,620
C)f ( 8 ) = 4,572
D)f ( 8 ) = 4,580
E)f ( 8 ) = 4,548

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
8
Use the factor theorem to decide whether 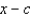 is a factor of the polynomial.
is a factor of the polynomial. 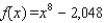 ;
; 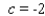
A)x - c is not a factor
B)x - c is a factor
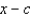 is a factor of the polynomial.
is a factor of the polynomial. 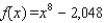 ;
; 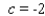
A)x - c is not a factor
B)x - c is a factor

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
9
Find the polynomial function of degree 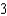 whose graph is shown in the figure.
whose graph is shown in the figure. 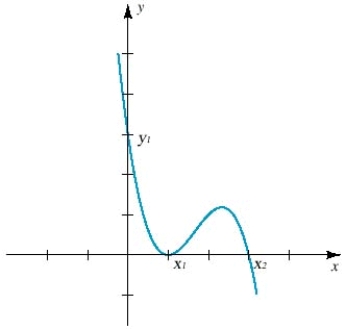
A)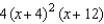
B)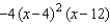
C)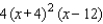
D)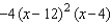
E)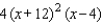
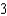 whose graph is shown in the figure.
whose graph is shown in the figure. 
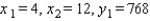
A)
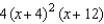
B)
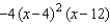
C)
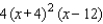
D)
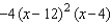
E)
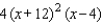

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
10
Find the quotient and remainder if 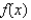 is divided by
is divided by 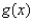 .
. 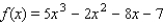 ,
, 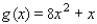
A)quotient: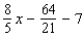 , remainder:
, remainder:
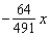
B)quotient: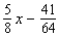 , remainder:
, remainder:
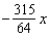
C)quotient: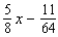 , remainder:
, remainder:
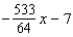
D)quotient :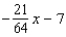 , remainder :
, remainder :
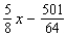
E)quotient: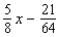 , remainder:
, remainder:
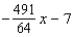
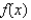 is divided by
is divided by 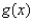 .
. 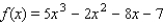 ,
, 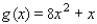
A)quotient:
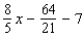 , remainder:
, remainder: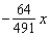
B)quotient:
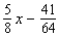 , remainder:
, remainder: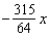
C)quotient:
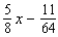 , remainder:
, remainder: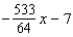
D)quotient :
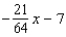 , remainder :
, remainder :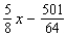
E)quotient:
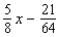 , remainder:
, remainder: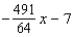

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
11
A herd of 575 deer is introduced onto a small island. At first the herd increases rapidly, but eventually food resources dwindle and the population declines. Suppose that the number of deer after  years is given by the following formula.
years is given by the following formula. 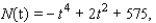 where
where 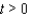 How many years does it take for the population to become extinct?
How many years does it take for the population to become extinct?
A)t = 8
B)t = 10
C)t = 4
D)t = 5
E)t = 6
 years is given by the following formula.
years is given by the following formula. 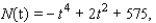 where
where 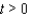 How many years does it take for the population to become extinct?
How many years does it take for the population to become extinct?A)t = 8
B)t = 10
C)t = 4
D)t = 5
E)t = 6

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
12
An aspirin tablet is in the shape of a right circular cylinder. The manufacturer also wishes to market the aspirin in capsule form. The capsule is to be 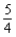 centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If
centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If 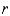 denotes the radius of a hemisphere, find a formula for the volume of the capsule.
denotes the radius of a hemisphere, find a formula for the volume of the capsule. 
A)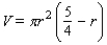
B)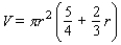
C)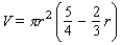
D)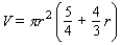
E)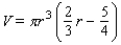
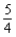 centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If
centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If 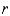 denotes the radius of a hemisphere, find a formula for the volume of the capsule.
denotes the radius of a hemisphere, find a formula for the volume of the capsule. 
A)
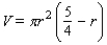
B)
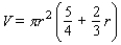
C)
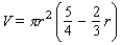
D)
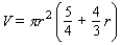
E)
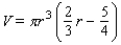

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
13
Find a polynomial with leading coefficient of 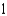 , degree
, degree 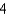 , and zeros: -
, and zeros: - 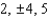 .
.
A)f ( x ) = x 4 - 3x 3 - 26x 2 + 26x + 160
B)f ( x ) = x 4 - 6x 2 - 22x + 40
C)f ( x ) = x 4 - 26x 2 + 48x + 160
D)f ( x ) = x 4 - 3x 3 - 26x 2 + 160
E)f ( x ) = x 4 - 3x 3 - 26x 2 + 48x + 160
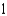 , degree
, degree 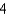 , and zeros: -
, and zeros: - 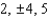 .
.A)f ( x ) = x 4 - 3x 3 - 26x 2 + 26x + 160
B)f ( x ) = x 4 - 6x 2 - 22x + 40
C)f ( x ) = x 4 - 26x 2 + 48x + 160
D)f ( x ) = x 4 - 3x 3 - 26x 2 + 160
E)f ( x ) = x 4 - 3x 3 - 26x 2 + 48x + 160

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
14
An arch has the shape of the parabola 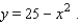 A rectangle is fit under the arch by selecting a point
A rectangle is fit under the arch by selecting a point 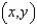 on the parabola (see the figure). If
on the parabola (see the figure). If 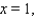 the rectangle has base
the rectangle has base 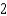 and height
and height 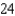 . Find the base of a second rectangle that has the same area.
. Find the base of a second rectangle that has the same area. 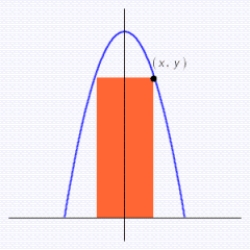
A)10.85
B)9.85
C)8.85
D)8.62
E)10.8
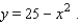 A rectangle is fit under the arch by selecting a point
A rectangle is fit under the arch by selecting a point 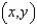 on the parabola (see the figure). If
on the parabola (see the figure). If 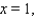 the rectangle has base
the rectangle has base 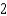 and height
and height 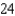 . Find the base of a second rectangle that has the same area.
. Find the base of a second rectangle that has the same area. 
A)10.85
B)9.85
C)8.85
D)8.62
E)10.8

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
15
Use synthetic division to decide whether 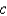 is a zero of
is a zero of 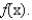
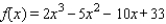 ;
; 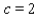
A)c is a zero of f ( x )
B)c is not a zero of f ( x )
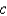 is a zero of
is a zero of 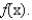
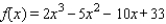 ;
; 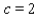
A)c is a zero of f ( x )
B)c is not a zero of f ( x )

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
16
Does there exist a polynomial of degree 3 with real coefficients that has zeros 9 , - 9, and i ?
A)no
B)yes
A)no
B)yes

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
17
Sketch the graph of 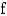 for the indicated value of
for the indicated value of 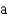 .
. 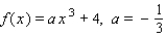
A)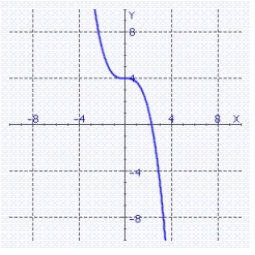
B)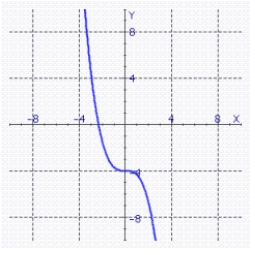
C)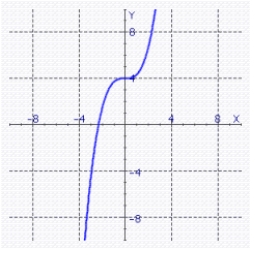
D)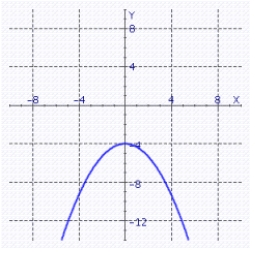
E)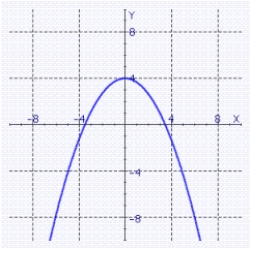
 for the indicated value of
for the indicated value of 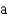 .
. 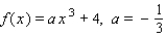
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
18
Find all solutions of the equation. 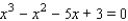
A)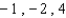
B)
C)
D)
E)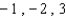
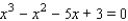
A)
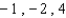
B)

C)

D)

E)

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
19
Find all solutions of the equation. 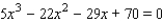
A)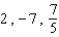
B)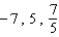
C)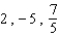
D)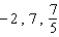
E)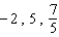
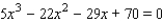
A)
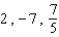
B)
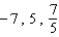
C)
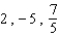
D)
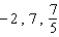
E)
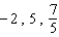

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
20
Find all solutions of the equation. 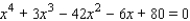
A)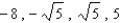
B)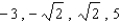
C)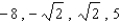
D)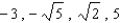
E)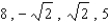
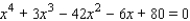
A)
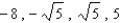
B)
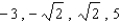
C)
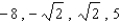
D)
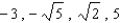
E)
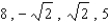

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
21
A flat metal plate is positioned in an xy-plane such that the temperature T (in  C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 20
C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 20  C, find the temperature at the point Q (6, 5).
C, find the temperature at the point Q (6, 5).
A)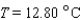
B)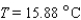
C)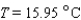
D)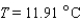
E)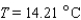
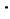 C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 20
C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 20 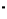 C, find the temperature at the point Q (6, 5).
C, find the temperature at the point Q (6, 5).A)
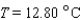
B)
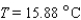
C)
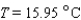
D)
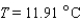
E)
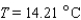

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
22
Use synthetic division to decide whether 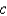 is a zero of
is a zero of 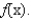
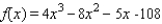 ;
; 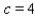
A)c is a zero of f ( x )
B)c is not a zero of f ( x )
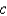 is a zero of
is a zero of 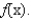
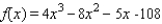 ;
; 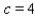
A)c is a zero of f ( x )
B)c is not a zero of f ( x )

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
23
Find an equation of a rational function 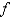 that satisfies the conditions. vertical asymptote: x = - 8, x = 0
that satisfies the conditions. vertical asymptote: x = - 8, x = 0
Horizontal asymptote: y = 0
X-intercept: 7; f (8) = 1
A)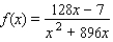
B)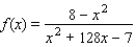
C)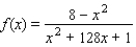
D)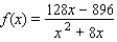
E)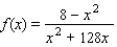
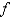 that satisfies the conditions. vertical asymptote: x = - 8, x = 0
that satisfies the conditions. vertical asymptote: x = - 8, x = 0Horizontal asymptote: y = 0
X-intercept: 7; f (8) = 1
A)
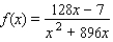
B)
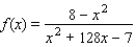
C)
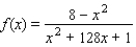
D)
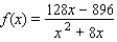
E)
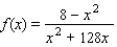

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
24
An arch has the shape of the parabola 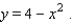 A rectangle is fit under the arch by selecting a point
A rectangle is fit under the arch by selecting a point 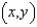 on the parabola (see the figure). If
on the parabola (see the figure). If 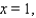 the rectangle has base
the rectangle has base 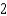 and height
and height 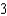 . Find the base of a second rectangle that has the same area.
. Find the base of a second rectangle that has the same area. 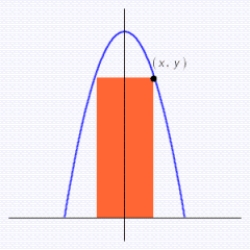
A)2.61
B)3.61
C)4.61
D)4.46
E)5
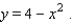 A rectangle is fit under the arch by selecting a point
A rectangle is fit under the arch by selecting a point 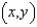 on the parabola (see the figure). If
on the parabola (see the figure). If 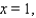 the rectangle has base
the rectangle has base 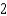 and height
and height 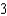 . Find the base of a second rectangle that has the same area.
. Find the base of a second rectangle that has the same area. 
A)2.61
B)3.61
C)4.61
D)4.46
E)5

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
25
Find a polynomial with leading coefficient of 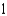 , degree
, degree 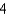 , and zeros: -
, and zeros: - 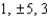 .
.
A)f ( x ) = x 4 - 6x 2 - 13x + 15
B)f ( x ) = x 4 - 2x 3 - 28x 2 + 50x + 75
C)f ( x ) = x 4 - 2x 3 - 28x 2 + 75
D)f ( x ) = x 4 - 2x 3 - 28x 2 + 28x + 75
E)f ( x ) = x 4 - 28x 2 + 50x + 75
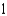 , degree
, degree 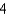 , and zeros: -
, and zeros: - 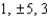 .
.A)f ( x ) = x 4 - 6x 2 - 13x + 15
B)f ( x ) = x 4 - 2x 3 - 28x 2 + 50x + 75
C)f ( x ) = x 4 - 2x 3 - 28x 2 + 75
D)f ( x ) = x 4 - 2x 3 - 28x 2 + 28x + 75
E)f ( x ) = x 4 - 28x 2 + 50x + 75

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
26
A herd of 575 deer is introduced onto a small island. At first the herd increases rapidly, but eventually food resources dwindle and the population declines. Suppose that the number of deer after 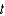 years is given by the following formula.
years is given by the following formula. 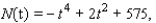 where
where 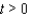 How many years does it take for the population to become extinct?
How many years does it take for the population to become extinct?
A)t = 10
B)t = 8
C)t = 5
D)t = 6
E)t = 4
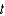 years is given by the following formula.
years is given by the following formula. 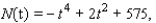 where
where 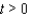 How many years does it take for the population to become extinct?
How many years does it take for the population to become extinct?A)t = 10
B)t = 8
C)t = 5
D)t = 6
E)t = 4

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
27
A thin flat plate is situated in an xy-plane such that the density d ( in lb/ft 2 ) at the point is inversely proportional to the square of the distance from the origin. What is the effect on the density at P if the x- and y-coordinates are each multiplied by 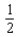
A)d is multiplied 2
B)d is multiplied 2.83
C)d is multiplied 0.25
D)d is multiplied 0.50
E)d is multiplied 4
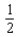
A)d is multiplied 2
B)d is multiplied 2.83
C)d is multiplied 0.25
D)d is multiplied 0.50
E)d is multiplied 4

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
28
Show that the number is a zero of 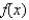 of the given multiplicity, and express
of the given multiplicity, and express 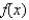 as a product of linear factors.
as a product of linear factors. 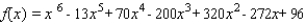
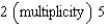
A)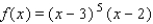
B)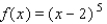
C)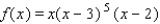
D)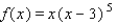
E)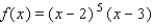
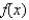 of the given multiplicity, and express
of the given multiplicity, and express 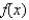 as a product of linear factors.
as a product of linear factors. 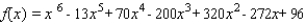
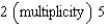
A)
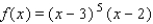
B)
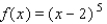
C)
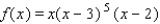
D)
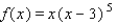
E)
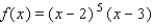

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
29
An aspirin tablet is in the shape of a right circular cylinder. The manufacturer also wishes to market the aspirin in capsule form. The capsule is to be 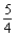 centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If
centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If 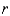 denotes the radius of a hemisphere, find a formula for the volume of the capsule.
denotes the radius of a hemisphere, find a formula for the volume of the capsule. 
A)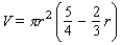
B)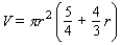
C)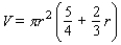
D)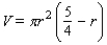
E)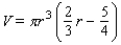
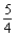 centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If
centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If 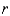 denotes the radius of a hemisphere, find a formula for the volume of the capsule.
denotes the radius of a hemisphere, find a formula for the volume of the capsule. 
A)
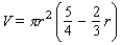
B)
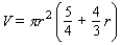
C)
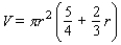
D)
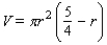
E)
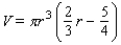

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
30
Find all values of 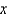 such that
such that 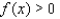
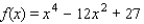
A)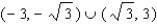
B)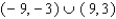
C)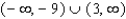
D)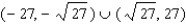
E)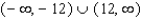
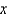 such that
such that 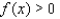
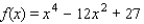
A)
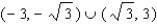
B)
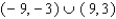
C)
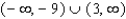
D)
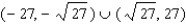
E)
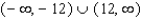

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
31
Sketch the graph of  for the indicated value of
for the indicated value of 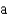 .
. 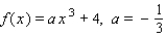
A)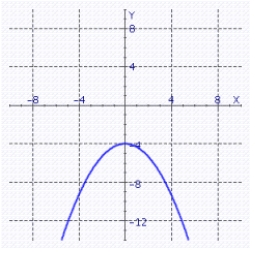
B)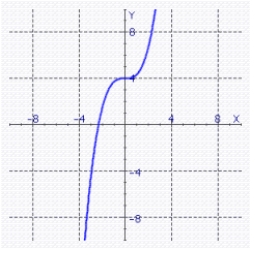
C)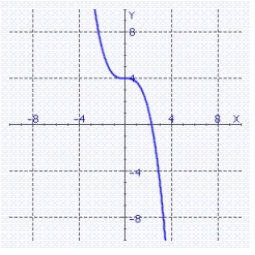
D)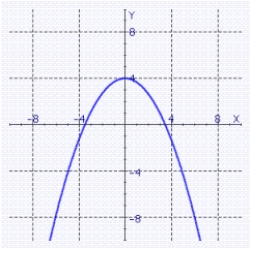
E)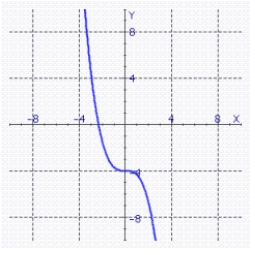
 for the indicated value of
for the indicated value of 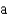 .
. 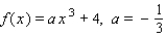
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
32
A meteorologist determines that the temperature T 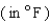 for a certain 24-hour period in winter was given by the following formula.
for a certain 24-hour period in winter was given by the following formula. 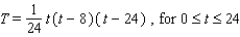
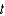 is time in hours and
is time in hours and 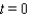 corresponds to 6 A.M.
corresponds to 6 A.M.
A)-8 < t < -24
B)0 < t < 24
C)0 < t < 16
D)8 < t < 24
E)0 < t < 8
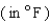 for a certain 24-hour period in winter was given by the following formula.
for a certain 24-hour period in winter was given by the following formula. 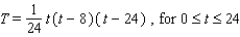
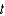 is time in hours and
is time in hours and 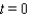 corresponds to 6 A.M.
corresponds to 6 A.M.A)-8 < t < -24
B)0 < t < 24
C)0 < t < 16
D)8 < t < 24
E)0 < t < 8

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
33
The period P of a simple pendulum - that is, the time required for one complete oscillation - is directly proportional to the square root of its length l. Express P in terms of l and a constant of proportionality k. If a pendulum 3.8 feet long has a period of 2.3 seconds, find the value of k.
A)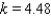
B)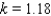
C)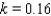
D)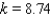
E)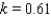
A)
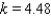
B)
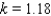
C)
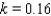
D)
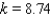
E)
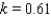

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
34
Find the quotient and remainder if 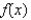 is divided by
is divided by 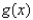 .
. 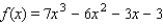 ,
, 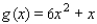
A)quotient :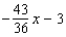 , remainder :
, remainder :
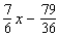
B)quotient: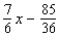 , remainder:
, remainder:
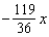
C)quotient: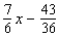 , remainder:
, remainder:
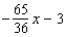
D)quotient: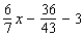 , remainder:
, remainder:
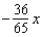
E)quotient: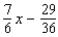 , remainder:
, remainder:
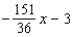
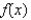 is divided by
is divided by 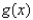 .
. 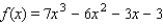 ,
, 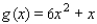
A)quotient :
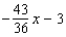 , remainder :
, remainder :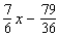
B)quotient:
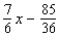 , remainder:
, remainder: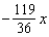
C)quotient:
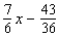 , remainder:
, remainder: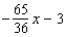
D)quotient:
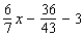 , remainder:
, remainder: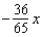
E)quotient:
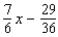 , remainder:
, remainder: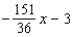

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
35
For the following function, find a number 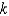 such that the graph of
such that the graph of 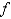 contains the point
contains the point 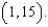
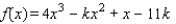
A)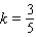
B)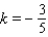
C)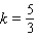
D)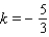
E)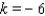
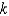 such that the graph of
such that the graph of 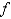 contains the point
contains the point 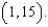
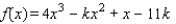
A)
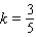
B)
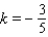
C)
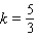
D)
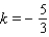
E)
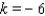

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
36
Find the polynomial function of degree 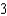 whose graph is shown in the figure.
whose graph is shown in the figure. 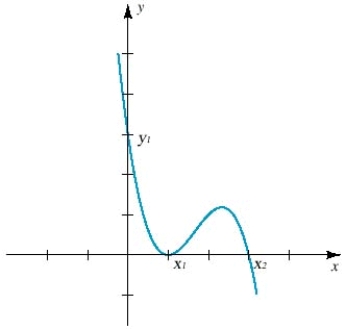
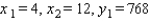
A)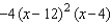
B)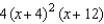
C)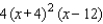
D)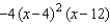
E)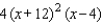
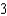 whose graph is shown in the figure.
whose graph is shown in the figure. 
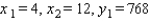
A)
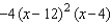
B)
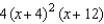
C)
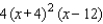
D)
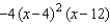
E)
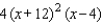

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
37
Express the statement as a formula that involves the variables u, v and a constant of proportionality k, and determine the value of k from the condition : u is directly proportional to v and if v = 40, then u = 3
A)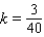
B)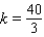
C)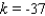
D)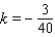
E)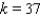
A)
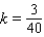
B)
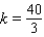
C)
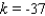
D)
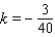
E)
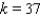

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
38
Use the remainder theorem to find 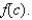
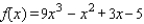 ,
, 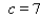
A)f ( 7 ) = 3,064
B)f ( 7 ) = 3,094
C)f ( 7 ) = 3,054
D)f ( 7 ) = 3,040
E)f ( 7 ) = 3,059
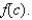
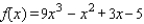 ,
, 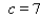
A)f ( 7 ) = 3,064
B)f ( 7 ) = 3,094
C)f ( 7 ) = 3,054
D)f ( 7 ) = 3,040
E)f ( 7 ) = 3,059

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
39
Use the factor theorem to decide whether 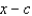 is a factor of the polynomial.
is a factor of the polynomial.  ;
; 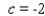
A)x - c is not a factor
B)x - c is a factor
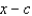 is a factor of the polynomial.
is a factor of the polynomial.  ;
; 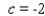
A)x - c is not a factor
B)x - c is a factor

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
40
Find all solutions of the equation. 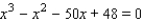
A)
B)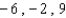
C)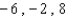
D)
E)
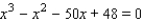
A)

B)
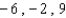
C)
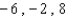
D)

E)


Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
41
Find all values of 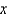 such that
such that 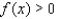
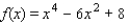
A)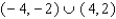
B)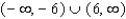
C)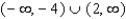
D)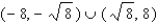
E)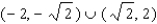
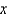 such that
such that 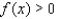
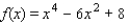
A)
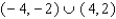
B)
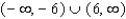
C)
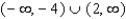
D)
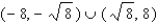
E)
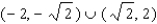

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
42
A herd of 575 deer is introduced onto a small island. At first the herd increases rapidly, but eventually food resources dwindle and the population declines. Suppose that the number of deer after 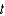 years is given by the following formula.
years is given by the following formula. 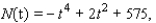 where
where 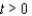 How many years does it take for the population to become extinct?
How many years does it take for the population to become extinct?
A)t = 4
B)t = 6
C)t = 10
D)t = 8
E)t = 5
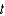 years is given by the following formula.
years is given by the following formula. 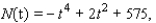 where
where 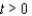 How many years does it take for the population to become extinct?
How many years does it take for the population to become extinct?A)t = 4
B)t = 6
C)t = 10
D)t = 8
E)t = 5

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
43
Find a polynomial with leading coefficient of 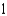 , degree
, degree 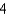 , and zeros: -
, and zeros: - 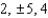 .
.
A)f ( x ) = x 4 - 33x 2 + 50x + 200
B)f ( x ) = x 4 - 2x 3 - 33x 2 + 200
C)f ( x ) = x 4 - 2x 3 - 33x 2 + 33x + 200
D)f ( x ) = x 4 - 2x 3 - 33x 2 + 50x + 200
E)f ( x ) = x 4 - 7x 2 - 18x + 40
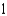 , degree
, degree 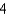 , and zeros: -
, and zeros: - 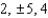 .
.A)f ( x ) = x 4 - 33x 2 + 50x + 200
B)f ( x ) = x 4 - 2x 3 - 33x 2 + 200
C)f ( x ) = x 4 - 2x 3 - 33x 2 + 33x + 200
D)f ( x ) = x 4 - 2x 3 - 33x 2 + 50x + 200
E)f ( x ) = x 4 - 7x 2 - 18x + 40

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
44
The period P of a simple pendulum - that is, the time required for one complete oscillation - is directly proportional to the square root of its length l. Express P in terms of l and a constant of proportionality k. If a pendulum 3.5 feet long has a period of 1.3 seconds, find the value of k.
A)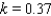
B)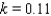
C)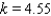
D)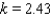
E)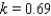
A)
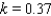
B)
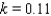
C)
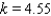
D)
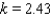
E)

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
45
Sketch the graph of 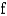 for the indicated value of
for the indicated value of 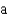 .
. 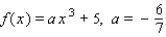
A)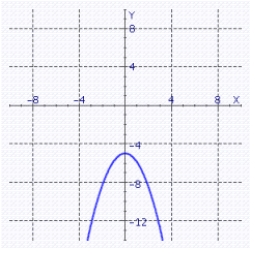
B)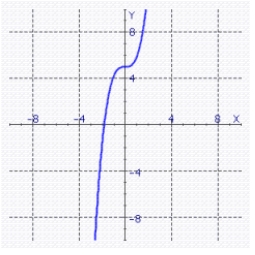
C)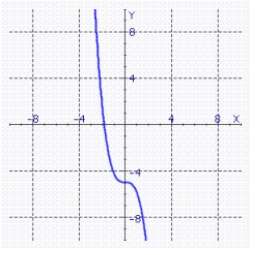
D)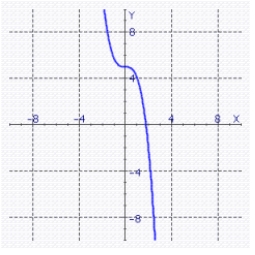
E)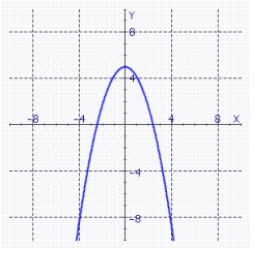
 for the indicated value of
for the indicated value of  .
. 
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
46
Find all solutions of the equation. 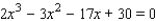
A)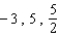
B)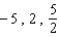
C)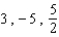
D)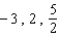
E)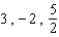
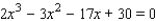
A)
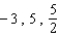
B)
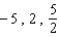
C)
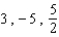
D)
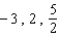
E)
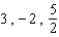

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
47
Express the statement as a formula that involves the variables u, v and a constant of proportionality k, and determine the value of k from the condition : u is directly proportional to v and if v = 30, then u = 3
A)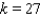
B)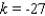
C)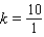
D)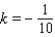
E)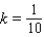
A)
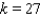
B)
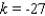
C)
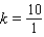
D)
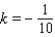
E)
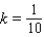

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
48
Does there exist a polynomial of degree 3 with real coefficients that has zeros 7 , - 7, and i ?
A)no
B)yes
A)no
B)yes

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
49
A meteorologist determines that the temperature T 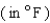 for a certain 24-hour period in winter was given by the following formula.
for a certain 24-hour period in winter was given by the following formula. 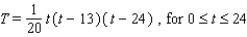
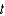 is time in hours and
is time in hours and 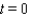 corresponds to 6 A.M.
corresponds to 6 A.M.
A)13 < t < 24
B)0 < t < 11
C)0 < t < 24
D)-13 < t < -24
E)0 < t < 13
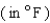 for a certain 24-hour period in winter was given by the following formula.
for a certain 24-hour period in winter was given by the following formula. 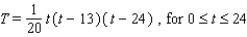
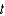 is time in hours and
is time in hours and 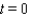 corresponds to 6 A.M.
corresponds to 6 A.M.A)13 < t < 24
B)0 < t < 11
C)0 < t < 24
D)-13 < t < -24
E)0 < t < 13

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
50
Sketch the graph of 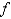 .
. 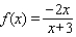
A)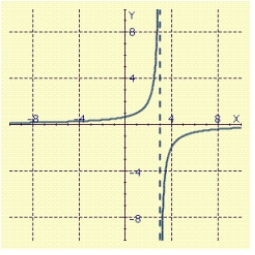
B)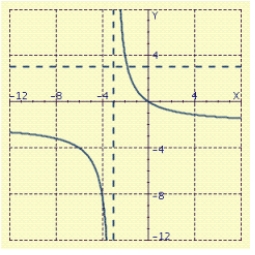
C)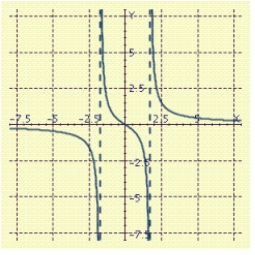
D)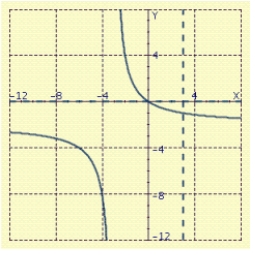
E)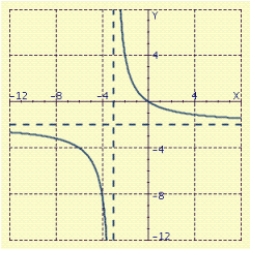
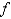 .
. 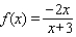
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
51
Use the remainder theorem to find 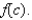
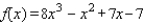 ,
, 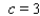
A)f ( 3 ) = 228
B)f ( 3 ) = 181
C)f ( 3 ) = 221
D)f ( 3 ) = 235
E)f ( 3 ) = 203
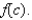
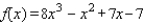 ,
, 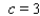
A)f ( 3 ) = 228
B)f ( 3 ) = 181
C)f ( 3 ) = 221
D)f ( 3 ) = 235
E)f ( 3 ) = 203

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
52
Use synthetic division to decide whether 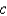 is a zero of
is a zero of 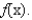
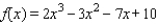 ;
; 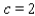
A)c is not a zero of f ( x )
B)c is a zero of f ( x )
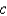 is a zero of
is a zero of 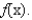
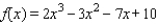 ;
; 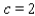
A)c is not a zero of f ( x )
B)c is a zero of f ( x )

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
53
For the following function, find a number 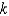 such that the graph of
such that the graph of 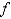 contains the point
contains the point 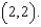
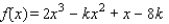
A)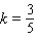
B)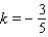
C)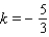
D)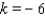
E)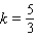
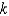 such that the graph of
such that the graph of 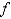 contains the point
contains the point 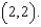
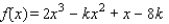
A)
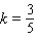
B)
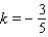
C)
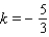
D)
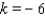
E)
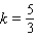

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
54
Find an equation of a rational function 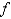 that satisfies the conditions. vertical asymptote: x = - 2, x = 0
that satisfies the conditions. vertical asymptote: x = - 2, x = 0
Horizontal asymptote: y = 0
X-intercept: 3; f (4) = 1
A)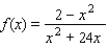
B)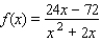
C)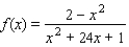
D)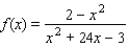
E)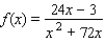
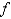 that satisfies the conditions. vertical asymptote: x = - 2, x = 0
that satisfies the conditions. vertical asymptote: x = - 2, x = 0Horizontal asymptote: y = 0
X-intercept: 3; f (4) = 1
A)
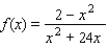
B)
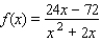
C)
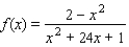
D)
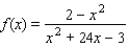
E)
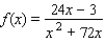

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
55
Find all solutions of the equation. 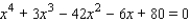
A)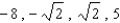
B)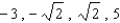
C)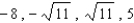
D)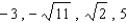
E)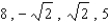
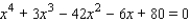
A)
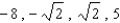
B)
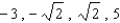
C)
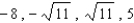
D)
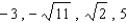
E)
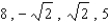

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
56
Use the factor theorem to decide whether 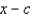 is a factor of the polynomial.
is a factor of the polynomial. 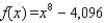 ;
; 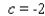
A)x - c is a factor
B)x - c is not a factor
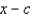 is a factor of the polynomial.
is a factor of the polynomial. 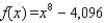 ;
; 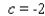
A)x - c is a factor
B)x - c is not a factor

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
57
An arch has the shape of the parabola 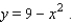 A rectangle is fit under the arch by selecting a point
A rectangle is fit under the arch by selecting a point 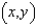 on the parabola (see the figure). If
on the parabola (see the figure). If 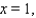 the rectangle has base
the rectangle has base 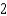 and height
and height  . Find the base of a second rectangle that has the same area.
. Find the base of a second rectangle that has the same area. 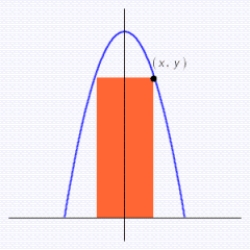
A)6.66
B)6.74
C)5.74
D)4.74
E)6.1
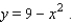 A rectangle is fit under the arch by selecting a point
A rectangle is fit under the arch by selecting a point 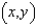 on the parabola (see the figure). If
on the parabola (see the figure). If 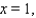 the rectangle has base
the rectangle has base 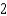 and height
and height  . Find the base of a second rectangle that has the same area.
. Find the base of a second rectangle that has the same area. 
A)6.66
B)6.74
C)5.74
D)4.74
E)6.1

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
58
A thin flat plate is situated in an xy-plane such that the density d ( in lb/ft 2 ) at the point is inversely proportional to the square of the distance from the origin. What is the effect on the density at P if the x- and y-coordinates are each multiplied by 
A)d is multiplied 0.14
B)d is multiplied 7
C)d is multiplied 49
D)d is multiplied 0.02
E)d is multiplied 9.90

A)d is multiplied 0.14
B)d is multiplied 7
C)d is multiplied 49
D)d is multiplied 0.02
E)d is multiplied 9.90

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
59
Find all solutions of the equation. 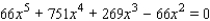
A)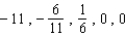
B)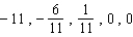
C)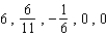
D)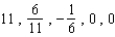
E)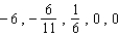
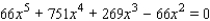
A)
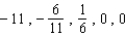
B)
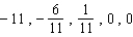
C)
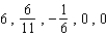
D)
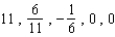
E)
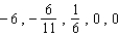

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
60
A flat metal plate is positioned in an xy-plane such that the temperature T (in  C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 30
C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 30  C, find the temperature at the point Q (6, 8).
C, find the temperature at the point Q (6, 8).
A)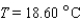
B)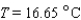
C)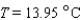
D)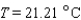
E)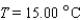
 C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 30
C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 30 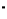 C, find the temperature at the point Q (6, 8).
C, find the temperature at the point Q (6, 8).A)
B)
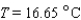
C)
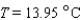
D)
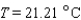
E)
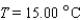

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
61
Find the polynomial function of degree 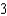 whose graph is shown in the figure.
whose graph is shown in the figure. 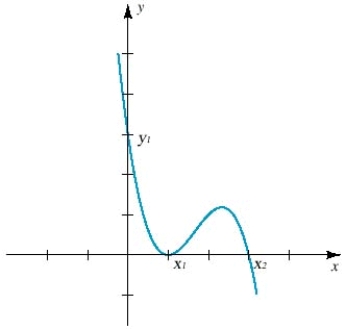
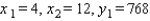
A)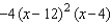
B)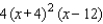
C)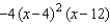
D)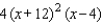
E)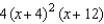
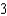 whose graph is shown in the figure.
whose graph is shown in the figure. 
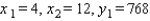
A)
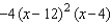
B)
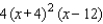
C)
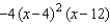
D)
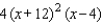
E)
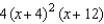

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
62
An aspirin tablet is in the shape of a right circular cylinder. The manufacturer also wishes to market the aspirin in capsule form. The capsule is to be 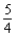 centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If
centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If 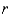 denotes the radius of a hemisphere, find a formula for the volume of the capsule.
denotes the radius of a hemisphere, find a formula for the volume of the capsule. 
A)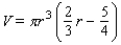
B)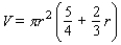
C)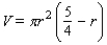
D)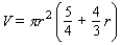
E)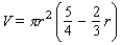
 centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If
centimeters long, in the shape of a right circular cylinder with hemispheres attached at both ends (see the figure below). If 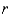 denotes the radius of a hemisphere, find a formula for the volume of the capsule.
denotes the radius of a hemisphere, find a formula for the volume of the capsule. 
A)
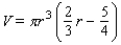
B)
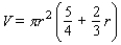
C)
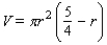
D)
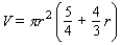
E)
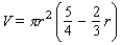

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
63
Find all solutions of the equation. 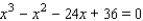
A)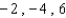
B)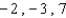
C)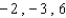
D)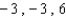
E)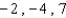
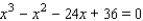
A)
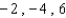
B)
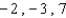
C)
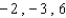
D)
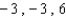
E)
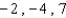

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
64
Find all solutions of the equation. 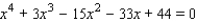
A)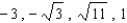
B)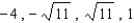
C)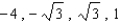
D)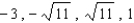
E)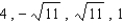
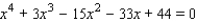
A)
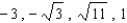
B)
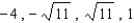
C)
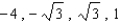
D)
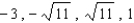
E)
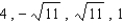

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
65
Find all values of 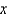 such that
such that 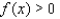
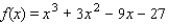
A)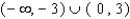
B)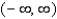
C)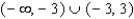
D)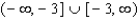
E)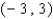
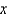 such that
such that 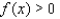
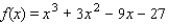
A)
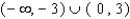
B)
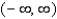
C)
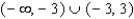
D)
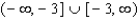
E)
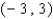

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
66
A flat metal plate is positioned in an xy-plane such that the temperature T (in 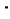 C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 15
C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 15 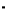 C, find the temperature at the point Q (9, 8).
C, find the temperature at the point Q (9, 8).
A)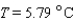
B)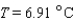
C)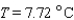
D)
E)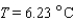
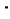 C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 15
C) at the point (x, y) is inversely proportional to the distance from the origin. If the temperature at the point P (3, 4) is 15 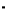 C, find the temperature at the point Q (9, 8).
C, find the temperature at the point Q (9, 8).A)
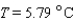
B)
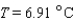
C)
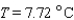
D)

E)
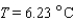

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
67
Find the quotient and remainder if 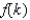 is divided by
is divided by 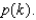
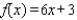
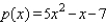
A)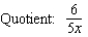
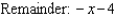
B)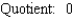
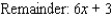
C)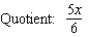
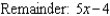
D)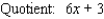
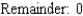
E)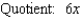
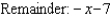
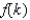 is divided by
is divided by 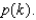
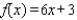
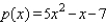
A)
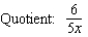
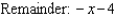
B)
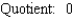
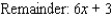
C)
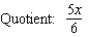
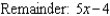
D)
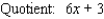
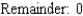
E)
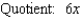
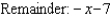

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
68
Find all solutions of the equation. 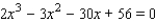
A)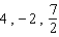
B)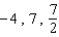
C)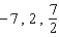
D)
E)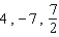
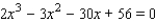
A)
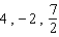
B)
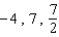
C)
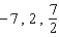
D)

E)
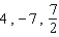

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
69
Show that the number is a zero of 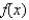 of the given multiplicity, and express
of the given multiplicity, and express 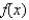 as a product of linear factors.
as a product of linear factors. 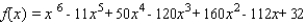
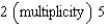
A)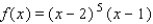
B)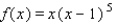
C)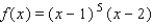
D)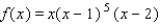
E)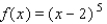
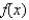 of the given multiplicity, and express
of the given multiplicity, and express 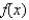 as a product of linear factors.
as a product of linear factors. 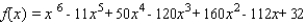
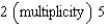
A)
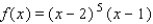
B)
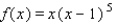
C)
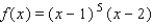
D)
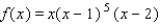
E)
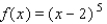

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
70
From a rectangular piece of cardboard having dimensions W = 16 and L = 30 an open box is to be made by cutting out identical squares of area x 2 from each corner and turning up the sides (see Illustration). Find all positive values of x such that the volume of the box V ( x ) > 0. Include only allowable values of x in your answer. 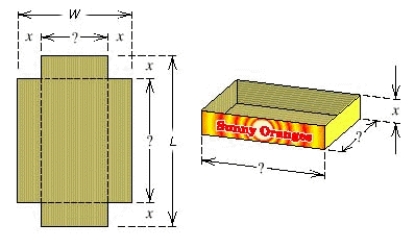
A)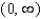
B)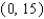
C)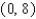
D)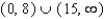
E)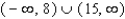

A)
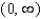
B)
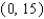
C)
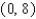
D)
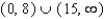
E)
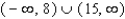

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
71
Express the statement as a formula that involves the variables u, v and a constant of proportionality k, and determine the value of k from the condition : u is directly proportional to v and if v = 40, then u = 3
A)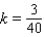
B)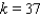
C)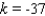
D)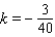
E)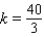
A)
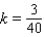
B)
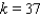
C)
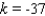
D)
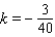
E)
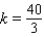

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
72
Find an equation of a rational function 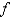 that satisfies the conditions. vertical asymptote: x = - 1, x = 0
that satisfies the conditions. vertical asymptote: x = - 1, x = 0
Horizontal asymptote: y = 0
X-intercept: 8; f (9) = 1
A)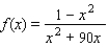
B)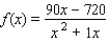
C)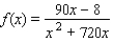
D)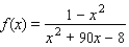
E)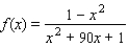
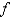 that satisfies the conditions. vertical asymptote: x = - 1, x = 0
that satisfies the conditions. vertical asymptote: x = - 1, x = 0Horizontal asymptote: y = 0
X-intercept: 8; f (9) = 1
A)
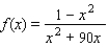
B)
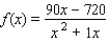
C)
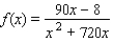
D)
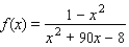
E)
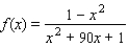

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
73
Find all values of 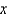 such that
such that 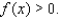
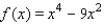
A)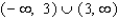
B)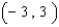
C)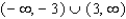
D)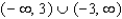
E)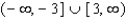
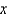 such that
such that 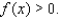
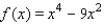
A)
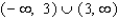
B)
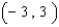
C)
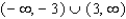
D)
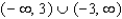
E)
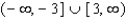

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
74
Find the quotient and remainder if 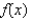 is divided by
is divided by 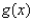 .
. 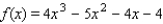 ,
, 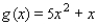
A)quotient: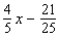 , remainder:
, remainder:
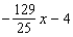
B)quotient: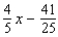 , remainder:
, remainder:
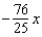
C)quotient :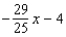 , remainder :
, remainder :
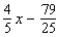
D)quotient: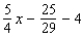 , remainder:
, remainder:
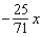
E)quotient: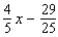 , remainder:
, remainder:
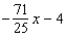
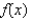 is divided by
is divided by 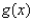 .
. 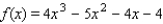 ,
, 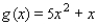
A)quotient:
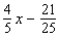 , remainder:
, remainder: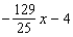
B)quotient:
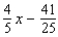 , remainder:
, remainder: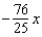
C)quotient :
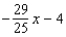 , remainder :
, remainder :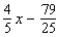
D)quotient:
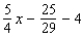 , remainder:
, remainder: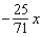
E)quotient:
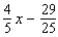 , remainder:
, remainder: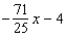

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
75
Sketch the graph of 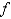 .
. 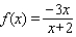
A)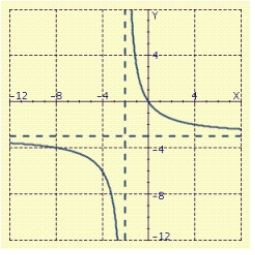
B)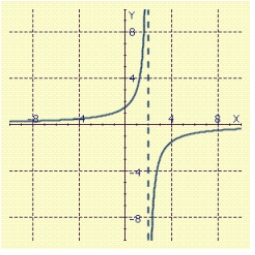
C)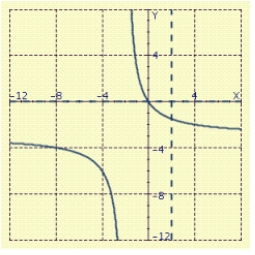
D)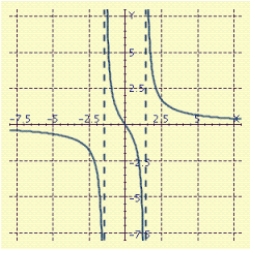
E)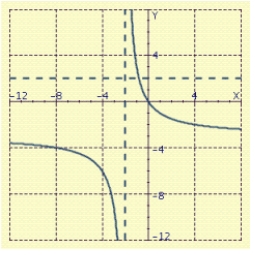
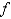 .
. 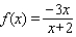
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
76
A thin flat plate is situated in an xy-plane such that the density d ( in lb/ft 2 ) at the point is inversely proportional to the square of the distance from the origin. What is the effect on the density at P if the x- and y-coordinates are each multiplied by 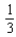
A)d is multiplied 0.11
B)d is multiplied 0.33
C)d is multiplied 3
D)d is multiplied 9
E)d is multiplied 4.24
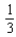
A)d is multiplied 0.11
B)d is multiplied 0.33
C)d is multiplied 3
D)d is multiplied 9
E)d is multiplied 4.24

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
77
The period P of a simple pendulum - that is, the time required for one complete oscillation - is directly proportional to the square root of its length l. Express P in terms of l and a constant of proportionality k. If a pendulum 2.7 feet long has a period of 2.3 seconds, find the value of k.
A)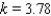
B)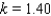
C)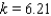
D)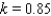
E)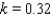
A)
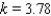
B)
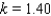
C)
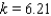
D)
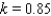
E)
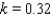

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
78
Find all values of 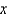 such that
such that 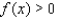
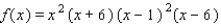 .
.
A)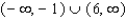
B)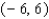
C)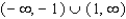
D)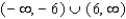
E)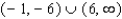
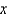 such that
such that 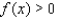
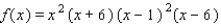 .
.A)
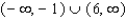
B)
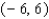
C)
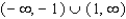
D)
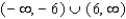
E)
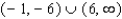

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
79
Find all solutions of the equation. 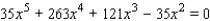
A)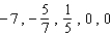
B)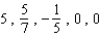
C)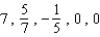
D)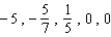
E)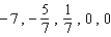
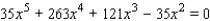
A)
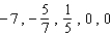
B)
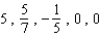
C)
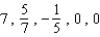
D)
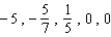
E)
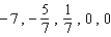

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck
80
Does there exist a polynomial of degree 3 with real coefficients that has zeros 5 , - 5, and i ?
A)yes
B)no
A)yes
B)no

Unlock Deck
Unlock for access to all 147 flashcards in this deck.
Unlock Deck
k this deck



