Deck 14: Multiple Integration
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/143
Play
Full screen (f)
Deck 14: Multiple Integration
1
Use spherical coordinates to find the volume of the solid inside 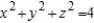 and outside
and outside 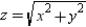 , and above the xy-plane.
, and above the xy-plane.
A)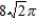
B)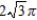
C)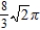
D)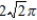
E)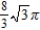
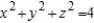 and outside
and outside 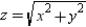 , and above the xy-plane.
, and above the xy-plane. A)
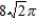
B)
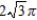
C)
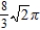
D)
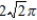
E)
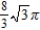
C
2
Given 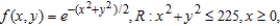 use polar coordinates to set up and evaluate the double integral
use polar coordinates to set up and evaluate the double integral 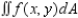 .
.
A)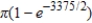
B)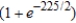
C)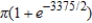
D)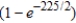
E)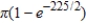
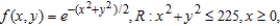 use polar coordinates to set up and evaluate the double integral
use polar coordinates to set up and evaluate the double integral 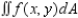 .
.A)
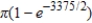
B)
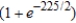
C)
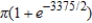
D)
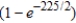
E)
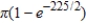
E
3
Find the mass of the lamina described by the inequalities 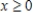 and
and 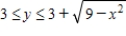 , given that its density is
, given that its density is 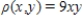 .
.
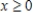 and
and 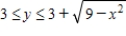 , given that its density is
, given that its density is 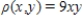 .
.A
4
Find the average value of 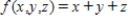 over the region Q, where Q is a tetrahedron in the first octant with vertices
over the region Q, where Q is a tetrahedron in the first octant with vertices 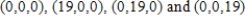 . The average value of a continuous function
. The average value of a continuous function 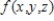 over a solid region Q is
over a solid region Q is 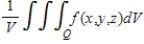 , where V is the volume of the solid region Q.
, where V is the volume of the solid region Q.
A) 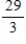
B) 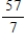
C) 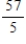
D) 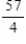
E)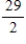
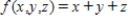 over the region Q, where Q is a tetrahedron in the first octant with vertices
over the region Q, where Q is a tetrahedron in the first octant with vertices 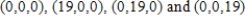 . The average value of a continuous function
. The average value of a continuous function 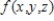 over a solid region Q is
over a solid region Q is 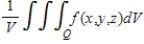 , where V is the volume of the solid region Q.
, where V is the volume of the solid region Q. A)
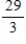
B)
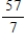
C)
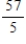
D)
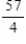
E)
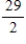

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
5
Find the mass of the lamina bounded by the graphs of the equations 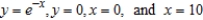 for the density
for the density 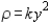 .
.
A)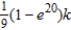
B)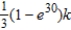
C)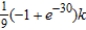
D)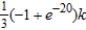
E)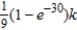
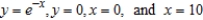 for the density
for the density 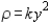 .
. A)
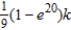
B)
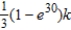
C)
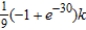
D)
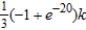
E)
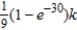

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
6
Find the centroid of the solid region bounded by the graphs of the equations. Use a computer algebra system to evaluate the triple integral. (Assume uniform density and find the center of mass.) 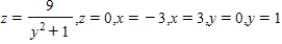
A)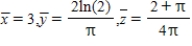
B) 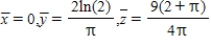
C) 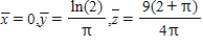
D) 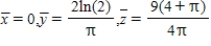
E) 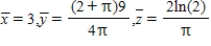
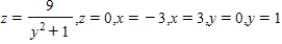
A)
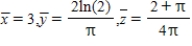
B)
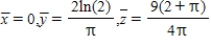
C)
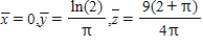
D)
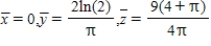
E)
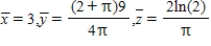

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
7
Find the center of mass of the lamina bounded by the graphs of the equations 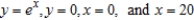 for the density
for the density 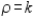 .
.
A)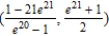
B)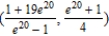
C)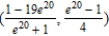
D)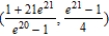
E)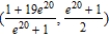
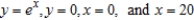 for the density
for the density 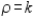 .
.A)
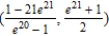
B)
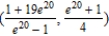
C)
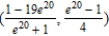
D)
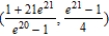
E)
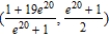

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
8
Evaluate the iterated integral 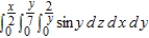 .
.
A)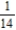
B)
C)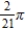
D)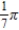
E)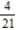
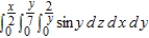 .
.A)
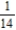
B)

C)
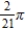
D)
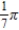
E)
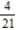

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
9
Find the average value of 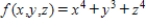 over the region Q, where Q is a cube in the first octant bounded by the coordinate planes, and the planes
over the region Q, where Q is a cube in the first octant bounded by the coordinate planes, and the planes 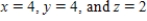 . The average value of a continuous function
. The average value of a continuous function 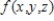 over a solid region Q is
over a solid region Q is 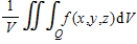 , where V is the volume of the solid region Q.
, where V is the volume of the solid region Q.
A) 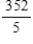
B) 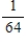
C) 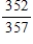
D) 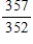
E) 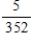
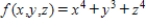 over the region Q, where Q is a cube in the first octant bounded by the coordinate planes, and the planes
over the region Q, where Q is a cube in the first octant bounded by the coordinate planes, and the planes 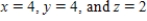 . The average value of a continuous function
. The average value of a continuous function 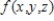 over a solid region Q is
over a solid region Q is 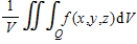 , where V is the volume of the solid region Q.
, where V is the volume of the solid region Q. A)
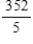
B)
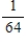
C)
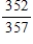
D)
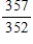
E)
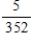

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
10
Write a double integral that represents the surface area of 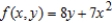 over the region R: triangle with vertices
over the region R: triangle with vertices 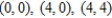 . Use a computer algebra system to evaluate the double integral. Round your answer to two decimal places.
. Use a computer algebra system to evaluate the double integral. Round your answer to two decimal places.
A)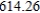
B)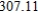
C)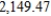
D)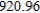
E)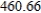
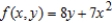 over the region R: triangle with vertices
over the region R: triangle with vertices 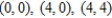 . Use a computer algebra system to evaluate the double integral. Round your answer to two decimal places.
. Use a computer algebra system to evaluate the double integral. Round your answer to two decimal places. A)
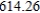
B)
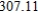
C)
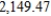
D)
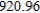
E)
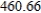

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
11
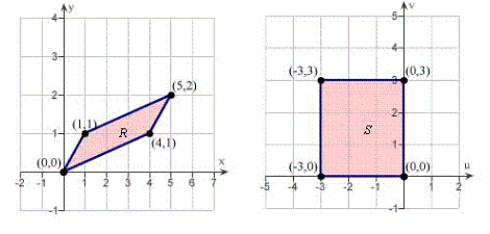
Find a transformation 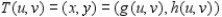 that when applied to region
that when applied to region 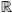 , its image will be
, its image will be 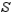 .
. 
A)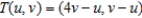
B)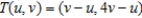
C)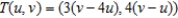
D)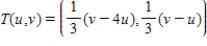
E)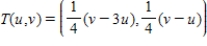
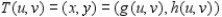 that when applied to region
that when applied to region 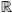 , its image will be
, its image will be 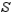 .
. 
A)
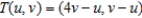
B)
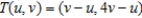
C)
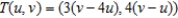
D)
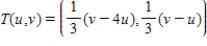
E)
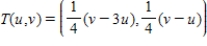

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
12
Set up an integral for both orders of integration, and use the more convenient order to evaluate the integral below over the region R. 
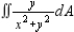

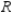
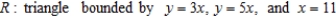
A)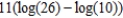
B)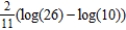
C)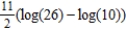
D)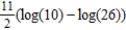
E)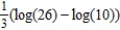

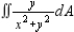

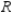
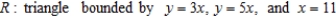
A)
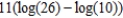
B)
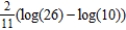
C)
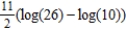
D)
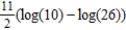
E)
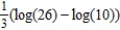

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
13
Identify the region of integration for the following integral. 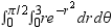
A)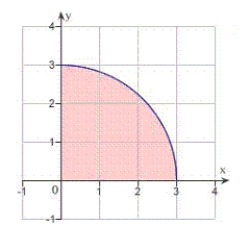
B)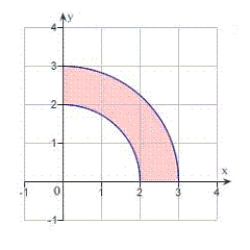
C)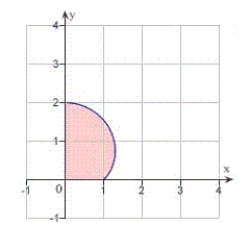
D)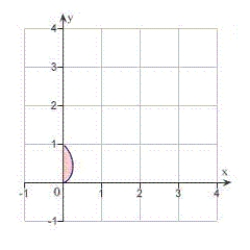
E)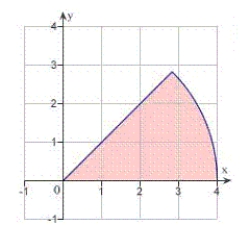
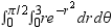
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
14
The area of a region R is given by the iterated integral 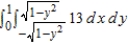 . Switch the order of integration and show that both orders yield the same area. What is this area?
. Switch the order of integration and show that both orders yield the same area. What is this area?
A)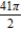
B)
C)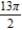
D)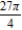
E)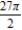
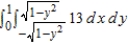 . Switch the order of integration and show that both orders yield the same area. What is this area?
. Switch the order of integration and show that both orders yield the same area. What is this area?A)
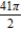
B)

C)
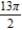
D)
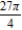
E)
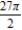

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
15
Find the center of mass of the rectangular lamina with vertices 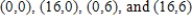 for the density
for the density 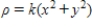 .
.
A) 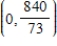
B) 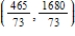
C) 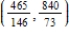
D) 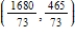
E) 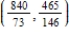
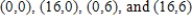 for the density
for the density 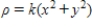 .
.A)
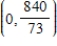
B)
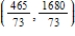
C)
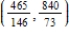
D)
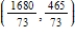
E)
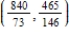

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
16
Find the mass and center of mass of the lamina bounded by the graphs of the equations given below for the given density. 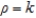
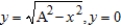
A)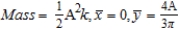
B)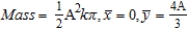
C)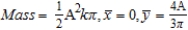
D)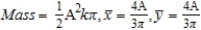
E)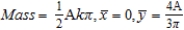
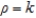
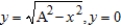
A)
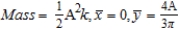
B)
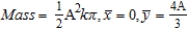
C)
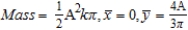
D)
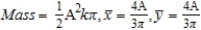
E)
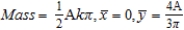

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
17
Rewrite the iterated integral 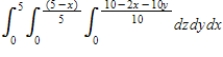 using the order dydxdz.
using the order dydxdz.
A) 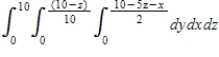
B) 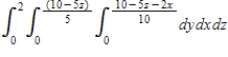
C) 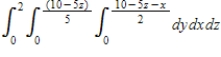
D) 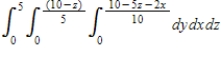
E) 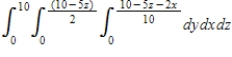
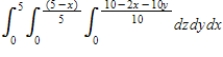 using the order dydxdz.
using the order dydxdz.
A)
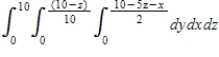
B)
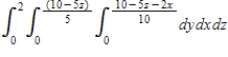
C)
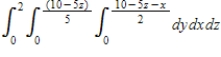
D)
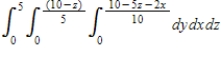
E)
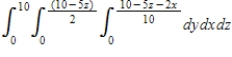

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
18
Use cylindrical coordinates to find the volume of the solid inside both 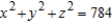 and
and 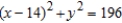 .
.
A)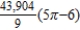
B)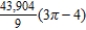
C)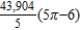
D)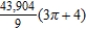
E)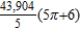
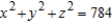 and
and 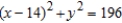 .
. A)
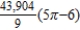
B)
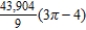
C)
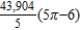
D)
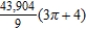
E)
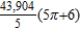

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
19
Set up the double integral required to find the moment of inertia 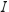 , about the line
, about the line 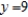 of the lamina bounded by the graphs of the equations
of the lamina bounded by the graphs of the equations 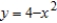 and
and 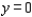 for the density
for the density 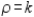 . Use a computer algebra system to evaluate the double integral.
. Use a computer algebra system to evaluate the double integral.
A)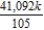
B)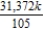
C)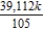
D)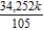
E)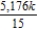
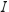 , about the line
, about the line 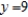 of the lamina bounded by the graphs of the equations
of the lamina bounded by the graphs of the equations 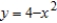 and
and 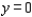 for the density
for the density 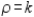 . Use a computer algebra system to evaluate the double integral.
. Use a computer algebra system to evaluate the double integral.A)
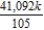
B)
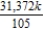
C)
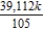
D)
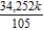
E)
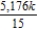

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
20
Use a double integral to find the volume of the indicated solid. 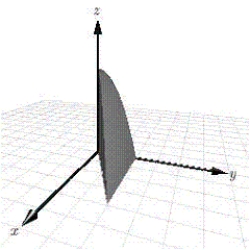
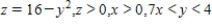
A)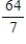
B)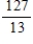
C)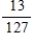
D)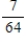
E) none of these

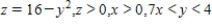
A)
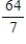
B)
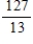
C)
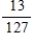
D)
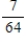
E) none of these

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
21
Evaluate the following iterated integral by converting to polar coordinates. 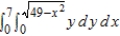
A)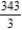
B)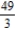
C)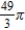
D)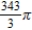
E)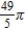
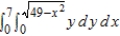
A)
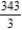
B)
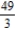
C)
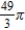
D)
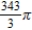
E)
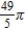

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
22
If 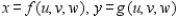 and
and 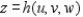 then the Jacobian of x, y, and z with respect to u, v, and w is
then the Jacobian of x, y, and z with respect to u, v, and w is 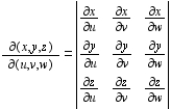 .
.
Find the Jacobian for the following change of variables:
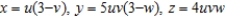
A)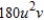
B)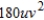
C)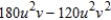
D)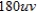
E)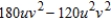
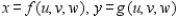 and
and 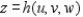 then the Jacobian of x, y, and z with respect to u, v, and w is
then the Jacobian of x, y, and z with respect to u, v, and w is 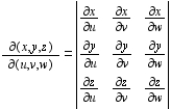 .
.
Find the Jacobian for the following change of variables:
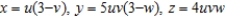
A)
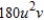
B)
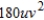
C)
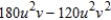
D)
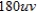
E)
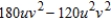

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
23
Use a change of variables to find the volume of the solid region lying below the surface 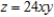 and above the plane region R: region bounded by the square with vertices
and above the plane region R: region bounded by the square with vertices 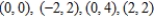 .
.
A)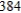
B)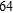
C)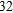
D)
E)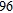
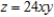 and above the plane region R: region bounded by the square with vertices
and above the plane region R: region bounded by the square with vertices 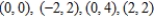 .
.A)
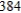
B)
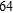
C)
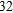
D)

E)
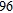

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
24
Find the area of the surface for the portion of the paraboloid 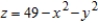 in the first octant.
in the first octant.
A)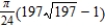
B)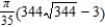
C)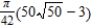
D)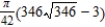
E)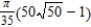
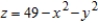 in the first octant.
in the first octant.A)
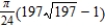
B)
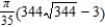
C)
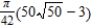
D)
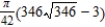
E)
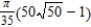

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
25
Use cylindrical coordinates to find the volume of the solid bounded above by 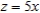 and below by
and below by 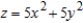 .
.
A)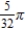
B)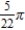
C)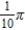
D)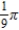
E)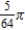
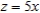 and below by
and below by 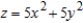 .
.A)
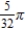
B)
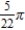
C)
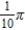
D)
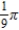
E)
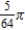

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
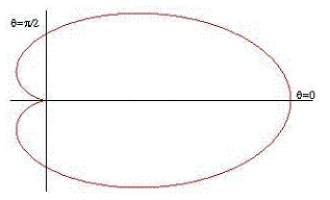
26
Use a double integral to find the area enclosed by the graph of 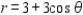 .
. 
A)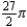
B)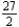
C)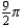
D)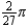
E)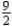
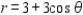 .
. 
A)
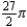
B)
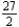
C)
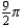
D)
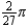
E)

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
27
Find the area of the surface for the portion of the sphere 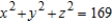 inside the cylinder
inside the cylinder 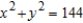 .
.
A)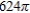
B)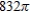
C)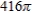
D)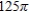
E)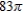
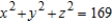 inside the cylinder
inside the cylinder 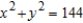 .
.A)
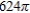
B)
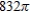
C)
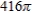
D)
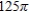
E)
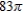

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
28
Use the indicated change of variables to evaluate the following double integral. 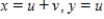
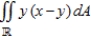
A)
B)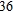
C)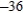
D)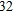
E)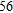
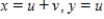
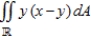

A)
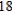
B)
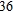
C)
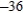
D)
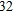
E)
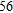

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
29
Evaluate the following integral. 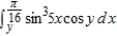
A)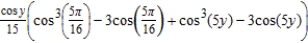
B)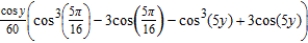
C)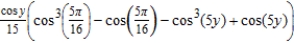
D)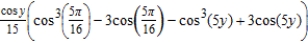
E)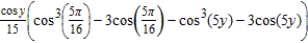
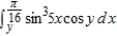
A)
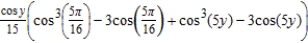
B)
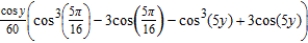
C)
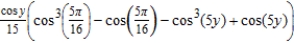
D)
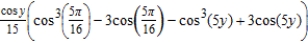
E)
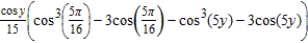

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
30
Evaluate the following iterated integral. 
A)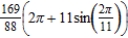
B)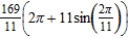
C)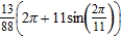
D)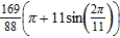
E)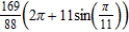

A)
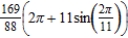
B)
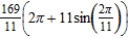
C)
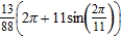
D)
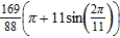
E)
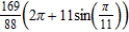

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
31
Find 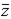 of the center of mass of the solid of given density
of the center of mass of the solid of given density 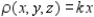 bounded by the graphs of the equations
bounded by the graphs of the equations 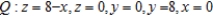 .
.
A)
B)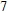
C)
D)
E)
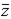 of the center of mass of the solid of given density
of the center of mass of the solid of given density 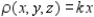 bounded by the graphs of the equations
bounded by the graphs of the equations 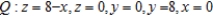 .
. A)

B)
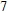
C)

D)

E)


Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
32
Evaluate the following iterated integral. 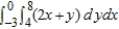
A) 43
B) 42
C) 36
D) 33
E) 38
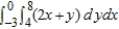
A) 43
B) 42
C) 36
D) 33
E) 38

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
33
Find the average value of 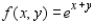 over the region R, where R is a triangle with vertices
over the region R, where R is a triangle with vertices 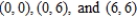 .
.
A)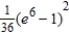
B)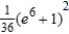
C)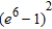
D)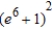
E)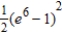
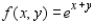 over the region R, where R is a triangle with vertices
over the region R, where R is a triangle with vertices 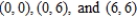 .
. A)
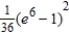
B)
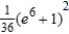
C)
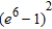
D)
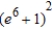
E)
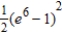

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
34
Use spherical coordinates to find the mass of the sphere 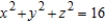 with the given density. The density at any point is proportional to the distance of the point from the z-axis.
with the given density. The density at any point is proportional to the distance of the point from the z-axis.
A)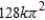
B)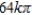
C)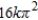
D)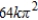
E)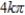
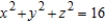 with the given density. The density at any point is proportional to the distance of the point from the z-axis.
with the given density. The density at any point is proportional to the distance of the point from the z-axis.A)
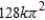
B)
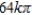
C)
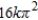
D)
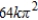
E)
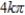

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
35
Evaluate the double integral below. 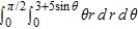
A)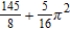
B)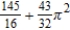
C)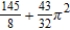
D)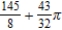
E)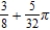
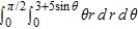
A)
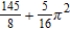
B)
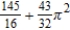
C)
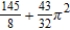
D)
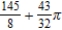
E)
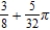

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
36
Set up and evaluate a double integral to find the volume of the solid bounded by the graphs of the equations given below. 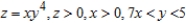
A)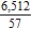
B)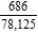
C)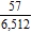
D)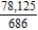
E)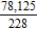
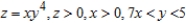
A)
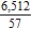
B)
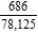
C)
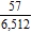
D)
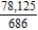
E)
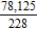

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
37
Find the area of the portion of the surface 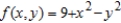 that lies above the region
that lies above the region 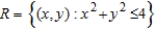 . Round your answer to two decimal places.
. Round your answer to two decimal places.
A) 29.20
B) 58.39
C) 439.36
D) 36.18
E) 1.64
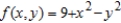 that lies above the region
that lies above the region 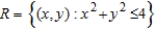 . Round your answer to two decimal places.
. Round your answer to two decimal places.A) 29.20
B) 58.39
C) 439.36
D) 36.18
E) 1.64

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
38
Evaluate the iterated integral 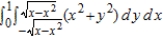 by converting to polar coordinates.
by converting to polar coordinates.
A)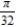
B)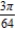
C)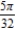
D)
E)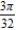
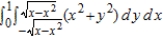 by converting to polar coordinates.
by converting to polar coordinates.A)
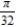
B)
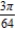
C)
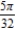
D)

E)
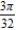

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
39
Evaluate the following improper integral. 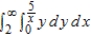
A)
B)
C)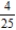
D)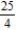
E) The integral does not converge.
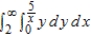
A)

B)

C)
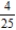
D)
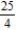
E) The integral does not converge.

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
40
Use cylindrical coordinates to find the volume of the solid inside the sphere 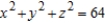 and above the upper nappe of the cone
and above the upper nappe of the cone 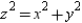 .
.
A)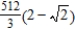
B)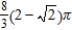
C)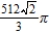
D)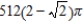
E)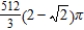
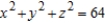 and above the upper nappe of the cone
and above the upper nappe of the cone 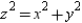 .
.A)
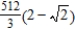
B)
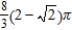
C)
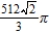
D)
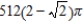
E)
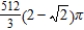

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
41
Evaluate the following iterated integral. 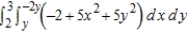
A) -514
B) -499
C) -473
D) -463
E) -399
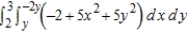
A) -514
B) -499
C) -473
D) -463
E) -399

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
42
Evaluate 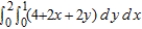 .
.
A) 6
B) 14
C) 12
D) 18
E) 8
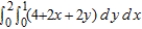 .
.A) 6
B) 14
C) 12
D) 18
E) 8

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
43
Find the center of mass of the lamina bounded by the graphs of the equations 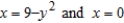 for the density
for the density 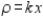 .
.
A)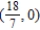
B)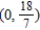
C)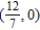
D)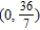
E)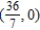
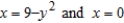 for the density
for the density 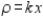 .
. A)
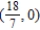
B)
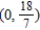
C)
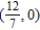
D)
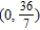
E)
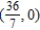

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
44
Evaluate the following iterated integral. 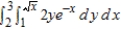
A)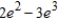
B)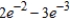
C)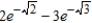
D)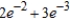
E)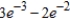
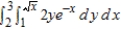
A)
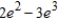
B)
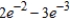
C)
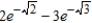
D)
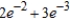
E)
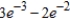

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
45
Use a double integral to find the volume of the indicated solid. 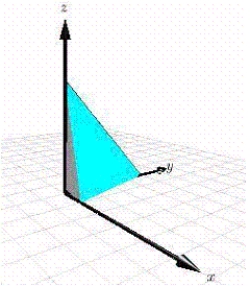
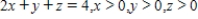
A)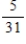
B)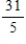
C)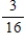
D)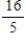
E)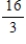

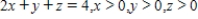
A)
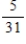
B)
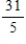
C)
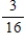
D)
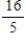
E)
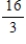

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
46
Set up and evaluate a double integral required to find the moment of inertia, I, about the given line, of the lamina bounded by the graphs of the following equations. Use a computer algebra system to evaluate the double integral. 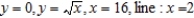

A)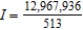
B)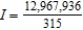
C)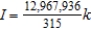
D)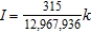
E)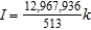
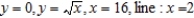

A)
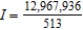
B)
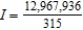
C)
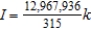
D)
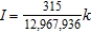
E)
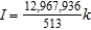

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
47
Use an iterated integral to find the area of the region bounded by 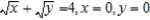 .
.
A)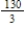
B)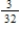
C)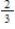
D)
E) The integral is improper and does not converge.
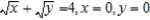 .
.A)
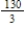
B)
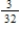
C)
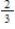
D)

E) The integral is improper and does not converge.

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
48
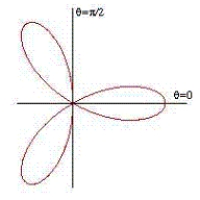
Use a double integral to find the area enclosed by the graph of  .
. 
A)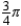
B)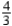
C)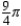
D)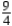
E)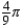
 .
. 
A)
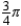
B)
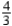
C)
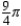
D)
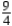
E)
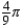

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
49
Evaluate the double integral below. 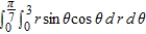
A)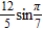
B)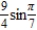
C)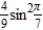
D)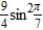
E)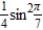
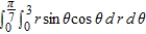
A)
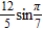
B)
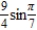
C)
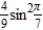
D)
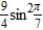
E)
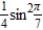

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
50
The area of a region R is given by the iterated integral 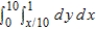 . Switch the order of integration and show that both orders yield the same area. What is this area?
. Switch the order of integration and show that both orders yield the same area. What is this area?
A) 101
B) 20
C) 30
D) 10
E) 5
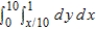 . Switch the order of integration and show that both orders yield the same area. What is this area?
. Switch the order of integration and show that both orders yield the same area. What is this area?A) 101
B) 20
C) 30
D) 10
E) 5

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
51
Evaluate the double integral below. 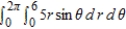
A)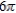
B) 0
C)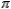
D)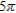
E)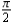
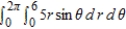
A)
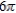
B) 0
C)
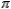
D)
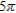
E)
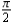

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
52
Use spherical coordinates to find the volume of the solid inside the torus given by 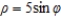 .
.
A)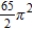
B)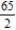
C)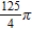
D)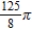
E)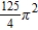
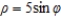 .
.A)
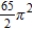
B)
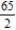
C)
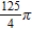
D)
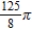
E)
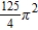

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
53
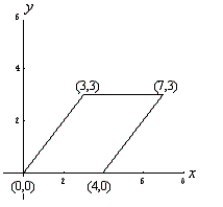
Use a change of variables to find the volume of the solid region lying below the surface 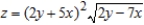 and above the plane region R: region bounded by the parallelogram with vertices
and above the plane region R: region bounded by the parallelogram with vertices 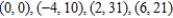 . Round your answer to two decimal places.
. Round your answer to two decimal places.
A)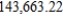
B)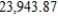
C)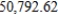
D)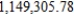
E)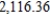
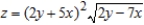 and above the plane region R: region bounded by the parallelogram with vertices
and above the plane region R: region bounded by the parallelogram with vertices 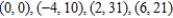 . Round your answer to two decimal places.
. Round your answer to two decimal places.A)
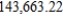
B)
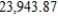
C)
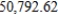
D)
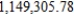
E)
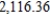

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
54
Evaluate the iterated integral below. Note that it is necessary to switch the order of integration. 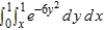
A)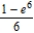
B)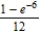
C)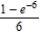
D)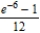
E)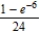
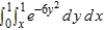
A)
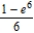
B)
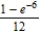
C)
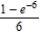
D)
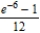
E)
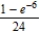

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
55
Use a double integral to find the area of the region inside the circle 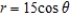 and outside the cardioid
and outside the cardioid 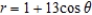 . Round your answer to two decimal places.
. Round your answer to two decimal places.
A) 44.76
B) 44.88
C) 17.88
D) 54.88
E) 21.88
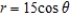 and outside the cardioid
and outside the cardioid 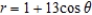 . Round your answer to two decimal places.
. Round your answer to two decimal places.A) 44.76
B) 44.88
C) 17.88
D) 54.88
E) 21.88

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
56
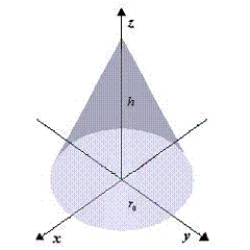
Use cylindrical coordinates to find the volume of the cone 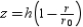 where
where 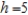 and
and 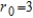 .
. 
A)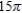
B)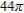
C)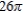
D)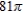
E)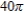
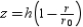 where
where 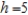 and
and 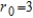 .
. 
A)
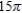
B)
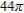
C)
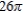
D)
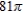
E)
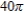

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
57
Use a double integral to find the area of the shaded region as shown in the figure below. 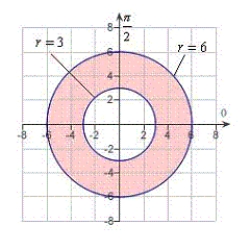
A)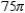
B)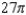
C)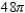
D)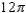
E)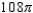

A)
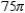
B)
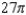
C)
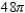
D)
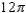
E)
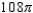

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
58
Determine the diameter of a hole that is drilled vertically through the center of the solid bounded by the graphs of the equations 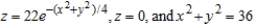 if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.
if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.
A) 1.2983
B) 36.5966
C) 3.2983
D) 5.5966
E) 37.2983
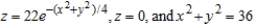 if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.
if one-tenth of the volume of the solid is removed. Round your answer to four decimal places.A) 1.2983
B) 36.5966
C) 3.2983
D) 5.5966
E) 37.2983

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
59
Find the mass of the lamina bounded by the graphs of the equations 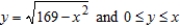 for the density
for the density 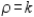 .
.
A)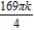
B)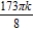
C)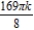
D)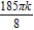
E)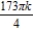
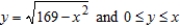 for the density
for the density 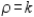 .
. A)
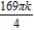
B)
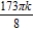
C)
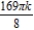
D)
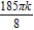
E)
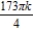

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
60
Find the mass of the triangular lamina with vertices 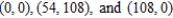 for the density
for the density 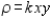 .
.
A) 11,337,408k
B) 22,674,826k
C) 11,337,398k
D) 11,337,413k
E) 22,674,816k
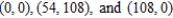 for the density
for the density 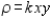 .
. A) 11,337,408k
B) 22,674,826k
C) 11,337,398k
D) 11,337,413k
E) 22,674,816k

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
61
Find the area of the portion of the surface 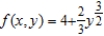 that lies above the region
that lies above the region 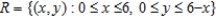 . Round your answer to two decimal places.
. Round your answer to two decimal places.
A) 0.67
B) 3.87
C) 30.30
D) 10.64
E) 30.84
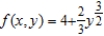 that lies above the region
that lies above the region 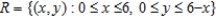 . Round your answer to two decimal places.
. Round your answer to two decimal places.A) 0.67
B) 3.87
C) 30.30
D) 10.64
E) 30.84

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
62
Evaluate the iterated integral below. Note that it is necessary to switch the order of integration. 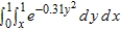
A)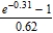
B)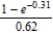
C)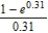
D)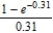
E)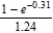
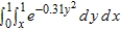
A)
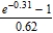
B)
C)
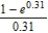
D)
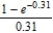
E)
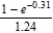

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
63
Sketch the region R of integration and then switch the order of integration for the following integral. 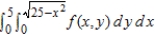
A)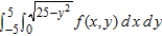
B)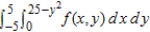
C)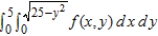
D)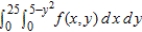
E)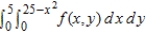
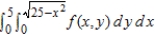
A)
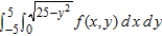
B)
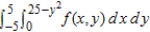
C)
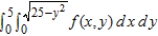
D)
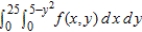
E)
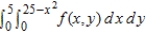

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
64
Find the area of the surface given by 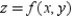 over the region R.
over the region R. 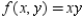
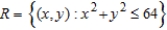
A)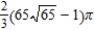
B)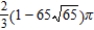
C)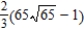
D)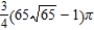
E)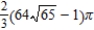
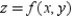 over the region R.
over the region R. 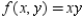
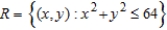
A)
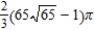
B)
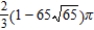
C)
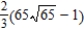
D)
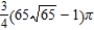
E)
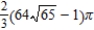

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
65
Find the Jacobian 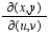 for the following change of variables:
for the following change of variables: 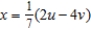 ,
, 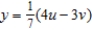
A)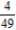
B)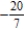
C)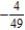
D)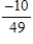
E)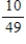
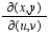 for the following change of variables:
for the following change of variables: 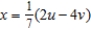 ,
, 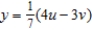
A)
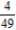
B)
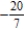
C)
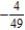
D)
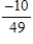
E)
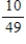

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
66
The area of a region R is given by the iterated integrals 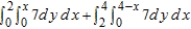 . Switch the order of integration and show that both orders yield the same area. What is this area?
. Switch the order of integration and show that both orders yield the same area. What is this area?
A) 14
B) 197
C) 28
D) 150
E) 27
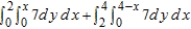 . Switch the order of integration and show that both orders yield the same area. What is this area?
. Switch the order of integration and show that both orders yield the same area. What is this area?A) 14
B) 197
C) 28
D) 150
E) 27

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
67
Use an iterated integral to find the area of the region bounded by 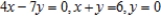 .
.
A)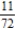
B)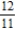
C)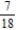
D)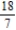
E)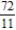
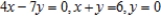 .
.A)
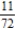
B)
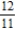
C)
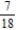
D)
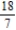
E)
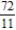

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
68
Set up a double integral that gives the area of the surface on the graph of  over the region
over the region 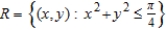 .
.
A)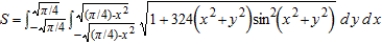
B)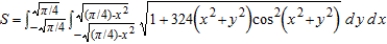
C)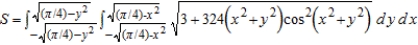
D)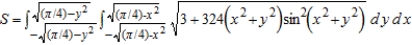
E)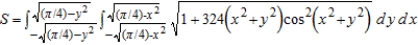
 over the region
over the region 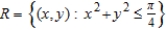 .
.A)
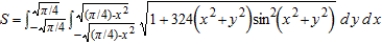
B)
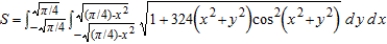
C)
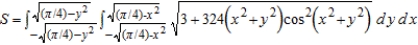
D)
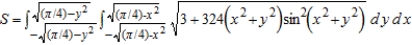
E)
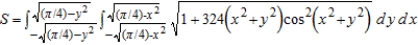

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
69
Evaluate the integral 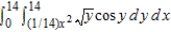 by switching the order of integration. Round your answer to two decimal places.
by switching the order of integration. Round your answer to two decimal places.
A) 5.16
B) 48.66
C) 15.38
D) 13.38
E) 56.14
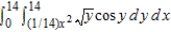 by switching the order of integration. Round your answer to two decimal places.
by switching the order of integration. Round your answer to two decimal places.A) 5.16
B) 48.66
C) 15.38
D) 13.38
E) 56.14

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
70
Set up and evaluate a double integral to find the volume of the solid bounded by the graphs of the equations 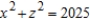 and
and 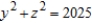 in the first octant.
in the first octant.
A) 273,375
B) 30,375
C) 182,250
D) 91,125
E) 60,750
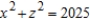 and
and 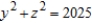 in the first octant.
in the first octant.A) 273,375
B) 30,375
C) 182,250
D) 91,125
E) 60,750

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
71
Find the area of the surface of the portion of the plane 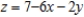 in the first octant.
in the first octant.
A)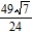
B)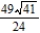
C)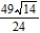
D)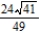
E)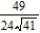
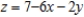 in the first octant.
in the first octant.A)
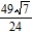
B)
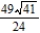
C)
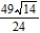
D)
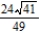
E)
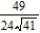

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
72
Use a double integral to find the volume of the indicated solid. 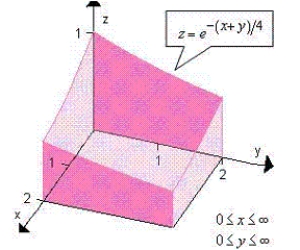
A) 16
B) 9
C) 4
D) 20
E) 6

A) 16
B) 9
C) 4
D) 20
E) 6

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
73
Evaluate the following integral. 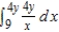
A)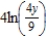
B)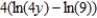
C)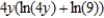
D)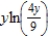
E)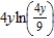
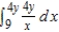
A)
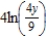
B)
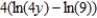
C)
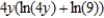
D)
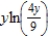
E)
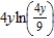

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
74
Set up a triple integral for the volume of the solid bounded by the coordinate planes and the plane given below. 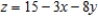
A)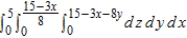
B)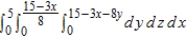
C)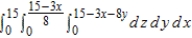
D)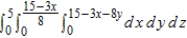
E)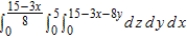
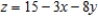
A)
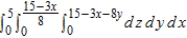
B)
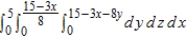
C)
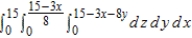
D)
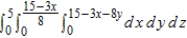
E)
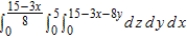

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
75
Use cylindrical coordinates to find the mass of the solid 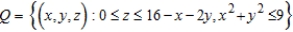 where
where 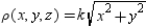 .
.
A)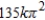
B)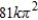
C)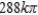
D)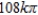
E)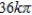
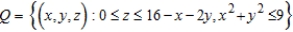 where
where 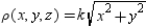 .
.A)
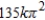
B)
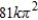
C)
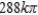
D)
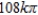
E)
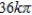

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
76
Consider the region  in the xy-plane bounded by the ellipse
in the xy-plane bounded by the ellipse 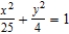 and the transformation
and the transformation 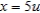 and
and 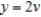 . Find
. Find 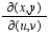 .
.
A)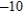
B) 0
C)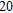
D)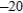
E)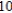
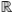 in the xy-plane bounded by the ellipse
in the xy-plane bounded by the ellipse 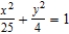 and the transformation
and the transformation 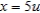 and
and 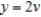 . Find
. Find 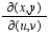 .
.A)
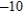
B) 0
C)
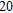
D)
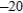
E)
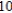

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
77
Use an iterated integral to find the area of the region bounded by the graphs of the equations 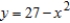 and
and 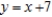 .
.
A)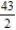
B)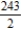
C)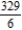
D)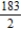
E)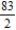
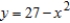 and
and 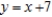 .
.A)
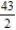
B)
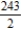
C)
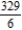
D)
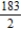
E)
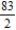

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
78
Use polar coordinates to describe the region as shown in the figure below: 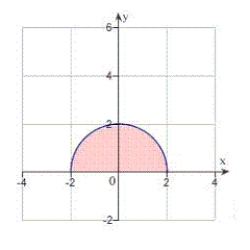
A)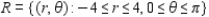
B)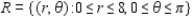
C)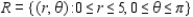
D)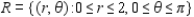
E)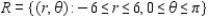

A)
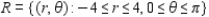
B)
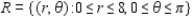
C)
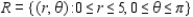
D)
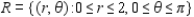
E)
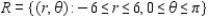

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
79
Find the area of the portion of the surface 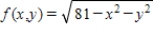 that lies above the region
that lies above the region 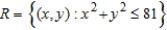 . Round your answer to two decimal places.
. Round your answer to two decimal places.
A) 81.00
B) 88.83
C) 508.94
D) 254.47
E) 799.44
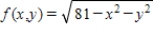 that lies above the region
that lies above the region 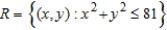 . Round your answer to two decimal places.
. Round your answer to two decimal places. A) 81.00
B) 88.83
C) 508.94
D) 254.47
E) 799.44

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck
80
Find the Jacobian for the change of variables given below. 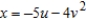 ,
, 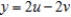
A)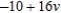
B)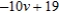
C)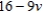
D)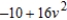
E)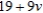
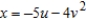 ,
, 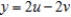
A)
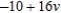
B)
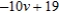
C)
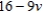
D)
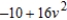
E)
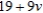

Unlock Deck
Unlock for access to all 143 flashcards in this deck.
Unlock Deck
k this deck



