Deck 10: Basic Regression Analysis With Time Series Data
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/27
Play
Full screen (f)
Deck 10: Basic Regression Analysis With Time Series Data
1
A static model is postulated when:
A)a change in the independent variable at time 't' is believed to have an effect on the dependent variable at period 't + 1'.
B)a change in the independent variable at time 't' is believed to have an effect on the dependent variable for all successive time periods.
C)a change in the independent variable at time 't' does not have any effect on the dependent variable.
D)a change in the independent variable at time 't' is believed to have an immediate effect on the dependent variable.
A)a change in the independent variable at time 't' is believed to have an effect on the dependent variable at period 't + 1'.
B)a change in the independent variable at time 't' is believed to have an effect on the dependent variable for all successive time periods.
C)a change in the independent variable at time 't' does not have any effect on the dependent variable.
D)a change in the independent variable at time 't' is believed to have an immediate effect on the dependent variable.
D
2
The model: Yt = 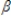 0 +
0 + 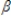 1ct + ut, t = 1,2,……., n is an example of a(n):
1ct + ut, t = 1,2,……., n is an example of a(n):
A)autoregressive conditional heteroskedasticity model.
B)static model.
C)finite distributed lag model.
D)infinite distributed lag model.
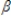 0 +
0 + 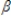 1ct + ut, t = 1,2,……., n is an example of a(n):
1ct + ut, t = 1,2,……., n is an example of a(n):A)autoregressive conditional heteroskedasticity model.
B)static model.
C)finite distributed lag model.
D)infinite distributed lag model.
B
3
If 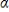 1 > 0, then yt in the linear function of time E(yt) =
1 > 0, then yt in the linear function of time E(yt) = 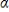 0 +
0 + 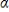 1t displays a(n):
1t displays a(n):
A)upward trend.
B)downward trend.
C)exponential trend.
D)quadratic trend.
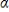 1 > 0, then yt in the linear function of time E(yt) =
1 > 0, then yt in the linear function of time E(yt) = 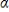 0 +
0 + 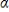 1t displays a(n):
1t displays a(n):A)upward trend.
B)downward trend.
C)exponential trend.
D)quadratic trend.
A
4
Time series regression is based on series which exhibit serial correlation.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
5
Refer to the following model yt =  0 +
0 + 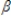 0st +
0st + 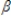 1st-1 +
1st-1 + 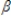 2st-2 +
2st-2 + 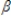 3st-3 + ut
3st-3 + ut
This is an example of a(n):
A)infinite distributed lag model.
B)finite distributed lag model of order 1.
C)finite distributed lag model of order 2.
D)finite distributed lag model of order 3.
 0 +
0 + 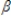 0st +
0st + 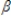 1st-1 +
1st-1 + 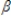 2st-2 +
2st-2 + 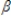 3st-3 + ut
3st-3 + utThis is an example of a(n):
A)infinite distributed lag model.
B)finite distributed lag model of order 1.
C)finite distributed lag model of order 2.
D)finite distributed lag model of order 3.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
6
Which of the following rules out perfect collinearity among the regressors?
A)Multiple regression
B)Simple regression
C)Time series regression
D)Cross-sectional regression
A)Multiple regression
B)Simple regression
C)Time series regression
D)Cross-sectional regression

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
7
Which of the following is an assumption on which time series regression is based?
A)A time series process follows a model that is nonlinear in parameters.
B)In a time series process, no independent variable is a perfect linear combination of the others.
C)In a time series process, at least one independent variable is a constant.
D)For each time period, the expected value of the error ut, given the explanatory variables for all time periods, is positive.
A)A time series process follows a model that is nonlinear in parameters.
B)In a time series process, no independent variable is a perfect linear combination of the others.
C)In a time series process, at least one independent variable is a constant.
D)For each time period, the expected value of the error ut, given the explanatory variables for all time periods, is positive.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
8
Which of the following statements is true?
A)The average of an exponential time series is a linear function of time.
B)The average of a linear sequence is an exponential function of time.
C)When a series has the same average growth rate from period to period, it can be approximated with an exponential trend.
D)When a series has the same average growth rate from period to period, it can be approximated with a linear trend.
A)The average of an exponential time series is a linear function of time.
B)The average of a linear sequence is an exponential function of time.
C)When a series has the same average growth rate from period to period, it can be approximated with an exponential trend.
D)When a series has the same average growth rate from period to period, it can be approximated with a linear trend.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
9
The sample size for a time series data set is the number of:
A)variables being measured.
B)time periods over which we observe the variables of interest less the number of variables being measured.
C)time periods over which we observe the variables of interest plus the number of variables being measured.
D)time periods over which we observe the variables of interest.
A)variables being measured.
B)time periods over which we observe the variables of interest less the number of variables being measured.
C)time periods over which we observe the variables of interest plus the number of variables being measured.
D)time periods over which we observe the variables of interest.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
10
Economic time series are outcomes of random variables.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
11
Refer to the following model. yt =  0 +
0 + 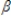 0st +
0st + 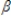 1st-1 +
1st-1 + 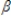 2st-2 +
2st-2 + 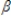 3st-3 + ut
3st-3 + ut 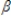 0 +
0 + 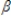 1 +
1 + 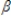 2 +
2 + 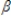 3 represents:
3 represents:
A)the short-run change in y given a temporary increase in s.
B)the short-run change in y given a permanent increase in s.
C)the long-run change in y given a permanent increase in s.
D)the long-run change in y given a temporary increase in s.
 0 +
0 + 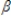 0st +
0st + 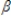 1st-1 +
1st-1 + 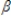 2st-2 +
2st-2 + 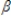 3st-3 + ut
3st-3 + ut 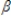 0 +
0 + 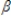 1 +
1 + 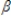 2 +
2 + 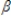 3 represents:
3 represents:A)the short-run change in y given a temporary increase in s.
B)the short-run change in y given a permanent increase in s.
C)the long-run change in y given a permanent increase in s.
D)the long-run change in y given a temporary increase in s.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
12
With base year 1990, the index of industrial production for the year 1999 is 112. What will be the value of the index in 1999, if the base year is changed to 1982 and the index measured 96 in 1982?
A)112.24
B)116.66
C)85.71
D)92.09
A)112.24
B)116.66
C)85.71
D)92.09

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
13
The propensity δ0 + δ1+ … + δk is sometimes called the:
A)short-run elasticity, which measures the percentage increase in a dependent variable after k quarters given a permanent 1% increase in the k independent variables.
B)long-run elasticity, which measures the percentage increase in a dependent variable after k quarters given a permanent 1% increase in the k independent variables.
C)short-run elasticity, which measures the percentage decrease in a dependent variable after k quarters given a permanent 1% decrease in the k independent variables.
D)long-run elasticity, which measures the percentage decrease in a dependent variable after k quarters given a permanent 1% decrease in the k independent variables.
A)short-run elasticity, which measures the percentage increase in a dependent variable after k quarters given a permanent 1% increase in the k independent variables.
B)long-run elasticity, which measures the percentage increase in a dependent variable after k quarters given a permanent 1% increase in the k independent variables.
C)short-run elasticity, which measures the percentage decrease in a dependent variable after k quarters given a permanent 1% decrease in the k independent variables.
D)long-run elasticity, which measures the percentage decrease in a dependent variable after k quarters given a permanent 1% decrease in the k independent variables.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
14
In a static model, one or more explanatory variables affect the dependent variable with a lag.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
15
A study which observes whether a particular occurrence influences some outcome is referred to as a(n):
A)event study.
B)exponential study.
C)laboratory study.
D)comparative study.
A)event study.
B)exponential study.
C)laboratory study.
D)comparative study.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
16
Adding a time trend can make an explanatory variable more significant if:
A)the dependent and independent variables have similar kinds of trends, but movement in the independent variable about its trend line causes movement in the dependent variable away from its trend line.
B)the dependent and independent variables have similar kinds of trends and movement in the independent variable about its trend line causes movement in the dependent variable towards its trend line.
C)the dependent and independent variables have different kinds of trends and movement in the independent variable about its trend line causes movement in the dependent variable towards its trend line.
D)the dependent and independent variables have different kinds of trends, but movement in the independent variable about its trend line causes movement in the dependent variable away from its trend line.
A)the dependent and independent variables have similar kinds of trends, but movement in the independent variable about its trend line causes movement in the dependent variable away from its trend line.
B)the dependent and independent variables have similar kinds of trends and movement in the independent variable about its trend line causes movement in the dependent variable towards its trend line.
C)the dependent and independent variables have different kinds of trends and movement in the independent variable about its trend line causes movement in the dependent variable towards its trend line.
D)the dependent and independent variables have different kinds of trends, but movement in the independent variable about its trend line causes movement in the dependent variable away from its trend line.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
17
A seasonally adjusted series is one which:
A)has had seasonal factors added to it.
B)has seasonal factors removed from it.
C)has qualitative dependent variables representing different seasons.
D)has qualitative explanatory variables representing different seasons.
A)has had seasonal factors added to it.
B)has seasonal factors removed from it.
C)has qualitative dependent variables representing different seasons.
D)has qualitative explanatory variables representing different seasons.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
18
Which of the following correctly identifies a difference between cross-sectional data and time series data?
A)Cross-sectional data is based on temporal ordering, whereas time series data is not.
B)Time series data is based on temporal ordering, whereas cross-sectional data is not.
C)Cross-sectional data consists of only qualitative variables, whereas time series data consists of only quantitative variables.
D)Time series data consists of only qualitative variables, whereas cross-sectional data does not include qualitative variables.
A)Cross-sectional data is based on temporal ordering, whereas time series data is not.
B)Time series data is based on temporal ordering, whereas cross-sectional data is not.
C)Cross-sectional data consists of only qualitative variables, whereas time series data consists of only quantitative variables.
D)Time series data consists of only qualitative variables, whereas cross-sectional data does not include qualitative variables.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
19
A stochastic process refers to a:
A)sequence of random variables indexed by time.
B)sequence of variables that can take fixed qualitative values.
C)sequence of random variables that can take binary values only.
D)sequence of random variables estimated at the same point of time.
A)sequence of random variables indexed by time.
B)sequence of variables that can take fixed qualitative values.
C)sequence of random variables that can take binary values only.
D)sequence of random variables estimated at the same point of time.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
20
If an explanatory variable is strictly exogenous it implies that:
A)changes in the lag of the variable does not affect future values of the dependent variable.
B)the variable is correlated with the error term in all future time periods.
C)the variable cannot react to what has happened to the dependent variable in the past.
D)the conditional mean of the error term given the variable is zero.
A)changes in the lag of the variable does not affect future values of the dependent variable.
B)the variable is correlated with the error term in all future time periods.
C)the variable cannot react to what has happened to the dependent variable in the past.
D)the conditional mean of the error term given the variable is zero.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
21
Supposed that you are interested in estimating country-level maternal mortality rate (mmrt) based just on the gross domestic product per capita (gdppct) and literacy rate (lrt) and you find that countries that have unusually high (for the given levels of gdppc and lr) mmr in one period also have unusually high mmr in the next period. Which of the following assumption for time series analysis does not hold?
A)No perfect collinearity.
B)Zero conditional mean.
C)Homoskedasticity.
D)No serial correlation.
A)No perfect collinearity.
B)Zero conditional mean.
C)Homoskedasticity.
D)No serial correlation.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
22
Refer to the following model
yt =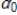 +
+ 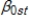 +
+ 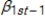 +
+ 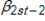 +
+ 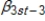 + ut.
+ ut.
Given a permanent increase in s,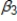 is the long-run propensity.
is the long-run propensity.
yt =
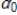 +
+ 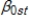 +
+ 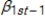 +
+ 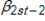 +
+ 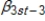 + ut.
+ ut.Given a permanent increase in s,
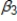 is the long-run propensity.
is the long-run propensity.
Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
23
Price indexes are necessary for turning a time series measured in real value into nominal value.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
24
Consider the following equation: Log(yt )= 0.7 + 1.2log(st ) + 0.3log(st-1) + 0.2log(st-2) + 0.1log(st-3)
What is the percentage increase in y given a permanent 1% increase in s?
A)1.2
B)1.8
C)2.5
D)0.5
What is the percentage increase in y given a permanent 1% increase in s?
A)1.2
B)1.8
C)2.5
D)0.5

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
25
Dummy variables can be used to address the problem of seasonality in regression models.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
26
The short-run elasticity measures the immediate percentage change in a dependent variable given a 1% increase in the independent variables.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck
27
When a series has the same average growth rate from period to period, then it can be approximated by an exponential trend.

Unlock Deck
Unlock for access to all 27 flashcards in this deck.
Unlock Deck
k this deck



