Deck 9: Mathematical Modeling Using Differential Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/121
Play
Full screen (f)
Deck 9: Mathematical Modeling Using Differential Equations
1
Which of the following graphs best describes the temperature in a large North American city over the course of a year? 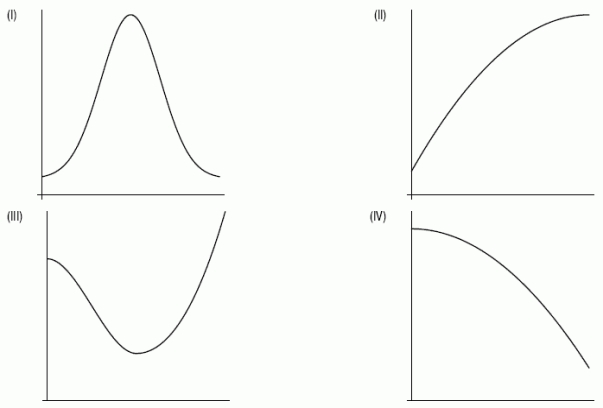

I
2
A quantity y satisfies the differential equation 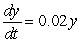 . Thus, y is decreasing when y is ________(less/greater) than _____.
. Thus, y is decreasing when y is ________(less/greater) than _____.
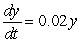 . Thus, y is decreasing when y is ________(less/greater) than _____.
. Thus, y is decreasing when y is ________(less/greater) than _____.Part A: less
Part B: 0
Part B: 0
3
A person withdraws money from a trust fund at a rate of $12,000 per year, and the account is earning interest at a rate of 5% per year, compounded continuously. Write a differential equation for the balance, B, in the account as a function of time, t, in years and use it to calculate 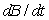 if B=$100,000.
if B=$100,000.
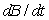 if B=$100,000.
if B=$100,000.dB / dt = -$7000
4
A cup of green tea contains 32 mg of caffeine when you are using the tea leaves for the first time. A cup from the second brew contains 12 mg of caffeine, while a cup from the third brew contains only 4 mg of caffeine. Caffeine leaves the body at a continuous rate of about 17% per hour.
a) Write a differential equation for the amount, C, of caffeine in the body at time t hours after drinking the green tea.
b) Use the differential equation to find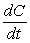 at the start of the first hour (right after drinking the tea) for a cup from the first brew, and use your answer to estimate the change in caffeine in the body during the first hour.
at the start of the first hour (right after drinking the tea) for a cup from the first brew, and use your answer to estimate the change in caffeine in the body during the first hour.
c) Does the initial amount of caffeine in the body (whether from the first, second or third brew) change the differential equation?
a) Write a differential equation for the amount, C, of caffeine in the body at time t hours after drinking the green tea.
b) Use the differential equation to find
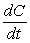 at the start of the first hour (right after drinking the tea) for a cup from the first brew, and use your answer to estimate the change in caffeine in the body during the first hour.
at the start of the first hour (right after drinking the tea) for a cup from the first brew, and use your answer to estimate the change in caffeine in the body during the first hour.c) Does the initial amount of caffeine in the body (whether from the first, second or third brew) change the differential equation?

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
5
A quantity Q satisfies the differential equation 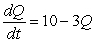 . Is Q increasing or decreasing at Q = 3?
. Is Q increasing or decreasing at Q = 3?
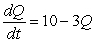 . Is Q increasing or decreasing at Q = 3?
. Is Q increasing or decreasing at Q = 3?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
6
A population of birds introduced onto an island without predators grows at a rate proportional to the size of the population. Write a differential equation for the size of the population, P, as a function of time. Is the constant of proportionality positive or negative?
A)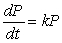 , positive
, positive
B)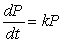 , negative
, negative
C)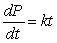 , negative
, negative
D)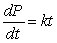 , positive
, positive
A)
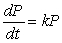 , positive
, positiveB)
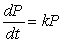 , negative
, negativeC)
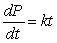 , negative
, negativeD)
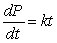 , positive
, positive
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
7
A quantity T satisfies the differential equation 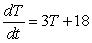 .
.
a) Is T increasing or decreasing when T = -5?
b) For what value of T is the rate of change of T equal to zero?
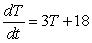 .
.a) Is T increasing or decreasing when T = -5?
b) For what value of T is the rate of change of T equal to zero?

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
8
A spherical raindrop evaporates at a rate proportional to its surface area. If V = volume of the raindrop and S = surface area, which of the following is a differential equation for 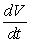 ?
?
A)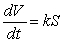 with k a negative constant
with k a negative constant
B)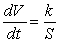 with k a negative constant
with k a negative constant
C)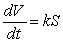 with k a positive constant
with k a positive constant
D)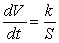 with k a positive constant
with k a positive constant
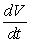 ?
?A)
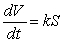 with k a negative constant
with k a negative constantB)
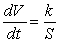 with k a negative constant
with k a negative constantC)
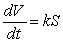 with k a positive constant
with k a positive constantD)
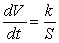 with k a positive constant
with k a positive constant
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
9
Which one(s) of the following are solutions to the differential equation 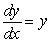 ?
?
A)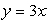
B)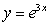
C)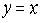
D)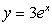
E)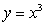
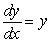 ?
?A)
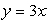
B)
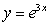
C)
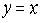
D)
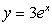
E)
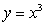

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
10
Carbon-14 decays at a rate proportional to the amount present. Which of the following is the differential equation for the amount, C, of carbon-14 present at time t?
A) , with k positive
, with k positive
B)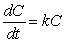 , with k negative
, with k negative
C)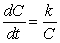 , with k positive
, with k positive
D)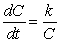 , with k negative
, with k negative
A)
 , with k positive
, with k positiveB)
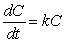 , with k negative
, with k negativeC)
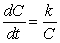 , with k positive
, with k positiveD)
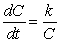 , with k negative
, with k negative
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
11
A bank account initially containing $4000 earns interest at a continuous rate of 6% per year. Deposits are made into the account at a constant rate of $500 per year. Which is the differential equation for the balance, B, in the account as a function of time, t, in years?
A)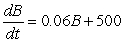
B)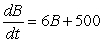
C)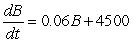
D)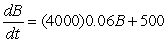
A)
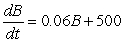
B)
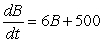
C)
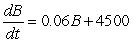
D)
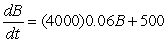

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
12
A population of rodents grows at a rate proportional to the size of the population. Which of the following is the the differential equation for the size of the population, P, as a function of time, t?
A)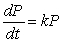 , with k positive
, with k positive
B)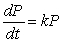 , with k negative
, with k negative
C)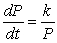 , with k positive
, with k positive
D)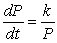 , with k negative
, with k negative
A)
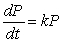 , with k positive
, with k positiveB)
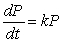 , with k negative
, with k negativeC)
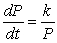 , with k positive
, with k positiveD)
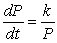 , with k negative
, with k negative
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
13
A drug is administered intravenously to a patient at a rate of 12 mg per day. About 40% of the drug in the patient's body is metabolized and leaves the body each day. Which is the differential equation for the amount of the drug, D, in the body as a function of time, t, in days?
A)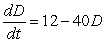
B)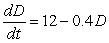
C)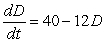
D)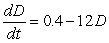
A)
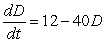
B)
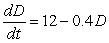
C)
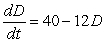
D)
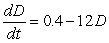

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
14
There is a theory that says the rate at which information spreads by word of mouth is proportional to the product of the number of people who have heard the information and the number who have not. Suppose the total population is N. Which of the following differential equations describe the rate, 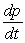 , at which the information spreads by word of mouth?
, at which the information spreads by word of mouth?
A)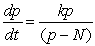
B)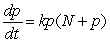
C)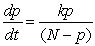
D)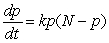
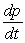 , at which the information spreads by word of mouth?
, at which the information spreads by word of mouth?A)
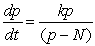
B)
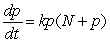
C)
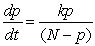
D)
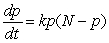

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
15
Water runs down a certain type of drainpipe at a rate proportional to the amount of water on the roof after a rainfall. Write a differential equation for the amount of water, W, on the roof at time t minutes after the rain stops.
A)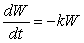
B)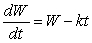
C)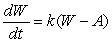

D)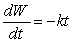
A)
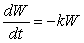
B)
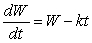
C)
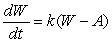

D)
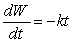

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
16
Water is being pumped into a pool at a rate of 150 gallons per day, and is evaporating at a rate of 0.2% per day. Which is the differential equation for the amount, A, of gallons of water in the pool as a function of time, t, in days?
A)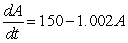
B)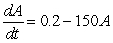
C)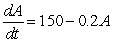
D)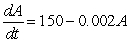
A)
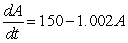
B)
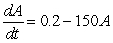
C)
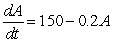
D)
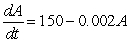

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
17
A quantity Q satisfies the differential equation 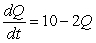 . For what value of Q is the rate of change equal to 0?
. For what value of Q is the rate of change equal to 0?
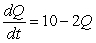 . For what value of Q is the rate of change equal to 0?
. For what value of Q is the rate of change equal to 0?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
18
Which of the following graphs best describes the population of a species that is introduced to a confined space?
I.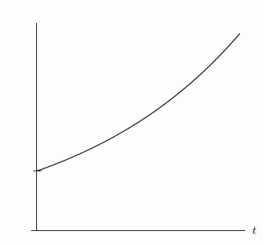 II.
II. 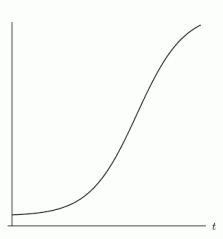 III.
III. 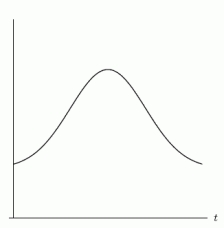 IV.
IV. 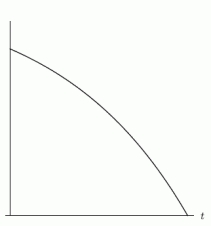
I.
 II.
II.  III.
III.  IV.
IV. 

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
19
Consider the differential equation for the logistic model representing a population of tarantulas introduced into a new habitat: 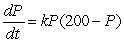 . What is the carrying capacity?
. What is the carrying capacity?
A)200 tarantulas
B)k tarantulas
C)200 - P tarantulas
D)1000 tarantulas
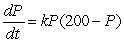 . What is the carrying capacity?
. What is the carrying capacity?A)200 tarantulas
B)k tarantulas
C)200 - P tarantulas
D)1000 tarantulas

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
20
The deer population, P, in an area is increasing at a rate of 25% per year due to breeding. At the same time, about 200 deer are shot by hunters each year. Which is the differential equation for the population of deer as a function of time t, in years?
A)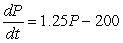
B)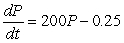
C)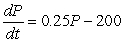
D)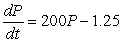
A)
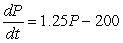
B)
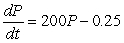
C)
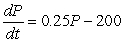
D)
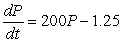

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
21
What is the solution of 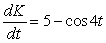 when
when 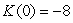 ?
?
A)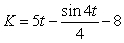
B)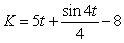
C)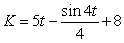
D)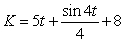
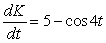 when
when 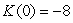 ?
?A)
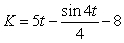
B)
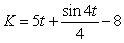
C)
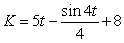
D)
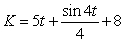

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
22
If 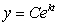 is a solution to the differential equation
is a solution to the differential equation 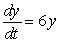 and y = 20 when t = 0, then k = _____ and C = _____.
and y = 20 when t = 0, then k = _____ and C = _____.
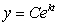 is a solution to the differential equation
is a solution to the differential equation 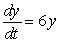 and y = 20 when t = 0, then k = _____ and C = _____.
and y = 20 when t = 0, then k = _____ and C = _____.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
23
Which one(s) of the following are solutions to the differential equation 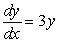 ?
?
A)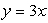
B)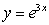
C)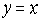
D)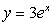
E)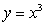
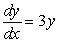 ?
?A)
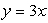
B)
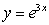
C)
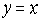
D)
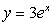
E)
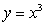

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
24
Is 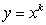 a solution to the differential equation
a solution to the differential equation 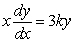 ?
?
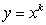 a solution to the differential equation
a solution to the differential equation 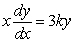 ?
?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
25
Is 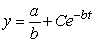 the general solution to the differential equation
the general solution to the differential equation 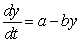 ?
?
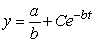 the general solution to the differential equation
the general solution to the differential equation 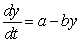 ?
?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
26
Consider the differential equation 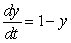 . Is
. Is 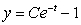 the general solution to the differential equation? If it is not, answer "not the solution". If it is the general solution and if y(3) = 5, what is the constant C?
the general solution to the differential equation? If it is not, answer "not the solution". If it is the general solution and if y(3) = 5, what is the constant C?
A)yes, C = 121
B)yes, 0.30
C)yes, 12.23
D)not the solution
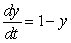 . Is
. Is 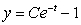 the general solution to the differential equation? If it is not, answer "not the solution". If it is the general solution and if y(3) = 5, what is the constant C?
the general solution to the differential equation? If it is not, answer "not the solution". If it is the general solution and if y(3) = 5, what is the constant C?A)yes, C = 121
B)yes, 0.30
C)yes, 12.23
D)not the solution

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
27
If 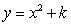 is a solution to the differential equation
is a solution to the differential equation 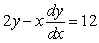 , then k = _____.
, then k = _____.
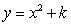 is a solution to the differential equation
is a solution to the differential equation 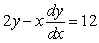 , then k = _____.
, then k = _____.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
28
Which one(s) of the following are solutions to the differential equation 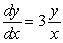 ?
?
A)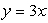
B)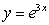
C)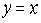
D)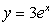
E)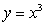
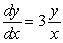 ?
?A)
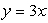
B)
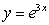
C)
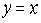
D)
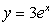
E)
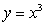

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
29
Which one(s) of the following are solutions to the differential equation 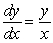 ?
?
A)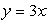
B)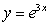
C)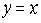
D)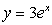
E)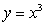
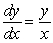 ?
?A)
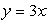
B)
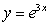
C)
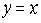
D)
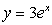
E)
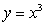

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
30
Find the solution of the differential equation 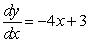 satisfying
satisfying 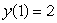 .
.
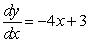 satisfying
satisfying 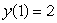 .
.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
31
Fill in the missing values in the table, given that 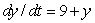 . Assume the growth rate, given by
. Assume the growth rate, given by 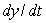 , is approximately constant over each time interval.
, is approximately constant over each time interval. 
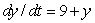 . Assume the growth rate, given by
. Assume the growth rate, given by 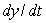 , is approximately constant over each time interval.
, is approximately constant over each time interval. 

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
32
In Kenya, the population P for the recent past has obeyed the growth model 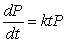 , with t the number of years since 1990. The solution to the differential equation is of the form
, with t the number of years since 1990. The solution to the differential equation is of the form 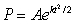 . If the population in 1990 was 24.64 million and in 1992 was 26.16 million, then A = _____ and k = _____. Thus, in the year 1997, the population was approximately _____ million. Round all answers to 2 decimal places.
. If the population in 1990 was 24.64 million and in 1992 was 26.16 million, then A = _____ and k = _____. Thus, in the year 1997, the population was approximately _____ million. Round all answers to 2 decimal places.
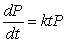 , with t the number of years since 1990. The solution to the differential equation is of the form
, with t the number of years since 1990. The solution to the differential equation is of the form 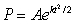 . If the population in 1990 was 24.64 million and in 1992 was 26.16 million, then A = _____ and k = _____. Thus, in the year 1997, the population was approximately _____ million. Round all answers to 2 decimal places.
. If the population in 1990 was 24.64 million and in 1992 was 26.16 million, then A = _____ and k = _____. Thus, in the year 1997, the population was approximately _____ million. Round all answers to 2 decimal places.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
33
What is the solution of 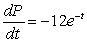 when
when 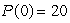 ?
?
A)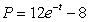
B)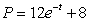
C)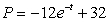
D)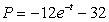
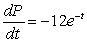 when
when 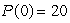 ?
?A)
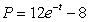
B)
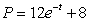
C)
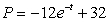
D)
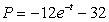

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
34
Given that 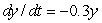 and that
and that 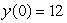 , estimate
, estimate 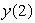 to 2 decimal places by first estimating y(1). Assume that the rate of growth given by
to 2 decimal places by first estimating y(1). Assume that the rate of growth given by 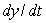 is approximately constant over each unit time interval.
is approximately constant over each unit time interval.
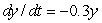 and that
and that 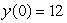 , estimate
, estimate 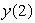 to 2 decimal places by first estimating y(1). Assume that the rate of growth given by
to 2 decimal places by first estimating y(1). Assume that the rate of growth given by 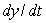 is approximately constant over each unit time interval.
is approximately constant over each unit time interval.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
35
Find the particular solution to the differential equation 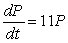 when
when 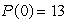 .
.
A)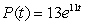
B)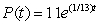
C)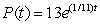
D)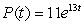
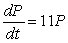 when
when 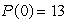 .
.A)
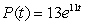
B)
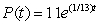
C)
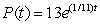
D)
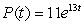

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
36
Does 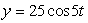 satisfy
satisfy 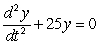 ?
?
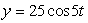 satisfy
satisfy 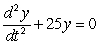 ?
?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
37
For 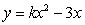 to be a solution to the differential equation
to be a solution to the differential equation 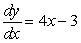 , what must be the constant k?
, what must be the constant k?
A)2
B)0
C)4
D)k can be any number
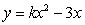 to be a solution to the differential equation
to be a solution to the differential equation 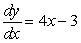 , what must be the constant k?
, what must be the constant k?A)2
B)0
C)4
D)k can be any number

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
38
Given that 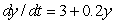 and that
and that 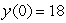 , estimate
, estimate 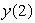 to 1 decimal place by first estimating y(1). Assume that the rate of growth given by
to 1 decimal place by first estimating y(1). Assume that the rate of growth given by 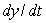 is approximately constant over each unit time interval.
is approximately constant over each unit time interval.
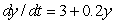 and that
and that 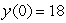 , estimate
, estimate 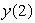 to 1 decimal place by first estimating y(1). Assume that the rate of growth given by
to 1 decimal place by first estimating y(1). Assume that the rate of growth given by 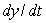 is approximately constant over each unit time interval.
is approximately constant over each unit time interval.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
39
Is 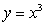 a solution to the differential equation
a solution to the differential equation 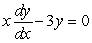 ?
?
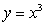 a solution to the differential equation
a solution to the differential equation 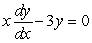 ?
?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
40
Suppose 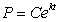 satisfies the differential equation
satisfies the differential equation 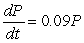 . Then k = _____ and C = _____. If either cannot be determined from the information given, enter "cannot tell".
. Then k = _____ and C = _____. If either cannot be determined from the information given, enter "cannot tell".
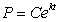 satisfies the differential equation
satisfies the differential equation 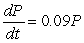 . Then k = _____ and C = _____. If either cannot be determined from the information given, enter "cannot tell".
. Then k = _____ and C = _____. If either cannot be determined from the information given, enter "cannot tell".
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
41
If the slope field for 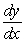 has constant slopes where x is fixed, what do we know about
has constant slopes where x is fixed, what do we know about 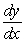 ?
?
A)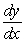 depends only on y.
depends only on y.
B)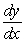 depends only on x.
depends only on x.
C)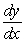 must be a constant.
must be a constant.
D)We can't determine anything about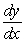 .
.
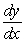 has constant slopes where x is fixed, what do we know about
has constant slopes where x is fixed, what do we know about 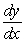 ?
?A)
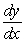 depends only on y.
depends only on y.B)
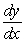 depends only on x.
depends only on x.C)
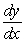 must be a constant.
must be a constant.D)We can't determine anything about
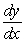 .
.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
42
Which of the following give a solution to the differential equation 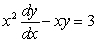 ? Select all that apply. first one:
? Select all that apply. first one: 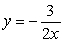 second one:
second one: 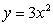
A)first one
B)second one
C)neither
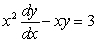 ? Select all that apply. first one:
? Select all that apply. first one: 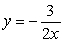 second one:
second one: 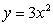
A)first one
B)second one
C)neither

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
43
The amount of medicine present in the blood of a patient decreases due to metabolism according to the exponential decay model. One hour after a dose was given, there were 3.7 nanograms/cm3 present, and a hour later there were 2.5 ng/cm3. After how many hours will there be less than 0.8 ng/cm3 present, assuming no more medication is taken? Round to 1 decimal place.

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
44
The following figure could be the slope field for the differential equation 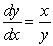 for
for 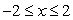 and
and 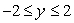 .
. 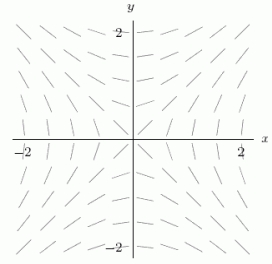
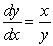 for
for 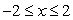 and
and 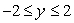 .
. 

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
45
Which of the following equations corresponds with the slope field shown below?
I.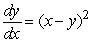 II.
II. 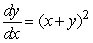 III.
III. 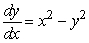 IV. None of them
IV. None of them 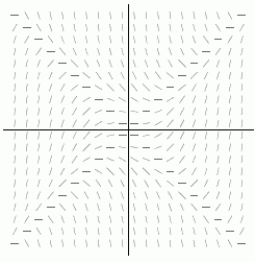
I.
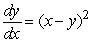 II.
II. 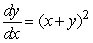 III.
III. 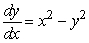 IV. None of them
IV. None of them 

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
46
An anti-inflammatory drug has a half-life in the human body of about 8 hours.
A. Use the half-life to find the value of k in the differential equation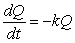 , where Q is the quantity of the drug in the body t hours after the drug is administered. Round to 4 decimal places.
, where Q is the quantity of the drug in the body t hours after the drug is administered. Round to 4 decimal places.
B. After how many hours will 45% of the original dose remain in the body? Round to 2 decimal places.
A. Use the half-life to find the value of k in the differential equation
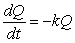 , where Q is the quantity of the drug in the body t hours after the drug is administered. Round to 4 decimal places.
, where Q is the quantity of the drug in the body t hours after the drug is administered. Round to 4 decimal places.B. After how many hours will 45% of the original dose remain in the body? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
47
Which of the following differential equations goes with the slope field in the figure? 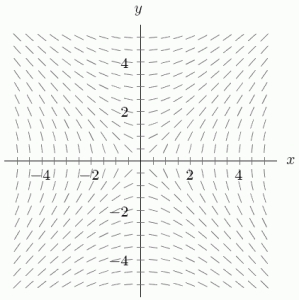
A)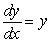
B)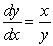
C)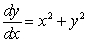
D)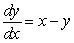

A)
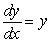
B)
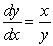
C)
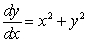
D)
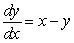

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
48
A radioactive isotope decays at a continuous rate of approximately 15% per day. If A is the amount of the isotope and t is time in days, what is the differential equation for this situation and its general solution?
A)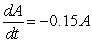 ;
; 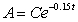
B)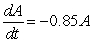 ;
; 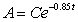
C)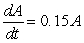 ;
; 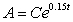
D)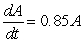 ;
; 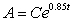
A)
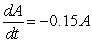 ;
; 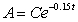
B)
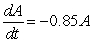 ;
; 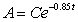
C)
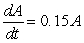 ;
; 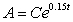
D)
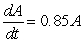 ;
; 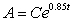

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
49
The following figure shows the slope field for the differential equation 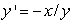 . Guess the equation of the solution curve that goes through the point (0,2).
. Guess the equation of the solution curve that goes through the point (0,2). 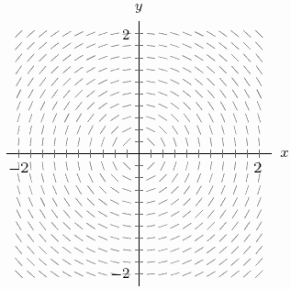
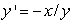 . Guess the equation of the solution curve that goes through the point (0,2).
. Guess the equation of the solution curve that goes through the point (0,2). 

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
50
Money in a bank account grows continuously at an annual rate of 4%. Suppose $10,000 is put into an account at time t = 0. If B is the balance in the account after t years, what is the differential equation for B and its solution?
A)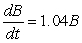 ;
; 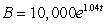
B)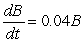 ;
; 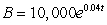
C)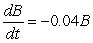 ;
; 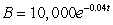
D)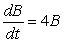 ;
; 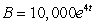
A)
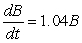 ;
; 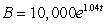
B)
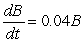 ;
; 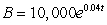
C)
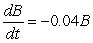 ;
; 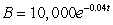
D)
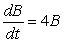 ;
; 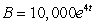

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
51
Which of the following slope fields goes with the differential equation 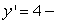 y?
y? 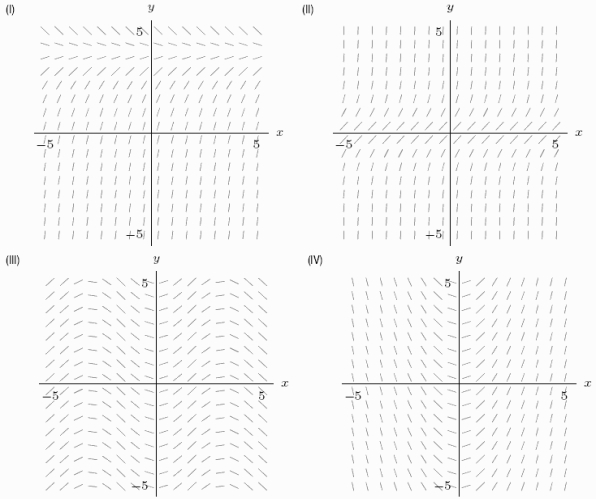
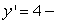 y?
y? 

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
52
Look at the slope field labeled I. Consider a solution curve for slope field (I). Which of the answer choices describes the long-run behavior of y at various starting points? 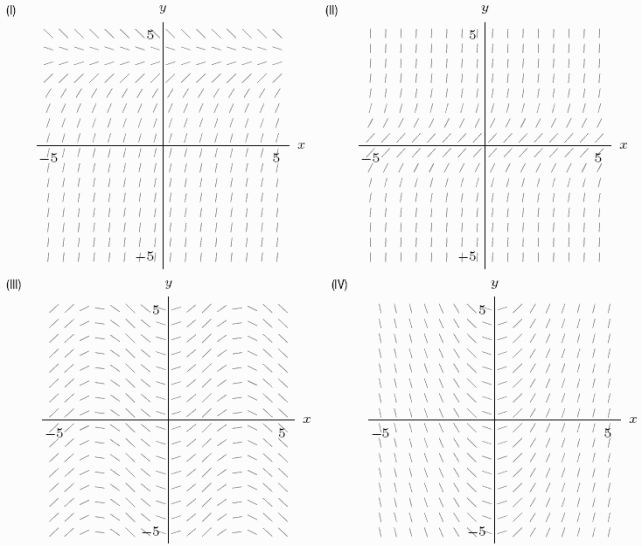
A)No matter what the starting point, as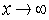 , y approaches the value 4.
, y approaches the value 4.
B)No matter what the starting point, as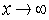 , y oscillates within a certain finite range.
, y oscillates within a certain finite range.
C)Depending on the starting point, as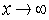 , y approaches
, y approaches
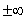 .
.
D)Depending on the starting point, as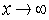 , y approaches
, y approaches
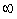 or 0.
or 0.

A)No matter what the starting point, as
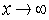 , y approaches the value 4.
, y approaches the value 4.B)No matter what the starting point, as
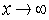 , y oscillates within a certain finite range.
, y oscillates within a certain finite range.C)Depending on the starting point, as
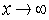 , y approaches
, y approaches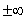 .
.D)Depending on the starting point, as
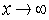 , y approaches
, y approaches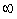 or 0.
or 0.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
53
The solution to the differential equation 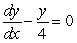 subject to the initial condition
subject to the initial condition 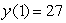 is
is 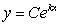 , where k = _____ and C = _____. Round answers to 2 decimal places.
, where k = _____ and C = _____. Round answers to 2 decimal places.
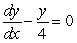 subject to the initial condition
subject to the initial condition 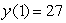 is
is 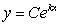 , where k = _____ and C = _____. Round answers to 2 decimal places.
, where k = _____ and C = _____. Round answers to 2 decimal places.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
54
For the first week, the spread of a rumor is proportional to the number of people who have heard the rumor. Find the particular solution to the differential equation for N, the number of people who have heard the rumor as a function of time in days, t, if 15 people have heard it at time t = 0, and 210 people have heard it at time t = 5.
A)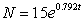
B)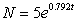
C)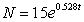
D)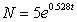
A)
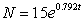
B)
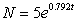
C)
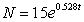
D)
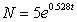

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
55
Sketch a slope field for the differential equation 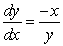 using the points indicated on the axes.
using the points indicated on the axes. 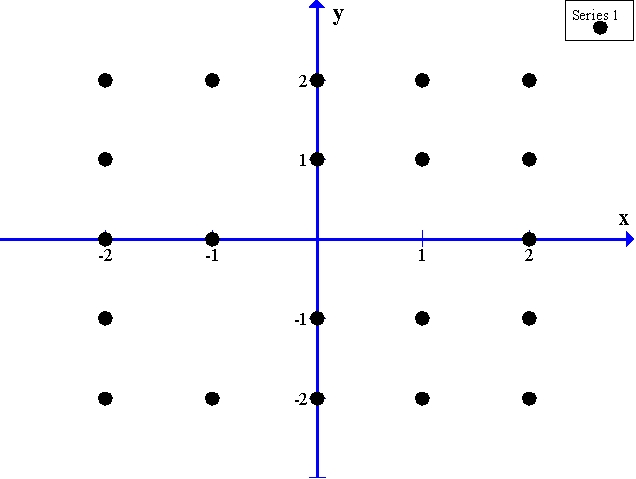
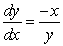 using the points indicated on the axes.
using the points indicated on the axes. 

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
56
On the slope field for the differential equation 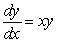 , sketch the solution curve in the fourth quadrant that goes through the point (0, -1).
, sketch the solution curve in the fourth quadrant that goes through the point (0, -1). 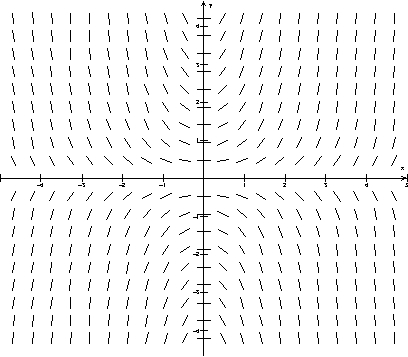
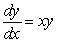 , sketch the solution curve in the fourth quadrant that goes through the point (0, -1).
, sketch the solution curve in the fourth quadrant that goes through the point (0, -1). 

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
57
Consider the slope field for 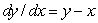 . What is the slope at the point (0,0)?
. What is the slope at the point (0,0)?
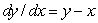 . What is the slope at the point (0,0)?
. What is the slope at the point (0,0)?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
58
Find a solution to the differential equation 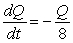 subject to the initial condition Q = 60 when t = 0.
subject to the initial condition Q = 60 when t = 0.
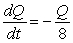 subject to the initial condition Q = 60 when t = 0.
subject to the initial condition Q = 60 when t = 0.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
59
What is the solution to the differential equation 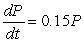 , given that P = 5 when t = 0?
, given that P = 5 when t = 0?
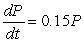 , given that P = 5 when t = 0?
, given that P = 5 when t = 0?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
60
The solution to the differential equation 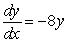 subject to the initial condition that y = 80 when x = 0 is
subject to the initial condition that y = 80 when x = 0 is 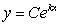 , where k = _____ and C = _____.
, where k = _____ and C = _____.
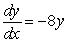 subject to the initial condition that y = 80 when x = 0 is
subject to the initial condition that y = 80 when x = 0 is 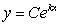 , where k = _____ and C = _____.
, where k = _____ and C = _____.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
61
On January 1, 1879, records show that 500 of a fish called Atlantic striped bass were introduced into the San Francisco Bay. In 1899, the first year fishing for bass was allowed, 100,000 of these bass were caught, representing 10% of the population at the start of 1899. Owing to reproduction, at any moment in time the bass population is growing at a rate proportional to the population at that moment. Write a differential equation satisfied by B(t), the number of Atlantic striped bass a time t, where t is in years since January 1, 1879 and 0 t < 20 and solve it for B(t).
A)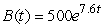
B)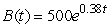
C)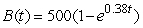
D)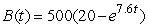
A)
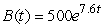
B)
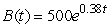
C)
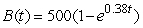
D)
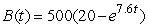

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
62
Newton's Law of Cooling states that the rate of change of temperature of an object is proportional to the difference between the temperature of the object and the temperature of the surrounding air. A detective discovers a corpse in an abandoned building, and finds its temperature to be 24°C. An hour later its temperature is 16°C. Assume that the air temperature is 8°C, that normal body temperature is 37°C, and that Newton's Law of Cooling applies to the corpse. How many hours has the corpse been dead at the moment it is discovered? Round to 2 decimal places.

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
63
What is the solution of the differential equation 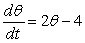 if
if 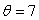 when
when 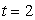 ?
?
A)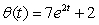
B)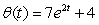
C)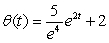
D)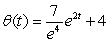
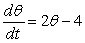 if
if 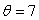 when
when 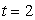 ?
?A)
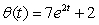
B)
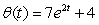
C)
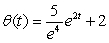
D)
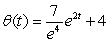

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
64
A person receives a drug intravenously at the rate of 3 mg per hour. The drug is eliminated from the body at a rate proportional to the amount present with a constant of proportionality of k = -0.4. What is the long term amount of the drug in the body, once the system has stabilized?

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
65
The logistic model for a population's growth is 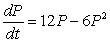 , where P is the size of the population in millions at any time t, measured in years. What is the size of the population when the rate of increase starts to decrease?
, where P is the size of the population in millions at any time t, measured in years. What is the size of the population when the rate of increase starts to decrease?
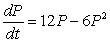 , where P is the size of the population in millions at any time t, measured in years. What is the size of the population when the rate of increase starts to decrease?
, where P is the size of the population in millions at any time t, measured in years. What is the size of the population when the rate of increase starts to decrease?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
66
You invest $2,500 in your nephew's catering business. He guarantees you a minimum return at a continuous interest rate of 4%. Of course, if the business continues to thrive, you will earn at a higher rate.
a) Write a differential equation for the minimum amount, B, of your return on investment at time t.
b) Solve the differential equation.
c) Graph the solution.
a) Write a differential equation for the minimum amount, B, of your return on investment at time t.
b) Solve the differential equation.
c) Graph the solution.

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
67
What is the general solution of 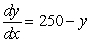 ?
?
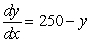 ?
?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
68
What is the general solution to the differential equation 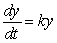 ?
?
A)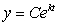
B)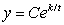
C)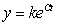
D)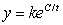
E)none of the above
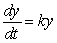 ?
?A)
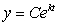
B)
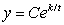
C)
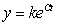
D)
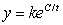
E)none of the above

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
69
According to Newton, the rate at which the temperature of water in a swimming pool changes is directly proportional to the difference between the temperature L outside and the temperature T of the water in the pool. Suppose the temperature outside stays at a constant 28 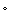 C for two hours, and that during that time the temperature of the water increases from 20
C for two hours, and that during that time the temperature of the water increases from 20 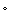 C to 24
C to 24 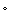 C. What is the equilibrium solution to the equation modeling this situation?
C. What is the equilibrium solution to the equation modeling this situation?
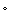 C for two hours, and that during that time the temperature of the water increases from 20
C for two hours, and that during that time the temperature of the water increases from 20 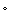 C to 24
C to 24 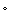 C. What is the equilibrium solution to the equation modeling this situation?
C. What is the equilibrium solution to the equation modeling this situation?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
70
According to Newton, the rate at which the temperature of water in a swimming pool changes is directly proportional to the difference between the temperature L outside and the temperature T of the water in the pool. Suppose the temperature outside stays at a constant 28 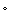 C for two hours, and that during that time the temperature of the water increases from 20
C for two hours, and that during that time the temperature of the water increases from 20  C to 24
C to 24  C. Which of the following shows the differential equation for this situation and its solution?
C. Which of the following shows the differential equation for this situation and its solution?
A)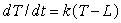 ;
; 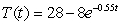
B)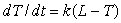 ;
; 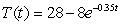
C)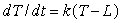 ;
; 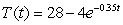
D)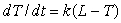 ;
; 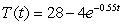
 C for two hours, and that during that time the temperature of the water increases from 20
C for two hours, and that during that time the temperature of the water increases from 20  C to 24
C to 24  C. Which of the following shows the differential equation for this situation and its solution?
C. Which of the following shows the differential equation for this situation and its solution?A)
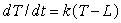 ;
; 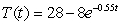
B)
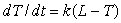 ;
; 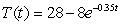
C)
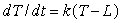 ;
; 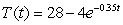
D)
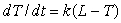 ;
; 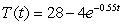

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
71
A lake with constant volume V, in km3, contains a quantity of Q km3 pollutant. Clean water enters the lake and causes a total outflow of r km3 per year. The rate at which the pollutant decreases at any time t equals the product of the pollutant Q per volume V and the rate at which the water flows out of the lake. If 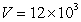 km3 and
km3 and 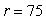 km3 per year, how many years will it take for the pollutant to decrease to half of its original quantity? Round to the nearest whole year.
km3 per year, how many years will it take for the pollutant to decrease to half of its original quantity? Round to the nearest whole year.
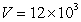 km3 and
km3 and 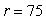 km3 per year, how many years will it take for the pollutant to decrease to half of its original quantity? Round to the nearest whole year.
km3 per year, how many years will it take for the pollutant to decrease to half of its original quantity? Round to the nearest whole year.
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
72
A country experiences a continuous inflation rate of about 5.7% per year. If a t-shirt had a value of $12 in 1995, write a differential equation and use it to find what the t-shirt's value was in 2005. Round to the nearest cent.

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
73
A certain bank account earns interest at the rate of 5% compounded continuously. Money is being withdrawn from the account in a continuous stream at a constant rate of $100,000 per year. Write a differential equation modeling how the balance B changes over time. Which of the following is the general solution, given an initial balance of B0?
A)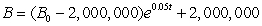
B)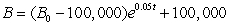
C)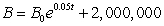
D)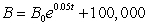
A)
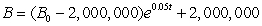
B)
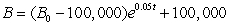
C)
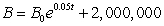
D)
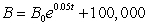

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
74
A certain bank account earns interest at the rate of 5% compounded continuously. Money is being withdrawn from the account in a continuous stream at a constant rate of $100,000 per year. Use differential equations to determine what the minimum initial balance should be in order for the account never to be depleted.

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
75
If no more pollutants are dumped into a lake, the amount of pollution in the lake will decrease at a rate proportional to the amount of pollution present. If there are 400 units of pollution present initially and 184 units left after 8 years, use differential equations to find the number of units left after 13 years. Round to 1 decimal place.

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
76
What is the general solution of 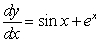 ?
?
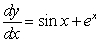 ?
?
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
77
A company earns a continuous annual rate of 11% of its net worth. At the same time, it has expenses of 6.2 million dollars per year. If the company's net worth at time t = 0 is 50 million, how many years will it take to go bankrupt? Round to the nearest year.

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
78
A company earns a continuous annual rate of 11% of its net worth. At the same time, it has expenses of 6.4 million dollars per year. Write a differential equation for the company's worth, W, in millions of dollars as a function of time t, in years. What is the general solution to your differential equation?
A)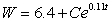
B)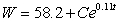
C)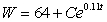
D)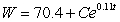
A)
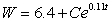
B)
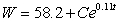
C)
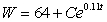
D)
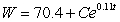

Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
79
The equilibrium solution for 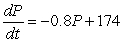 is P = _____. This solution is ________ (stable/unstable).
is P = _____. This solution is ________ (stable/unstable).
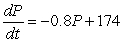 is P = _____. This solution is ________ (stable/unstable).
is P = _____. This solution is ________ (stable/unstable).
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck
80
The equilibrium solution for 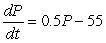 is P = _____. This solution is ________ (stable/unstable).
is P = _____. This solution is ________ (stable/unstable).
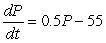 is P = _____. This solution is ________ (stable/unstable).
is P = _____. This solution is ________ (stable/unstable).
Unlock Deck
Unlock for access to all 121 flashcards in this deck.
Unlock Deck
k this deck



