Deck 8: Calculus of Several Variables
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/219
Play
Full screen (f)
Deck 8: Calculus of Several Variables
1
Find the volume of the solid bounded above by the surface 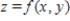 and below by the plane region R.
and below by the plane region R. 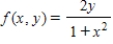 ; R is the region bounded by
; R is the region bounded by 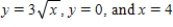 .
.
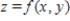 and below by the plane region R.
and below by the plane region R. 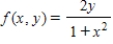 ; R is the region bounded by
; R is the region bounded by 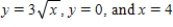 .
.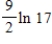
2
The Country Workshop's total weekly profit (in dollars) realized in manufacturing and selling its rolltop desks is given by the profit function 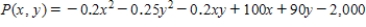
Where stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies betweenand 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies betweenand 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
A)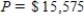 per week
per week
B)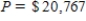 per week
per week
C)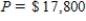 per week
per week
D)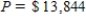 per week
per week
E)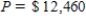 per week
per week
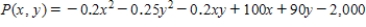
Where
 stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies betweenand 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies betweenand 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
A)
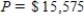 per week
per weekB)
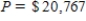 per week
per weekC)
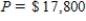 per week
per weekD)
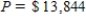 per week
per weekE)
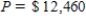 per week
per week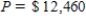 per week
per week 3
Find the average value of the given function 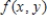 over the plane region R.
over the plane region R. 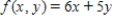 ; R is the triangle with vertices (0, 0), (2, 0) and (2, 2).
; R is the triangle with vertices (0, 0), (2, 0) and (2, 2).
A) 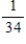
B) 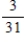
C) 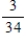
D) 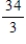
E) 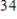
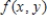 over the plane region R.
over the plane region R. 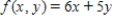 ; R is the triangle with vertices (0, 0), (2, 0) and (2, 2).
; R is the triangle with vertices (0, 0), (2, 0) and (2, 2).
A)
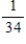
B)
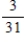
C)
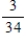
D)
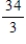
E)
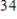
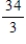
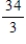
4
Find the volume of the solid bounded above by the surfac 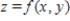 and below by the plane region R.
and below by the plane region R. 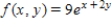 ; R is the triangle with vertices (0, 0), (2, 0) and (0, 2).
; R is the triangle with vertices (0, 0), (2, 0) and (0, 2).
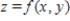 and below by the plane region R.
and below by the plane region R. 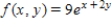 ; R is the triangle with vertices (0, 0), (2, 0) and (0, 2).
; R is the triangle with vertices (0, 0), (2, 0) and (0, 2).
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
5
Use a double integral to find the volume of the solid shown in the figure. 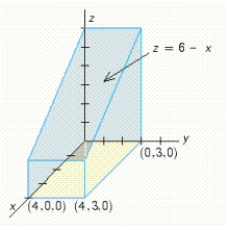
A) 36
B) 24
C) 144
D) 12
E) 48

A) 36
B) 24
C) 144
D) 12
E) 48

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
6
Find the volume of the solid bounded above by the surface 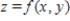 and below by the plane region R.
and below by the plane region R. 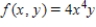 and R is the region bounded by the graphs of
and R is the region bounded by the graphs of 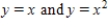 .
.
A)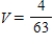
B) 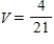
C) 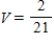
D) 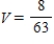
E) 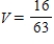
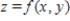 and below by the plane region R.
and below by the plane region R. 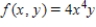 and R is the region bounded by the graphs of
and R is the region bounded by the graphs of 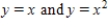 .
.
A)
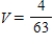
B)
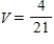
C)
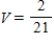
D)
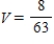
E)
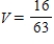

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
7
Use a double integral to find the volume of the solid shown in the figure.
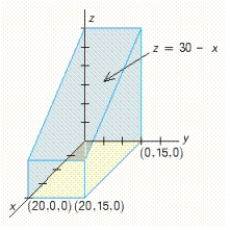
__________ cu units.

__________ cu units.

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
8
Use a double integral to find the volume of the solid shown in the figure. 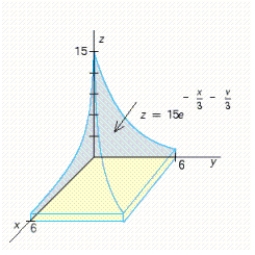
A)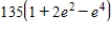
B) 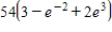
C) 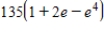
D) 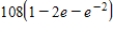
E) 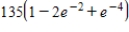

A)
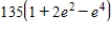
B)
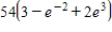
C)
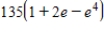
D)
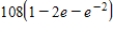
E)
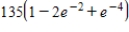

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
9
Find the volume of the solid bounded above by the surface 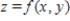 and below by the plane region R.
and below by the plane region R. 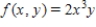 and R is the region bounded by the graphs of
and R is the region bounded by the graphs of  and
and 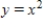 .
.
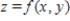 and below by the plane region R.
and below by the plane region R. 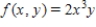 and R is the region bounded by the graphs of
and R is the region bounded by the graphs of  and
and 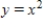 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
10
The population density of a certain city is given by the function 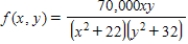
Where the origin (0, 0) gives the location of the government center. Find the population inside the rectangular area described by
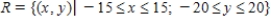
A)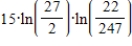
B) 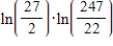
C) 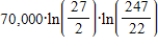
D) 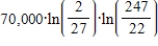
E) 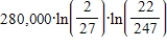
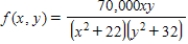
Where the origin (0, 0) gives the location of the government center. Find the population inside the rectangular area described by
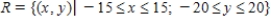
A)
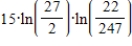
B)
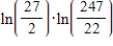
C)
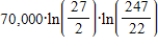
D)
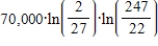
E)
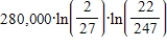

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
11
Use a double integral to find the volume of the solid shown in the figure. 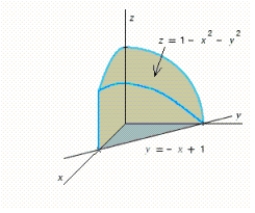
A)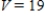
B) 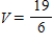
C) 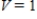
D) 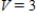
E) 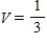

A)
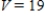
B)
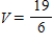
C)
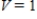
D)
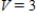
E)
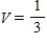

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
12
Find the average value of the function 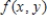 over the plane region R.
over the plane region R. 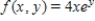 and R is the triangle with vertices
and R is the triangle with vertices 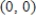 ,
, 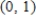 and
and 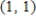 .
.
A) 5
B) 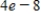
C) 25
D) 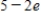
E)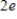
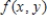 over the plane region R.
over the plane region R. 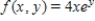 and R is the triangle with vertices
and R is the triangle with vertices 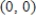 ,
, 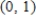 and
and 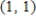 .
. A) 5
B)
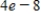
C) 25
D)
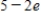
E)
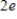

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
13
Find the volume of the solid bounded above by the surface 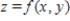
And below by the plane region R.
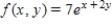
R is the triangle with vertices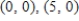 and
and 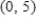 .
.
A)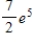
B) 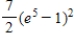
C) 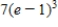
D) 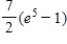
E) 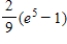
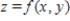
And below by the plane region R.
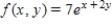
R is the triangle with vertices
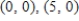 and
and 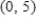 .
.
A)
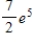
B)
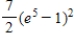
C)
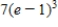
D)
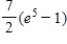
E)
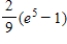

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
14
Use a double integral to find the volume of the solid shown in the figure. 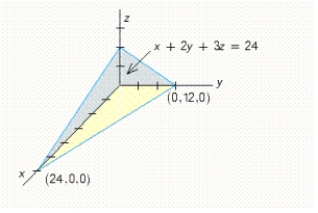
A) 12
B) 96
C) 64
D) 256
E) 384

A) 12
B) 96
C) 64
D) 256
E) 384

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
15
Find the volume of the solid bounded above by the surface 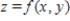 and below by the plane region R.
and below by the plane region R. 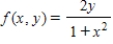 ; R is the region bounded by
; R is the region bounded by 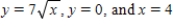 .
.
A)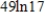
B) 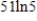
C) 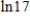
D) 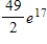
E) 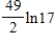
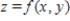 and below by the plane region R.
and below by the plane region R. 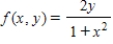 ; R is the region bounded by
; R is the region bounded by 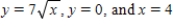 .
.
A)
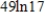
B)
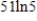
C)
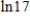
D)
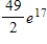
E)
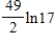

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
16
The Country Workshop's total weekly profit (in dollars) realized in manufacturing and selling its rolltop desks is given by the profit function
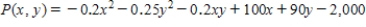
where x stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies between 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
P = $__________ per week.
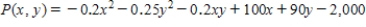
where x stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies between 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
P = $__________ per week.

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
17
Use a double integral to find the volume of the solid shown in the figure.
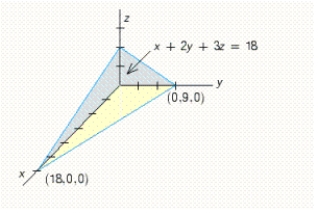
__________ cu units.

__________ cu units.

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
18
Find the average value of the given function 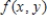 over the plane region R.
over the plane region R. 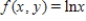 ; R is the region bounded by the graph of
; R is the region bounded by the graph of 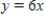 and
and 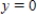 from
from 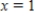 to
to 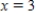 .
.
A)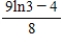
B) 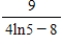
C) 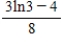
D) 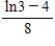
E) 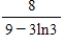
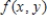 over the plane region R.
over the plane region R. 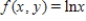 ; R is the region bounded by the graph of
; R is the region bounded by the graph of 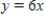 and
and 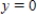 from
from 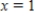 to
to 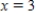 .
.
A)
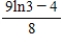
B)
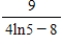
C)
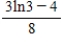
D)
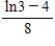
E)
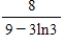

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
19
Use a double integral to find the volume of the solid shown in the figure.
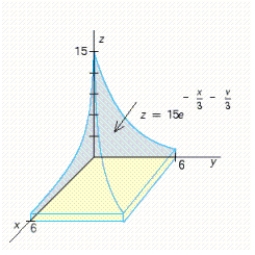


Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
20
Use a double integral to find the volume of the solid shown in the figure. 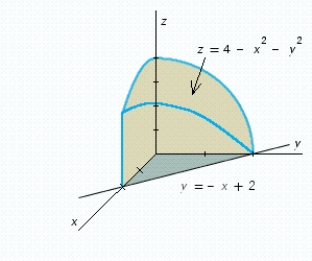


Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
21
Evaluate the double integral 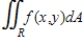
For the given function f(x, y) and the region R.
F(x, y) = 6x + 6y; R is bounded by x = 0,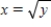 , y = 0 and y = 4.
, y = 0 and y = 4.
A)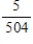
B)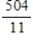
C)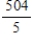
D)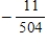
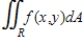
For the given function f(x, y) and the region R.
F(x, y) = 6x + 6y; R is bounded by x = 0,
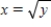 , y = 0 and y = 4.
, y = 0 and y = 4.
A)
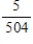
B)
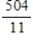
C)
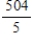
D)
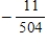

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
22
Find the volume of the solid bounded above by the surface
Z = f(x, y)
And below by the plane region R.
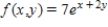 ; R is the triangle with vertices (0, 0), (5, 0) and (0, 5).
; R is the triangle with vertices (0, 0), (5, 0) and (0, 5).
A)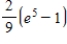
B)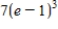
C)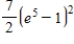
Z = f(x, y)
And below by the plane region R.
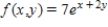 ; R is the triangle with vertices (0, 0), (5, 0) and (0, 5).
; R is the triangle with vertices (0, 0), (5, 0) and (0, 5).
A)
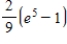
B)
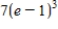
C)
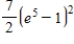

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
23
Evaluate the double integral 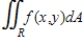
For the function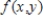 and the region R.
and the region R.
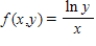 and R is the rectangle defined by
and R is the rectangle defined by 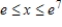 and
and 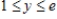 .
.
A) 8
B) 6
C) 2
D) 3
E) 5
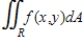
For the function
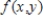 and the region R.
and the region R.
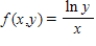 and R is the rectangle defined by
and R is the rectangle defined by 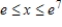 and
and 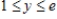 .
.
A) 8
B) 6
C) 2
D) 3
E) 5

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
24
Evaluate the double integral 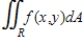
For the given function f(x, y) and the region R.
F(x, y) = 4x + 2y; R is bounded by x = 0, x = 4, y = 0 and y = x.
A)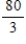
B)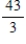
C)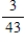
D)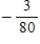
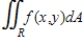
For the given function f(x, y) and the region R.
F(x, y) = 4x + 2y; R is bounded by x = 0, x = 4, y = 0 and y = x.
A)
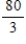
B)
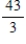
C)
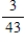
D)
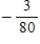

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
25
Evaluate the double integral 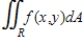
For the given function f(x, y) and the region R.
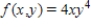 ; R is the rectangle defined by
; R is the rectangle defined by 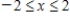 and
and 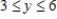 .
.
A) 0
B) 4
C) -4
D) 1
For the given function f(x, y) and the region R.
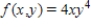 ; R is the rectangle defined by
; R is the rectangle defined by 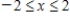 and
and 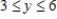 .
.
A) 0
B) 4
C) -4
D) 1

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
26
Use a double integral to find the volume of the solid shown in the figure. 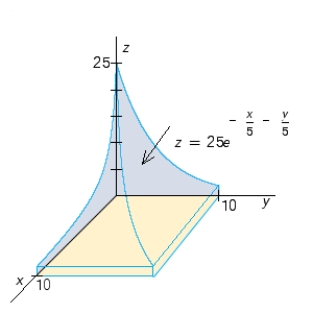
A)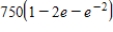
B)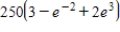
C)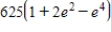
D)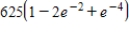

A)
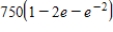
B)
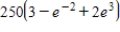
C)
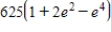
D)
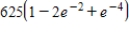

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
27
Evaluate the double integral 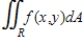
For the given function f(x, y) and the region R.
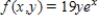 ; R is bounded by
; R is bounded by 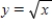 and y = x.
and y = x.
A)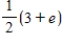
B)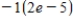
C)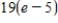
D)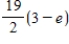
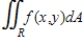
For the given function f(x, y) and the region R.
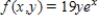 ; R is bounded by
; R is bounded by 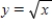 and y = x.
and y = x.
A)
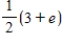
B)
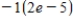
C)
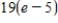
D)
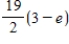

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
28
Find the average value of the given function 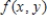 over the plane region R.
over the plane region R.
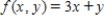 ; R is the triangle with vertices (0, 0), (1, 0) and (1, 1).
; R is the triangle with vertices (0, 0), (1, 0) and (1, 1).
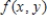 over the plane region R.
over the plane region R.
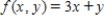 ; R is the triangle with vertices (0, 0), (1, 0) and (1, 1).
; R is the triangle with vertices (0, 0), (1, 0) and (1, 1).
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
29
Evaluate the double integral 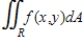
For the given function f(x, y) and the region R.
F(x, y) = 2y + x; R is the rectangle defined by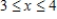 and
and 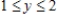 .
.
A)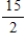
B)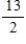
C)
D) 26
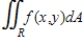
For the given function f(x, y) and the region R.
F(x, y) = 2y + x; R is the rectangle defined by
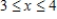 and
and 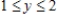 .
.
A)
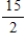
B)
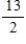
C)

D) 26

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
30
Use a double integral to find the volume of the solid shown in the figure. 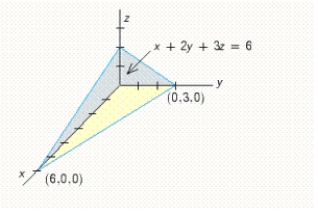
A) 6
B) 4
C) 9
D) 14

A) 6
B) 4
C) 9
D) 14

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
31
Use a double integral to find the volume of the solid shown in the figure. 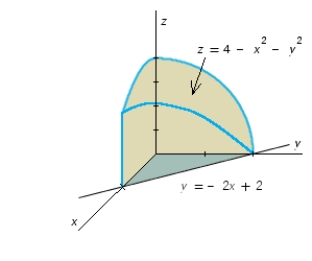
A)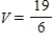
B)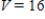
C)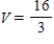
D)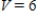
E)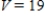

A)
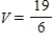
B)
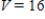
C)
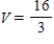
D)
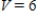
E)
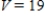

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
32
Evaluate the double integral 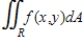
For the given function f(x, y) and the region R.
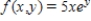 ; R is bounded by x = 0, x = 1, y = 0 and y = x.
; R is bounded by x = 0, x = 1, y = 0 and y = x.
A) 2.5
B) 3.5e
C) -3.5
D) -2.5
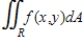
For the given function f(x, y) and the region R.
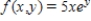 ; R is bounded by x = 0, x = 1, y = 0 and y = x.
; R is bounded by x = 0, x = 1, y = 0 and y = x.
A) 2.5
B) 3.5e
C) -3.5
D) -2.5

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
33
Evaluate the double integral 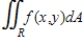
For the given function f(x, y) and the region R.
F(x, y) = 6x + 12y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
A)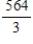
B)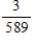
C)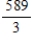
D)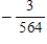
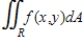
For the given function f(x, y) and the region R.
F(x, y) = 6x + 12y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
A)
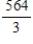
B)
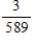
C)
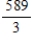
D)
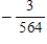

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
34
Find the average value of the given function 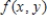 over the plane region R.
over the plane region R.
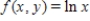 ; R is the region bounded by the graph of
; R is the region bounded by the graph of 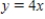 and
and 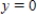 from
from 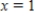 to
to 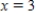 .
.
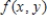 over the plane region R.
over the plane region R.
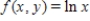 ; R is the region bounded by the graph of
; R is the region bounded by the graph of 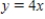 and
and 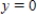 from
from 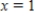 to
to 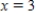 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
35
Evaluate the double integral 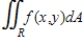
For the given function f(x, y) and the region R.
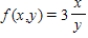 ; R is the rectangle defined by
; R is the rectangle defined by 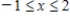 and
and 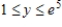
A)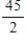
B)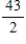
C)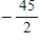
D)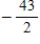
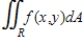
For the given function f(x, y) and the region R.
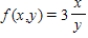 ; R is the rectangle defined by
; R is the rectangle defined by 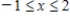 and
and 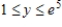
A)
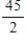
B)
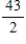
C)
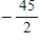
D)
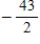

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
36
Find the volume of the solid bounded above by the surface
Z = f(x, y)
And below by the plane region R.
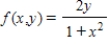 ; R is the region bounded by
; R is the region bounded by 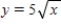 ,
, 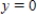 , and
, and 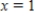 .
.
A)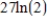
B)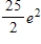
C)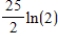
Z = f(x, y)
And below by the plane region R.
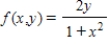 ; R is the region bounded by
; R is the region bounded by 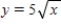 ,
, 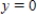 , and
, and 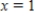 .
.
A)
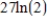
B)
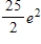
C)
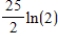

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
37
Evaluate the double integral 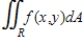
For the function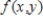 and the region R.
and the region R.
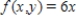 and R is bounded by
and R is bounded by 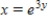 ,
,  ,
, 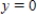 and
and 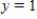 .
.
A)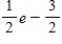
B)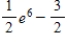
C)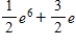
D)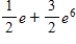
E)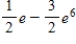
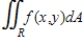
For the function
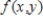 and the region R.
and the region R.
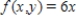 and R is bounded by
and R is bounded by 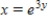 ,
,  ,
, 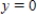 and
and 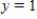 .
.
A)
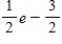
B)
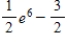
C)
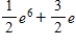
D)
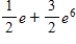
E)
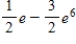

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
38
Evaluate the double integral 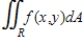
For the given function f(x, y) and the region R.
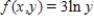 ; R is the rectangle defined by
; R is the rectangle defined by 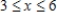 and
and 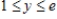 .
.
A) -10
B) 10e
C) 9
D) -9e
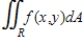
For the given function f(x, y) and the region R.
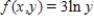 ; R is the rectangle defined by
; R is the rectangle defined by 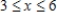 and
and 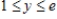 .
.
A) -10
B) 10e
C) 9
D) -9e

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
39
Use a double integral to find the volume of the solid shown in the figure. 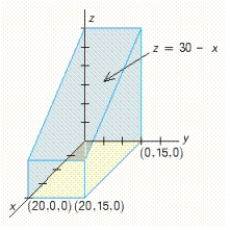
A) 5,996
B) 6,008
C) 6,000
D) 5,994

A) 5,996
B) 6,008
C) 6,000
D) 5,994

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
40
Find the average value of the function 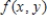 over the plane region R.
over the plane region R.
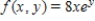 and R is the triangle with vertices (0, 0), (0, 1) and (1, 1).
and R is the triangle with vertices (0, 0), (0, 1) and (1, 1).
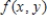 over the plane region R.
over the plane region R.
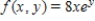 and R is the triangle with vertices (0, 0), (0, 1) and (1, 1).
and R is the triangle with vertices (0, 0), (0, 1) and (1, 1).
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
41
Find the volume of the solid bounded above by the surface 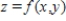
And below by the plane region R.
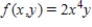 and R is the region bounded by the graphs of
and R is the region bounded by the graphs of  and
and 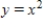 .
.
A)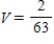
B)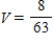
C)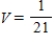
D)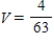
E)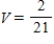
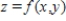
And below by the plane region R.
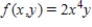 and R is the region bounded by the graphs of
and R is the region bounded by the graphs of  and
and 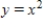 .
.
A)
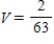
B)
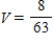
C)
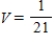
D)
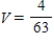
E)
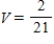

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
42
Evaluate the double integral 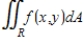 for the given function f(x, y) and the region R. Enter your answer as a fraction.
for the given function f(x, y) and the region R. Enter your answer as a fraction. 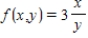 ; R is the rectangle defined by
; R is the rectangle defined by 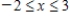 and
and 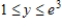 .
.
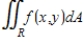 for the given function f(x, y) and the region R. Enter your answer as a fraction.
for the given function f(x, y) and the region R. Enter your answer as a fraction. 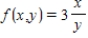 ; R is the rectangle defined by
; R is the rectangle defined by 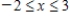 and
and 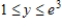 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
43
The Country Workshop's total weekly profit (in dollars) realized in manufacturing and selling its rolltop desks is given by the profit function 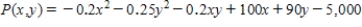
Where x stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies between 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
A)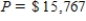 per week
per week
B)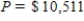 per week
per week
C)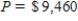 per week
per week
D)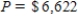 per week
per week
E)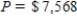 per week
per week
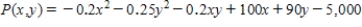
Where x stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies between 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
A)
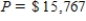 per week
per weekB)
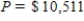 per week
per weekC)
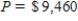 per week
per weekD)
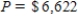 per week
per weekE)
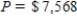 per week
per week
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
44
Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false.
If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_6725_b0a1_abb0ce5e996b_TB8255_11.jpg) where
where ![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_6726_b0a1_05e29c894151_TB8255_11.jpg) .
.
A) From the definition follows![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_6727_b0a1_2b5f95c919b1_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e38_b0a1_93acc4ae3fb9_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e39_b0a1_01db2b4e5c74_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3a_b0a1_e99c7fc18596_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3b_b0a1_c100a560b7f6_TB8255_11.jpg) , so the statement is false.
, so the statement is false.
B) From the definition follows![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3c_b0a1_3b81855ebcad_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3d_b0a1_9f958824f5c9_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3e_b0a1_e5aea5cc6ad2_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3f_b0a1_b9cefa9d035c_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e40_b0a1_bf28c28213bb_TB8255_11.jpg) , so the statement is true.
, so the statement is true.
C) From the definition follows![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b551_b0a1_6deebf5c8047_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b552_b0a1_0d06b43c908a_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b553_b0a1_f57b73363d8b_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b554_b0a1_153e0fa2a49d_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b555_b0a1_b94a8229bff6_TB8255_11.jpg) , so the statement is false.
, so the statement is false.
If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_6725_b0a1_abb0ce5e996b_TB8255_11.jpg) where
where ![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_6726_b0a1_05e29c894151_TB8255_11.jpg) .
.
A) From the definition follows
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_6727_b0a1_2b5f95c919b1_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e38_b0a1_93acc4ae3fb9_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e39_b0a1_01db2b4e5c74_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3a_b0a1_e99c7fc18596_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3b_b0a1_c100a560b7f6_TB8255_11.jpg) , so the statement is false.
, so the statement is false.B) From the definition follows
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3c_b0a1_3b81855ebcad_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3d_b0a1_9f958824f5c9_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3e_b0a1_e5aea5cc6ad2_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e3f_b0a1_b9cefa9d035c_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_8e40_b0a1_bf28c28213bb_TB8255_11.jpg) , so the statement is true.
, so the statement is true.C) From the definition follows
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b551_b0a1_6deebf5c8047_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b552_b0a1_0d06b43c908a_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b553_b0a1_f57b73363d8b_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b554_b0a1_153e0fa2a49d_TB8255_11.jpg)
![<strong>Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where . </strong> A) From the definition follows , so the statement is false. B) From the definition follows , so the statement is true. C) From the definition follows , so the statement is false.](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cadb_b555_b0a1_b94a8229bff6_TB8255_11.jpg) , so the statement is false.
, so the statement is false.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
45
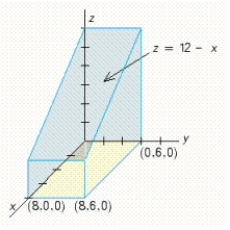
Use a double integral to find the volume of the solid shown in the figure.


Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
46
Find the average value of the function 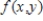
Over the plane region R.
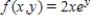 and R is the triangle with vertices (0, 0), (0, 1) and (1, 1).
and R is the triangle with vertices (0, 0), (0, 1) and (1, 1).
A)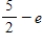
B)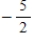
C)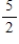
D)
E)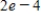
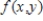
Over the plane region R.
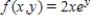 and R is the triangle with vertices (0, 0), (0, 1) and (1, 1).
and R is the triangle with vertices (0, 0), (0, 1) and (1, 1).
A)
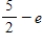
B)
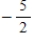
C)
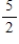
D)

E)
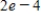

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
47
The Country Workshop's total weekly profit (in dollars) realized in manufacturing and selling its rolltop desks is given by the profit function
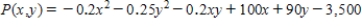
where x stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies between 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
P = $__________ per week.
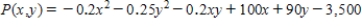
where x stands for the number of finished units and y stands for the number of unfinished units manufactured and sold each week. Find the average weekly profit if the number of finished units manufactured and sold varies between 180 and 200 and the number of unfinished units varies between 100 and 120 per week. Please round your answer to the nearest dollar, if necessary.
P = $__________ per week.

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
48
Evaluate the double integral
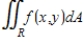
for the given function f(x, y) and the region R. Enter your answer as a fraction.
f(x, y) = 0x + 0y; R is bounded by x = 0,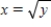 , y = 0 and y = 4.
, y = 0 and y = 4.
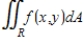
for the given function f(x, y) and the region R. Enter your answer as a fraction.
f(x, y) = 0x + 0y; R is bounded by x = 0,
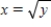 , y = 0 and y = 4.
, y = 0 and y = 4.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
49
Evaluate the double integral
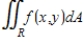
for the function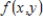 and the region R.
and the region R.
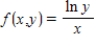 and R is the rectangle defined by
and R is the rectangle defined by 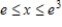 and
and 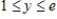 .
.
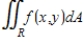
for the function
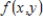 and the region R.
and the region R.
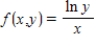 and R is the rectangle defined by
and R is the rectangle defined by 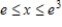 and
and 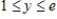 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
50
Evaluate the double integral
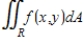
for the given function f(x, y) and the region R.
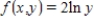 ; R is the rectangle defined by
; R is the rectangle defined by 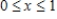 and
and 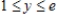 .
.
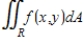
for the given function f(x, y) and the region R.
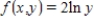 ; R is the rectangle defined by
; R is the rectangle defined by 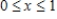 and
and 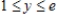 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
51
Evaluate the double integral 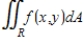 for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 2y + 3x; R is the rectangle defined by
for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 2y + 3x; R is the rectangle defined by 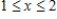 and
and 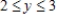 .
.
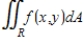 for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 2y + 3x; R is the rectangle defined by
for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 2y + 3x; R is the rectangle defined by 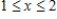 and
and 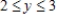 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
52
Find the average value of the given function 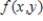
Over the plane region R.
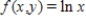 ; R is the region bounded by the graph of y = 8x and y = 0 from x = 1 to x = 7.
; R is the region bounded by the graph of y = 8x and y = 0 from x = 1 to x = 7.
A)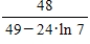
B)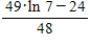
C)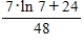
D)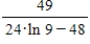
Over the plane region R.
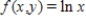 ; R is the region bounded by the graph of y = 8x and y = 0 from x = 1 to x = 7.
; R is the region bounded by the graph of y = 8x and y = 0 from x = 1 to x = 7.
A)
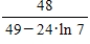
B)
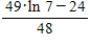
C)
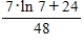
D)
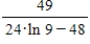

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
53
Evaluate the double integral
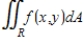
for the given function f(x, y) and the region R.
 ; R is bounded by x = 0, x = 1, y = 0 and y = x.
; R is bounded by x = 0, x = 1, y = 0 and y = x.
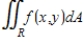
for the given function f(x, y) and the region R.
 ; R is bounded by x = 0, x = 1, y = 0 and y = x.
; R is bounded by x = 0, x = 1, y = 0 and y = x.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
54
Evaluate the double integral 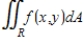 for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 3x + 4y; R is bounded by x = 0, x = 1, y = 0 and y = x.
for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 3x + 4y; R is bounded by x = 0, x = 1, y = 0 and y = x.
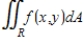 for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 3x + 4y; R is bounded by x = 0, x = 1, y = 0 and y = x.
for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 3x + 4y; R is bounded by x = 0, x = 1, y = 0 and y = x.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
55
Use a double integral to find the volume of the solid shown in the figure.
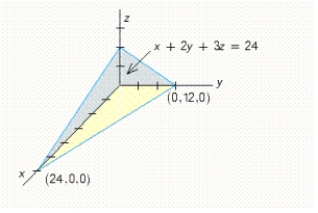


Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
56
The population density of a certain city is given by the function 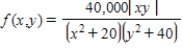
Where the origin (0, 0) gives the location of the government center. Find the population inside the rectangular area described by
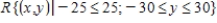
A)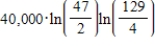
B)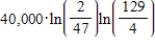
C)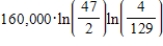
D)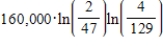
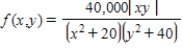
Where the origin (0, 0) gives the location of the government center. Find the population inside the rectangular area described by
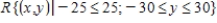
A)
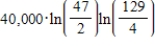
B)
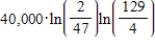
C)
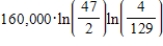
D)
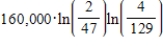

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
57
Evaluate the double integral 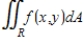 for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 4x + 8y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 4x + 8y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
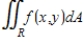 for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 4x + 8y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
for the given function f(x, y) and the region R. Enter your answer as a fraction. f(x, y) = 4x + 8y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
58
Find the average value of the given function
F(x, y)
Over the plane region R.
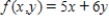 ; R is the triangle with vertices (0, 0), (2, 0) and (2, 2).
; R is the triangle with vertices (0, 0), (2, 0) and (2, 2).
A)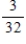
B)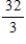
C)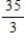
D)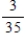
F(x, y)
Over the plane region R.
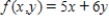 ; R is the triangle with vertices (0, 0), (2, 0) and (2, 2).
; R is the triangle with vertices (0, 0), (2, 0) and (2, 2).
A)
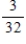
B)
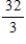
C)
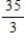
D)
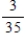

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
59
Evaluate the double integral
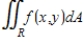
for the function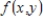 and the region R. Enter your answer as an expression.
and the region R. Enter your answer as an expression.
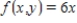 and R is bounded by
and R is bounded by 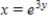 ,
,  ,
, 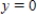 and
and 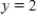 .
.
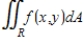
for the function
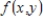 and the region R. Enter your answer as an expression.
and the region R. Enter your answer as an expression.
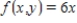 and R is bounded by
and R is bounded by 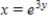 ,
,  ,
, 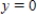 and
and 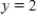 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
60
Evaluate the double integral
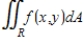
for the given function f(x, y) and the region R.
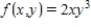 ; R is the rectangle defined by
; R is the rectangle defined by 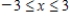 and
and 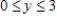 .
.
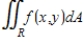
for the given function f(x, y) and the region R.
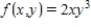 ; R is the rectangle defined by
; R is the rectangle defined by 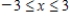 and
and 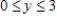 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
61
Find the total differential of the function. 
A)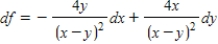
B)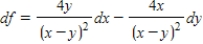
C)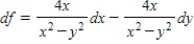
D)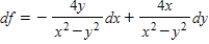
E)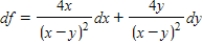

A)
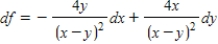
B)
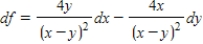
C)
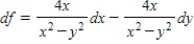
D)
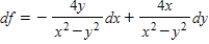
E)
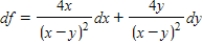

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
62
Find the total differential of the function. 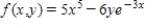
A)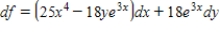
B)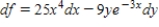
C)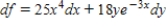
D)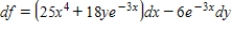
E)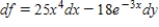
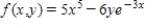
A)
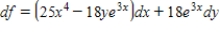
B)
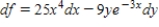
C)
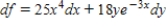
D)
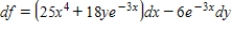
E)
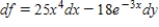

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
63
Find the approximate change in z when the point 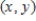 changes from
changes from  to
to 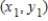 .
. 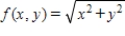 ; from
; from 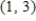 to
to 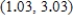
A) 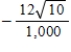
B) 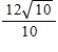
C) 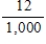
D) 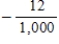
E)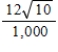
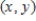 changes from
changes from  to
to 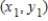 .
. 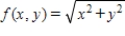 ; from
; from 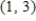 to
to 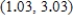
A)
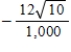
B)
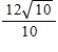
C)
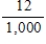
D)
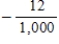
E)
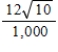

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
64
Find the average value of the given function
f(x, y)
over the plane region R. Enter your answer as a fraction.
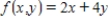 ; R is the triangle with vertices (0, 0), (5, 0) and (5, 5).
; R is the triangle with vertices (0, 0), (5, 0) and (5, 5).
f(x, y)
over the plane region R. Enter your answer as a fraction.
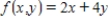 ; R is the triangle with vertices (0, 0), (5, 0) and (5, 5).
; R is the triangle with vertices (0, 0), (5, 0) and (5, 5).
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
65
Find the total differential of the function.
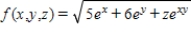
A)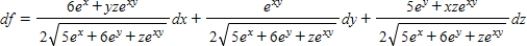
B)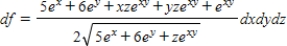
C)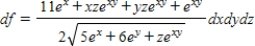
D)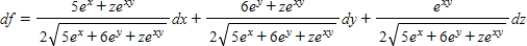
E)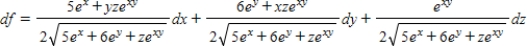
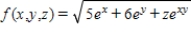
A)
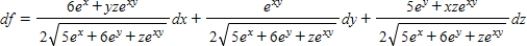
B)
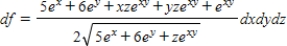
C)
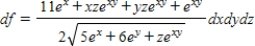
D)
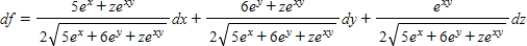
E)
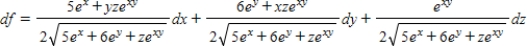

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
66
Find the volume of the solid bounded above by the surface
z = f(x, y)
and below by the plane region R. Enter your answer as an expression.
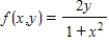 ; R is the region bounded by
; R is the region bounded by 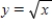 ,
, 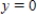 and
and 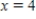 .
.
z = f(x, y)
and below by the plane region R. Enter your answer as an expression.
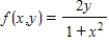 ; R is the region bounded by
; R is the region bounded by 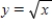 ,
, 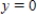 and
and 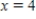 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
67
Find the volume of the solid bounded above by the surface
z = f(x, y)
and below by the plane region R. Enter your answer as an expression.
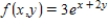 ; R is the triangle with vertices (0, 0), (4, 0) and (0, 4).
; R is the triangle with vertices (0, 0), (4, 0) and (0, 4).
z = f(x, y)
and below by the plane region R. Enter your answer as an expression.
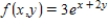 ; R is the triangle with vertices (0, 0), (4, 0) and (0, 4).
; R is the triangle with vertices (0, 0), (4, 0) and (0, 4).
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
68
Use a double integral to find the volume of the solid shown in the figure. Enter your answer as an expression.



Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
69
Find the total differential of the function. 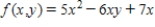
A)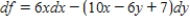
B)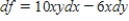
C)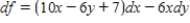
D)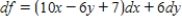
E)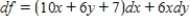
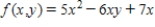
A)
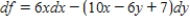
B)
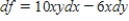
C)
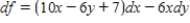
D)
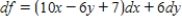
E)
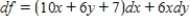

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
70
Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false.
If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then
![Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where .](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cade_26a1_b0a1_652ca1a399ab_TB8255_11.jpg) where
where ![Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where .](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cade_26a2_b0a1_bd6e5e1559fb_TB8255_11.jpg) .
.
If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then
![Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where .](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cade_26a1_b0a1_652ca1a399ab_TB8255_11.jpg) where
where ![Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false. If h(x, y) = f(x)g(y), where f is continuous on [a, b] and g is continuous on [c, d], then where .](https://d2lvgg3v3hfg70.cloudfront.net/TB8255/11eb651f_cade_26a2_b0a1_bd6e5e1559fb_TB8255_11.jpg) .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
71
Find the approximate change in z when the point 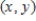 changes from
changes from 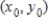 to
to 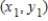 .
. 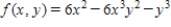 ; from
; from 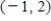 to
to 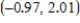
A) 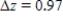
B) 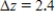
C) 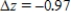
D) 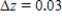
E)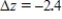
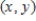 changes from
changes from 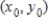 to
to 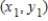 .
. 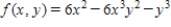 ; from
; from 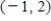 to
to 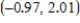
A)
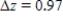
B)
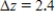
C)
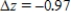
D)
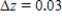
E)
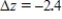

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
72
Find the approximate change in z when the point 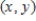 changes from
changes from 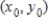 to
to 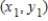 .
. 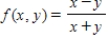 ; from
; from 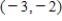 to
to 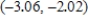
A) 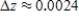
B)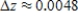
C) 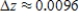
D) 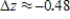
E) 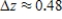
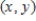 changes from
changes from 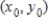 to
to 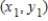 .
. 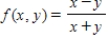 ; from
; from 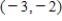 to
to 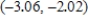
A)
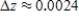
B)
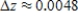
C)
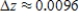
D)
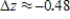
E)
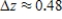

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
73
Evaluate the double integral
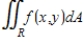
for the given function f(x, y) and the region R. Enter your answer as an expression.
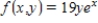 ; R is bounded by
; R is bounded by 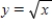 and y = x.
and y = x.
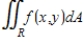
for the given function f(x, y) and the region R. Enter your answer as an expression.
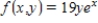 ; R is bounded by
; R is bounded by 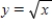 and y = x.
and y = x.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
74
Find the average value of the given function
f(x, y)
over the plane region R. Enter your answer as an expression.
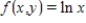 ; R is the region bounded by the graph of y = 2x and y = 0 from x = 1 to x = 7.
; R is the region bounded by the graph of y = 2x and y = 0 from x = 1 to x = 7.
f(x, y)
over the plane region R. Enter your answer as an expression.
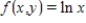 ; R is the region bounded by the graph of y = 2x and y = 0 from x = 1 to x = 7.
; R is the region bounded by the graph of y = 2x and y = 0 from x = 1 to x = 7.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
75
Use a double integral to find the volume of the solid shown in the figure. Enter your answer as a formula.
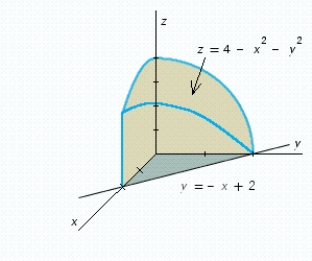


Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
76
Find the total differential of the function. 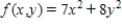
A)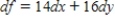
B)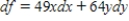
C)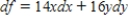
D)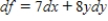
E)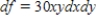
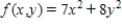
A)
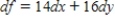
B)
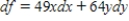
C)
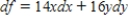
D)
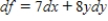
E)
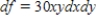

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
77
Find the total differential of the function. 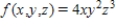
A)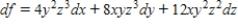
B)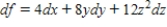
C)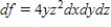
D)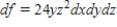
E)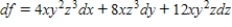
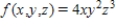
A)
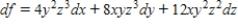
B)
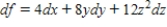
C)
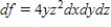
D)
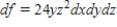
E)
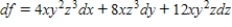

Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
78
Find the average value of the function
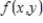
over the plane region R. Enter your answer as an expression.
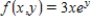 and R is the triangle with vertices
and R is the triangle with vertices 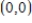 ,
, 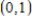 and
and 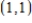 .
.
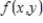
over the plane region R. Enter your answer as an expression.
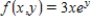 and R is the triangle with vertices
and R is the triangle with vertices 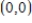 ,
, 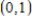 and
and 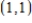 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
79
Find the volume of the solid bounded above by the surface
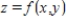
and below by the plane region R. Enter your answer as a formula.
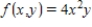 and R is the region bounded by the graphs of
and R is the region bounded by the graphs of  and
and 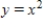 .
.
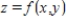
and below by the plane region R. Enter your answer as a formula.
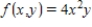 and R is the region bounded by the graphs of
and R is the region bounded by the graphs of  and
and 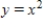 .
.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck
80
The population density of a certain city is given by the function
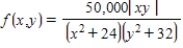
where the origin (0, 0) gives the location of the government center. Find the population inside the rectangular area described by
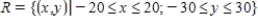 . Enter your answer as an expression.
. Enter your answer as an expression.
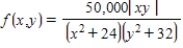
where the origin (0, 0) gives the location of the government center. Find the population inside the rectangular area described by
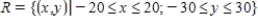 . Enter your answer as an expression.
. Enter your answer as an expression.
Unlock Deck
Unlock for access to all 219 flashcards in this deck.
Unlock Deck
k this deck



