Deck 5: Exponential and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/106
Play
Full screen (f)
Deck 5: Exponential and Logarithmic Functions
1
Match the function with one of the graphs.
A)
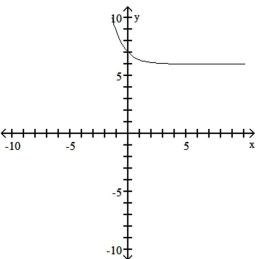
B)
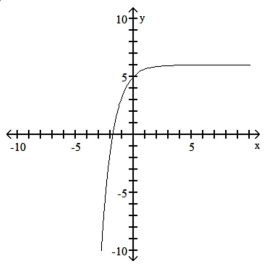
C)
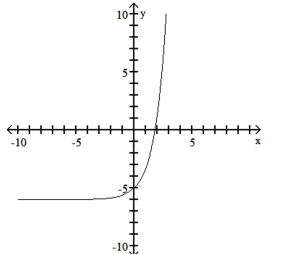
D)
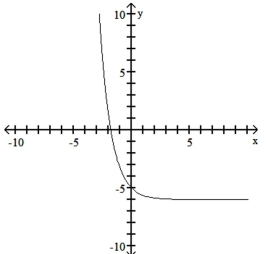
A)

B)

C)

D)

C
2
For the function f, use composition of functions to show that f
Answers may vary. One possible solution is: 1. of ;
2.
2.
3
Match the function with one of the graphs.
A)
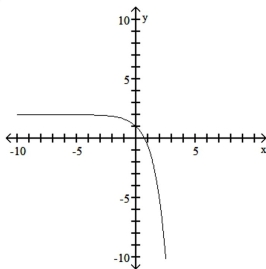
B)
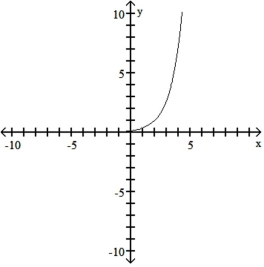
C)
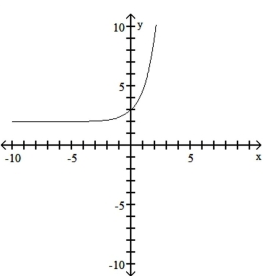
D)
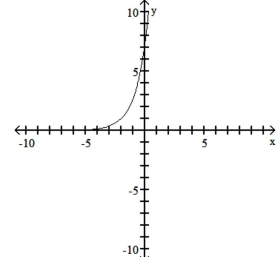
A)

B)

C)

D)

D
4
Choose the function that might be used as a model for the data in the scatter plot.
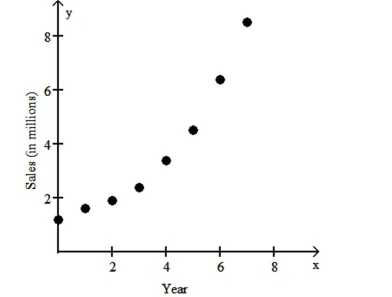
A) Logarithmic,
B) Exponential, or
C) Quadratic,
D) Exponential, or

A) Logarithmic,
B) Exponential, or
C) Quadratic,
D) Exponential, or

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
5
Choose the function that might be used as a model for the data in the scatter plot.
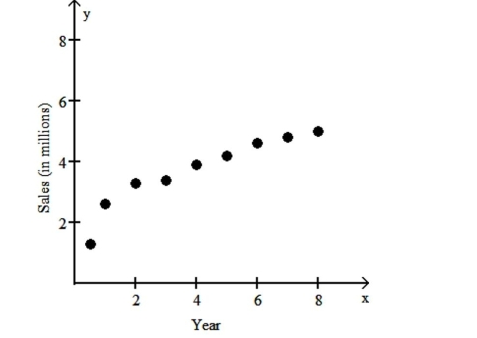
A) Logarithmic,
B) Exponential, or
C) Polynomial, not quadratic
D) Logistic,

A) Logarithmic,
B) Exponential, or
C) Polynomial, not quadratic
D) Logistic,

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
6
Choose the function that might be used as a model for the data in the scatter plot.
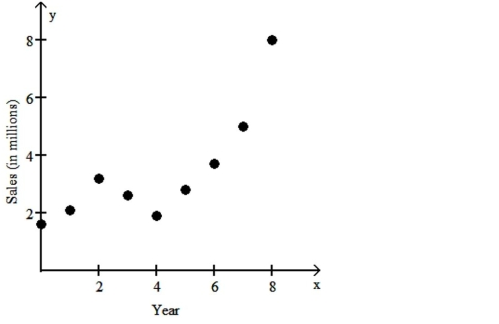
A) Logistic,
B) Exponential, or
C) Quadratic,
D) Polynomial, not quadratic

A) Logistic,
B) Exponential, or
C) Quadratic,
D) Polynomial, not quadratic

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
7
Match the function with one of the graphs.
A)
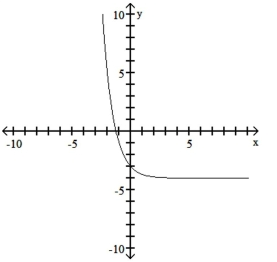
B)
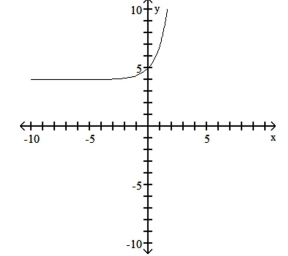
C)
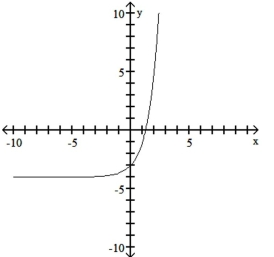
D)
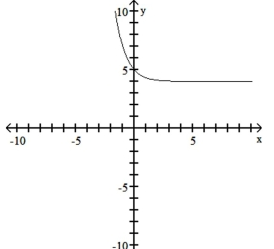
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
8
Provide an appropriate response.
Without using a calculator, determine which of these numbers is larger:
Without using a calculator, determine which of these numbers is larger:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
9
Choose the function that might be used as a model for the data in the scatter plot.
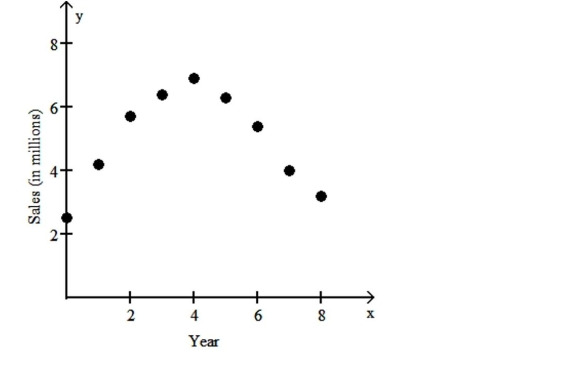
A) Polynomial, not quadratic
B) Logarithmic,
C) Quadratic,
D) Exponential, or

A) Polynomial, not quadratic
B) Logarithmic,
C) Quadratic,
D) Exponential, or

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
10
Match the function with one of the graphs.
A)
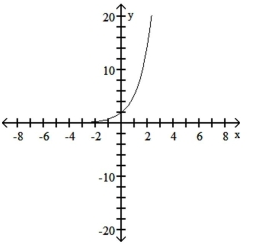
B)
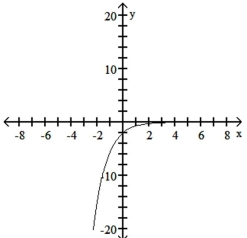
C)
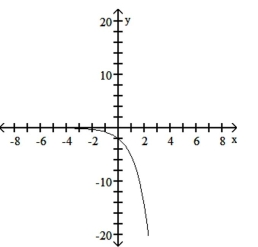
D)
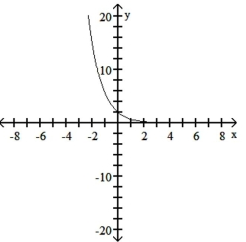
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
11
Match the function with one of the graphs.
A)
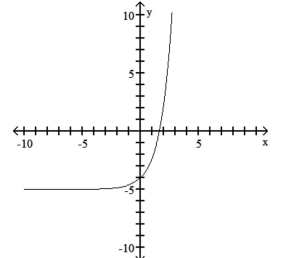
B)
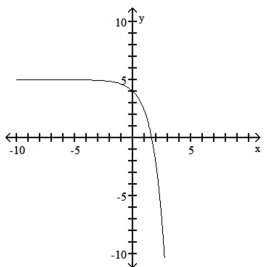
C)
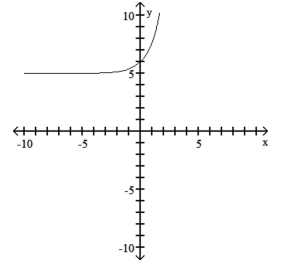
D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
12
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
13
Choose the function that might be used as a model for the data in the scatter plot.
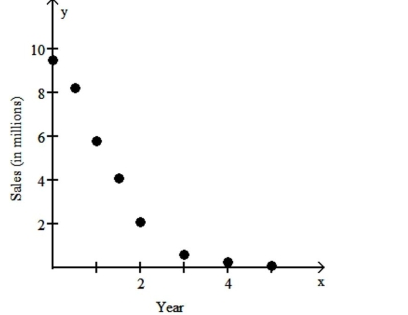
A) Logarithmic,
B) Polynomial, not quadratic
C) Exponential, or
D) Exponential, or

A) Logarithmic,
B) Polynomial, not quadratic
C) Exponential, or
D) Exponential, or

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
14
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
15
Match the function with one of the graphs.
A)
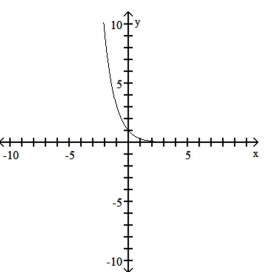
B)
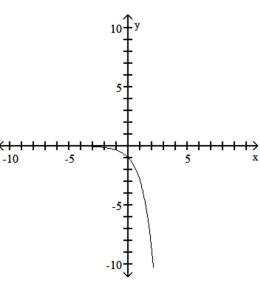
C)
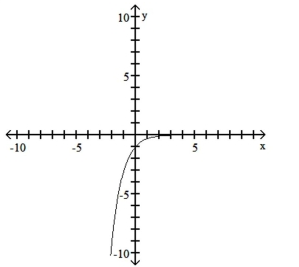
D)
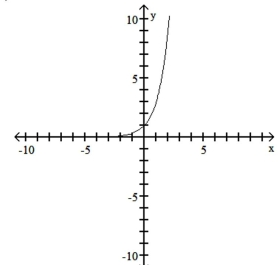
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
16
Choose the function that might be used as a model for the data in the scatter plot.
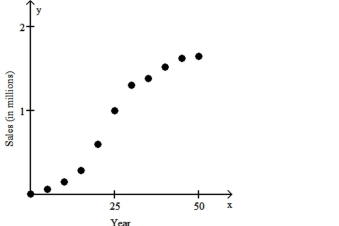
A) Polynomial, not quadratic
B) Exponential, or
C) Logistic,
D) Logarithmic,

A) Polynomial, not quadratic
B) Exponential, or
C) Logistic,
D) Logarithmic,

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
17
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
18
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
19
For the function f, use composition of functions to show that f

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
20
Provide an appropriate response.
Prove that the function f is one-to-one.
Prove that the function f is one-to-one.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
21
Provide an appropriate response.
Prove that the function f is not one-to-one.
Prove that the function f is not one-to-one.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
22
Provide an appropriate response.
Explain the error in the following:
Explain the error in the following:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
23
For the function f, use composition of functions to show that f

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
24
For the function f, use composition of functions to show that f

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
25
Provide an appropriate response.
Without using a calculator, determine which of these numbers is larger:
Without using a calculator, determine which of these numbers is larger:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
26
Provide an appropriate response.
Prove that the function f is one-to-one.
Prove that the function f is one-to-one.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
27
For the function f, use composition of functions to show that f

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
28
For the function f, use composition of functions to show that f

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
29
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
30
Provide an appropriate response.
Prove that the function f is not one-to-one.
Prove that the function f is not one-to-one.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
31
Provide an appropriate response.
Suppose that $1000 is invested for 5 years at 4% interest, compounded annually. In what
year will the most interest be earned? Why?
Suppose that $1000 is invested for 5 years at 4% interest, compounded annually. In what
year will the most interest be earned? Why?

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
32
For the function f, use composition of functions to show that f

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
33
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
34
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
35
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
36
Provide an appropriate response.
Prove that the function f is one-to-one.
Prove that the function f is one-to-one.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
37
Provide an appropriate response.
Explain why 1 is excluded from being a logarithmic base.
Explain why 1 is excluded from being a logarithmic base.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
38
For the function f, use composition of functions to show that f

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
39
Provide an appropriate response.
Prove that the function f is not one-to-one.
Prove that the function f is not one-to-one.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
40
Provide an appropriate response.
Prove that the function f is not one-to-one.
Prove that the function f is not one-to-one.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
41
Provide an appropriate response.
Prove that the function f is one-to-one.
Prove that the function f is one-to-one.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
42
Using the horizontal-line test, determine whether the function is one-to-one.
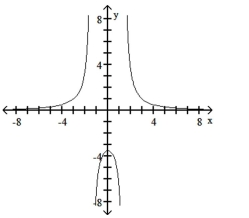
A) Yes
B)

A) Yes
B)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
43
Find the logarithm using the change-of-base formula.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
44
Provide an appropriate response.
The product, power, and quotient rules enable us to simplify expressions like . Explain why such expressions can always be simplified without using the quotient rule.
The product, power, and quotient rules enable us to simplify expressions like . Explain why such expressions can always be simplified without using the quotient rule.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
45
Find the inverse of the relation.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
46
Provide an appropriate response.
Explain why negative numbers do not have logarithms.
Explain why negative numbers do not have logarithms.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
47
Sketch the graph of the function. Describe how the graph can be obtained from the graph of a basic logarithmic function.
A) Shift to the left 2 units,
stretch it vertically, and shift up 4 units
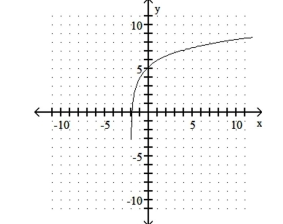
B) Shift to the right 2 units
,shrink it vertically, and shift up 4 units
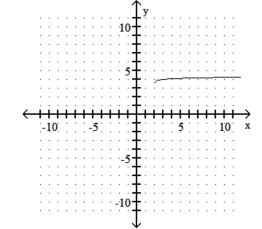
C) Shift to the left 2 units,
shrink it vertically, and shift down 4 units
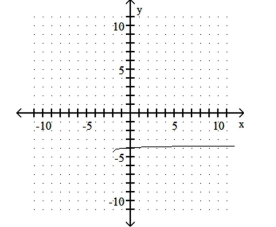
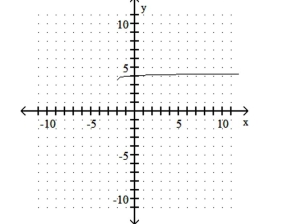
D) Shift to the left 2 units,
shrink it vertically, and shift up 4 units

A) Shift to the left 2 units,
stretch it vertically, and shift up 4 units

B) Shift to the right 2 units
,shrink it vertically, and shift up 4 units

C) Shift to the left 2 units,
shrink it vertically, and shift down 4 units

D) Shift to the left 2 units,
shrink it vertically, and shift up 4 units


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
48
Find the domain and range of the inverse of the given function.
A) Domain: ; range:
B) Domain and range: all real numbers
C) Domain: ; range:
D) Domain: all real numbers; range:
A) Domain: ; range:
B) Domain and range: all real numbers
C) Domain: ; range:
D) Domain: all real numbers; range:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
49
Sketch the graph of the function. Describe how the graph can be obtained from the graph of a basic logarithmic function.
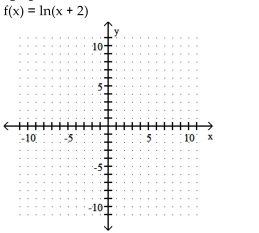 A) Shift right 2 units
A) Shift right 2 units
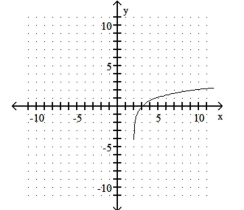
B) Shift left 2 units
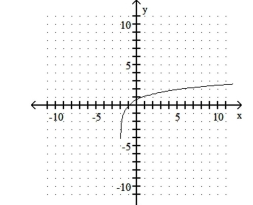
C) Shift right 2 units
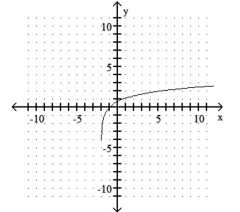
D) Shift left 2 units
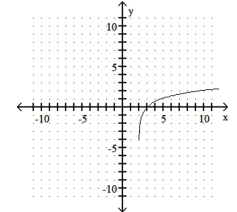
 A) Shift right 2 units
A) Shift right 2 units
B) Shift left 2 units

C) Shift right 2 units

D) Shift left 2 units


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
50
Graph the function.
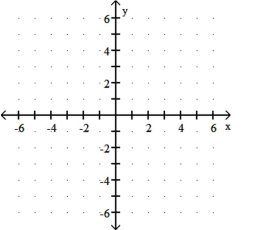
A)
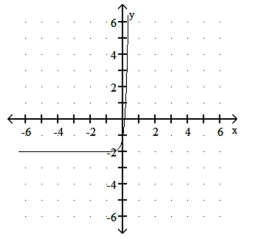
B)
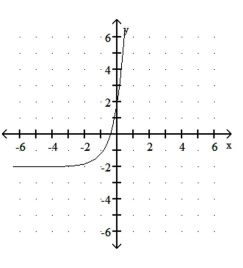
C)
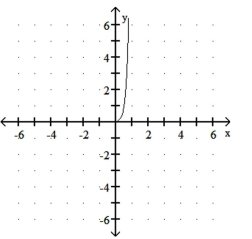
D)
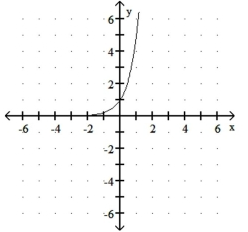

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
51
Provide an appropriate response.

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
52
Solve.
In 1985, the number of female athletes participating in Summer Olympic-Type Games was 450. In 1996, about 3650 participated in the Summer Olympics in Atlanta. Assuming that P(0)= 500 and
That the exponential model applies, find the value of k rounded to the hundredths place, and write
The function. A)
B)
C)
D)
In 1985, the number of female athletes participating in Summer Olympic-Type Games was 450. In 1996, about 3650 participated in the Summer Olympics in Atlanta. Assuming that P(0)= 500 and
That the exponential model applies, find the value of k rounded to the hundredths place, and write
The function. A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
53
Simplify.
A) 5
B)
C) 3
D)
A) 5
B)
C) 3
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
54
Solve the logarithmic equation.
A)
B)
C)
D) 25
A)
B)
C)
D) 25

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
55
Express as a single logarithm and, if possible, simplify.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
56
Solve.
How long will it take for to grow to at an interest rate of if the interest is compounded continuously? Round the number of years to the nearest hundredth.
A)
B)
C)
D)
How long will it take for to grow to at an interest rate of if the interest is compounded continuously? Round the number of years to the nearest hundredth.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
57
Convert to an exponential equation.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
58
Solve the logarithmic equation.
A)
B) 256
C) 65,536
D) 4
A)
B) 256
C) 65,536
D) 4

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
59
Find the domain and the vertical asymptote of the function.
A) Domain: ; vertical asymptote:
B) Domain: ; vertical asymptote: none
C) Domain: ; vertical asymptote:
D) Domain: ; vertical asymptote:
A) Domain: ; vertical asymptote:
B) Domain: ; vertical asymptote: none
C) Domain: ; vertical asymptote:
D) Domain: ; vertical asymptote:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
60
Determine whether the given function is one-to-one. If it is one-to-one, find a formula for the inverse.
A)
B)
C)
D) Not one-to-one
A)
B)
C)
D) Not one-to-one

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
61
Find the following using a calculator. Round to four decimal places.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
62
Express as a difference of logarithms.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
63
Graph the piecewise function.
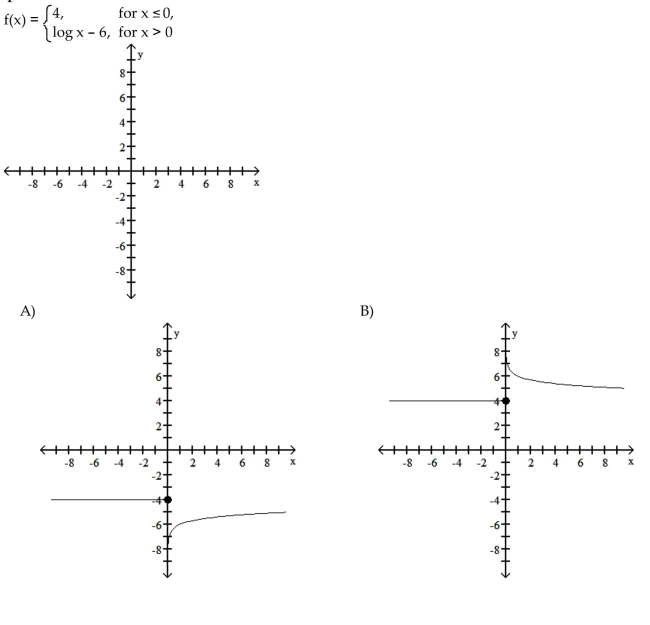
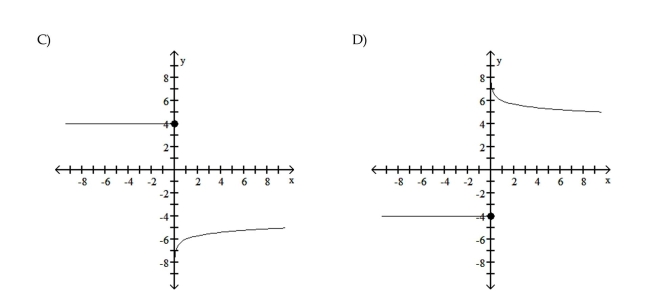



Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
64
Solve.
Given and , evaluate .
A)
B)
C)
D)
Given and , evaluate .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
65
Evaluate to four decimal places using a calculator.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
66
Solve the problem.
Suppose the amount of a radioactive element remaining in a sample of 100 milligrams after years can be described by . How much is remaining after 58 years? Round the answer to the nearest hundredth of a milligram.
A)
B)
C)
D)
Suppose the amount of a radioactive element remaining in a sample of 100 milligrams after years can be described by . How much is remaining after 58 years? Round the answer to the nearest hundredth of a milligram.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
67
Convert to a logarithmic equation.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
68
Graph the function.
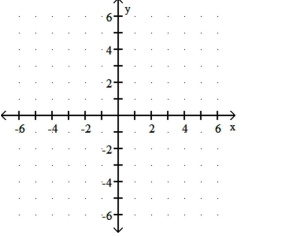
A)
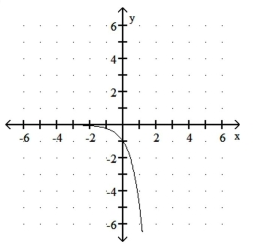
B)
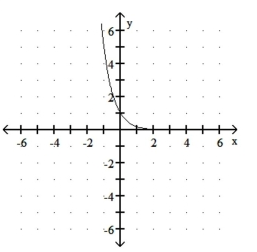
C)
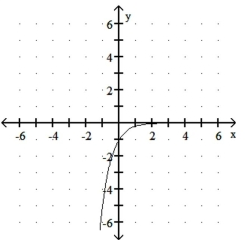
D)
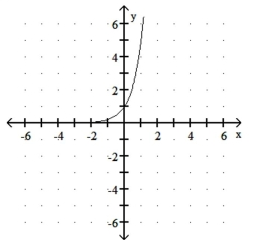

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
69
Convert to a logarithmic equation.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
70
Graph the function.
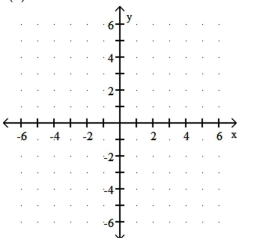
A)
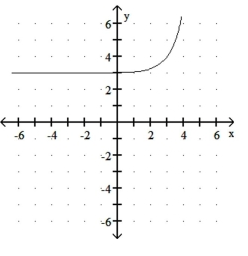
B)
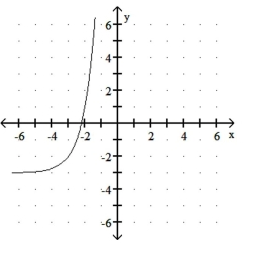
C)
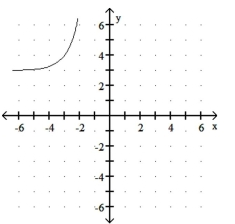
D)
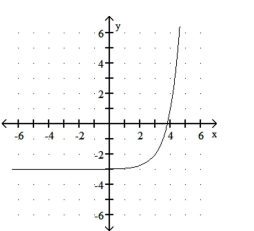

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
71
Graph the function.
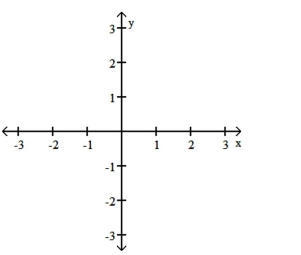
A)
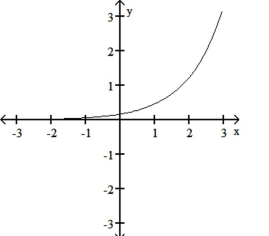
B)
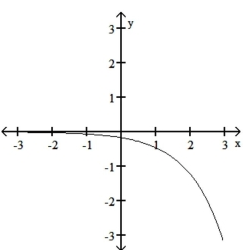
C)
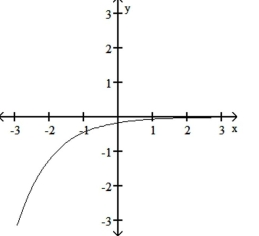
D)
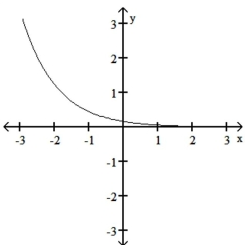

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
72
Graph the function. Describe its position relative to the graph of the indicated basic function.
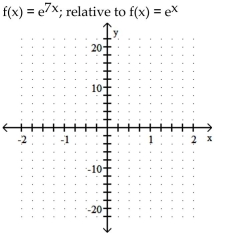 A) Shrunk vertically
A) Shrunk vertically
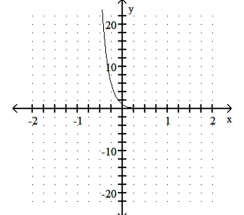
B) Shrunk horizontally
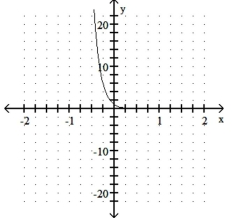
C) Shrunk vertically
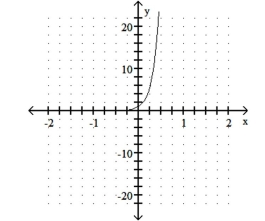
D) Shrunk horizontally
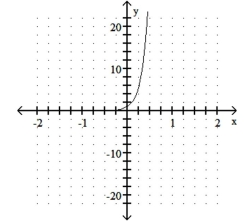
 A) Shrunk vertically
A) Shrunk vertically
B) Shrunk horizontally

C) Shrunk vertically

D) Shrunk horizontally


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
73
The graph of a one-to-one function f is given. Sketch the graph of the inverse function , on the same set of axes. Use a
dashed line for the inverse.
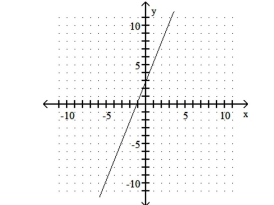
A)
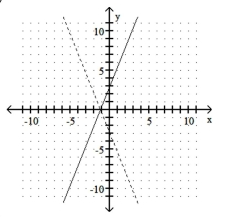
B)
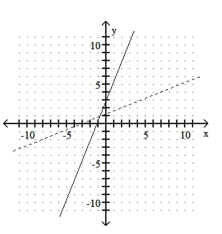
C)
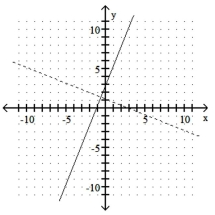
D)
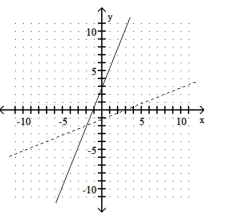
dashed line for the inverse.

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
74
Find the domain and range of the inverse of the given function.
A) Domain: ; range: all real numbers
B) Domain and range: all real numbers
C) Domain: ; range:
D) Domain: ; range:
A) Domain: ; range: all real numbers
B) Domain and range: all real numbers
C) Domain: ; range:
D) Domain: ; range:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
75
Find the domain and the vertical asymptote of the function.
A) Domain ; vertical asymptote:
B) Domain ; vertical asymptote:
C) Domain ; vertical asymptote:
D) Domain ; vertical asymptote:
A) Domain ; vertical asymptote:
B) Domain ; vertical asymptote:
C) Domain ; vertical asymptote:
D) Domain ; vertical asymptote:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
76
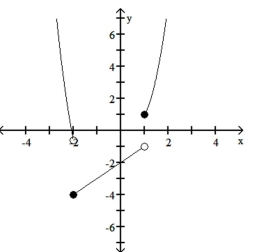
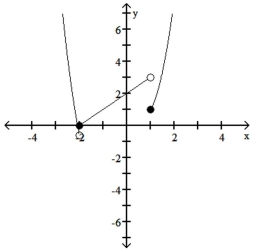
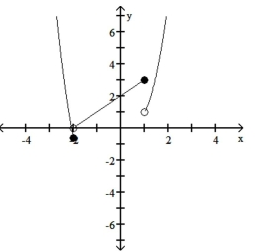
Graph the piecewise function.
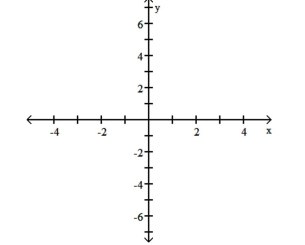
A)
B)
C)
D)
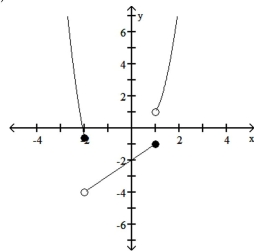

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
77
Solve the problem.
An initial investment of $14,000 is appreciated for 4 years in an account that earns 13% interest, compounded semiannually. Find the amount of money in the account at the end of the period.
A)$22,826.63
B)$23,169.94
C)$9169.94
D)$21,755.81
An initial investment of $14,000 is appreciated for 4 years in an account that earns 13% interest, compounded semiannually. Find the amount of money in the account at the end of the period.
A)$22,826.63
B)$23,169.94
C)$9169.94
D)$21,755.81

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
78
Solve.
Find the hydrogen ion concentration of a solution whose is . Use the formula .
A)
B)
C)
D)
Find the hydrogen ion concentration of a solution whose is . Use the formula .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
79
Solve the problem.
In September 1998 the population of the country of West Goma in millions was modeled by . At the same time the population of East Goma in millions was modeled by . In both formulas is the year, where corresponds to September Assuming these trends continue, estimate the year when the population of West Goma will equal the population of East Goma.
A) 1980
B) 18
C) 2013
D) 2016
In September 1998 the population of the country of West Goma in millions was modeled by . At the same time the population of East Goma in millions was modeled by . In both formulas is the year, where corresponds to September Assuming these trends continue, estimate the year when the population of West Goma will equal the population of East Goma.
A) 1980
B) 18
C) 2013
D) 2016

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck
80
Find the domain and the vertical asymptote of the function.
A) Domain ; vertical asymptote:
B) Domain ; vertical asymptote:
C) Domain: ; vertical asymptote:
D) Domain ; vertical asymptote:
A) Domain ; vertical asymptote:
B) Domain ; vertical asymptote:
C) Domain: ; vertical asymptote:
D) Domain ; vertical asymptote:

Unlock Deck
Unlock for access to all 106 flashcards in this deck.
Unlock Deck
k this deck



