Deck 1: Functions and Their Graphs
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/301
Play
Full screen (f)
Deck 1: Functions and Their Graphs
1
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function
function
2
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function
function
3
Determine whether the relation represents a function. If it is a function, state the domain and range.
-
A) function
domain:
range:
B) function
domain:
range:
C)
-
A) function
domain:
range:
B) function
domain:
range:
C)
4
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
5
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
6
Determine whether the relation represents a function. If it is a function, state the domain and range.
-
A) function
domain:
range:
B) function
domain:
range:
C)
-
A) function
domain:
range:
B) function
domain:
range:
C)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
7
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
8
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
9
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
10
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
11
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
12
Determine whether the relation represents a function. If it is a function, state the domain and range.
-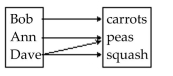
A)
function
B)
function
C)
-

A)
function
B)
function
C)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
13
Determine whether the relation represents a function. If it is a function, state the domain and range.
-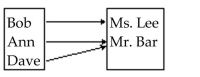
A) function
domain: {Ms. Lee, Mr. Bar}
range: Bob, Ann, Dave
B) function
domain: {Bob, Ann, Dave}
range:{Ms. Lee, Mr. Bar}
C)
-

A) function
domain: {Ms. Lee, Mr. Bar}
range: Bob, Ann, Dave
B) function
domain: {Bob, Ann, Dave}
range:{Ms. Lee, Mr. Bar}
C)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
14
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
15
Determine whether the relation represents a function. If it is a function, state the domain and range.
-
A) function
domain:
range:
B) function
domain:
range:
C)
-
A) function
domain:
range:
B) function
domain:
range:
C)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
16
Determine whether the relation represents a function. If it is a function, state the domain and range.
-
A) function
domain:
range:
B) function
domain:
range:
C)
-
A) function
domain:
range:
B) function
domain:
range:
C)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
17
Determine whether the relation represents a function. If it is a function, state the domain and range.
-
A) function
domain:
range:
B) function
domain:
range:
C)
-
A) function
domain:
range:
B) function
domain:
range:
C)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
18
Find the value for the function.
-
A) -3
B) 35
C) 3
D) 29
-
A) -3
B) 35
C) 3
D) 29

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
19
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
20
Determine whether the equation defines y as a function of x.
-
A) function
B) not a function
-
A) function
B) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
21
Find the value for the function.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
22
Solve the problem.
-If a rock falls from a height of 70 meters on Earth, the height H (in meters) after x seconds is approximately When does the rock strike the ground? Round to the nearest hundredth, if necessary.
A) 14.29 sec
B) 2.92 sec
C) 3.78 sec
D) 1.71 sec
-If a rock falls from a height of 70 meters on Earth, the height H (in meters) after x seconds is approximately When does the rock strike the ground? Round to the nearest hundredth, if necessary.
A) 14.29 sec
B) 2.92 sec
C) 3.78 sec
D) 1.71 sec

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
23
Find the value for the function.
-Find when
A)
B)
C)
D)
-Find when
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
24
Find the value for the function.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
25
Find the value for the function.
-Find when
A)
B)
C)
D)
-Find when
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
26
Find the value for the function.
-Find when .
A)
B)
C)
D)
-Find when .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
27
Find the value for the function.
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
28
Find the value for the function.
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
29
Solve the problem.
-If , and is undefined, what are the values of and ?
A)
B)
C)
D)
-If , and is undefined, what are the values of and ?
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
30
Find the domain of the function.
-
A)
B)
C)
D) all real numbers
-
A)
B)
C)
D) all real numbers

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
31
Find the value for the function.
-
A) 9
B)
C)
D) 1
-
A) 9
B)
C)
D) 1

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
32
Solve the problem.
-If and , what is the value of ?
A)
B)
C)
D)
-If and , what is the value of ?
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
33
Find the value for the function.
-
A) 15
B)-15
C) -3
D)3
-
A) 15
B)-15
C) -3
D)3

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
34
Find the value for the function.
-Find when
A)
B)
C)
D)
-Find when
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
35
Solve the problem.
-If and , what is the value of ?
A)
B)
C)
D)
-If and , what is the value of ?
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
36
Find the value for the function.
-Find when
A)
B)
C)
D)
-Find when
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
37
Find the value for the function.
-
A)
B) 0
C) 2
D)
-
A)
B) 0
C) 2
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
38
Find the value for the function.
-Find when
A)
B)
C)
D)
-Find when
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
39
Solve the problem.
-If a rock falls from a height of 80 meters on Earth, the height H (in meters) after x seconds is approximately What is the height of the rock when x = 1.5 seconds? Round to the nearest hundredth, if necessary.
A) 72.65 m
B) 68.98 m
C) 91.03 m
D) 69.2 m
-If a rock falls from a height of 80 meters on Earth, the height H (in meters) after x seconds is approximately What is the height of the rock when x = 1.5 seconds? Round to the nearest hundredth, if necessary.
A) 72.65 m
B) 68.98 m
C) 91.03 m
D) 69.2 m

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
40
Find the domain of the function.
-
A)
B)
C) all real numbers
D)
-
A)
B)
C) all real numbers
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
41
For the given functions f and g, find the requested function and state its domain.
-
Find .
A)
B) ; all real numbers
C) ; all real numbers
D) ; all real numbers
-
Find .
A)
B) ; all real numbers
C) ; all real numbers
D) ; all real numbers

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
42
Solve the problem.
Jacey, a commissioned salesperson, earns $140 base pay plus $35 per item sold. Express Jacey's gross salary G as a function of the number x of items sold.
A) G(x) = 35(x + 140)
B) G(x) = 35x + 140
C) G(x) = 140(x + 35)
D) G(x) = 140x +35
Jacey, a commissioned salesperson, earns $140 base pay plus $35 per item sold. Express Jacey's gross salary G as a function of the number x of items sold.
A) G(x) = 35(x + 140)
B) G(x) = 35x + 140
C) G(x) = 140(x + 35)
D) G(x) = 140x +35

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
43
For the given functions f and g, find the requested function and state its domain.
-
Find .
A) ; all real numbers
B)
C)
D) ; all real numbers
-
Find .
A) ; all real numbers
B)
C)
D) ; all real numbers

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
44
Find the domain of the function.
-
A)
B)
C)
D)
-
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
45
For the given functions f and g, find the requested function and state its domain.
-
Find .
A)
B)
C)
D)
-
Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
46
For the given functions f and g, find the requested function and state its domain.
-
Find .
A)
B)
C) ; all real numbers
D) ; all real numbers
-
Find .
A)
B)
C) ; all real numbers
D) ; all real numbers

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
47
Find and simplify the difference quotient of f, , for the function.
-f(x) = 5x - 8
A) 0
B)
C) 5
D)
-f(x) = 5x - 8
A) 0
B)
C) 5
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
48
For the given functions f and g, find the requested function and state its domain.
-
Find .
A)
B)
C)
D)
-
Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
49
For the given functions f and g, find the requested function and state its domain.
-
Find
A) ; all real numbers
B) ; all real numbers
C)
D)
-
Find
A) ; all real numbers
B) ; all real numbers
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
50
Solve the problem.
-Given and , find the function .
A)
B)
C)
D)
-Given and , find the function .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
51
Find the domain of the function.
-
A)
B)
C) all real numbers
D)
-
A)
B)
C) all real numbers
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
52
For the given functions f and g, find the requested function and state its domain.
-
Find .
A)
B)
C)
D)
-
Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
53
Find the domain of the function.
-
A)
B)
C) all real numbers
D)
-
A)
B)
C) all real numbers
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
54
For the given functions f and g, find the requested function and state its domain.
-
Find .
A) ; all real numbers
B) ; all real numbers
C) ; all real numbers
D)
-
Find .
A) ; all real numbers
B) ; all real numbers
C) ; all real numbers
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
55
Find the domain of the function.
-
A)
B)
C)
D) all real numbers
-
A)
B)
C)
D) all real numbers

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
56
For the given functions f and g, find the requested function and state its domain.
-
Find f
A)
B) ; all real numbers
C) ; all real numbers
D)
-
Find f
A)
B) ; all real numbers
C) ; all real numbers
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
57
For the given functions f and g, find the requested function and state its domain.
-
Find .
A)
B)
C)
D)
-
Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
58
Find the domain of the function.
-
A) all real numbers
B)
C)
D)
-
A) all real numbers
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
59
For the given functions f and g, find the requested function and state its domain.
-
Find .
A)
B)
C)
D)
-
Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
60
Solve the problem.
-Express the gross salary G of a person who earns $35 per hour as a function of the number x of hours worked.
A)
B)
C)
D)
-Express the gross salary G of a person who earns $35 per hour as a function of the number x of hours worked.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
61
Solve the problem.
A retail store buys 120 VCRs from a distributor at a cost of $200 each plus an overhead charge of $35 per order. The retail markup is 30% on the total price paid. Find the profit on the sale of one VCR.
A) $6,009.00
B) $60.00
C) $59.91
D) $60.09
A retail store buys 120 VCRs from a distributor at a cost of $200 each plus an overhead charge of $35 per order. The retail markup is 30% on the total price paid. Find the profit on the sale of one VCR.
A) $6,009.00
B) $60.00
C) $59.91
D) $60.09

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
62
Find and simplify the difference quotient of f, , for the function.
-
A)
function
domain:
range:
intercepts:
symmetry: -axis, -axis
B)
function
domain:
range:
intercepts:
symmetry: -axis, -axis, origin
C)
function
domain:
range:
intercepts:
symmetry: origin
D)not a function
-

A)
function
domain:
range:
intercepts:
symmetry: -axis, -axis
B)
function
domain:
range:
intercepts:
symmetry: -axis, -axis, origin
C)
function
domain:
range:
intercepts:
symmetry: origin
D)not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
63
Find and simplify the difference quotient of f, , for the function.
-
A)
B)
C) 0
D)
-
A)
B)
C) 0
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
64
The graph of a function f is given. Use the graph to answer the question.
-For what numbers is ?

A)
B)
C)
D)
-For what numbers is ?

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
65
The graph of a function f is given. Use the graph to answer the question.
Is f(3) positive or negative?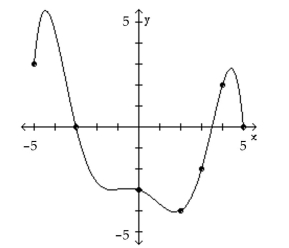
A) positive
B) negative
Is f(3) positive or negative?

A) positive
B) negative

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
66
Find and simplify the difference quotient of f, , for the function.
-
A)
B) 1
C)
D)
-
A)
B) 1
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
67
Find and simplify the difference quotient of f, , for the function.
-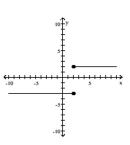
A)
B)
C)
function
domain: all real numbers
range: or
intercept:
symmetry: none
D)
not a function
-

A)
B)
C)
function
domain: all real numbers
range: or
intercept:
symmetry: none
D)
not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
68
Find and simplify the difference quotient of f, , for the function.
-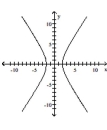
A)
function
domain: all real numbers
range: \( \{y \mid y \leq-2 \) or \( y \geq 2\} \)
intercepts: \( (-2,0),(2,0) \)
symmetry: \( y \)-axis\[\begin{array} { l l }
B)
function
domain: \( \{x \mid-2 \leq x \leq 2\} \)
range: all real numbers
intercepts: \( (-2,0),(2,0) \)
symmetry: \( \mathrm{x} \)-axis, \( y \)-axis
C)
function
domain: \( \{x \mid x \leq-2 \) or \( x \geq 2\} \)
range: all real numbers
intercepts: \( (-2,0),(2,0) \)
symmetry: \( \mathrm{x} \)-axis, \( \mathrm{y} \)-axis, origirn
D)
\(\text { not a function }\)
-

A)
function
domain: all real numbers
range: \( \{y \mid y \leq-2 \) or \( y \geq 2\} \)
intercepts: \( (-2,0),(2,0) \)
symmetry: \( y \)-axis\[\begin{array} { l l }
B)
function
domain: \( \{x \mid-2 \leq x \leq 2\} \)
range: all real numbers
intercepts: \( (-2,0),(2,0) \)
symmetry: \( \mathrm{x} \)-axis, \( y \)-axis
C)
function
domain: \( \{x \mid x \leq-2 \) or \( x \geq 2\} \)
range: all real numbers
intercepts: \( (-2,0),(2,0) \)
symmetry: \( \mathrm{x} \)-axis, \( \mathrm{y} \)-axis, origirn
D)
\(\text { not a function }\)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
69
The graph of a function f is given. Use the graph to answer the question.
Use the graph of f given below to find f(10).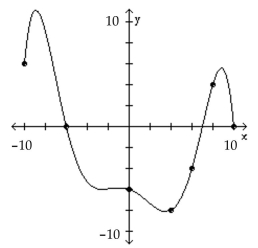
A) 12
B) 10
C) 20
D) 0
Use the graph of f given below to find f(10).

A) 12
B) 10
C) 20
D) 0

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
70
The graph of a function f is given. Use the graph to answer the question.
Is f(8) positive or negative?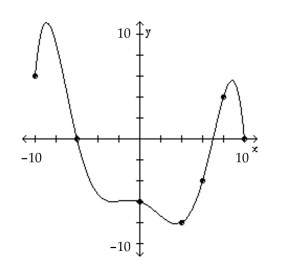
A) positive
B) negative
Is f(8) positive or negative?

A) positive
B) negative

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
71
The graph of a function f is given. Use the graph to answer the question.
-For what numbers x is f(x) > 0?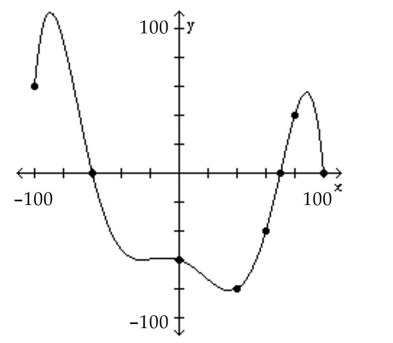
A)
B)
C)
D)
-For what numbers x is f(x) > 0?

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
72
The graph of a function f is given. Use the graph to answer the question.
-For what numbers x is f(x) = 0?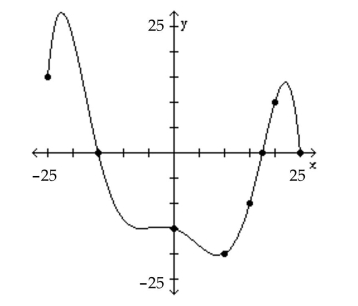
A)
B)
C)
D)
-For what numbers x is f(x) = 0?

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
73
The graph of a function f is given. Use the graph to answer the question.
-What is the y-intercept?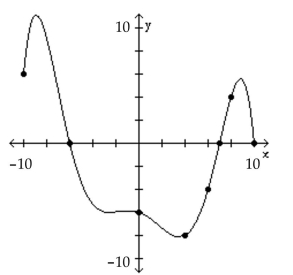
A)
B) 10
C) 7
D)
-What is the y-intercept?

A)
B) 10
C) 7
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
74
Find and simplify the difference quotient of f, , for the function.
-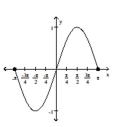
A)
B)
function
domain: all real numbers
range:
intercepts:
symmetry: origin
C)
function
domain:
range:
intercepts:
symmetry: none
D) not function
-

A)
B)
function
domain: all real numbers
range:
intercepts:
symmetry: origin
C)
function
domain:
range:
intercepts:
symmetry: none
D) not function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
75
The graph of a function f is given. Use the graph to answer the question.
-What are the x-intercepts?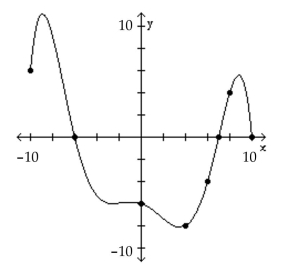
A)
B)
C)
D)
-What are the x-intercepts?

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
76
Find and simplify the difference quotient of f, , for the function.
-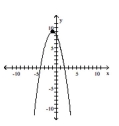
A)
B)
function
domain:
range: all real numbers
intercepts:
symmetry: -axis
C)
function
domain: all real numbers
range:
intercepts:
symmetry: none
D) not function
-

A)
B)
function
domain:
range: all real numbers
intercepts:
symmetry: -axis
C)
function
domain: all real numbers
range:
intercepts:
symmetry: none
D) not function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
77
Solve the problem.
-Suppose that P(x) represents the percentage of income spent on food in year x and I(x) represents income in year x. Determine a function F that represents total food expenditures in year x.
A)
B)
C)
D)
-Suppose that P(x) represents the percentage of income spent on food in year x and I(x) represents income in year x. Determine a function F that represents total food expenditures in year x.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
78
Find and simplify the difference quotient of f, , for the function.
-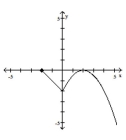
A)
B)
function
domain:
range:
intercepts:
symmetry: none
C)
function
domain: all real numbers
range: all real numbers
intercepts:
symmetry: none
D) not a function
-

A)
B)
function
domain:
range:
intercepts:
symmetry: none
C)
function
domain: all real numbers
range: all real numbers
intercepts:
symmetry: none
D) not a function

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
79
Find and simplify the difference quotient of f, , for the function.
-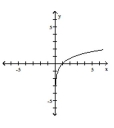
A)
B)
C)
D)
-

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck
80
The graph of a function f is given. Use the graph to answer the question.
-What is the domain of f?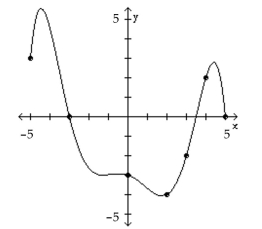
A)
B) all real numbers
C)
D)
-What is the domain of f?

A)
B) all real numbers
C)
D)

Unlock Deck
Unlock for access to all 301 flashcards in this deck.
Unlock Deck
k this deck



