Deck 13: A Preview of Calculus: the Limit, Derivative, and Integral of a Function
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/145
Play
Full screen (f)
Deck 13: A Preview of Calculus: the Limit, Derivative, and Integral of a Function
1
Use the graph shown to determine if the limit exists. If it does, find its value.
-
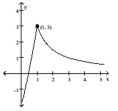
A) 1
B) 3
C) 0
D) does not exist
-

A) 1
B) 3
C) 0
D) does not exist
3
2
Use the TABLE feature of a graphing utility to find the limit.
-
A) 2
B) 0
C) 1
D) does not exist
-
A) 2
B) 0
C) 1
D) does not exist
0
3
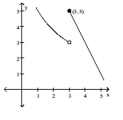
Use the grid to graph the function. Find the limit, if it exists
-
A) 1
B) -2
C) -8
D) does not exist
-

A) 1
B) -2
C) -8
D) does not exist
-8
4
Find the limit algebraically.
-
A) -6
B) 1
C) 0
D) 6
-
A) -6
B) 1
C) 0
D) 6

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
5
Use the graph shown to determine if the limit exists. If it does, find its value.
-
A) 4
B) 3
C) 5
D) does not exist
-

A) 4
B) 3
C) 5
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
6
Use the grid to graph the function. Find the limit, if it exists
-
A) -2
B) -6
C) -8
D) does not exist
-

A) -2
B) -6
C) -8
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
7
Use the TABLE feature of a graphing utility to find the limit.
-
A) 2
B) -2
C) 0
D) does not exist
-
A) 2
B) -2
C) 0
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
8
Use the TABLE feature of a graphing utility to find the limit.
-
A) -1
B) 0
C) 1
D) does not exist
-
A) -1
B) 0
C) 1
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
9
Use the grid to graph the function. Find the limit, if it exists
-
A) 0
B) 1
C) 5
D) does not exist
-
A) 0
B) 1
C) 5
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
10
Use the grid to graph the function. Find the limit, if it exists
-
A) -1
B) 3
C) -2
D) does not exist
-
A) -1
B) 3
C) -2
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
11
Use the grid to graph the function. Find the limit, if it exists
-
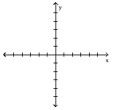
A) 12
B) 11
C) 2
D) does not exist
-

A) 12
B) 11
C) 2
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
12
Use the graph shown to determine if the limit exists. If it does, find its value.
-
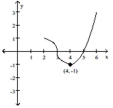
A) 1
B) -1
C) 4
D) does not exist
-

A) 1
B) -1
C) 4
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
13
Find the limit algebraically.
-
A) -18
B) 9
C) 3
D) does not exist
-
A) -18
B) 9
C) 3
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
14
Use the TABLE feature of a graphing utility to find the limit.
-
A)
B) 0
C) 1
D) does not exist
-
A)
B) 0
C) 1
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
15
Use the TABLE feature of a graphing utility to find the limit.
-
A) 27
B) 9
C) 0
D) does not exist
-
A) 27
B) 9
C) 0
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
16
Use a graphing utility to find the indicated limit rounded to two decimal places.
-
A) 2.04
B) 2.96
C) 3.00
D) 2.00
-
A) 2.04
B) 2.96
C) 3.00
D) 2.00

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
17
Use the TABLE feature of a graphing utility to find the limit.
-
A) 0
B) -18
C) 18
D) does not exist
-
A) 0
B) -18
C) 18
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
18
Use the grid to graph the function. Find the limit, if it exists
-
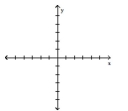
A) -5
B) 1
C) 5
D) does not exist
-

A) -5
B) 1
C) 5
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
19
Use the graph shown to determine if the limit exists. If it does, find its value.
-
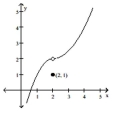
A) 0
B) 1
C) 2
D) does not exist
-

A) 0
B) 1
C) 2
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
20
Find the limit algebraically.
-
A) 0
B) 6
C) -6
D) 1
-
A) 0
B) 6
C) -6
D) 1

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
21
Find the limit algebraically.
-
A) 100
B) -352
C) 256
D) 144
-
A) 100
B) -352
C) 256
D) 144

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
22
Find the limit algebraically.
-
A) -125
B) -5
C) 125
D) 25
-
A) -125
B) -5
C) 125
D) 25

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
23
Find the limit algebraically.
-
A) 15
B) 0
C) 29
D) does not exist
-
A) 15
B) 0
C) 29
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
24
Find the limit algebraically.
-
A) -5
B) 0
C) 5
D) does not exist
-
A) -5
B) 0
C) 5
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
25
Find the limit algebraically.
-
A)
B) 0
C) 8
D) does not exist
-
A)
B) 0
C) 8
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
26
Find the limit algebraically.
-
A) - 59
B) - 75
C) - 12
D) does not exist
-
A) - 59
B) - 75
C) - 12
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
27
Find the limit algebraically.
-
A) Does not exist
B) 3
C) 1
D) 6
-
A) Does not exist
B) 3
C) 1
D) 6

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
28
Find the limit algebraically.
-
A) -3
B) -1
C) 3
D) 1
-
A) -3
B) -1
C) 3
D) 1

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
29
Find the limit algebraically.
-
A) 2
B) 0
C) 4
D) Does not exist
-
A) 2
B) 0
C) 4
D) Does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
30
Find the limit algebraically.
-
A) Does not exist
B) 5
C) 0
D) -8
-
A) Does not exist
B) 5
C) 0
D) -8

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
31
Find the limit algebraically.
-
A) -2
B) 2
C) 0
D) does not exist
-
A) -2
B) 2
C) 0
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
32
Find the limit algebraically.
-
A) 31
B) -1
C) 1
D) 33
-
A) 31
B) -1
C) 1
D) 33

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
33
Find the limit algebraically.
-
A) -5
B) 0
C) 5
D) does not exist
-
A) -5
B) 0
C) 5
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
34
Find the limit algebraically.
-
A) 0
B) -1
C) 5
D) Does not exist
-
A) 0
B) -1
C) 5
D) Does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
35
Find the limit algebraically.
-
A) -8
B) -1,000
C) -10
D) 8,000
-
A) -8
B) -1,000
C) -10
D) 8,000

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
36
Find the limit algebraically.
-
A) -1
B) 1
C) 2
D) does not exist
-
A) -1
B) 1
C) 2
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
37
Find the limit algebraically.
-
A) -4
B) 4
C) Does not exist
D) 0
-
A) -4
B) 4
C) Does not exist
D) 0

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
38
Find the limit algebraically.
-
A) 0
B) 6
C) Does not exist
D) -6
-
A) 0
B) 6
C) Does not exist
D) -6

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
39
Find the limit algebraically.
-
A) -48
B) -237
C) 249
D) -21
-
A) -48
B) -237
C) 249
D) -21

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
40
Find the limit algebraically.
-
A) Does not exist
B) -2
C) 1
D) -4
-
A) Does not exist
B) -2
C) 1
D) -4

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
41
Find the limit algebraically.
-
A) -16
B) 0
C) 16
D) does not exist
-
A) -16
B) 0
C) 16
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
42
Use the graph of y = g(x) to answer the question. 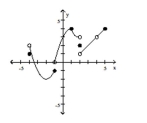
-Does xist? If it does, what is it?
A) yes; 0
B) yes; 3
C) yes; 4
D) does not exist

-Does xist? If it does, what is it?
A) yes; 0
B) yes; 3
C) yes; 4
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
43
Use the graph of y = g(x) to answer the question. 
Find f(1).
A) 3
B) 4
C) 0
D) -1

Find f(1).
A) 3
B) 4
C) 0
D) -1

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
44
Find the limit as x approaches c of the average rate of change of the function from c to x.
-c = 3;
A) 108
B) 57
C) 30
D) 90
-c = 3;
A) 108
B) 57
C) 30
D) 90

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
45
Use the graph of y = g(x) to answer the question. 
-What is the domain of g?
A)
B) or
C)
D) or or

-What is the domain of g?
A)
B) or
C)
D) or or

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
46
Use the graph of y = g(x) to answer the question. 
-Find the y-intercept(s), if any, of g.
A) 3
B) 0
C) -1
D)

-Find the y-intercept(s), if any, of g.
A) 3
B) 0
C) -1
D)

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
47
Use the graph of y = g(x) to answer the question. 
Find f(-1).
A) 1
B) 0
C) 4
D) -1

Find f(-1).
A) 1
B) 0
C) 4
D) -1

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
48
Use the graph of y = g(x) to answer the question. 
-Find f(2)
A) 1
B) 2
C)
D) 3

-Find f(2)
A) 1
B) 2
C)
D) 3

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
49
Find the limit as x approaches c of the average rate of change of the function from c to x.
-c = -3;
A) 18
B) -9
C) -12
D) Does not exist
-c = -3;
A) 18
B) -9
C) -12
D) Does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
50
Find the limit algebraically.
-
A) Does not exist
B)
C) 1
D) 0
-
A) Does not exist
B)
C) 1
D) 0

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
51
Find the limit as x approaches c of the average rate of change of the function from c to x.
c = 3; f(x) = 5x + 3
A) -5
B) 3
C) 5
D) -3
c = 3; f(x) = 5x + 3
A) -5
B) 3
C) 5
D) -3

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
52
Find the limit as x approaches c of the average rate of change of the function from c to x.
-c = 4;
A)
B)
C)
D) Does not exist
-c = 4;
A)
B)
C)
D) Does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
53
Use the graph of y = g(x) to answer the question. 
Find f(-4).
A) -4
B) 0
C) 2
D) 1

Find f(-4).
A) -4
B) 0
C) 2
D) 1

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
54
Use the graph of y = g(x) to answer the question. 
-
A) 1
B) 0
C) 4
D) does not exist

-
A) 1
B) 0
C) 4
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
55
Find the limit as x approaches c of the average rate of change of the function from c to x.
-c = 9;
A) 3
B) Does not exist
C) 13
D)
-c = 9;
A) 3
B) Does not exist
C) 13
D)

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
56
Find the limit algebraically.
-
A) 4
B) 0
C) -4
D) does not exist
-
A) 4
B) 0
C) -4
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
57
Find the limit algebraically.
-
A) 1
B) Does not exist
C)
D) 0
-
A) 1
B) Does not exist
C)
D) 0

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
58
Find the limit as x approaches c of the average rate of change of the function from c to x.
-c = -4;
A) 0
B) 16
C) 48
D) 32
-c = -4;
A) 0
B) 16
C) 48
D) 32

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
59
Use the graph of y = g(x) to answer the question. 
-What is the range of g?
A) all real numbers
B)
C)
D)

-What is the range of g?
A) all real numbers
B)
C)
D)

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
60
Find the one-sided limit.
-
A) 0
B) -1
C) 4
D) does not exist
-
A) 0
B) -1
C) 4
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
61
Determine whether f is continuous at c.
-
A) not continuous
B) continuous
-
A) not continuous
B) continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
62
Determine whether f is continuous at c.
-
A) continuous
B) not continuous
-
A) continuous
B) not continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
63
Determine whether f is continuous at c.
-
A) continuous
B) not continuous
-
A) continuous
B) not continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
64
Determine whether f is continuous at c.
-
A) not continuous
B) continuous
-
A) not continuous
B) continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
65
Determine whether f is continuous at c.
-
A) continuous
B) not continuous
-
A) continuous
B) not continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
66
Determine whether f is continuous at c.
-
A) continuous
B) not continuous
-
A) continuous
B) not continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
67
Determine whether f is continuous at c.
-
A) not continuous
B) continuous
-
A) not continuous
B) continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
68
Find the one-sided limit.
-
A)
B) 0
C)
D) 1
-
A)
B) 0
C)
D) 1

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
69
Find the one-sided limit.
-
A) 3
B) -6
C) 6
D) -3
-
A) 3
B) -6
C) 6
D) -3

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
70
Determine whether f is continuous at c.
-
A) not continuous
B) continuous
-
A) not continuous
B) continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
71
Determine whether f is continuous at c.
-
A) not continuous
B) continuous
-
A) not continuous
B) continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
72
Determine whether f is continuous at c.
-
A) continuous
B) not continuous
-
A) continuous
B) not continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
73
Find the one-sided limit.
-
A) 8
B) 0
C) 4
D) does not exist
-
A) 8
B) 0
C) 4
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
74
Determine whether f is continuous at c.
-
A) not continuous
B) continuous
-
A) not continuous
B) continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
75
Determine whether f is continuous at c.
-
A) continuous
B) not continuous
-
A) continuous
B) not continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
76
Find the one-sided limit.
-
A) -2
B) 2
C) 0
D) does not exist
-
A) -2
B) 2
C) 0
D) does not exist

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
77
Determine whether f is continuous at c.
-
A) continuous
B) not continuous
-
A) continuous
B) not continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
78
Find the one-sided limit.
-
A) Does not exist
B) 5
C) -8
D) 0
-
A) Does not exist
B) 5
C) -8
D) 0

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
79
Determine whether f is continuous at c.
-
A) not continuous
B) continuous
-
A) not continuous
B) continuous

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck
80
Find the one-sided limit.
-
A) 13
B) -5
C) 1
D) 7
-
A) 13
B) -5
C) 1
D) 7

Unlock Deck
Unlock for access to all 145 flashcards in this deck.
Unlock Deck
k this deck



