Deck 10: Counting Methods
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/213
Play
Full screen (f)
Deck 10: Counting Methods
1
A male president and three representatives
A)ABCD, ACDE, CABD, CABE, EABC, EABD; 6
B)ABCD, CABD, CABE, EABD, ADCB, AEDC, CDBA, ECBA, EDBA; 9
C)ABCD, CABD, EABC; 3
D)ABCD, ACDE, ABCE, ABDE, CABD, CABE, CBDE, CADE, EABC, EABD, EBCD, EACD; 12
A)ABCD, ACDE, CABD, CABE, EABC, EABD; 6
B)ABCD, CABD, CABE, EABD, ADCB, AEDC, CDBA, ECBA, EDBA; 9
C)ABCD, CABD, EABC; 3
D)ABCD, ACDE, ABCE, ABDE, CABD, CABE, CBDE, CADE, EABC, EABD, EBCD, EACD; 12
D
2
Using the 36 possibilities found in the product table for rolling two dice, list and count the outcomes for which the sum
(for both dice)is the following.
Equal to 8
A)(2,6), (3,5), (4,4), (4,4), (5,3), (6,2); 6
B)(2,6), (3,5); 2
C)(2,6), (3,5), (4,4); 3
D)(2,6), (3,5), (4,4), (5,3), (6,2); 5
(for both dice)is the following.
Equal to 8
A)(2,6), (3,5), (4,4), (4,4), (5,3), (6,2); 6
B)(2,6), (3,5); 2
C)(2,6), (3,5), (4,4); 3
D)(2,6), (3,5), (4,4), (5,3), (6,2); 5
D
3
Three representatives, if two must be male and one must be female
A)ACB, ACD, AEB, AED, CEB, CED, DEC, BEC, DEA, BEA, DCA, BCA; 12
B)ABC, CDE; 2
C)ACB, ACD, AEB, AED, CEB, CED; 6
D)ACB, ACD, AEB, AED; 4
A)ACB, ACD, AEB, AED, CEB, CED, DEC, BEC, DEA, BEA, DCA, BCA; 12
B)ABC, CDE; 2
C)ACB, ACD, AEB, AED, CEB, CED; 6
D)ACB, ACD, AEB, AED; 4
C
4
Solve the problem.
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}. List the square numbers in the table.
A){4, 9}
B){16}
C){16, 22}
D)none
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}. List the square numbers in the table.
A){4, 9}
B){16}
C){16, 22}
D)none

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
5
Using the 36 possibilities found in the product table for rolling two dice, list and count the outcomes for which the sum
(for both dice)is the following.
Between 7 and 10
A)(2,6), (3,6), (5,3), (4,4), (4,5); 5
B)(2,6), (6,2), (6,3), (3,6), (5,3), (3,5), (4,4), (4,5), (5,4); 9
C)(2,6), (6,2), (6,3), (3,6), (5,3), (3,5), (4,5); 8
D)(2,6), (6,2), (3,6), (6,3), (5,3), (3,5), (4,4), (4,5), (5,4), (3,4), (4,3), (6,4), (4,6), (5,5); 14
(for both dice)is the following.
Between 7 and 10
A)(2,6), (3,6), (5,3), (4,4), (4,5); 5
B)(2,6), (6,2), (6,3), (3,6), (5,3), (3,5), (4,4), (4,5), (5,4); 9
C)(2,6), (6,2), (6,3), (3,6), (5,3), (3,5), (4,5); 8
D)(2,6), (6,2), (3,6), (6,3), (5,3), (3,5), (4,4), (4,5), (5,4), (3,4), (4,3), (6,4), (4,6), (5,5); 14

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
6
Solve the problem.
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}.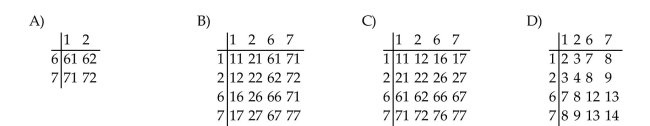
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}.


Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
7
Using the 36 possibilities found in the product table for rolling two dice, list and count the outcomes for which the sum
(for both dice)is the following.
Multiple of 5
A)(1,4), (4,1), (2,3), (3,2); 4
B)(1,5), (2,5), (3,5), (4,5), (5,5), (6,5); 6
C)None
D)(1,4), (4,1), (2,3), (3,2), (4,6), (6,4), (5,5); 7
(for both dice)is the following.
Multiple of 5
A)(1,4), (4,1), (2,3), (3,2); 4
B)(1,5), (2,5), (3,5), (4,5), (5,5), (6,5); 6
C)None
D)(1,4), (4,1), (2,3), (3,2), (4,6), (6,4), (5,5); 7

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
8
Using the 36 possibilities found in the product table for rolling two dice, list and count the outcomes for which the sum
(for both dice)is the following.
Multiple of 3
A)(1,3), (3,1), (3,3), (2,3), (3,2), (3,4), (4,3), (5,3), (3,5), (6,3), (3,6); 11
B)(1,2), (2,1), (2,4), (4,2), (1,5), (5,1), (3,3), (3,6), (6,3), (4,5), (5,4), (6,6); 12
C)(1,2), (1,5), (3,3), (3,6), (4,5), (6,6); 6
D)(3,3), (6,6); 2
(for both dice)is the following.
Multiple of 3
A)(1,3), (3,1), (3,3), (2,3), (3,2), (3,4), (4,3), (5,3), (3,5), (6,3), (3,6); 11
B)(1,2), (2,1), (2,4), (4,2), (1,5), (5,1), (3,3), (3,6), (6,3), (4,5), (5,4), (6,6); 12
C)(1,2), (1,5), (3,3), (3,6), (4,5), (6,6); 6
D)(3,3), (6,6); 2

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
9
Solve the problem.
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}. List the prime numbers in the table.
A){11, 17, 61, 67, 71, 77}
B){11, 17, 61, 67, 71}
C){61, 71}
D){2, 3, 7, 13}
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}. List the prime numbers in the table.
A){11, 17, 61, 67, 71, 77}
B){11, 17, 61, 67, 71}
C){61, 71}
D){2, 3, 7, 13}

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
10
Using the 36 possibilities found in the product table for rolling two dice, list and count the outcomes for which the sum
(for both dice)is the following.
Greater than 10
A)(6,5), (5,6), (6,6); 3
B)(6,5), (6,6); 2
C)(6,6); 1
D)(4,6), (6,4), (5,6), (6,5), (5,5); 5
(for both dice)is the following.
Greater than 10
A)(6,5), (5,6), (6,6); 3
B)(6,5), (6,6); 2
C)(6,6); 1
D)(4,6), (6,4), (5,6), (6,5), (5,5); 5

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
11
Solve the problem.
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}. List the even numbers in the table.
A){12, 26, 26, 62, 66, 72, 76}
B){2, 4, 8, 12, 14}
C){62, 72}
D){12, 16, 22, 26, 62, 66, 72, 76}
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}. List the even numbers in the table.
A){12, 26, 26, 62, 66, 72, 76}
B){2, 4, 8, 12, 14}
C){62, 72}
D){12, 16, 22, 26, 62, 66, 72, 76}

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
12
Using the 36 possibilities found in the product table for rolling two dice, list and count the outcomes for which the sum
(for both dice)is the following.
Less than 3
A)(1,1), (1,2); 2
B)(1,1), (1,2), (2, 1), (2,2); 4
C)(1,1), (1,2), (2,1); 3
D)(1,1); 1
(for both dice)is the following.
Less than 3
A)(1,1), (1,2); 2
B)(1,1), (1,2), (2, 1), (2,2); 4
C)(1,1), (1,2), (2,1); 3
D)(1,1); 1

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
13
Three representatives, if two must be female and one must be male
A)BDA, BDC, BDE, BAD, BCD, BED;6
B)BDA, BDC, BDE, DBA, DBC; 5
C)BDA, BDC, BDE; 3
D)BDA, BDC; 2
A)BDA, BDC, BDE, BAD, BCD, BED;6
B)BDA, BDC, BDE, DBA, DBC; 5
C)BDA, BDC, BDE; 3
D)BDA, BDC; 2

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
14
A president, a secretary, and a treasurer, if the president must be a woman and the other two must be men
A)BAC, BAE, DAC, DAE; 4
B)BAC, BAE, BCE, DAC, DAE, DCE, BCA, BEA, BEC, DCA, DEA, DEC; 12
C)BAC, BAE, BCE, DAC, DAE, DCE; 6
D)ABD, CBD, EBD; 3
A)BAC, BAE, DAC, DAE; 4
B)BAC, BAE, BCE, DAC, DAE, DCE, BCA, BEA, BEC, DCA, DEA, DEC; 12
C)BAC, BAE, BCE, DAC, DAE, DCE; 6
D)ABD, CBD, EBD; 3

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
15
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
The first die shows a 3.
A)(3,3)
B)(3,1),(3,2),(3,4),(3,5),(3,6)
C)(3,1),(3,2),(3,3),(3,4),(3,5),(3,6)
D)(3,1),(3,3),(3,5)
The first die shows a 3.
A)(3,3)
B)(3,1),(3,2),(3,4),(3,5),(3,6)
C)(3,1),(3,2),(3,3),(3,4),(3,5),(3,6)
D)(3,1),(3,3),(3,5)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
16
Using the 36 possibilities found in the product table for rolling two dice, list and count the outcomes for which the sum
(for both dice)is the following.
Less than 4
A)(1,1), (1,2), (2,1); 3
B)(1,1), (1,2); 2
C)(1,1), (2,2), (1,2), (2,1); 4
D)(1,1), (1,2), (2,1), (1,3), (3,1), (2,2); 6
(for both dice)is the following.
Less than 4
A)(1,1), (1,2), (2,1); 3
B)(1,1), (1,2); 2
C)(1,1), (2,2), (1,2), (2,1); 4
D)(1,1), (1,2), (2,1), (1,3), (3,1), (2,2); 6

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
17
Solve the problem.
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}. List the numbers with repeating digits.
A){11, 66, 77}
B){22, 66}
C){11, 22, 66, 77}
D)none
Construct a product table showing all possible two-digit numbers using digits from the set {1, 2, 6, 7}. List the numbers with repeating digits.
A){11, 66, 77}
B){22, 66}
C){11, 22, 66, 77}
D)none

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
18
Using the 36 possibilities found in the product table for rolling two dice, list and count the outcomes for which the sum
(for both dice)is the following.
Multiple of 11
A)(5,6), (6,5); 2
B)None
C)(1,1); 1
D)(5,6); 1
(for both dice)is the following.
Multiple of 11
A)(5,6), (6,5); 2
B)None
C)(1,1); 1
D)(5,6); 1

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
19
A treasurer and a secretary if the two must not be the same sex
A)AB, AD, CB, CD, EB, ED, BA, DA, BC, DC, BE, DE; 12
B)AB, AC, AD, AE, BC, BD, BE, CD, CE, DE; 10
C)AB, CB, EB; 3
D)AB, AD, CB, CD, EB, ED; 6
A)AB, AD, CB, CD, EB, ED, BA, DA, BC, DC, BE, DE; 12
B)AB, AC, AD, AE, BC, BD, BE, CD, CE, DE; 10
C)AB, CB, EB; 3
D)AB, AD, CB, CD, EB, ED; 6

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
20
Four representatives
A)ABCD; 1
B)ABCD, ABCE, ACDE, ADEB, BCDE; 5
C)ABCD, ABCE, ACDE, ADEB; 4
D)ABCD, ABCE, ACDE, ADEB, BCDE, BCEA, BDEA, CABD, CEDB, DACE; 10
A)ABCD; 1
B)ABCD, ABCE, ACDE, ADEB, BCDE; 5
C)ABCD, ABCE, ACDE, ADEB; 4
D)ABCD, ABCE, ACDE, ADEB, BCDE, BCEA, BDEA, CABD, CEDB, DACE; 10

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
21
Use a tree diagram showing all possible results when four fair coins are tossed. Then list the ways of getting the indicated
result.
the same on all four coins
A)hhhh, hhht, tttt, ttth
B)tttt
C)hhhh, tttt
D)hhhh
result.
the same on all four coins
A)hhhh, hhht, tttt, ttth
B)tttt
C)hhhh, tttt
D)hhhh

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
22
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
The second die shows a 3.
A)(1,3),(2,3),(3,3),(4,3),(5,3),(6,3)
B)(1,3),(3,3),(5,3)
C)(1,3),(2,3),(4,3),(5,3),(6,3)
D)(3,3)
The second die shows a 3.
A)(1,3),(2,3),(3,3),(4,3),(5,3),(6,3)
B)(1,3),(3,3),(5,3)
C)(1,3),(2,3),(4,3),(5,3),(6,3)
D)(3,3)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
23
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
The sum of the numbers showing is either 4 or 5 and one die is a 2.
A)(2,2),(2,3)
B)(2,2),(3,2)
C)(2,2),(2,3),(3,2)
D)(2,3),(3,2)
The sum of the numbers showing is either 4 or 5 and one die is a 2.
A)(2,2),(2,3)
B)(2,2),(3,2)
C)(2,2),(2,3),(3,2)
D)(2,3),(3,2)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
24
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
The sum of the numbers showing is 7 and one die is a 3.
A)(3,4)
B)(4,3)
C)0
D)(3,4),(4,3)
The sum of the numbers showing is 7 and one die is a 3.
A)(3,4)
B)(4,3)
C)0
D)(3,4),(4,3)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
25
Determine the number of figures (of any size)in the design.
Squares (of any size)
Squares (of any size)


Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
26
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
The first and second die are even.
A)(2,2),(4,4),(6,6)
B)(2,4),(2,6),(4,2),(4,6)(6,2),(6,4)
C)(2,2),(2,4),(2,6),(4,2),(4,4),(4,6),(6,2),(6,4),(6,6)
D)(2,2),(2,4),(2,6)
The first and second die are even.
A)(2,2),(4,4),(6,6)
B)(2,4),(2,6),(4,2),(4,6)(6,2),(6,4)
C)(2,2),(2,4),(2,6),(4,2),(4,4),(4,6),(6,2),(6,4),(6,6)
D)(2,2),(2,4),(2,6)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
27
Use a tree diagram showing all possible results when four fair coins are tossed. Then list the ways of getting the indicated
result.
exactly two tails
A)hhtt, htht, htth, thth, tthh
B)tthh
C)hhtt, htht, htth, thht, thth, tthh, ttht
D)hhtt, htht, htth, thht, thth, tthh
result.
exactly two tails
A)hhtt, htht, htth, thth, tthh
B)tthh
C)hhtt, htht, htth, thht, thth, tthh, ttht
D)hhtt, htht, htth, thht, thth, tthh

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
28
Use a tree diagram showing all possible results when four fair coins are tossed. Then list the ways of getting the indicated
result.
tails on the first coin
A)thhh, thht, thtt, tthh, ttht, tttt
B)thhh, thht, thth, thtt, tthh, ttth, tttt
C)thhh, tthh, ttth, tttt
D)thhh, thht, thth, thtt, tthh, ttht, ttth, tttt
result.
tails on the first coin
A)thhh, thht, thtt, tthh, ttht, tttt
B)thhh, thht, thth, thtt, tthh, ttth, tttt
C)thhh, tthh, ttth, tttt
D)thhh, thht, thth, thtt, tthh, ttht, ttth, tttt

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
29
Determine the number of figures (of any size)in the design.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
30
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
Exactly one die shows a 3.
A)(3,3)
B)(3, 1),(3, 2),(3, 3), (3, 4),(3, 5),(3, 6), (1, 3), (2, 3), (4, 3), (5, 3), (6, 3)
C)(3, 1),(3, 2),(3, 4),(3, 5),(3, 6)
D)(3, 1),(3, 2),(3, 4),(3, 5),(3, 6), (1, 3), (2, 3), (4, 3), (5, 3), (6, 3)
Exactly one die shows a 3.
A)(3,3)
B)(3, 1),(3, 2),(3, 3), (3, 4),(3, 5),(3, 6), (1, 3), (2, 3), (4, 3), (5, 3), (6, 3)
C)(3, 1),(3, 2),(3, 4),(3, 5),(3, 6)
D)(3, 1),(3, 2),(3, 4),(3, 5),(3, 6), (1, 3), (2, 3), (4, 3), (5, 3), (6, 3)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
31
Determine the number of figures (of any size)in the design.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
32
Use a tree diagram showing all possible results when four fair coins are tossed. Then list the ways of getting the indicated
result.
more than two tails
A)httt, thtt, ttht, ttth
B)httt, thtt, ttht, ttth, tttt
C)hhtt, htht, htth, httt, thht, thth, thtt, tthh, ttht, ttth, tttt
D)hhtt, htht, htth, thht, thth, tthh
result.
more than two tails
A)httt, thtt, ttht, ttth
B)httt, thtt, ttht, ttth, tttt
C)hhtt, htht, htth, httt, thht, thth, thtt, tthh, ttht, ttth, tttt
D)hhtt, htht, htth, thht, thth, tthh

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
33
Use a tree diagram showing all possible results when four fair coins are tossed. Then list the ways of getting the indicated
result.
the same outcome on the first two coins
A)hhhh, hhht, hhth, hthh, hhtt, tthh, ttht, ttth, tttt
B)hhhh, hhht, hhth, hhtt, tthh, ttht, ttth, tttt
C)hhhh, hhht, hhtt, tthh, ttht, ttth, tttt
D)hhhh, hhht, hhth, hhtt
result.
the same outcome on the first two coins
A)hhhh, hhht, hhth, hthh, hhtt, tthh, ttht, ttth, tttt
B)hhhh, hhht, hhth, hhtt, tthh, ttht, ttth, tttt
C)hhhh, hhht, hhtt, tthh, ttht, ttth, tttt
D)hhhh, hhht, hhth, hhtt

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
34
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
The sum of the numbers showing is 5.
A)(2,3),(3,2)
B)(2,3),(4,1)
C)(1,4),(2,3),(3,2),(4,1)
D)(3,2),(4,1)
The sum of the numbers showing is 5.
A)(2,3),(3,2)
B)(2,3),(4,1)
C)(1,4),(2,3),(3,2),(4,1)
D)(3,2),(4,1)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
35
Determine the number of figures (of any size)in the design.
Triangles (of any size)
Triangles (of any size)


Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
36
Determine the number of figures (of any size)in the design.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
37
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
The sum of the numbers showing is either 3 or 4.
A)(1,2),(2,2)
B)(1,2),(1,3),(2,2)
C)(1,2),(2,1),(1,3),(3,1),(2,2)
D)(2,1),(3,1),(2,2)
The sum of the numbers showing is either 3 or 4.
A)(1,2),(2,2)
B)(1,2),(1,3),(2,2)
C)(1,2),(2,1),(1,3),(3,1),(2,2)
D)(2,1),(3,1),(2,2)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
38
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
At least one die shows a 3.
A)(3, 1),(3, 2),(3, 3), (3, 4),(3, 5),(3, 6), (1, 3), (2, 3), (4, 3), (5, 3), (6, 3)
B)(3, 1),(3, 2),(3, 4),(3, 5),(3, 6), (1, 3), (2, 3), (4, 3), (5, 3), (6, 3)
C)(3, 1),(3, 2),(3, 4),(3, 5),(3, 6)
D)(3,3)
At least one die shows a 3.
A)(3, 1),(3, 2),(3, 3), (3, 4),(3, 5),(3, 6), (1, 3), (2, 3), (4, 3), (5, 3), (6, 3)
B)(3, 1),(3, 2),(3, 4),(3, 5),(3, 6), (1, 3), (2, 3), (4, 3), (5, 3), (6, 3)
C)(3, 1),(3, 2),(3, 4),(3, 5),(3, 6)
D)(3,3)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
39
Use a tree diagram showing all possible results when a die is rolled twice. List the ways of getting the following result.
The sum of the numbers showing is 8.
A)(2,6),(3,5),(4,4),(5,3),(6,2)
B)(2,6),(3,5),(4,4)
C)(2,6),(3,5),(5,3),(6,2)
D)(4,4)
The sum of the numbers showing is 8.
A)(2,6),(3,5),(4,4),(5,3),(6,2)
B)(2,6),(3,5),(4,4)
C)(2,6),(3,5),(5,3),(6,2)
D)(4,4)

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
40
Use a tree diagram showing all possible results when four fair coins are tossed. Then list the ways of getting the indicated
result.
at least two tails
A)hhtt, htht, httt, thht, thth, tthh, ttht, ttth, tttt
B)hhtt, htht, htth, httt, thht, thth, thtt, tthh, ttht, ttth, tttt
C)hhtt, htht, htth, thht, thth, tthh
D)httt, thtt, ttht, ttth, tttt
result.
at least two tails
A)hhtt, htht, httt, thht, thth, tthh, ttht, ttth, tttt
B)hhtt, htht, htth, httt, thht, thth, thtt, tthh, ttht, ttth, tttt
C)hhtt, htht, htth, thht, thth, tthh
D)httt, thtt, ttht, ttth, tttt

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
41
Solve the problem.
Suppose there are 8 roads connecting town A to town B and 6 roads connecting town B to town C. In how many ways can a person travel from A to C via B?
A)14 ways
B)36 ways
C)48 ways
D)64 ways
Suppose there are 8 roads connecting town A to town B and 6 roads connecting town B to town C. In how many ways can a person travel from A to C via B?
A)14 ways
B)36 ways
C)48 ways
D)64 ways

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
42
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
43
Solve the problem.
Six strangers arrive at a business seminar and each person shakes hands with every other person. How many handshakes are there?
A)18
B)20
C)15
D)30
Six strangers arrive at a business seminar and each person shakes hands with every other person. How many handshakes are there?
A)18
B)20
C)15
D)30

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
44
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
45
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
46
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
47
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
48
Solve the problem.
A computer printer allows for optional settings with a panel of four on-off switches in a row. How many different settings can be selected if no three adjacent switches can all be off?
A)13
B)12
C)14
D)10
A computer printer allows for optional settings with a panel of four on-off switches in a row. How many different settings can be selected if no three adjacent switches can all be off?
A)13
B)12
C)14
D)10

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
49
Solve the problem.
A sports shop sold tennis rackets in 3 different weights, 3 types of string, and 4 grip sizes. How many different rackets could be sold?
A)24 rackets
B)36 rackets
C)27 rackets
D)10 rackets
A sports shop sold tennis rackets in 3 different weights, 3 types of string, and 4 grip sizes. How many different rackets could be sold?
A)24 rackets
B)36 rackets
C)27 rackets
D)10 rackets

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
50
Solve the problem.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
51
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
52
Solve the problem.
A salesperson packed 3 shirts and 5 ties. With one shirt, he could wear all 5 ties. With another shirt, he could wear 4 ties. With the third shirt, he could wear only 2 ties. How many different
Combinations did he have?
A)40 combinations
B)11 combinations
C)10 combinations
D)22 combinations
A salesperson packed 3 shirts and 5 ties. With one shirt, he could wear all 5 ties. With another shirt, he could wear 4 ties. With the third shirt, he could wear only 2 ties. How many different
Combinations did he have?
A)40 combinations
B)11 combinations
C)10 combinations
D)22 combinations

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
53
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
54
Solve the problem.
A restaurant offered salads with 3 type(s)of dressing and 6 different topping(s). How many different types of salad could be ordered?
A)36
B)18
C)9
D)9
A restaurant offered salads with 3 type(s)of dressing and 6 different topping(s). How many different types of salad could be ordered?
A)36
B)18
C)9
D)9

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
55
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
56
Solve the problem.
In how many ways can the number 44 be written as the sum of two primes?
A)3
B)6
C)4
D)5
In how many ways can the number 44 be written as the sum of two primes?
A)3
B)6
C)4
D)5

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
57
A shirt company has 3 designs, each of which can be made with short or long sleeves. There are 7 color patterns available. How many different types of shirts are available from this company?
A)21 types
B)42 types
C)10 types
D)12 types
A)21 types
B)42 types
C)10 types
D)12 types

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
58
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
59
Solve the problem.
How many three-digit numbers have the sum of their digits equal to 20?
A)30
B)40
C)36
D)32
How many three-digit numbers have the sum of their digits equal to 20?
A)30
B)40
C)36
D)32

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
60
Evaluate the factorial expression.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
61
Solve the problem.
How many different 4-letter radio-station call letters can be made if the first letter must be K or W, repeats are allowed, but the call letters cannot end in an O?
A)35,152
B)456,976
C)33,800
D)16,900
How many different 4-letter radio-station call letters can be made if the first letter must be K or W, repeats are allowed, but the call letters cannot end in an O?
A)35,152
B)456,976
C)33,800
D)16,900

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
62
Solve the problem.
License plates are made using 2 letters followed by 2 digits. How many plates can be made if repetition of letters and digits is allowed?
A)456,976
B)10,000
C)67,600
D)6760
License plates are made using 2 letters followed by 2 digits. How many plates can be made if repetition of letters and digits is allowed?
A)456,976
B)10,000
C)67,600
D)6760

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
63
Solve the problem.
A local department store sells carpets in 4 sizes. Each carpet comes in 3 different qualities. One of the sizes comes in 5 colors. The other sizes come in 3 colors. How many choices of carpet are there?
A)42
B)44
C)47
D)51
A local department store sells carpets in 4 sizes. Each carpet comes in 3 different qualities. One of the sizes comes in 5 colors. The other sizes come in 3 colors. How many choices of carpet are there?
A)42
B)44
C)47
D)51

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
64
Solve the problem.
How many different sequences of 4 digits are possible if the first digit must be 3, 4, or 5 and if the sequence may not end in 000? Repetition of digits is allowed.
A)2,997
B)2,000
C)1,512
D)2,999
How many different sequences of 4 digits are possible if the first digit must be 3, 4, or 5 and if the sequence may not end in 000? Repetition of digits is allowed.
A)2,997
B)2,000
C)1,512
D)2,999

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
65
Solve the problem.
How many 5-digit numbers can be formed using the digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, if repetitions of digits are allowed?
A)3125
B)120
C)99,999
D)100,000
How many 5-digit numbers can be formed using the digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, if repetitions of digits are allowed?
A)3125
B)120
C)99,999
D)100,000

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
66
Solve the problem.
A salesman packed 3 shirts and 6 ties. With one shirt, he could wear all 6 ties. With another shirt, he could wear 5 ties. With the third shirt, he could wear only 3 ties. How many different
Combinations did he have?
A)14
B)90
C)33
D)13
A salesman packed 3 shirts and 6 ties. With one shirt, he could wear all 6 ties. With another shirt, he could wear 5 ties. With the third shirt, he could wear only 3 ties. How many different
Combinations did he have?
A)14
B)90
C)33
D)13

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
67
Solve the problem.
Given a committee of 8 women and 11 men, count the number of different ways of choosing a president, a secretary, and a treasurer, if the president must be a woman and the secretary and
Treasurer must be men. Assume no one can hold more than one office.
A)440
B)5814
C)968
D)880
Given a committee of 8 women and 11 men, count the number of different ways of choosing a president, a secretary, and a treasurer, if the president must be a woman and the secretary and
Treasurer must be men. Assume no one can hold more than one office.
A)440
B)5814
C)968
D)880

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
68
Solve the problem.
Mark can remember only the first 4 digits of his friend's phone number. He also knows that the number has 7 digits and that the last digit is not a 0. If Mark were to dial all of the possible numbers
And if it takes him 21 seconds to try each one, how long would it take to try every possibility?
A)35 minutes
B)10.5 minutes
C)350.1 minutes
D)315 minutes
Mark can remember only the first 4 digits of his friend's phone number. He also knows that the number has 7 digits and that the last digit is not a 0. If Mark were to dial all of the possible numbers
And if it takes him 21 seconds to try each one, how long would it take to try every possibility?
A)35 minutes
B)10.5 minutes
C)350.1 minutes
D)315 minutes

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
69
Solve the problem.
A restaurant offers salads with 2 types of lettuce, 5 different toppings, and 5 different dressings. How many different salads could be ordered?
A)50
B)35
C)20
D)12
A restaurant offers salads with 2 types of lettuce, 5 different toppings, and 5 different dressings. How many different salads could be ordered?
A)50
B)35
C)20
D)12

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
70
Solve the problem.
In how many ways can 6 people line up for play tickets?
A)1
B)720
C)46,656
D)6
In how many ways can 6 people line up for play tickets?
A)1
B)720
C)46,656
D)6

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
71
Solve the problem.
How many ways can a president, vice-president, and secretary be chosen from a club with 12 members?
A)1320
B)6
C)220
D)36
How many ways can a president, vice-president, and secretary be chosen from a club with 12 members?
A)1320
B)6
C)220
D)36

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
72
Solve the problem.
At a lumber company, shelves are sold in 4 types of wood, 2 different widths and 5 different lengths. How many different types of shelves could be ordered?
A)30
B)32
C)11
D)40
At a lumber company, shelves are sold in 4 types of wood, 2 different widths and 5 different lengths. How many different types of shelves could be ordered?
A)30
B)32
C)11
D)40

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
73
Solve the problem.
A shoe store carries one brand of shoe in 4 different styles, 5 sizes, and 5 colors. How many different shoes are available of this one brand?
A)100
B)45
C)80
D)14
A shoe store carries one brand of shoe in 4 different styles, 5 sizes, and 5 colors. How many different shoes are available of this one brand?
A)100
B)45
C)80
D)14

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
74
Solve the problem.
A saleswoman packed 3 jackets and 6 skirts. With one jacket, she could wear all 6 skirts. With another jacket, she could wear 5 skirts. With the third jacket, she could wear only 2 skirts. How
Many different combinations did she have?
A)13
B)12
C)32
D)60
A saleswoman packed 3 jackets and 6 skirts. With one jacket, she could wear all 6 skirts. With another jacket, she could wear 5 skirts. With the third jacket, she could wear only 2 skirts. How
Many different combinations did she have?
A)13
B)12
C)32
D)60

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
75
Solve the problem.
A sports shop sells tennis rackets in 4 different weights, 3 types of string, and 3 grip sizes. How many different rackets could they sell?
A)10
B)21
C)36
D)48
A sports shop sells tennis rackets in 4 different weights, 3 types of string, and 3 grip sizes. How many different rackets could they sell?
A)10
B)21
C)36
D)48

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
76
Solve the problem.
If 5 newborn babies are randomly selected, how many different gender sequences are possible?
A)25
B)10
C)120
D)32
If 5 newborn babies are randomly selected, how many different gender sequences are possible?
A)25
B)10
C)120
D)32

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
77
Solve the problem.



Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
78
Solve the problem.
Given a group of 8 women and 11 men, how many different ways are there of choosing one man and one woman for a committee?
A)361
B)19
C)88
D)342
Given a group of 8 women and 11 men, how many different ways are there of choosing one man and one woman for a committee?
A)361
B)19
C)88
D)342

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
79
Solve the problem.
A baseball manager has 10 players of the same ability. How many 9 player starting lineups can he create?
A)10
B)362,880
C)90
D)3,628,800
A baseball manager has 10 players of the same ability. How many 9 player starting lineups can he create?
A)10
B)362,880
C)90
D)3,628,800

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck
80
Solve the problem.
A musician plans to perform 5 selections for a concert. If he can choose from 7 different selections, how many ways can he arrange his program?
A)16,807
B)35
C)21
D)2520
A musician plans to perform 5 selections for a concert. If he can choose from 7 different selections, how many ways can he arrange his program?
A)16,807
B)35
C)21
D)2520

Unlock Deck
Unlock for access to all 213 flashcards in this deck.
Unlock Deck
k this deck



