Deck 2: Functions and Graphs
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/359
Play
Full screen (f)
Deck 2: Functions and Graphs
1
Determine whether the relation is a function.
A) Function
B) Not a function
A) Function
B) Not a function
A
2
Give the domain and range of the relation.
A) domain: range:
B) domain: range:
C) domain: range:
D) domain: range:
A) domain: range:
B) domain: range:
C) domain: range:
D) domain: range:
A
3
Determine whether the relation is a function.
A) Function
B) Not a function
A) Function
B) Not a function
A
4
Give the domain and range of the relation.
A) domain ; range
B) domain ; range
C) domain range
D) domain ; range
A) domain ; range
B) domain ; range
C) domain range
D) domain ; range

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
5
Give the domain and range of the relation.
A) domain range
B) domain range
C) domain ; range
D) domain range
A) domain range
B) domain range
C) domain ; range
D) domain range

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
6
Give the domain and range of the relation.
A) domain ; range
B) domain ; range
C) domain ; range
D) domain ; range
A) domain ; range
B) domain ; range
C) domain ; range
D) domain ; range

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
7
Give the domain and range of the relation.
A) domain ; range
B) domain ; range
C) domain ; range
D) domain ; range
A) domain ; range
B) domain ; range
C) domain ; range
D) domain ; range

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
8
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
9
Determine whether the relation is a function.
A) Not a function
B) Function
A) Not a function
B) Function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
10
Determine whether the relation is a function.
A) Function
B) Not a function
A) Function
B) Not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
11
Determine whether the relation is a function.
A) Not a function
B) Function
A) Not a function
B) Function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
12
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
13
Give the domain and range of the relation.
A) domain: ; range:
B) domain: ; range:
C) domain: ; range:
D) domain: ; range:
A) domain: ; range:
B) domain: ; range:
C) domain: ; range:
D) domain: ; range:

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
14
Give the domain and range of the relation.
A) domain range
B) domain ; range
C) domain ; range
D) domain ; range
A) domain range
B) domain ; range
C) domain ; range
D) domain ; range

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
15
Determine whether the relation is a function.
A) Not a function
B) Function
A) Not a function
B) Function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
16
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
17
Determine whether the relation is a function.
A) Function
B) Not a function
A) Function
B) Not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
18
Determine whether the relation is a function.
A) Not a function
B) Function
A) Not a function
B) Function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
19
Determine whether the relation is a function.
A) Function
B) Not a function
A) Function
B) Not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
20
Determine whether the relation is a function.
A) Not a function
B) Function
A) Not a function
B) Function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
21
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
22
Evaluate the function at the given value of the independent variable and simplify.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
23
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
24
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
25
Evaluate the function at the given value of the independent variable and simplify.
A) 13
В)
C) 19
D)
A) 13
В)
C) 19
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
26
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
27
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
28
Evaluate the function at the given value of the independent variable and simplify.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
29
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
30
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not function of
A) is a function of
B) is not function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
31
Evaluate the function at the given value of the independent variable and simplify.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
32
Evaluate the function at the given value of the independent variable and simplify.
The total cost in dollars for a certain company to produce x empty jars to be used by a jelly producer is given by the function . Find , the cost of producing 50,000 jars.
A)
B)
C)
D)
The total cost in dollars for a certain company to produce x empty jars to be used by a jelly producer is given by the function . Find , the cost of producing 50,000 jars.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
33
Evaluate the function at the given value of the independent variable and simplify.
A) 4
В)
C) 2
D) not a real number
A) 4
В)
C) 2
D) not a real number

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
34
Evaluate the function at the given value of the independent variable and simplify.
A) 13
B) 33
C) 23
D)
A) 13
B) 33
C) 23
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
35
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
36
Evaluate the function at the given value of the independent variable and simplify.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
37
Evaluate the function at the given value of the independent variable and simplify.
The function models the relationship between the number of pretzels that a certain vendor sells and the profit the vendor makes. Find , the profit the vendor makes from selling 500 pretzels.
A) \$211
B)
C)
D)
The function models the relationship between the number of pretzels that a certain vendor sells and the profit the vendor makes. Find , the profit the vendor makes from selling 500 pretzels.
A) \$211
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
38
Evaluate the function at the given value of the independent variable and simplify.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
39
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
40
Determine whether the equation defines y as a function of x.
A) is a function of
B) is not a function of
A) is a function of
B) is not a function of

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
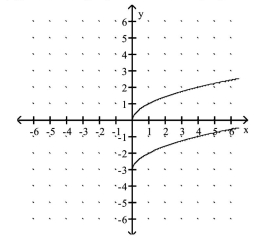
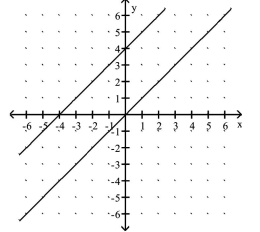
41
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) shifts the graph of vertically down 3 units
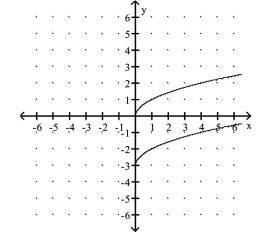
B) shifts the graph of vertically up 3 units
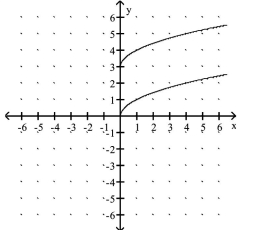
C) g shifts the graph of vertically down 3 units
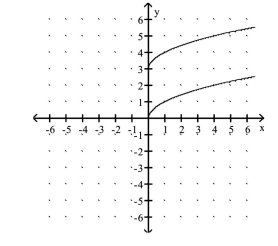
D) g shifts the graph of vertically up 3 units
graph of f.

A) shifts the graph of vertically down 3 units

B) shifts the graph of vertically up 3 units

C) g shifts the graph of vertically down 3 units

D) g shifts the graph of vertically up 3 units


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
42
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
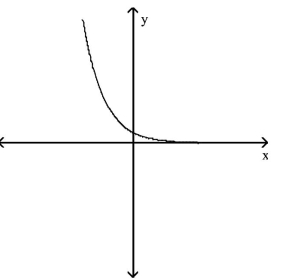
A) function
B) not a function

A) function
B) not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
43
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
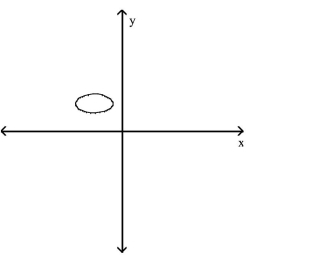
A) not a function
B) function

A) not a function
B) function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
44
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
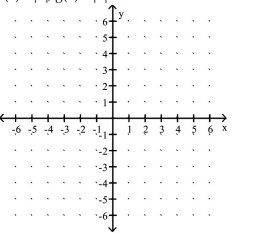
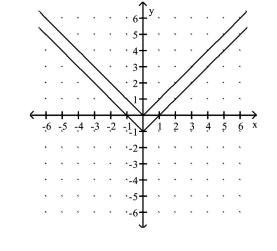
A) shifts the graph of vertically up 1 unit
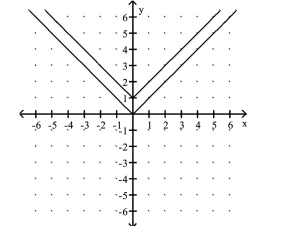
B) shifts the graph of vertically down 1 unit
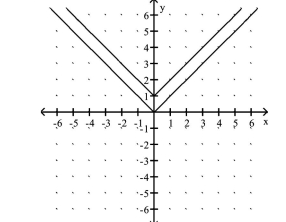
C) g shifts the graph of vertically down 1 unit
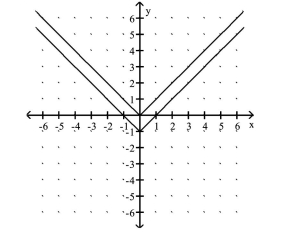
D) shifts the graph of vertically up 1 unit
graph of f.

A) shifts the graph of vertically up 1 unit

B) shifts the graph of vertically down 1 unit

C) g shifts the graph of vertically down 1 unit

D) shifts the graph of vertically up 1 unit


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
45
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
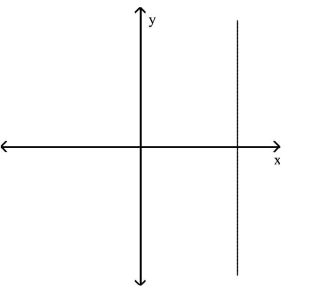
A)
B) function

A)
B) function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
46
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) shifts the graph of vertically up 4 units
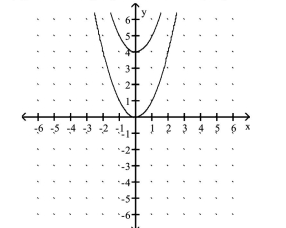
B) shifts the graph of vertically down 4 units
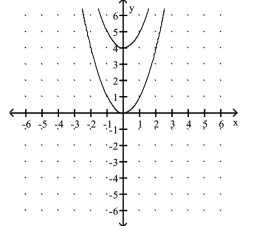
C) g shifts the graph of vertically down 4 units
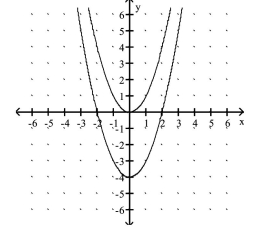
D) shifts the graph of vertically up 4 units
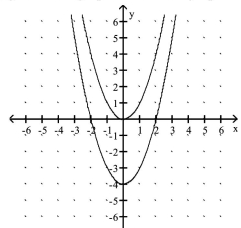
graph of f.

A) shifts the graph of vertically up 4 units

B) shifts the graph of vertically down 4 units

C) g shifts the graph of vertically down 4 units

D) shifts the graph of vertically up 4 units


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
47
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
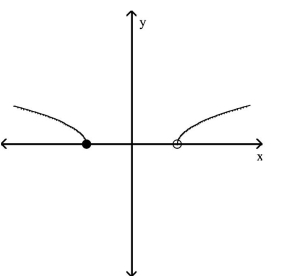
A) function
B) not a function

A) function
B) not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
48
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) shifts the graph of vertically up 3 units
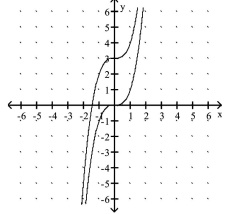
B) shifts the graph of vertically down 3 units
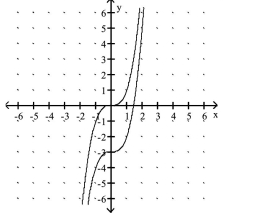
C) g shifts the graph of vertically up 3 units
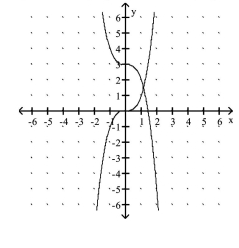
D) g shifts the graph of vertically down 3 units
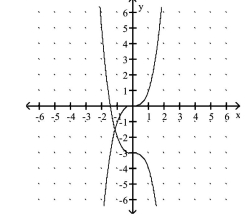
graph of f.

A) shifts the graph of vertically up 3 units

B) shifts the graph of vertically down 3 units

C) g shifts the graph of vertically up 3 units

D) g shifts the graph of vertically down 3 units


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
49
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) shifts the graph of vertically down 2 units
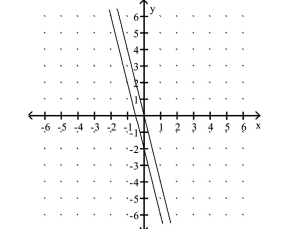
B) shifts the graph of vertically down 2 units
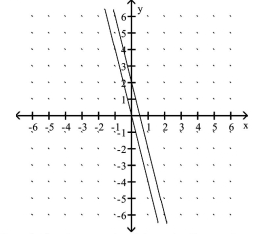
C) g shifts the graph of vertically up 2 units
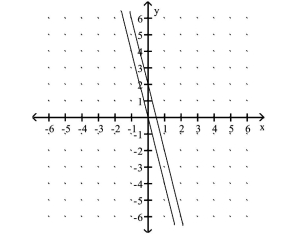
D) shifts the graph of vertically up 2 units
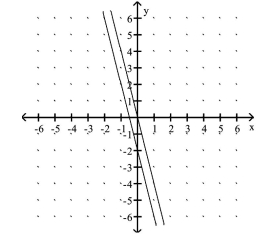
graph of f.

A) shifts the graph of vertically down 2 units

B) shifts the graph of vertically down 2 units

C) g shifts the graph of vertically up 2 units

D) shifts the graph of vertically up 2 units


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
50
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) shifts the graph of vertically down 4 units
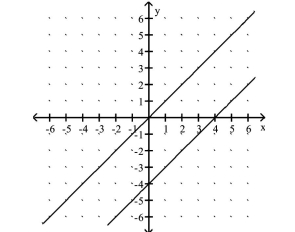
B) g shifts the graph of vertically down 4 units
C) shifts the graph of vertically up 4 units
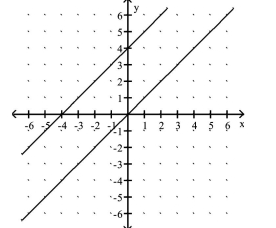
D) shifts the graph of vertically up 4 units
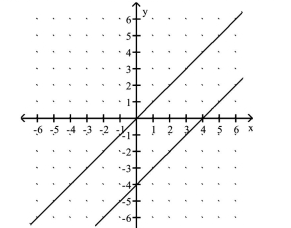
graph of f.

A) shifts the graph of vertically down 4 units

B) g shifts the graph of vertically down 4 units

C) shifts the graph of vertically up 4 units

D) shifts the graph of vertically up 4 units


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
51
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
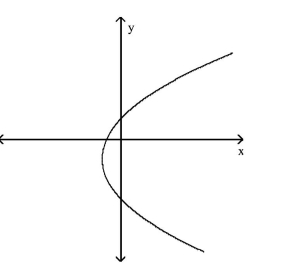
A)
B) function

A)
B) function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
52
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
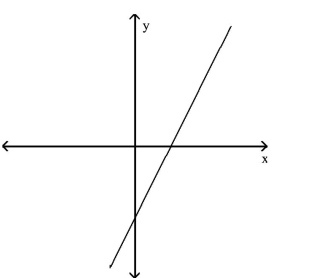
A) function
B) not a function

A) function
B) not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
53
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
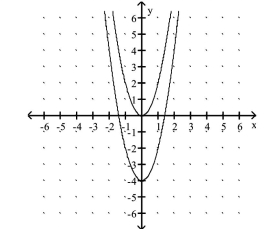
A) g shifts the graph of vertically down 4 units
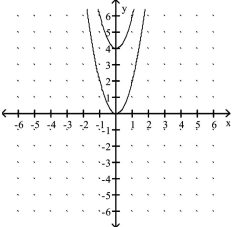
B) shifts the graph of vertically down 4 units
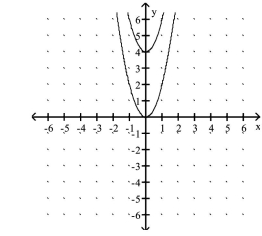
C) g shifts the graph of f vertically up 4 units
D) g shifts the graph of vertically up 4 units
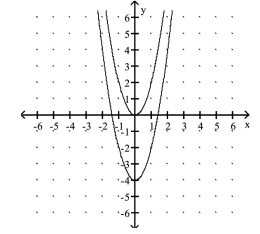
graph of f.

A) g shifts the graph of vertically down 4 units

B) shifts the graph of vertically down 4 units

C) g shifts the graph of f vertically up 4 units

D) g shifts the graph of vertically up 4 units


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
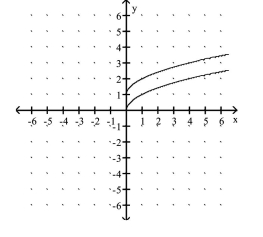
54
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) shifts the graph of unit to the left
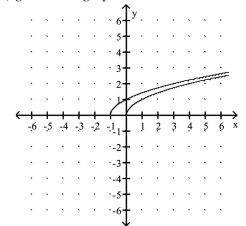
B) shifts the graph of unit to the right
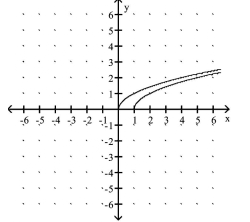
C) g shifts the graph of f vertically up 1 unit
D) g shifts the graph of vertically down 1 unit
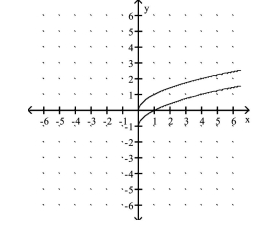
graph of f.

A) shifts the graph of unit to the left

B) shifts the graph of unit to the right

C) g shifts the graph of f vertically up 1 unit

D) g shifts the graph of vertically down 1 unit


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
55
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) shifts the graph of vertically up 4 units
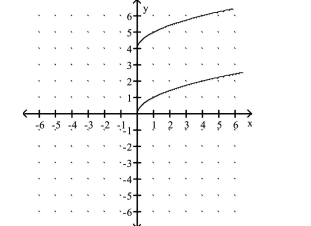
B) shifts the graph of vertically down 4 units
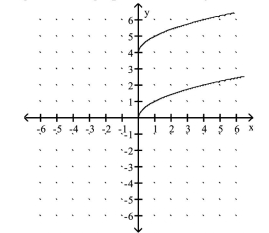
C) g shifts the graph of vertically down 4 units
D) g shifts the graph of vertically up 4 units
graph of f.

A) shifts the graph of vertically up 4 units

B) shifts the graph of vertically down 4 units

C) g shifts the graph of vertically down 4 units

D) g shifts the graph of vertically up 4 units


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
56
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
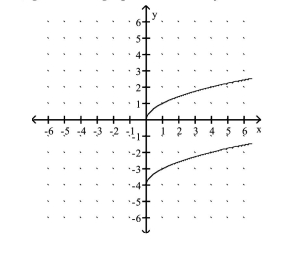
A) shifts the graph of vertically up 2 units
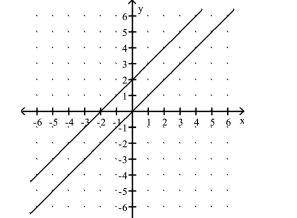
B) shifts the graph of vertically down 2 units
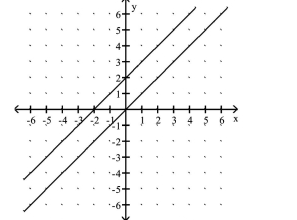
C) g shifts the graph of vertically down 2 units
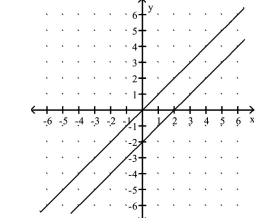
D) g shifts the graph of vertically up 2 units
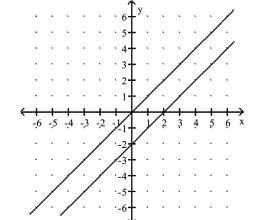
graph of f.

A) shifts the graph of vertically up 2 units

B) shifts the graph of vertically down 2 units

C) g shifts the graph of vertically down 2 units

D) g shifts the graph of vertically up 2 units


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
57
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) g shifts the graph of f vertically down 2 units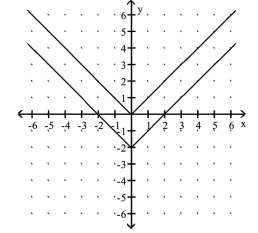
B) shifts the graph of f vertically up 2 units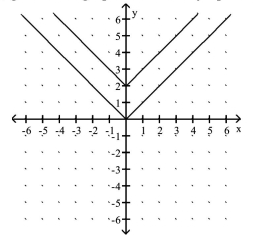
C) g shifts the graph of vertically down 2 units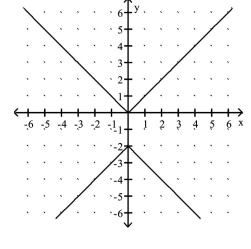
D) shifts the graph of vertically up 2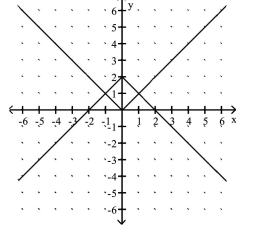
graph of f.

A) g shifts the graph of f vertically down 2 units

B) shifts the graph of f vertically up 2 units

C) g shifts the graph of vertically down 2 units

D) shifts the graph of vertically up 2


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
58
Graph the given functions on the same rectangular coordinate system. Describe how the graph of g is related to the
graph of f.
A) shifts the graph of unit to the right
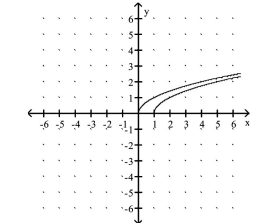
B) shifts the graph of unit to the left
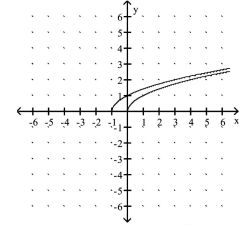
C) g shifts the graph of f vertically up 1 unit
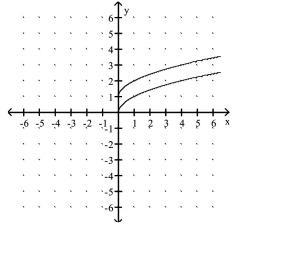
D) shifts the graph of vertically down 1 unit
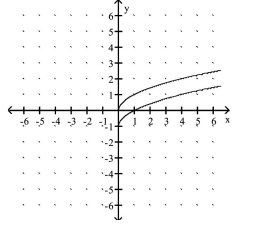
graph of f.

A) shifts the graph of unit to the right

B) shifts the graph of unit to the left

C) g shifts the graph of f vertically up 1 unit

D) shifts the graph of vertically down 1 unit


Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
59
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
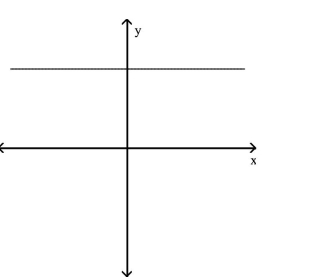
A) function
B) not a function

A) function
B) not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
60
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
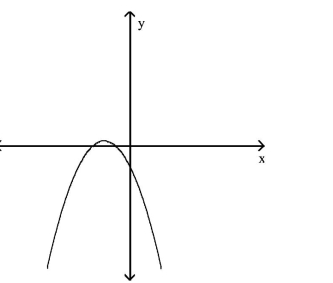
A) function
B) not a function

A) function
B) not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
61
The graph below shows the percentage of students enrolled in the College of Engineering at State University. Use
the graph to answer the question.
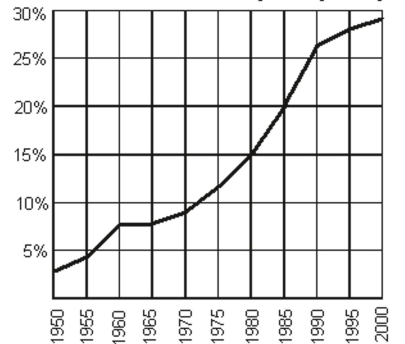
Does the graph represent a function?
A) yes
B) no
the graph to answer the question.

Does the graph represent a function?
A) yes
B) no

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
62
Use the graph to find the indicated function value.
Find
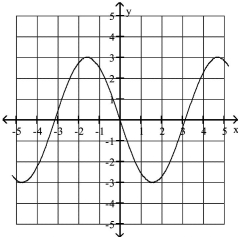
A)
B)
C)
D)
Find

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
63
The graph below shows the percentage of students enrolled in the College of Engineering at State University. Use
the graph to answer the question.

Between what two years is the difference in function values equal to 5%?
A) between 1980 and 1985
B) between 1985 and 1990
C) between 1970 and 1975
D) between 1960 and 1965
the graph to answer the question.

Between what two years is the difference in function values equal to 5%?
A) between 1980 and 1985
B) between 1985 and 1990
C) between 1970 and 1975
D) between 1960 and 1965

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
64
The graph below shows the percentage of students enrolled in the College of Engineering at State University. Use
the graph to answer the question.

If f(x) = 15%, what year is represented by x?
A) 1980
B) 1975
C) 1985
D) 1970
the graph to answer the question.

If f(x) = 15%, what year is represented by x?
A) 1980
B) 1975
C) 1985
D) 1970

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
65
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
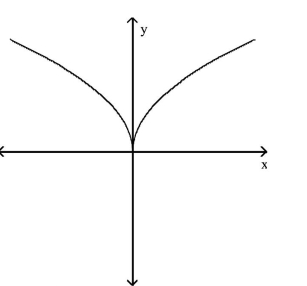
A) function
B) not a function

A) function
B) not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
66
Use the graph to find the indicated function value.
. Find .
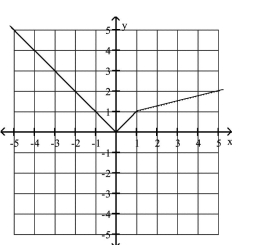
A)
B) 3
C) 9
D)
. Find .

A)
B) 3
C) 9
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
67
Identify the intercepts.
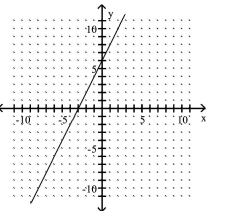
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
68
Identify the Domain and Range of a Function from Its Graph
Use the graph to determine the function's domain and range.
![<strong>Identify the Domain and Range of a Function from Its Graph Use the graph to determine the function's domain and range. </strong> A) domain: ( - \infty , \infty ) range: ( - \infty , 3 ] B) domain: ( - \infty , \infty ) range: ( - \infty , \infty ) C) domain: ( - \infty , 5 ] range: ( - \infty , 3 ] D) domain: ( - \infty , 5 ) or ( 5 , \infty ) range: ( - \infty , 3 ) or ( 3 , \infty )](https://d2lvgg3v3hfg70.cloudfront.net/TB7044/11ecb7f3_e913_39ec_b9c9_efdc27f5ea73_TB7044_00.jpg)
A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain: or
range: or
Use the graph to determine the function's domain and range.
![<strong>Identify the Domain and Range of a Function from Its Graph Use the graph to determine the function's domain and range. </strong> A) domain: ( - \infty , \infty ) range: ( - \infty , 3 ] B) domain: ( - \infty , \infty ) range: ( - \infty , \infty ) C) domain: ( - \infty , 5 ] range: ( - \infty , 3 ] D) domain: ( - \infty , 5 ) or ( 5 , \infty ) range: ( - \infty , 3 ) or ( 3 , \infty )](https://d2lvgg3v3hfg70.cloudfront.net/TB7044/11ecb7f3_e913_39ec_b9c9_efdc27f5ea73_TB7044_00.jpg)
A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain: or
range: or

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
69
Identify the Domain and Range of a Function from Its Graph
Use the graph to determine the function's domain and range.
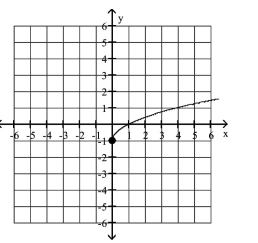
A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain:
range:
Use the graph to determine the function's domain and range.

A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain:
range:

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
70
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
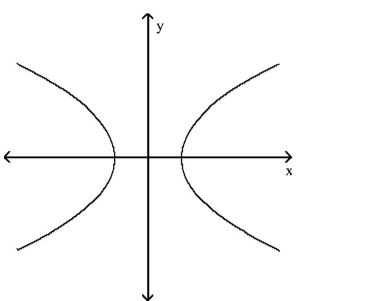
A) not a function
B) function

A) not a function
B) function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
71
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
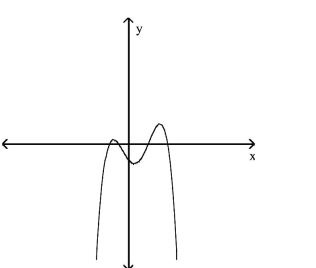
A) function
B) not a function

A) function
B) not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
72
Identify the Domain and Range of a Function from Its Graph
Use the graph to determine the function's domain and range.
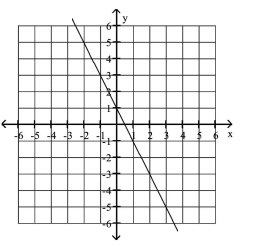
A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain:
range:
Use the graph to determine the function's domain and range.

A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain:
range:

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
73
Identify the Domain and Range of a Function from Its Graph
Use the graph to determine the function's domain and range.
![<strong>Identify the Domain and Range of a Function from Its Graph Use the graph to determine the function's domain and range. </strong> A) domain: ( - \infty , \infty ) range: [ 0,5 ] B) domain: ( - \infty , \infty ) range: [ 3,5 ] C) domain: [ 0,5 ] range: ( - \infty , \infty ) D) domain: [ 3,5 ] range: ( - \infty , \infty )](https://d2lvgg3v3hfg70.cloudfront.net/TB7044/11ecb7f4_1405_cb4e_b9c9_239062ea1201_TB7044_00.jpg)
A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain:
range:
Use the graph to determine the function's domain and range.
![<strong>Identify the Domain and Range of a Function from Its Graph Use the graph to determine the function's domain and range. </strong> A) domain: ( - \infty , \infty ) range: [ 0,5 ] B) domain: ( - \infty , \infty ) range: [ 3,5 ] C) domain: [ 0,5 ] range: ( - \infty , \infty ) D) domain: [ 3,5 ] range: ( - \infty , \infty )](https://d2lvgg3v3hfg70.cloudfront.net/TB7044/11ecb7f4_1405_cb4e_b9c9_239062ea1201_TB7044_00.jpg)
A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain:
range:

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
74
Identify the Domain and Range of a Function from Its Graph
Use the graph to determine the function's domain and range.
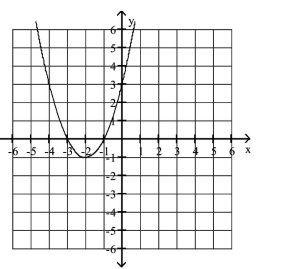
A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain: or
range: or
Use the graph to determine the function's domain and range.

A) domain:
range:
B) domain:
range:
C) domain:
range:
D) domain: or
range: or

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
75
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
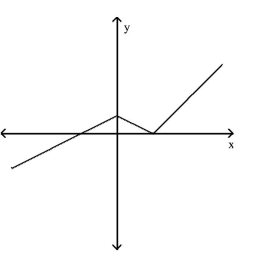
A) function
B) not a function

A) function
B) not a function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
76
Use the graph to find the indicated function value.
. Find
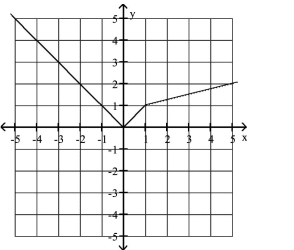
A) 2
В)
C) 5
D)
. Find

A) 2
В)
C) 5
D)

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
77
Use the graph to find the indicated function value.
. Find
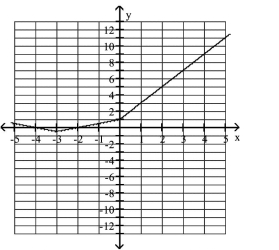
A) 11
B) 9
C)
D) 2
. Find

A) 11
B) 9
C)
D) 2

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
78
Use the graph to find the indicated function value.
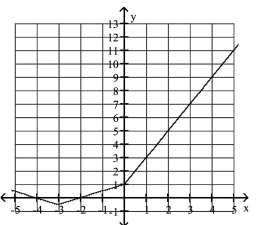
A) 0
B) 9
C) 4
D) 3

A) 0
B) 9
C) 4
D) 3

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
79
Use the vertical line test to determine whether or not the graph is a graph in which y is a function of x.
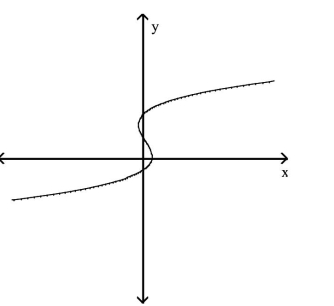
A) not a function
B) function

A) not a function
B) function

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck
80
The graph below shows the percentage of students enrolled in the College of Engineering at State University. Use
the graph to answer the question.

If f represents the function, find f(1980).
A) approximately 15%
B) approximately 17%
C) approximately 11.5%
D) approximately 10%
the graph to answer the question.

If f represents the function, find f(1980).
A) approximately 15%
B) approximately 17%
C) approximately 11.5%
D) approximately 10%

Unlock Deck
Unlock for access to all 359 flashcards in this deck.
Unlock Deck
k this deck



