Deck 5: Exponential and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/304
Play
Full screen (f)
Deck 5: Exponential and Logarithmic Functions
1
Graph the function.
-
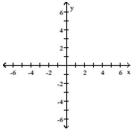
A)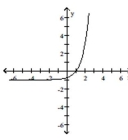
B)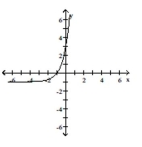
C)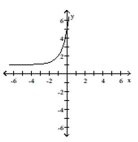
D)
-

A)

B)

C)

D)


2
Graph the function.
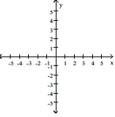
A)
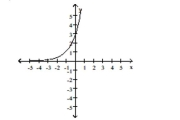
B)
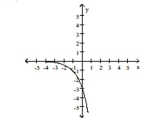
C)
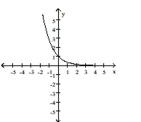
D)
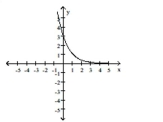

A)

B)

C)

D)

D
3
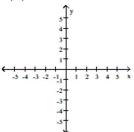
A)
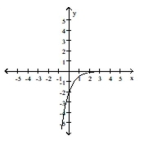
B)
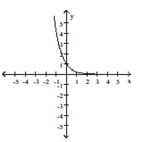
C)
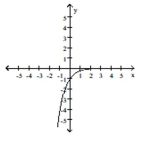
D)
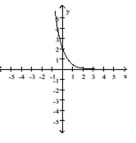
D
4
Determine whether or not the given function is an exponential function.
A) exponential
B) not exponential
A) exponential
B) not exponential

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
5
Graph the function.
-
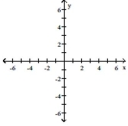
A)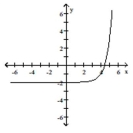
B)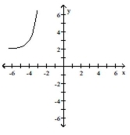
C)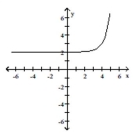
D)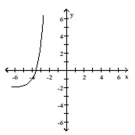
-

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
6
Determine if the function is a growth exponential or a decay exponential.
A) Growth
B) Decay
A) Growth
B) Decay

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
7
Determine whether or not the given function is an exponential function.
A) exponential
B) not exponential
A) exponential
B) not exponential

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
8
Determine whether or not the given function is an exponential function.
A) exponential
B) not exponential
A) exponential
B) not exponential

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
9
Determine if the function is a growth exponential or a decay exponential.
A) Growth
B) Decay
A) Growth
B) Decay

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
10
Determine if the function is a growth exponential or a decay exponential.
A) Growth
B) Decay
A) Growth
B) Decay

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
11
Graph the function.
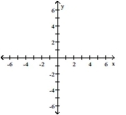
A)
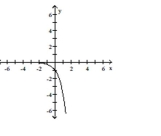
B)
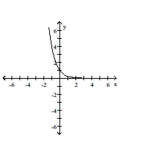
C)
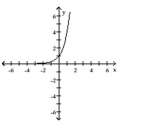
D)
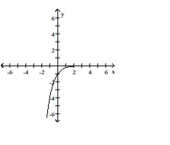

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
12
Determine whether or not the given function is an exponential function.
y
A) exponential
B) not exponential
y
A) exponential
B) not exponential

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
13
Graph the function.
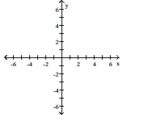
A)
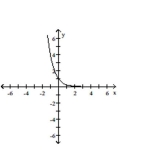
B)
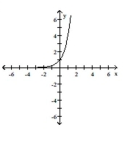
C)
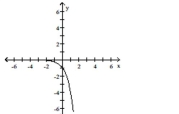
D)
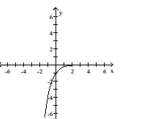

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
14
Graph the function.
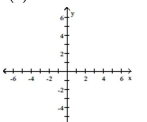
A)
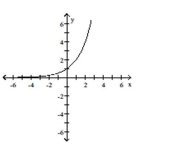
B)
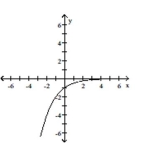 C)
C)
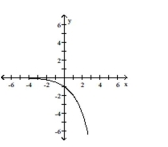
D)
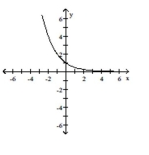

A)

B)
 C)
C)
D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
15
Determine whether or not the given function is an exponential function.
A) exponential
B) not exponential
A) exponential
B) not exponential

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
16
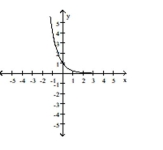
Graph the function.
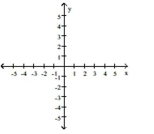
A)
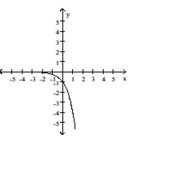
B)
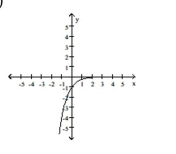
C)
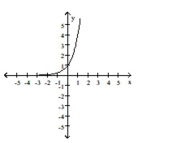
D)

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
17
Determine whether or not the given function is an exponential function.
A) exponential
B) not exponential
A) exponential
B) not exponential

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
18
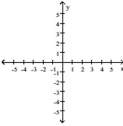
A)
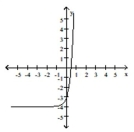
B)
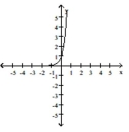
C)
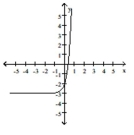
D)
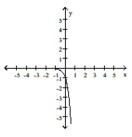

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
19
Determine if the function is a growth exponential or a decay exponential.
A) Growth
B) Decay
A) Growth
B) Decay

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
20
Graph the function.
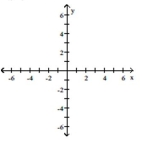
A)
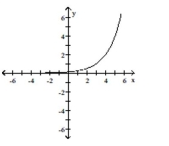
B)
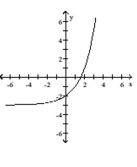
C)
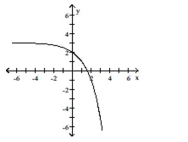
D)
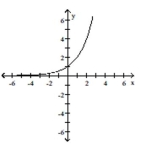

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
21
Find the function value.
Let . Find , rounded to four decimal places.
A)
B) -6.1143
C)
D)
Let . Find , rounded to four decimal places.
A)
B) -6.1143
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
22
Match the equation with its graph.
A)
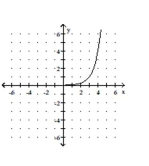
B)
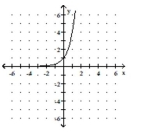
C)
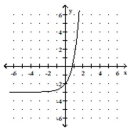
D)
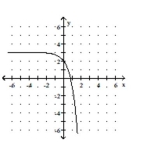
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
23
In September 1998 the population of the country of West Goma in millions was modeled by . At the same time the population of East Goma in millions was modeled by . In both formulas is the year, where corresponds to September 1998. Assuming these trends continue, estimate what the population will be when the populations are equal.
A) 18 million
B) 1 million
C) 16 million
D) 17 million
A) 18 million
B) 1 million
C) 16 million
D) 17 million

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
24
In September 1998 the population of the country of West Goma in millions was modeled by . At the same time the population of East Goma in millions was modeled by . In both formulas is the year, where corresponds to September 1998 . Assuming these trends continue, estimate the year when the population of West Goma will equal the population of East Goma.
A) 21
B) 1977
C) 2019
D) 2016
A) 21
B) 1977
C) 2019
D) 2016

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
25
Match the equation with its graph.
A)
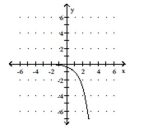
B)
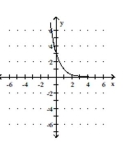
C)
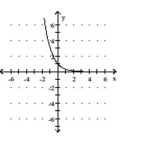
D)
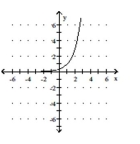
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
26
Match the equation with its graph.
A)
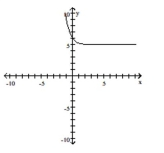
B)
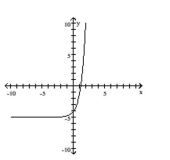
C)
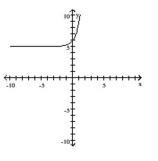
D)
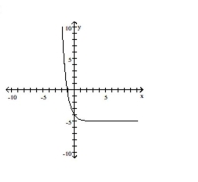
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
27
Find the function value.
Let . Find .
A)
B)
C) 36
D)
Let . Find .
A)
B)
C) 36
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
28
Find the function value.
Let . Find , rounded to four decimal places.
A)
B)
C)
D)
Let . Find , rounded to four decimal places.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
29
Find the function value.
A) 1.4347
B) 10.6013
C) 49.4024
D) 0.0202
A) 1.4347
B) 10.6013
C) 49.4024
D) 0.0202

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
30
The sales of a mature product (one which has passed its peak) will decline by the function , where is time in years. Find the sales after 8 years if and .
A) 58,065
B) 5746
C) 245,075
D) 11,491
A) 58,065
B) 5746
C) 245,075
D) 11,491

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
31
A)
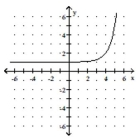
B)
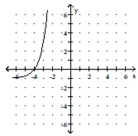
C)
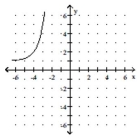
D)
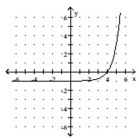

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
32
Find the function value.
Let . Find .
A) 9
B)
C)
D)
Let . Find .
A) 9
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
33
Match the equation with its graph.
A)
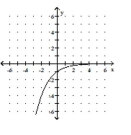
B)
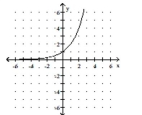
C)
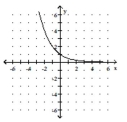
D)
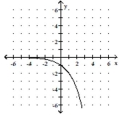
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
34
A)
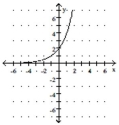
B)
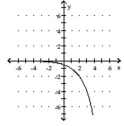
C)
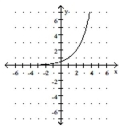
D)
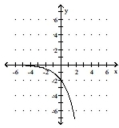

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
35
Find the function value.
Let . Find .
A) 216
B)
C)
D)
Let . Find .
A) 216
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
36
Match the equation with its graph.
A)
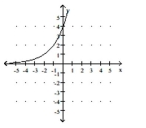
B)
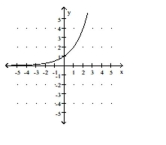
C)
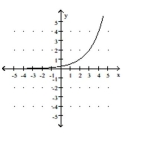
D)
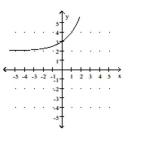
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
37
The growth in the population of a certain rodent at a dump site fits the exponential function where t is the number of years since 1965. Estimate the population in the year 2000.
A) 96
B) 195
C) 104
D) 192
A) 96
B) 195
C) 104
D) 192

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
38
Find the function value.
A) 27
B) 8
C) 9
D) 6
A) 27
B) 8
C) 9
D) 6

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
39
Match the equation with its graph.
A)
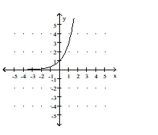
B)
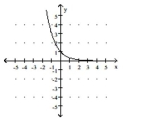
C)
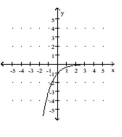
D)
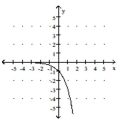
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
40
Find the function value.
Let . Find .
A)
B)
C)
D)
Let . Find .
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
41
Give a definition for the following term: Exponential function .

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
42
Provide an appropriate response.
What are the domain and range for the equation ?
A) Domain: ; range:
B) Domain: ; range:
C) Domain: ; range:
D) ; range:
What are the domain and range for the equation ?
A) Domain: ; range:
B) Domain: ; range:
C) Domain: ; range:
D) ; range:

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
43
The population of a small country increases according to the function where t is measured in years. How many people will the country have after 3 years?
A) 3,054,622
B) 7,033,527
C) 2,870,384
D) 2,654,591
A) 3,054,622
B) 7,033,527
C) 2,870,384
D) 2,654,591

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
44
Explain how the graph of .

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
45
Explain how the graph of

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
46
Provide an appropriate response.
Explain why f(x) is not.
Explain why f(x) is not.

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
47
Write the logarithmic equation in exponential form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
48
A computer is purchased for $4100. Its value each year is about 75% of the value the preceding year. Its value, in dollars, after t years is given by the exponential function Find the value of the computer after
2 years.
A) $1297.27
B) $1729.69
C) $2306.25
D) $6150.00
2 years.
A) $1297.27
B) $1729.69
C) $2306.25
D) $6150.00

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
49
The number of acres in a landfill is given by the function where t is measured in years. How many acres will the landfill have after 8 years? (Round to the nearest acre.)
A) 2657 acres
B) 1944 acres
C) 1154 acres
D) 1155 acres
A) 2657 acres
B) 1944 acres
C) 1154 acres
D) 1155 acres

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
50
Provide an appropriate response.
Why can'
Why can'

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
51
Provide an appropriate response.
Why is an exponential function one-to-one?
Why is an exponential function one-to-one?

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
52
What is the range of the function ?
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
53
The amount of a certain radioactive isotope present at time t is given by grams, where t is the time in years that the isotope decays. The initial amount present is 400 grams. How many grams remain
After 20 years? Round to the nearest hundredth.
A) 681.48 g
B) 234.78 g
C) 379.25 g
D) 397.87 g
After 20 years? Round to the nearest hundredth.
A) 681.48 g
B) 234.78 g
C) 379.25 g
D) 397.87 g

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
54
Provide an appropriate response.
With the exponential function f(x)
With the exponential function f(x)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
55
Write the logarithmic equation in exponential form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
56
The half-life of Titanium 45 is hours. If the formula gives the percent (as a decimal) remaining after time (in hours), sketch versus t.

A)
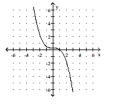
B)
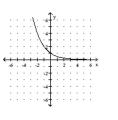
C)
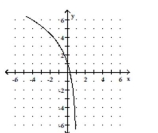
D)
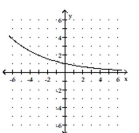

A)

B)

C)

D)


Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
57
Explain how the graph of

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
58
The number of books in a small library increases according to the function B = 2700e0.05t, where t is measured in years. How many books will the library have after 4 years?
A) 3298 books
B) 4279 books
C) 4345 books
D) 1887 books
A) 3298 books
B) 4279 books
C) 4345 books
D) 1887 books

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
59
Provide an appropriate response.
What is one ordered pair that is always on the graph of f( ?
What is one ordered pair that is always on the graph of f( ?

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
60
What is the domain of the function ?
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
61
Write in logarithmic form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
62
Evaluate the logarithm, if possible. Round the answer to four decimal places.
ln 0.000873 A)
B)
C)
D)
ln 0.000873 A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
63
Write the logarithmic equation in exponential form.
A)
B) No solution
C)
D)
A)
B) No solution
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
64
Evaluate the logarithm, if possible. Round the answer to four decimal places.
log 2.81
A) 0.4487
B) 0.4639
C) 1.0332
D) 0.4330
log 2.81
A) 0.4487
B) 0.4639
C) 1.0332
D) 0.4330

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
65
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
66
Write the logarithmic equation in exponential form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
67
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
68
Write the logarithmic equation in exponential form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
69
Evaluate the logarithm, if possible. Round the answer to four decimal places.
log 169
A) 5.1299
B) 2.2279
C) 2.2253
D) 2.2304
log 169
A) 5.1299
B) 2.2279
C) 2.2253
D) 2.2304

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
70
Evaluate the logarithm, if possible. Round the answer to four decimal places.
ln 5,500,000
A) 4.0073
B) 15.5203
C) 6.7404
D) 0.0642
ln 5,500,000
A) 4.0073
B) 15.5203
C) 6.7404
D) 0.0642

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
71
Evaluate the logarithm, if possible. Round the answer to four decimal places.
ln 0.994
A) 0.0060
B) 0.0026
C) -0.0026
D) -0.0060
ln 0.994
A) 0.0060
B) 0.0026
C) -0.0026
D) -0.0060

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
72
Write in logarithmic form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
73
Write in logarithmic form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
74
Write in logarithmic form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
75
Evaluate the logarithm, if possible. Round the answer to four decimal places.
log 4508
A) 3.6549
B) 3.6530
C) 3.6540
D) 8.4136
log 4508
A) 3.6549
B) 3.6530
C) 3.6540
D) 8.4136

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
76
Write the logarithmic equation in exponential form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
77
Evaluate the logarithm, if possible. Round the answer to four decimal places.
log 0.0743
A)
В)
C)
D)
log 0.0743
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
78
Write in logarithmic form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
79
Evaluate the logarithm, if possible. Round the answer to four decimal places.
log 0.00474
A)
B)
C)
D)
log 0.00474
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck
80
Write in logarithmic form.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 304 flashcards in this deck.
Unlock Deck
k this deck



