Deck 4: Additional Topics With Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/299
Play
Full screen (f)
Deck 4: Additional Topics With Functions
1
A)
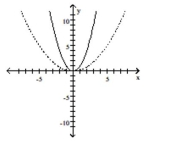
B)
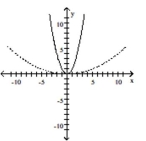 C)
C)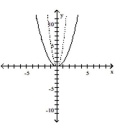
D)
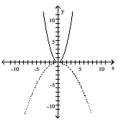
A
2
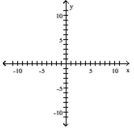
A)
B)
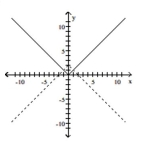
C)
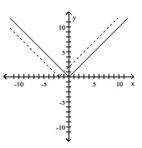
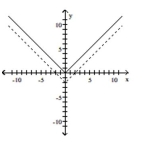
D)
D
3
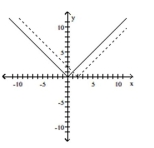
A)
B)
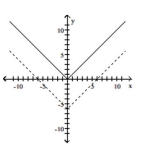
C)
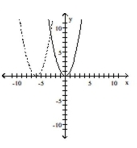
D)
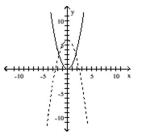
A
4
Fill in each blank with the appropriate response.
The graph of can be obtained from the graph of by shifting horizontallyــــــــــ units to theـــــــــــ , vertically stretching by a factor ofــــــــــ , reflecting across the ــــــــــــ-axis, and shifting vertically ـــــــــunits in the ــــــــdirection.
A) 2; right; 8; y; 6; downward
B) 2; right; 8; x; 6; upward
C) 2; right; 6; x; 8; upward
D) 2; left; 6; x; 8; upward
The graph of can be obtained from the graph of by shifting horizontallyــــــــــ units to theـــــــــــ , vertically stretching by a factor ofــــــــــ , reflecting across the ــــــــــــ-axis, and shifting vertically ـــــــــunits in the ــــــــdirection.
A) 2; right; 8; y; 6; downward
B) 2; right; 8; x; 6; upward
C) 2; right; 6; x; 8; upward
D) 2; left; 6; x; 8; upward

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
5
Fill in each blank with the appropriate response.
The graph of can be obtained from the graph of by shifting horizontallyــــــــــ units to theـــــــــ , vertically stretching by a factor ofـــــــــــــــ and shifting vertically ــــــــــــunits in the directionـــــــــــــــــ.
A) 1; left; 3; 2; downward
B) 1; left; 2; 3; downward
C) 1; right; 2; 3; downward
D) 1; right; 3; 2; upward
The graph of can be obtained from the graph of by shifting horizontallyــــــــــ units to theـــــــــ , vertically stretching by a factor ofـــــــــــــــ and shifting vertically ــــــــــــunits in the directionـــــــــــــــــ.
A) 1; left; 3; 2; downward
B) 1; left; 2; 3; downward
C) 1; right; 2; 3; downward
D) 1; right; 3; 2; upward

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
6
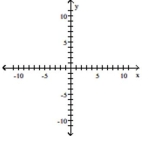
A)
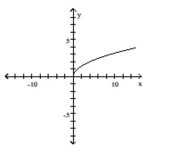
B)
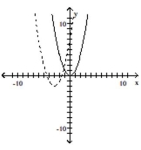 C)
C)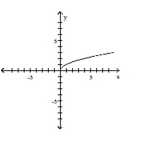
D)
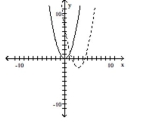

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
7

A)
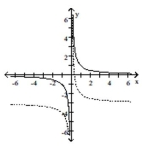
B)
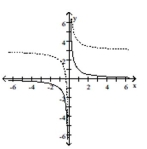
C)
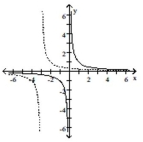
D)
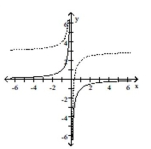

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
8
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{x}+5 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_1884_5085_8a2d_69b69ad7c3b2_TB6590_00.jpg)
A)
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{x}+5 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_2051_d7b6_8a2d_695ed792e931_TB6590_00.jpg)
B)
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{x}+5 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_256b_8987_8a2d_875ca5dfb2b0_TB6590_00.jpg)
C)
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{x}+5 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_2b02_81a8_8a2d_c3e94004f6e5_TB6590_00.jpg)
D)
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{x}+5 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_304f_5169_8a2d_933073049e70_TB6590_00.jpg)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
9
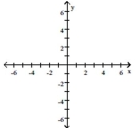
A)
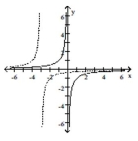
B)
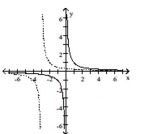
C)
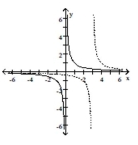
D)
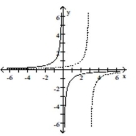

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
10
Fill in each blank with the appropriate response.
-The graph of can be obtained from the graph of by shifting horizontally units to the vertically compressing by a factor of , reflecting across the -axis, and shifting vertically units in the direction.
A) left; downward
B) 3 ; right; downward
C) left; downward
D) 3 ; right; ; upward
-The graph of can be obtained from the graph of by shifting horizontally units to the vertically compressing by a factor of , reflecting across the -axis, and shifting vertically units in the direction.
A) left; downward
B) 3 ; right; downward
C) left; downward
D) 3 ; right; ; upward

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
11
Fill in each blank with the appropriate response.
The graph of can be obtained from the graph of by shifting horizontallyــــــــ units to the , vertically stretching by a factor ofــــــــ , reflecting across theـــــــــــ -axis, and shifting vertically ــــــــــــunits in the ــــــــــــdirection.
A) 3; right; 5; x; 7; downward
B) 3; right; 5; x; 7; upward
C) 3; left; 7; x; 5; downward
D) 3; left; 5; x; 7; downward
The graph of can be obtained from the graph of by shifting horizontallyــــــــ units to the , vertically stretching by a factor ofــــــــ , reflecting across theـــــــــــ -axis, and shifting vertically ــــــــــــunits in the ــــــــــــdirection.
A) 3; right; 5; x; 7; downward
B) 3; right; 5; x; 7; upward
C) 3; left; 7; x; 5; downward
D) 3; left; 5; x; 7; downward

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
12
A)
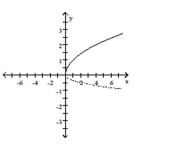
B)
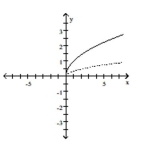
C)
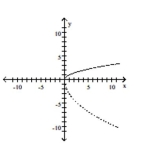
D)
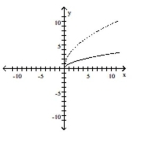

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
13
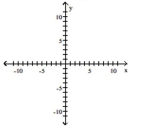
A)
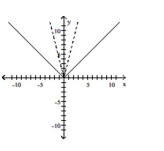
B)
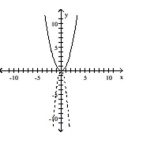
C)
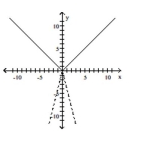
D)
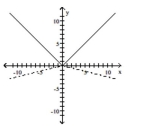

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
14
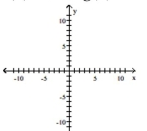
A)
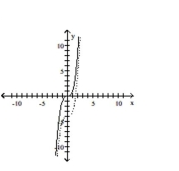
B)
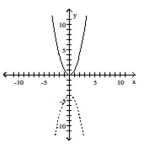
C)
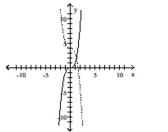
D)
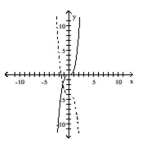

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
15
Fill in each blank with the appropriate response.
The graph of can be obtained from the graph of by vertically stretching by a factor ofــــــــ and reflecting across the ــــــــــ-axis.
A)
B)
C)
D)
The graph of can be obtained from the graph of by vertically stretching by a factor ofــــــــ and reflecting across the ــــــــــ-axis.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
16
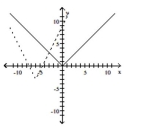
A)
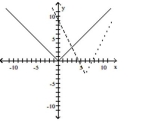
B)
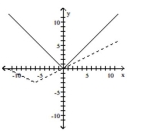
C)
D)
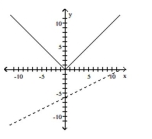

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
17
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{-x}+4 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_424e_406a_8a2d_e703f8ac8251_TB6590_00.jpg)
A)
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{-x}+4 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_477e_ae8b_8a2d_4b4b2764c040_TB6590_00.jpg)
B)
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{-x}+4 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_4e61_397d_8a2d_3f141d406554_TB6590_00.jpg)
C)
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{-x}+4 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_5cb2_62be_8a2d_51f7a8b11c60_TB6590_00.jpg)
D)
![<strong> f(x)=\sqrt[3]{x}, g(x)=\sqrt[3]{-x}+4 </strong> A) B) C) D)](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb661_618b_d73f_8a2d_d1569f2ceb0e_TB6590_00.jpg)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
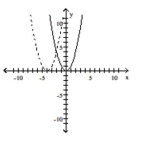
18
A)
B)
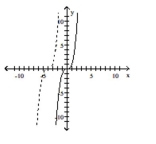
C)
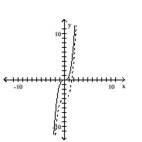
D)
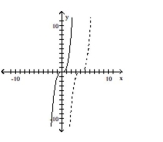

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
19
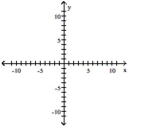
A)
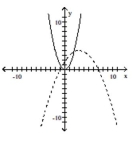
B)
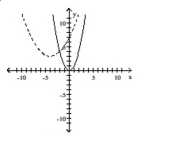
C )
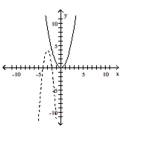
D)
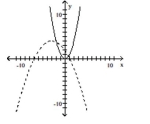

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
20
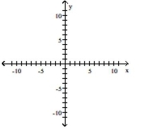
A)
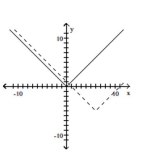
B)
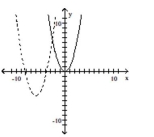
C)
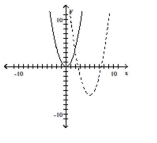
D)
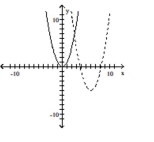

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
21
The graph of y= is shifted 3.5 units to the left. This graph is then vertically stretched by a factor of 5.1. Finally, the graph is reflected across the x -axis.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
22
A) x -axis
B) y -axis
C) No symmetry
D) Origin

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
23
Write the equation of the graph after the indicated transformation(s).
The graph of is shifted 3 units to the left. This graph is then vertically compressed by a factor of and reflected across the -axis. Finally, the graph is shifted 7 units downward.
A)
B)
C)
D)
The graph of is shifted 3 units to the left. This graph is then vertically compressed by a factor of and reflected across the -axis. Finally, the graph is shifted 7 units downward.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
24
Write the equation of the graph after the indicated transformation(s).
The graph of is shifted 5 units to the left and 4 units downward.
A)
B)
C)
D)
The graph of is shifted 5 units to the left and 4 units downward.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
25
Write the equation of the function g(x) that is transformed from the given function f(x), and whose graph is shown.
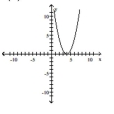
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
26
Fill in each blank with the appropriate response.
The graph of can be obtained from the graph of by shifting horizontally ـــــــــunits to theـــــــــ , and shifting vertically ـــــــــــunits in theــــــــــــــ direction.
A) 3; right; 4 downward
B) 3; right; 4; upward
C) 4; right; 3; upward
D) 4; left; 3; upward
The graph of can be obtained from the graph of by shifting horizontally ـــــــــunits to theـــــــــ , and shifting vertically ـــــــــــunits in theــــــــــــــ direction.
A) 3; right; 4 downward
B) 3; right; 4; upward
C) 4; right; 3; upward
D) 4; left; 3; upward

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
27
Write the equation of the function g(x) that is transformed from the given function f(x), and whose graph is shown.
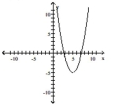
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
28
A) x -axis
B) Origin
C) No symmetry
D) y -axis

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
29
A) x -axis
B) x -axis, y -axis
C) x -axis, origin
D) Origin

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
30
Write the equation of the function g(x) that is transformed from the given function f(x), and whose graph is shown.
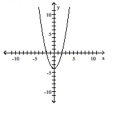
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
31
Write the equation of the function g(x) that is transformed from the given function f(x), and whose graph is shown.
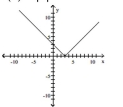
A)
B)
C)
D)

A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
32
Write the equation of the graph after the indicated transformation(s).
The graph of is vertically stretched by a factor of 2 , and the resulting graph is reflected across the -axis.
A)
B)
C)
D)
The graph of is vertically stretched by a factor of 2 , and the resulting graph is reflected across the -axis.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
33
A) y -axis, origin
B) x -axis, y -axis, origin
C) Origin
D) x -axis

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
34
Determine whether the graph of the given equation is symmetric with respect to the x-axis, the y-axis, and/or the origin.
A) No symmetry
B) Origin
C) x-axis
D) y-axis
A) No symmetry
B) Origin
C) x-axis
D) y-axis

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
35
Write the equation of the graph after the indicated transformation(s).
The graph of is shifted 2 units to the left. This graph is then vertically stretched by a factor of 6 and reflected across the -axis. Finally, the graph is shifted 8 units downward.
A)
B)
C)
D)
The graph of is shifted 2 units to the left. This graph is then vertically stretched by a factor of 6 and reflected across the -axis. Finally, the graph is shifted 8 units downward.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
36
The graph of y= x3 is shifted 3.0 units to the right and then vertically compressed by a factor of 0.7 .
A) y=3.0(x-0.7)3
B) y=0.7 x3+3.0
C) y=0.7(x+3.0)3
D) y=0.7(x-3.0)3
A) y=3.0(x-0.7)3
B) y=0.7 x3+3.0
C) y=0.7(x+3.0)3
D) y=0.7(x-3.0)3

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
37
The graph of is shifted 4 units to the left. Then the graph is shifted 9 units upward.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
38
Write the equation of the graph after the indicated transformation(s).
The graph of is shifted 4 units to the right. This graph is then vertically stretched by a factor of 6 and reflected across the -axis. Finally, the graph is shifted 8 units upward.
A)
B)
C)
D)
The graph of is shifted 4 units to the right. This graph is then vertically stretched by a factor of 6 and reflected across the -axis. Finally, the graph is shifted 8 units upward.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
39
Determine whether the graph of the given equation is symmetric with respect to the x-axis, the y-axis, and/or the origin.
A) No symmetry
B) y-axis
C) x-axis
D) Origin
A) No symmetry
B) y-axis
C) x-axis
D) Origin

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
40
The graph of is shifted 4 units to the right.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
41
Determine if the complete graph represents a function that is even, odd, or neither.
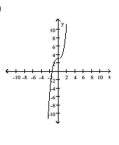
A) odd
B) neither
C) even

A) odd
B) neither
C) even

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
42
Determine whether the function is even, odd, or neither.
A) Odd
B) Neither
C) Even
A) Odd
B) Neither
C) Even

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
43
Determine if the complete graph represents a function that is even, odd, or neither.
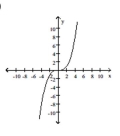
A) neither
B) even
C) odd

A) neither
B) even
C) odd

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
44
Determine whether the function is even, odd, or neither.
A) Even
B) Neither
C) Odd
A) Even
B) Neither
C) Odd

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
45
Determine whether the function is even, odd, or neither.
A) Odd
B) Neither
C) Even
A) Odd
B) Neither
C) Even

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
46
Determine whether the function is even, odd, or neither.
A) Odd
B) Neither
C) Even
A) Odd
B) Neither
C) Even

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
47
Determine whether the function is even, odd, or neither.
A) Odd
B) Neither
C) Even
A) Odd
B) Neither
C) Even

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
48
The year y when sales were s million dollars for a particular electronics company can be modeled by the radical equation , where represents 2010 , and so on. Use the model to predict the sales for 2015 to the nearest tenth of a million.
A) $89.2 million
B) $87.2 million
C) $90.2 million
D) $88.2 million
A) $89.2 million
B) $87.2 million
C) $90.2 million
D) $88.2 million

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
49
A manufacturer's cost is given by , where is the cost and is the number of parts produced. Describe the transformations needed to obtain the graph of this function from the graph of .
A) Stretch vertically by a factor of 500 and shift up 512 units.
B) Stretch vertically by a factor of 500 and shift 512 units to the left.
C) Stretch vertically by a factor of 500 and shift 512 units to the right.
D) Shift 500 units to the left and shift up 512 units.
A) Stretch vertically by a factor of 500 and shift up 512 units.
B) Stretch vertically by a factor of 500 and shift 512 units to the left.
C) Stretch vertically by a factor of 500 and shift 512 units to the right.
D) Shift 500 units to the left and shift up 512 units.

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
50
The price per unit of a product is and the number of units of the product is denoted by . The demand function for this commodity is given by . Describe the transformations needed to obtain the graph of this function from the graph of .
A) Stretch vertically by a factor of 20,000 and shift 50 units to the right.
B) Stretch vertically by a factor of 20,000 and shift down 50 units.
C) Shift up 20,000 units and shift 50 units to the right.
D) Stretch vertically by a factor of 20,000, reflect across the x-axis, and shift down 50 units.
A) Stretch vertically by a factor of 20,000 and shift 50 units to the right.
B) Stretch vertically by a factor of 20,000 and shift down 50 units.
C) Shift up 20,000 units and shift 50 units to the right.
D) Stretch vertically by a factor of 20,000, reflect across the x-axis, and shift down 50 units.

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
51
Determine whether the function is even, odd, or neither.
A) Odd
B) Neither
C) Even
A) Odd
B) Neither
C) Even

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
52
Determine if the complete graph represents a function that is even, odd, or neither.
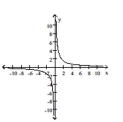
A) odd
B) neither
C) even

A) odd
B) neither
C) even

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
53
y=
A) x -axis, y -axis, origin
B) Origin
C) y -axis
D) No symmetry
A) x -axis, y -axis, origin
B) Origin
C) y -axis
D) No symmetry

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
54
Determine whether the function is even, odd, or neither.
A) Odd
B) Neither
C) Even
A) Odd
B) Neither
C) Even

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
55
Determine if the complete graph represents a function that is even, odd, or neither.
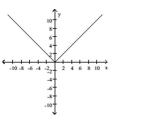
A) even
B) neither
C) odd

A) even
B) neither
C) odd

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
56
Determine whether the function is even, odd, or neither.
A) Even
B) Neither
C) Odd
A) Even
B) Neither
C) Odd

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
57
y= x+
A) Origin
B) y-axis, origin
C) y -axis
D) No symmetry
A) Origin
B) y-axis, origin
C) y -axis
D) No symmetry

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
58
The year y when sales were s million dollars for a particular electronics company can be modeled by the radical equation , where represents 2010 , and so on. Describe the transformations needed to obtain the graph of this function from the graph of .
A) Shift 1.2 units to the right, stretch vertically by a factor of 4, and shift down by 6 units.
B) Shift 4 units to the left, stretch vertically by a factor of 1.2, and shift down by 6 units.
C) Shift 4 units down, stretch vertically by a factor of 1.2, and shift 6 units to the right.
D) Shift 4 units to the right, stretch vertically by a factor of 1.2, and shift down by 6 units.
A) Shift 1.2 units to the right, stretch vertically by a factor of 4, and shift down by 6 units.
B) Shift 4 units to the left, stretch vertically by a factor of 1.2, and shift down by 6 units.
C) Shift 4 units down, stretch vertically by a factor of 1.2, and shift 6 units to the right.
D) Shift 4 units to the right, stretch vertically by a factor of 1.2, and shift down by 6 units.

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
59
Determine if the complete graph represents a function that is even, odd, or neither.
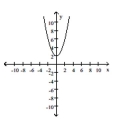
A) odd
B) even
C) neither

A) odd
B) even
C) neither

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
60
Determine whether the function is even, odd, or neither.
A) Even
B) Neither
C) Odd
A) Even
B) Neither
C) Odd

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
61
For the pair of functions, perform the indicated operation.
Find
A)
B)
C)
D)
Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
62
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
63
The linear equation y = 82.67x = 1181 provides an approximation of the va;ue (in dollars) of an account opend on january 1, 2010, in the amount of $1181 and earning 7% simple interest, where x = 0 represents january 1, 2010, x=1 represents january 1, 2011, x=2 represents january 1, 2012. and so on. Write an equation that yields the same y-values when the exact year number is entered.
A) y= 82.67(x - 2011) + 1181
B) y= 82.67(2011 - x) + 1181
C) y= 82.67(2010 - x) + 1181
D) y= 82.67(x- 2010) + 1181
A) y= 82.67(x - 2011) + 1181
B) y= 82.67(2011 - x) + 1181
C) y= 82.67(2010 - x) + 1181
D) y= 82.67(x- 2010) + 1181

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
64
The linear equation y=443 x+3420 provides an approximation of the annual cost (in dollars) of health insurance for a family of three, where x=1 represents 2011, x=2 represents 2012, and so on. Write an equation that yields the same y -values when the exact year number is entered.
A) y=443(2010-x)+3420
B) y=443(x-2011)+3420
C) y=443(x-2010)+3420
D) y=443(2011-x)+3420
A) y=443(2010-x)+3420
B) y=443(x-2011)+3420
C) y=443(x-2010)+3420
D) y=443(2011-x)+3420

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
65
The linear equation y=229 x+6320 provides an approximation of the annual cost (in dollars) to rent an apartment at the Leisure Village Retirement Community, where x=1 represents 2011, x=2 represents 2012, and so on. Write an equation that yields the same y-values when the exact year number is entered.
A) y=229(x-2010)+6320
B) y=229(2010-x)+6320
C) y=229(2011-x)+6320
D) y=229(x-2011)+6320
A) y=229(x-2010)+6320
B) y=229(2010-x)+6320
C) y=229(2011-x)+6320
D) y=229(x-2011)+6320

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
66
Find the specified domain and express it in interval notation.
For and , what is the domain of ?
A)
B) All real numbers
C) All real numbers except
D)
For and , what is the domain of ?
A)
B) All real numbers
C) All real numbers except
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
67
Find (f+g)(x) .
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
68
For the pair of functions, perform the indicated operation.
Find
A)
B)
C)
D)
Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
69
A)
B)
C)
D) -12 x+14

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
70
The price per unit of a product is and the number of units of the product is denoted by q. The demand function for this commodity is given by . Describe the transformations needed to obtain the graph of this function from the graph of .
A) Shift 60 units to the left and stretch vertically by a factor of 4000.
B) Shift up 60 units and stretch vertically by a factor of 4000.
C) Shift 60 units to the right and stretch vertically by a factor of 4000.
D) Shift 60 units to the left and shift up 4000 units.
A) Shift 60 units to the left and stretch vertically by a factor of 4000.
B) Shift up 60 units and stretch vertically by a factor of 4000.
C) Shift 60 units to the right and stretch vertically by a factor of 4000.
D) Shift 60 units to the left and shift up 4000 units.

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
71
The daily cost (in dollars) of removing pollution from the smokestack of an electric power plant is related to the percent of pollution removed according to the equation . Describe the transformations needed to obtain the graph of this function from the graph of .
A) Shift 100 units to the left, reflect across either axis,and stretch vertically by a factor of 16,400.
B) Reflect across the p-axis, shift 100 units to the left and shift up 16,400 units.
C) Shift 100 units to the right, reflect across either axis,and stretch vertically by a factor of 16,400.
D) Shift 100 units to the right and stretch vertically by a factor of 16,400.
A) Shift 100 units to the left, reflect across either axis,and stretch vertically by a factor of 16,400.
B) Reflect across the p-axis, shift 100 units to the left and shift up 16,400 units.
C) Shift 100 units to the right, reflect across either axis,and stretch vertically by a factor of 16,400.
D) Shift 100 units to the right and stretch vertically by a factor of 16,400.

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
72
f(x) = Find (f - g)(x) .
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
73
A study in a small town showed that the percent of residents who have college degrees can be modeled by the function of years from 2015 . , where x is the number of years from 2010 . Rewrite the model with x equal to the number
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
74
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
75
The number of people present at a stadium holding a big rock concert can be estimated with the following function: where y is the number of people present and x is the amount of time since 3:00 p.m. on the day of the concert. Rewrite the model with x equal to the amount of time since 1.00 p.m.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
76
For the pair of functions, perform the indicated operation.
Find
A)
B)
C)
D)
Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
77
For the pair of functions, perform the indicated operation.
Find
A)
B)
C)
D)
Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
78
For the pair of functions, perform the indicated operation.
Find
A)
B)
C)
D)
Find
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
79
Find the specified domain and express it in interval notation.
For and , what is the domain of ?
A)
B) All real numbers
C)
D)
For and , what is the domain of ?
A)
B) All real numbers
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck
80
Find the specified domain and express it in interval notation.
For and , what is the domain of ?
A)
В)
C)
D)
For and , what is the domain of ?
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 299 flashcards in this deck.
Unlock Deck
k this deck



