Deck 1: Functions, Graphs, and Models; Linear Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/306
Play
Full screen (f)
Deck 1: Functions, Graphs, and Models; Linear Functions
1
Evaluate the function.
A) 44
B) 26
C) 1
D) 41
A) 44
B) 26
C) 1
D) 41
D
2
Determine whether or not the relationship shown in the table is a function.
Does the table define x as a function of y?
A) Yes
B) No
Does the table define x as a function of y?
A) Yes
B) No
B
3
Determine whether or not the relationship shown in the table is a function.
Does the table define the price of a home that a particular family can afford as a function of the percent down payment?
A) Yes
B) No
Does the table define the price of a home that a particular family can afford as a function of the percent down payment?
A) Yes
B) No
A
4
Determine whether or not the relationship shown in the table is a function.
Does the table define y as a function of x?
A) Yes
B) No
Does the table define y as a function of x?
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
5
Determine whether or not the relationship shown in the table is a function.
Does the table define the number of classes missed as a function of the average final exam score?
A) Yes
B) No
Does the table define the number of classes missed as a function of the average final exam score?
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
6
Determine whether or not the relationship shown in the table is a function.
Does the table define y as a function of x?
A) Yes
B) No
Does the table define y as a function of x?
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
7
Determine whether or not the relationship shown in the table is a function.
Does the table define weight as a function of the day in January?
A) Yes
B) No
Does the table define weight as a function of the day in January?
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
8
Use the table to answer the question.
Is -3 an input or output of this function?
A) Output
B) Input
Is -3 an input or output of this function?
A) Output
B) Input

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
9
Use the table to answer the question.
Is 31 an input or output of this function?
A) Input
B) Output
Is 31 an input or output of this function?
A) Input
B) Output

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
10

For the function described by the table, find .
A) 7
B) 0
C) 2
D) Not shown

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
11
Given , find
A)
B)
C) 15
D) 13
A)
B)
C) 15
D) 13

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
12
Determine whether or not the relationship shown in the table is a function.
Does the table define test score as a function of name?
A) Yes
B) No
Does the table define test score as a function of name?
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
13
Use the table to answer the question.
Is g(6) an input or output of this function?
A) Input
B) Output
Is g(6) an input or output of this function?
A) Input
B) Output

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
14
Determine whether or not the relationship shown in the table is a function.
Does the table define the day in January as a function of weight?
A) Yes
B) No
Does the table define the day in January as a function of weight?
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
15
Use the table to answer the question.
Is f(16) an input or output of this function?
A) Input
B) Output
Is f(16) an input or output of this function?
A) Input
B) Output

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
16
Determine whether or not the relationship shown in the table is a function.
Does the table define x as a function of y?
A) Yes
B) No
Does the table define x as a function of y?
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
17
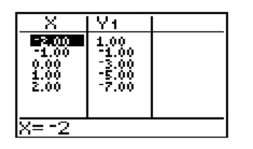
For the function described by the table, find .
A)
B)
C) 0
D) Not shown

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
18
Determine whether or not the relationship shown in the table is a function.
Does the table define the average final exam score as a function of the number of classes missed?
A) Yes
B) No
Does the table define the average final exam score as a function of the number of classes missed?
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
19
Evaluate the function.
Given , find .
A) 19
B) 319
C)
D) 327
Given , find .
A) 19
B) 319
C)
D) 327

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
20
Evaluate the function.
Given , find
A) 1
B)
C) 10
D) 25
Given , find
A) 1
B)
C) 10
D) 25

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
21
State whether the graph is or is not that of a function.
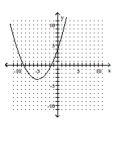
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
22
Decide whether or not the set of ordered pairs defines a function.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
23
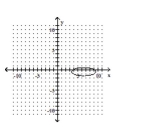
State whether the graph is or is not that of a function.
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
24
Decide whether or not the set of ordered pairs defines a function.
A) No
B) Yes
A) No
B) Yes

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
25
State whether the graph is or is not that of a function.
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
26
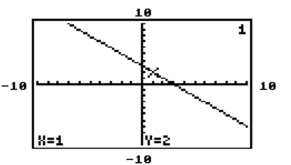
If , find .
A) 1
B) 2
C) 0
D)

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
27
State whether the graph is or is not that of a function.
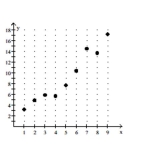
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
28
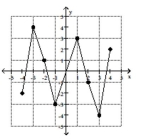
If , find .
A)
B) 1
C)
D) 4

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
29

A) 10
B) 13
C) 7
D) 16

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
30
Decide whether or not the set of ordered pairs defines a function.
A) No
B) Yes
A) No
B) Yes

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
31
Decide whether or not the set of ordered pairs defines a function.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
32
State whether the graph is or is not that of a function.
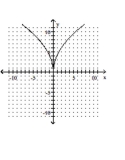
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
33
State whether the graph is or is not that of a function.
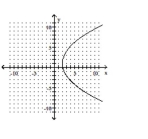
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
34
Decide whether or not the set of ordered pairs defines a function.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
35
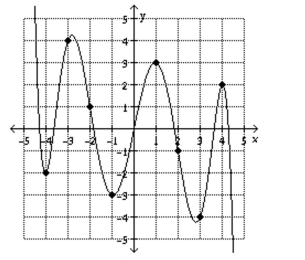
If , find .
A)
B) 2
C)
D) 3

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
36
State whether the graph is or is not that of a function.
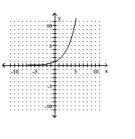
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
37
Decide whether or not the set of ordered pairs defines a function.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
38
State whether the graph is or is not that of a function.
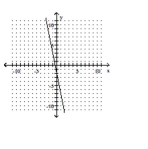
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
39
Decide whether or not the set of ordered pairs defines a function.
A) No
B) Yes
A) No
B) Yes

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
40
Decide whether or not the set of ordered pairs defines a function.
A) No
B) Yes
A) No
B) Yes

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
41
Find the domain of the function.
A)
B) all real numbers except
C)
D) all real numbers except 2
A)
B) all real numbers except
C)
D) all real numbers except 2

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
42
Decide whether or not the arrow diagram defines a function.
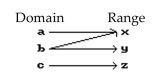
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
43
Decide whether or not the set of ordered pairs defines a function.
A) No
B) Yes
A) No
B) Yes

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
44
Find the domain of the function.
A)
B) all real numbers except 4
C)
D)
A)
B) all real numbers except 4
C)
D)

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
45
Find the domain and range for the function.
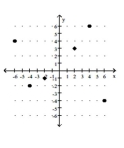
A) D: R:
B) D:
C) D:
D) D:

A) D: R:
B) D:
C) D:
D) D:

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
46
Find the domain of the function.
A)
В)
C)
D)
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
47
Decide whether or not the arrow diagram defines a function.
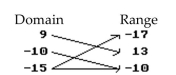
A) No
B) Yes

A) No
B) Yes

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
48
![<strong> </strong> A) D: [ 0,4 ] ; R : [ - 3,4 ] B) D: [ - 3,4 ] ; R: [ 0,4 ] C) D: ( - 3,4 ) ; R: ( 0,4 ) D) D: [ 3,4 ] ; R : [ 0,4 ]](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11eb4038_b104_0990_ad6e_3fabfc323828_TB6590_00.jpg)
![<strong> </strong> A) D: [ 0,4 ] ; R : [ - 3,4 ] B) D: [ - 3,4 ] ; R: [ 0,4 ] C) D: ( - 3,4 ) ; R: ( 0,4 ) D) D: [ 3,4 ] ; R : [ 0,4 ]](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb64c_0b8a_ac9c_8a2d_e90daaea5e3b_TB6590_00.jpg)
A) D:
B) D: R:
C) D: R:
D) D:

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
49
Find the domain of the function.
A)
B)
C) all real numbers except 0
D)
A)
B)
C) all real numbers except 0
D)

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
50
Find the domain of the function.
A)
B)
C) all real numbers except 20
D)
A)
B)
C) all real numbers except 20
D)

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
51
Decide whether or not the set of ordered pairs defines a function.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
52
Find the domain of the function.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
53
Find the domain of the function.
A) All real numbers except 12
B)
C) All real numbers except
D)
A) All real numbers except 12
B)
C) All real numbers except
D)

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
54
Decide whether or not the arrow diagram defines a function.
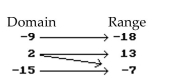
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
55
Decide whether or not the arrow diagram defines a function.
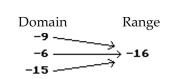
A) No
B) Yes

A) No
B) Yes

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
56
Decide whether or not the arrow diagram defines a function.
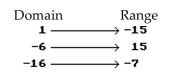
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
57
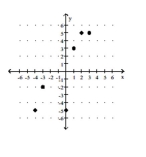
A) D: R:
B) D: R:
C) D: R:
D) D:

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
58
Decide whether or not the arrow diagram defines a function.
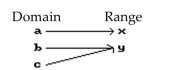
A) No
B) Yes

A) No
B) Yes

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
59
Decide whether or not the arrow diagram defines a function.
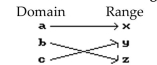
A) Yes
B) No

A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
60
![<strong> </strong> A) D: [ - 3,0 ] ; R: [ - 1,3 ] B) D: [ 0,3 ] ; \mathrm { R } : ( - \infty , 3 ] C) D: ( - \infty , 3 ] ; R: [ 0,3 ] D) D: [ - 1,3 ] ; R: [ - 3,0 ]](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11eb4038_b104_0990_ad6e_3fabfc323828_TB6590_00.jpg)
![<strong> </strong> A) D: [ - 3,0 ] ; R: [ - 1,3 ] B) D: [ 0,3 ] ; \mathrm { R } : ( - \infty , 3 ] C) D: ( - \infty , 3 ] ; R: [ 0,3 ] D) D: [ - 1,3 ] ; R: [ - 3,0 ]](https://d2lvgg3v3hfg70.cloudfront.net/TB6590/11ecb64c_0625_72db_8a2d_175ea6981d4e_TB6590_00.jpg)
A) D: R:
B) D:
C) D: R:
D) D: R:

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
61
y=10+
A)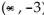
B) all real numbers except 3
C)
D) all real numbers except -3
A)
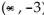
B) all real numbers except 3
C)
D) all real numbers except -3

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
62
The salary s of an employee in a given year y.
A) No
B) Yes; y, s; there is one salary s in any given year y.
A) No
B) Yes; y, s; there is one salary s in any given year y.

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
63
Decide whether or not the equation defines y as a function of x.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
64
Suppose the cost of producing x objects was defined by the function What is the domain of the function defined by this equation?
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
65
Decide whether or not the equation defines y as a function of x.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
66
Decide whether or not the equation defines y as a function of x.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
67
Determine whether the given relationship defines a function. If so, identify the independent and dependent variable, and
why the relationship is a function.
The balance b in a checking account on a given day x.
A) Yes; x, b; there is one balance b on a given day x.
B) No
why the relationship is a function.
The balance b in a checking account on a given day x.
A) Yes; x, b; there is one balance b on a given day x.
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
68
Determine whether the given relationship defines a function. If so, identify the independent and dependent variable, and
why the relationship is a function.
Derek's weight w in second grade g.
A) No
B) Yes; g, w; there is one weight for second grade.
why the relationship is a function.
Derek's weight w in second grade g.
A) No
B) Yes; g, w; there is one weight for second grade.

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
69
A customer's savings account number n given the number of years y the account has been active.
A) No
B) Yes; y, n; there is one account number n in any given year y.
A) No
B) Yes; y, n; there is one account number n in any given year y.

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
70
Decide whether or not the equation defines y as a function of x.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
71
Decide whether or not the equation defines y as a function of x.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
72
Determine whether the given relationship defines a function. If so, identify the independent and dependent variable, and
why the relationship is a function.
The temperature t on a backyard thermometer at 5 pm on a given day x.
A) Yes; x, t; there is one temperature t on any given day x at 5 pm.
B) No
why the relationship is a function.
The temperature t on a backyard thermometer at 5 pm on a given day x.
A) Yes; x, t; there is one temperature t on any given day x at 5 pm.
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
73
Decide whether or not the equation defines y as a function of x.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
74
Determine whether the given relationship defines a function. If so, identify the independent and dependent variable, and
why the relationship is a function.
Addy's height h on the first day d of school throughout elementary school.
A) No
B) Yes; d, h; there is one height for each school year.
why the relationship is a function.
Addy's height h on the first day d of school throughout elementary school.
A) No
B) Yes; d, h; there is one height for each school year.

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
75
Determine whether the given relationship defines a function. If so, identify the independent and dependent variable, and
why the relationship is a function.
The temperature t on a backyard thermometer on a given day x.
A) Yes; x, t; there is one temperature t on any given day x.
B) No
why the relationship is a function.
The temperature t on a backyard thermometer on a given day x.
A) Yes; x, t; there is one temperature t on any given day x.
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
76
The number of shares s of a certain stock traded on a given day x.
A) No
B) Yes; x, s; there is one number of shares s traded on any given day x.
A) No
B) Yes; x, s; there is one number of shares s traded on any given day x.

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
77
This chart shows the fees for an 18-hole round of golf for each of the last 5 years at a local municipal golf course. Assume that this chart defines a function with the name of f. State the domain of f.
A) {(2008, 22), (2009, 24), (2010, 26), (2011, 26), (2012, 30)}
B) {2008, 2009, 2010, 2011, 2012}
C) {22, 24, 26, 30}
D) {(22, 2008), (24, 2009), (26, 2010), (26, 2011), (30, 2012)}
A) {(2008, 22), (2009, 24), (2010, 26), (2011, 26), (2012, 30)}
B) {2008, 2009, 2010, 2011, 2012}
C) {22, 24, 26, 30}
D) {(22, 2008), (24, 2009), (26, 2010), (26, 2011), (30, 2012)}

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
78
Determine whether the given relationship defines a function. If so, identify the independent and dependent variable, and
why the relationship is a function.
The salary s of an employee on her hiring date d.
A) No
B) Yes; d, s; there is one salary s on the hiring date d.
why the relationship is a function.
The salary s of an employee on her hiring date d.
A) No
B) Yes; d, s; there is one salary s on the hiring date d.

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
79
Determine whether the given relationship defines a function. If so, identify the independent and dependent variable, and
why the relationship is a function.
The balance in a checking account b at the close of business on a given day x.
A) Yes; x, b; there is one balance b on any given day x at the close of the business day.
B) No
why the relationship is a function.
The balance in a checking account b at the close of business on a given day x.
A) Yes; x, b; there is one balance b on any given day x at the close of the business day.
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck
80
Decide whether or not the equation defines y as a function of x.
A) Yes
B) No
A) Yes
B) No

Unlock Deck
Unlock for access to all 306 flashcards in this deck.
Unlock Deck
k this deck



