Deck 5: Number Theory and the Real Number System
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/197
Play
Full screen (f)
Deck 5: Number Theory and the Real Number System
1
Determine whether or not the first number is divisible by the second number
125,992; 9
A) No
B) Yes
125,992; 9
A) No
B) Yes
A
2
Find the prime factorization of the number
468
468

A
3
Determine whether or not the first number is divisible by the second number
22,669; 6
A) No
B) Yes
22,669; 6
A) No
B) Yes
A
4
Determine whether or not the first number is divisible by the second number
42,020; 4
A) Yes
B) No
42,020; 4
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
5
Determine whether or not the first number is divisible by the second number
47,490; 10
A) Yes
B) No
47,490; 10
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
6
Find the prime factorization of the number
45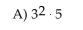
A)5
B)9 ∙ 3
C)52
D) 9 ∙ 5
45
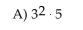
A)5
B)9 ∙ 3
C)52
D) 9 ∙ 5

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
7
Determine whether or not the first number is divisible by the second number
27,140; 8
A) Yes
B) No
27,140; 8
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
8
Determine whether or not the first number is divisible by the second number
1978; 2
A) Yes
B) No
1978; 2
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
9
Find the prime factorization of the number
90
90


Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
10
Determine whether or not the first number is divisible by the second number
12,803; 3
A) Yes
B) No
12,803; 3
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
11
Find the prime factorization of the number
7425
7425


Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
12
Determine whether or not the first number is divisible by the second number
521,364; 3
A) Yes
B) No
521,364; 3
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
13
Find the prime factorization of the number
231
231


Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
14
Determine whether or not the first number is divisible by the second number
398,763; 9
A) No
B) Yes
398,763; 9
A) No
B) Yes

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
15
Determine whether or not the first number is divisible by the second number
394,091; 4
A) Yes
B) No
394,091; 4
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
16
Determine whether or not the first number is divisible by the second number
652,523; 5
A) Yes
B) No
652,523; 5
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
17
Determine whether or not the first number is divisible by the second number
955,575; 5
A) Yes
B) No
955,575; 5
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
18
Determine whether or not the first number is divisible by the second number
22,308; 6
A) No
B) Yes
22,308; 6
A) No
B) Yes

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
19
Determine whether or not the first number is divisible by the second number
22,259; 2
A) No
B) Yes
22,259; 2
A) No
B) Yes

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
20
Determine whether or not the first number is divisible by the second number
15,416; 8
A) Yes
B) No
15,416; 8
A) Yes
B) No

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
21
Find the least common multiple (LCM).
At Lincoln High School, there are 1472 students in the Junior Class and 2576 students in the Senior
Class. The teachers want to assign the students to committees with the same number of juniors in
Each committee and the same number of seniors in each committee. (For example, there might be 2
Juniors and 3 seniors in every committee). What are all the possible numbers of committees they
Could form and still meet this goal?
A) 4, 16, 92, 368, or 644 different committees
B) 4, 23, 64, or 368 different committees
C) 4, 23, 16, 92, or 368 different committees
D) 4, 23, 16, or 64 different committees
At Lincoln High School, there are 1472 students in the Junior Class and 2576 students in the Senior
Class. The teachers want to assign the students to committees with the same number of juniors in
Each committee and the same number of seniors in each committee. (For example, there might be 2
Juniors and 3 seniors in every committee). What are all the possible numbers of committees they
Could form and still meet this goal?
A) 4, 16, 92, 368, or 644 different committees
B) 4, 23, 64, or 368 different committees
C) 4, 23, 16, 92, or 368 different committees
D) 4, 23, 16, or 64 different committees

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
22
Find the prime factorization of the number
62
62


Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
23
Find the least common multiple (LCM).
120 and 90
A) 30
B) 720
C) 1080
D) 360
120 and 90
A) 30
B) 720
C) 1080
D) 360

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
24
Find the least common multiple (LCM).
Janine and Thor are both running for class president. Janine goes down a hallway in the school
And puts a sticker on every fourth locker. Thor goes down the same hallway, putting one of his
Stickers on every fifth locker. If there are 130 lockers in the hallway, how many have both studentsʹ
Stickers?
A) 20
B) 13
C) 6
D) 26
Janine and Thor are both running for class president. Janine goes down a hallway in the school
And puts a sticker on every fourth locker. Thor goes down the same hallway, putting one of his
Stickers on every fifth locker. If there are 130 lockers in the hallway, how many have both studentsʹ
Stickers?
A) 20
B) 13
C) 6
D) 26

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
25
Find the least common multiple (LCM).
Several different bus routes stop at the corner of Second St. and Lincoln Ave. A Wilkenson bus
Arrives every 18 minutes, a Fifth Avenue bus arrives every 45 minutes and a Harris Road bus
Arrives every 10 minutes. If both buses arrive at the stop at 5:07 AM, how many minutes will pass
Before both buses arrive at the same time again?
A) 10 minutes
B) 90 minutes
C) 45 minutes
D) 8100 minutes
Several different bus routes stop at the corner of Second St. and Lincoln Ave. A Wilkenson bus
Arrives every 18 minutes, a Fifth Avenue bus arrives every 45 minutes and a Harris Road bus
Arrives every 10 minutes. If both buses arrive at the stop at 5:07 AM, how many minutes will pass
Before both buses arrive at the same time again?
A) 10 minutes
B) 90 minutes
C) 45 minutes
D) 8100 minutes

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
26
Find the greatest common divisor (GCD).
14, 18, and 20
A) 1
B) 14
C) 4
D) 2
14, 18, and 20
A) 1
B) 14
C) 4
D) 2

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
27
Find the prime factorization of the number
32,625
32,625


Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
28
Find the greatest common divisor (GCD).
42, 56, and 98
A) 7
B) 1
C) 14
D) 42
42, 56, and 98
A) 7
B) 1
C) 14
D) 42

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
29
Find the least common multiple (LCM).
Several different bus routes stop at the corner of Second St. and Lincoln Ave. A Wilkenson bus
Arrives every 27 minutes and a Harris Road bus arrives every 15 minutes. If both buses arrive at
The stop at 5:07 AM, when will they again arrive at the same time?
A) 6:42 AM
B) 7:22 AM
C) 11:52 AM
D) 9:12 AM
Several different bus routes stop at the corner of Second St. and Lincoln Ave. A Wilkenson bus
Arrives every 27 minutes and a Harris Road bus arrives every 15 minutes. If both buses arrive at
The stop at 5:07 AM, when will they again arrive at the same time?
A) 6:42 AM
B) 7:22 AM
C) 11:52 AM
D) 9:12 AM

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
30
Find the least common multiple (LCM).
48, 162, and 9
A) 1296
B) 432
C) 324
D) 648
48, 162, and 9
A) 1296
B) 432
C) 324
D) 648

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
31
Find the least common multiple (LCM).
56 and 96
A) 1344
B) 672
C) 168
D) 224
56 and 96
A) 1344
B) 672
C) 168
D) 224

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
32
Find the least common multiple (LCM).
45, 28, and 150
A) 1,050
B) 630
C) 1260
D) 6300
45, 28, and 150
A) 1,050
B) 630
C) 1260
D) 6300

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
33
Find the greatest common divisor (GCD).
120 and 270
A) 30
B) 6
C) 10
D) 15
120 and 270
A) 30
B) 6
C) 10
D) 15

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
34
Find the least common multiple (LCM).
At Northwest High School, there are 1472 students in the Junior Class and 1012 students in the
Senior Class. To let the juniors work with more experienced students, the teachers want to assign
The students to committees with the same number of juniors in each committee and the same
Number of seniors in each committee. (For example, there might be 2 juniors and 3 seniors in every
Committee). What is the largest number of committees that can be formed?
A) 23 committees
B) 368 committees
C) 4 committees
D) 92 committees
At Northwest High School, there are 1472 students in the Junior Class and 1012 students in the
Senior Class. To let the juniors work with more experienced students, the teachers want to assign
The students to committees with the same number of juniors in each committee and the same
Number of seniors in each committee. (For example, there might be 2 juniors and 3 seniors in every
Committee). What is the largest number of committees that can be formed?
A) 23 committees
B) 368 committees
C) 4 committees
D) 92 committees

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
35
Find the least common multiple (LCM).
At Northwest High School, there are 207 students in the Sophomore Class, 243 students in the
Junior Class, and 216 students in the Senior Class. The teachers want to assign the students to
Committees with the same number of sophomores, juniors, and seniors in each committee. (For
Example, there might be 4 sophomores, 2 juniors, and 3 seniors in every committee). What is the
Largest number of committees that can be formed?
A) 72
B) 3
C) 27
D) 9
At Northwest High School, there are 207 students in the Sophomore Class, 243 students in the
Junior Class, and 216 students in the Senior Class. The teachers want to assign the students to
Committees with the same number of sophomores, juniors, and seniors in each committee. (For
Example, there might be 4 sophomores, 2 juniors, and 3 seniors in every committee). What is the
Largest number of committees that can be formed?
A) 72
B) 3
C) 27
D) 9

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
36
Find the greatest common divisor (GCD).
8 and 12
A) 4
B) 2
C) 1
D) 8
8 and 12
A) 4
B) 2
C) 1
D) 8

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
37
Find the greatest common divisor (GCD).
30 and 19
A) 19
B) 3
C) 10
D) 1
30 and 19
A) 19
B) 3
C) 10
D) 1

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
38
Find the least common multiple (LCM).
60, 80, and 50
A) 240
B) 600
C) 400
D) 1200
60, 80, and 50
A) 240
B) 600
C) 400
D) 1200

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
39
Find the greatest common divisor (GCD).
30 and 36
A) 6
B) 1
C) 3
D) 2
30 and 36
A) 6
B) 1
C) 3
D) 2

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
40
Find the prime factorization of the number
1162
1162


Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
41
Evaluate the expression.
-17 + 14 + (-1)
A) -2
B)32
C)-4
D)30
-17 + 14 + (-1)
A) -2
B)32
C)-4
D)30

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
42
Provide an appropriate response.
True or False? 3 is a factor of 6.
True or False? 3 is a factor of 6.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
43
Provide an appropriate response.
True or False? If a number is divisible by 2, then every digit of the number is divisible by 2.
True or False? If a number is divisible by 2, then every digit of the number is divisible by 2.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
44
Provide an appropriate response.
True or False? If a number is not divisible by 10, then it is not divisible by 20.
True or False? If a number is not divisible by 10, then it is not divisible by 20.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
45
Provide an appropriate response.
True or False? 2|6
True or False? 2|6

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
46
Evaluate the expression.
-23 + 9
A) 14
B) -32
C) 32
D) -14
-23 + 9
A) 14
B) -32
C) 32
D) -14

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
47
Provide an appropriate response.
Choose the number that is divisible by 2, 3, 7, 6, and 14.
A) 114
B) 142
C) 42
D) 21
Choose the number that is divisible by 2, 3, 7, 6, and 14.
A) 114
B) 142
C) 42
D) 21

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
48
Provide an appropriate response.
True or False? 10|2
True or False? 10|2

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
49
Evaluate the expression.
1 + (-25) + (-15)
A) -39
B) 11
C) 41
D) -9
1 + (-25) + (-15)
A) -39
B) 11
C) 41
D) -9

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
50
Evaluate the expression.
-60 + 61
A) -1B)-121
C) 121
D) 1
-60 + 61
A) -1B)-121
C) 121
D) 1

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
51
Provide an appropriate response.
True or False? 15 is divisible by 3.
True or False? 15 is divisible by 3.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
52
Evaluate the expression.
-10 + (-21)
A) 11
B) -31
C) 31
D) -11
-10 + (-21)
A) 11
B) -31
C) 31
D) -11

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
53
Evaluate the expression.
-17 + (-50) + 70 + (-60)
A) -23
B) 63
C) -197
D) -57
-17 + (-50) + 70 + (-60)
A) -23
B) 63
C) -197
D) -57

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
54
Evaluate the expression.
9 + (-13)
A) -22
B) 22
C) -4D)4
9 + (-13)
A) -22
B) 22
C) -4D)4

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
55
Evaluate the expression.
11 + 23 + (-12)
A) -24
B) 46
C) 0
D) 22
11 + 23 + (-12)
A) -24
B) 46
C) 0
D) 22

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
56
Provide an appropriate response.
True or False? If a number is divisible by 3, then the number must also be divisible by 6.
True or False? If a number is divisible by 3, then the number must also be divisible by 6.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
57
Provide an appropriate response.
True or False? 6 is a multiple of 3.
True or False? 6 is a multiple of 3.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
58
Provide an appropriate response.
True or False? 3 is a multiple of 15.
True or False? 3 is a multiple of 15.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
59
Provide an appropriate response.
True or False? If a number is divisible by 7 and 3, then the number is divisible by 21.
True or False? If a number is divisible by 7 and 3, then the number is divisible by 21.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
60
Provide an appropriate response.
True or False? 3 is divisible by 6.
True or False? 3 is divisible by 6.

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
61
Evaluate the expression.
-7 - (-3)
A) 10
B) 4
C) -4D)-10
-7 - (-3)
A) 10
B) 4
C) -4D)-10

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
62
Evaluate the expression.



Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
63
Evaluate the expression.
15 - (-10)
A) 5
B) -5C)-25
D) 25
15 - (-10)
A) 5
B) -5C)-25
D) 25

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
64
Evaluate the expression.
[4 + (-4)] + (-5 + 8)
A) 3
B) -13
C) 21
D) 13
[4 + (-4)] + (-5 + 8)
A) 3
B) -13
C) 21
D) 13

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
65
Evaluate the expression.
(-3)(9)(-5)
A) -17
B) -135
C) 135
D) 17
(-3)(9)(-5)
A) -17
B) -135
C) 135
D) 17

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
66
Evaluate the expression.
(-16)(-9)
A) -144
B) -7
C) 144
D) 7
(-16)(-9)
A) -144
B) -7
C) 144
D) 7

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
67
Evaluate the expression.
(-4)(-4)(-8)
A) -128
B) -28
C) 128
D) -138
(-4)(-4)(-8)
A) -128
B) -28
C) 128
D) -138

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
68
Evaluate the expression.
-5 ∙ 3
A) -2B) 2C)15
D) -15
-5 ∙ 3
A) -2B) 2C)15
D) -15

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
69
Evaluate the expression.
(-16 + 17) + (-3 + 10)
A) 8
B) 14
C) -26
D) 40
(-16 + 17) + (-3 + 10)
A) 8
B) 14
C) -26
D) 40

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
70
Evaluate the expression.
(-9)(-9)(-9)
A) -739
B) -729
C) 729
D) -719
(-9)(-9)(-9)
A) -739
B) -729
C) 729
D) -719

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
71
Evaluate the expression.
(-3)(-6)
A) -9B)-18
C) 9D) 18
(-3)(-6)
A) -9B)-18
C) 9D) 18

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
72
Evaluate the expression.
(-5)(-3)(6)
A) 80
B) 190
C) 90
D) -90
(-5)(-3)(6)
A) 80
B) 190
C) 90
D) -90

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
73
Evaluate the expression.
9 - 14
A) -5
B) 5
C) 23
D) -23
9 - 14
A) -5
B) 5
C) 23
D) -23

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
74
Evaluate the expression.
(-3)(-3)
A) -19
B) 19
C) -9D)9
(-3)(-3)
A) -19
B) 19
C) -9D)9

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
75
Evaluate the expression.
[(-3)(-3)] ∙ [7(-5)]
A) 315
B) 45
C) -45
D) -315
[(-3)(-3)] ∙ [7(-5)]
A) 315
B) 45
C) -45
D) -315

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
76
Evaluate the expression.
(-6)(-8)(-8)
A) 384
B) -374
C) 96
D) -384
(-6)(-8)(-8)
A) 384
B) -374
C) 96
D) -384

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
77
Evaluate the expression.
(15 + 11) - (13 - 15)
A) 28
B) 32
C) 2
D) -32
(15 + 11) - (13 - 15)
A) 28
B) 32
C) 2
D) -32

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
78
Evaluate the expression.
(-6 - 1) - (-14 + 11)
A) -32
B) -2C)10D)-4
(-6 - 1) - (-14 + 11)
A) -32
B) -2C)10D)-4

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
79
Evaluate the expression.
11 - (12 - 20)
A) 19
B) 3
C) -21
D) 43
11 - (12 - 20)
A) 19
B) 3
C) -21
D) 43

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck
80
Evaluate the expression.
-5 - 10
A) 5
B) -15
C) -5D)15
-5 - 10
A) 5
B) -15
C) -5D)15

Unlock Deck
Unlock for access to all 197 flashcards in this deck.
Unlock Deck
k this deck



