Deck 6: Matrices and Determinants and Applications
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/154
Play
Full screen (f)
Deck 6: Matrices and Determinants and Applications
1
Choose the one alternative that best completes the statement or answers the question.
Write the augmented matrix for the given system.
\[\begin{array} { r }
x + 3 y + 5 z = - 3 \\
- 5 y + 3 z = - 8 \\
- 8 z = - 6
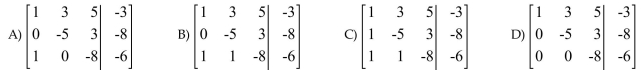
Write the augmented matrix for the given system.
\[\begin{array} { r }
x + 3 y + 5 z = - 3 \\
- 5 y + 3 z = - 8 \\
- 8 z = - 6
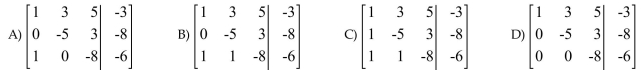
D
2
Explain the meaning of the notation
Add 3 times row 1 to row 2 and replace the original row 2 with the result.
3
Explain the meaning of the notation
Add 4 times row 2 to row 3 and replace the original row 3 with the result.
4
A rectangular array of elements is called a .

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
5
Choose the one alternative that best completes the statement or answers the question.
Write the augmented matrix for the given system.
A)
B)
C)
D)
Write the augmented matrix for the given system.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
6
Write a system of linear equations represented by the augmented matrix.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
7
Choose the one alternative that best completes the statement or answers the question.
Write the augmented matrix for the given system.
A)
B)
C)
D)
Write the augmented matrix for the given system.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
8
Perform the elementary row operation on the given matrix.
![Perform the elementary row operation on the given matrix. 3 R _ { 2 } + R _ { 1 } \rightarrow R _ { 1 } \left[ \begin{array} { r r | r } 4 & 5 & 1 \\ - 2 & - 7 & - 1 \end{array} \right]](https://d2lvgg3v3hfg70.cloudfront.net/TB7600/11ecb0e8_7a04_5c11_8acf_837fa470f47c_TB7600_00.jpg)
![Perform the elementary row operation on the given matrix. 3 R _ { 2 } + R _ { 1 } \rightarrow R _ { 1 } \left[ \begin{array} { r r | r } 4 & 5 & 1 \\ - 2 & - 7 & - 1 \end{array} \right]](https://d2lvgg3v3hfg70.cloudfront.net/TB7600/11ecb0e8_7a04_5c11_8acf_837fa470f47c_TB7600_00.jpg)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
9
Identify the elements on the main diagonal.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
10
Explain the meaning of the notation

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
11
Explain the meaning of the notation

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
12
An matrix is used to represent a system of linear equations written in standard form.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
13
Write a system of linear equations represented by the augmented matrix.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
14
Explain the meaning of the notation

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
15
Explain the meaning of

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
16
Perform the elementary row operation on the given matrix.
![Perform the elementary row operation on the given matrix. - \frac { 1 } { 2 } R _ { 2 } \rightarrow R _ { 2 } \left[ \begin{array} { r r | r } 9 & 11 & 1 \\ 16 & 32 & 20 \end{array} \right]](https://d2lvgg3v3hfg70.cloudfront.net/TB7600/11ecb0e8_69f6_8eb0_8acf_39538e27cbb8_TB7600_00.jpg)
![Perform the elementary row operation on the given matrix. - \frac { 1 } { 2 } R _ { 2 } \rightarrow R _ { 2 } \left[ \begin{array} { r r | r } 9 & 11 & 1 \\ 16 & 32 & 20 \end{array} \right]](https://d2lvgg3v3hfg70.cloudfront.net/TB7600/11ecb0e8_69f6_8eb0_8acf_39538e27cbb8_TB7600_00.jpg)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
17
Choose the one alternative that best completes the statement or answers the question.
Write the augmented matrix for the given system.

Write the augmented matrix for the given system.


Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
18
Write a system of linear equations represented by the augmented matrix.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
19
Write a system of linear equations represented by the augmented matrix.
A)
[x - 5 y = 11\]
B)[5 x + 8 y = 6\]
(- 5 x = 11\)
C)(5 x = 6\)
(8 x - 5 y = 11\)
D)(5 x + 8 y = 6\)
(- 5 y = 11\)
A)
[x - 5 y = 11\]
B)[5 x + 8 y = 6\]
(- 5 x = 11\)
C)(5 x = 6\)
(8 x - 5 y = 11\)
D)(5 x + 8 y = 6\)
(- 5 y = 11\)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
20
row operations performed on an augmented matrix results in a new augmented
matrix that represents an equivalent system of equations.
matrix that represents an equivalent system of equations.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
21
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
22
Perform the elementary row operation on the given matrix.
![Perform the elementary row operation on the given matrix. \begin{array} { l } R _ { 2 } \Leftrightarrow R _ { 3 } \\ { \left[ \begin{array} { r r r | r } - 8 & 2 & - 9 & - 4 \\ - 3 & 7 & - 6 & 6 \\ 5 & 0 & 3 & 6 \end{array} \right] } \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB7600/11ecb0e8_83b9_b612_8acf_c5a40d58e287_TB7600_00.jpg)
![Perform the elementary row operation on the given matrix. \begin{array} { l } R _ { 2 } \Leftrightarrow R _ { 3 } \\ { \left[ \begin{array} { r r r | r } - 8 & 2 & - 9 & - 4 \\ - 3 & 7 & - 6 & 6 \\ 5 & 0 & 3 & 6 \end{array} \right] } \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB7600/11ecb0e8_83b9_b612_8acf_c5a40d58e287_TB7600_00.jpg)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
23
Perform the indicated row operations, then write the new matrix.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
24
Determine if the matrix is in row-echelon form.
A) No
B) Yes
A) No
B) Yes

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
25
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
True or false? A system of linear equations in three variables may have exactly two solutions.
Provide the missing information.
True or false? A system of linear equations in three variables may have exactly two solutions.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
26
Find the partial fraction decomposition for the given rational expression. Use the technique of Gaussian
elimination to find A, B, and C.
A)
B)
C)
D)
elimination to find A, B, and C.
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
27
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
В)
C)
D)
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
28
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
29
Use a calculator to approximate the reduced row-echelon form of the augmented matrix representing the
given system. Give the solution set where x, y, and z are rounded to 2 decimal places.
A)
В)
C)
D)
given system. Give the solution set where x, y, and z are rounded to 2 decimal places.
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
30
Perform the elementary row operation on the given matrix.
A) C)
В) D)
A) C)
В) D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
31
Determine if the matrix is in row-echelon form.
A) No
B) Yes
A) No
B) Yes

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
32
Solve the problem.
Danielle stayed in three different cities (Washington, D.C., Atlanta, Georgia, and Dallas, Texas) for a total of 22 nights. She spent twice as many nights in Dallas as she did in Washington. The total
Cost for 22 nights (excluding tax) was $3,100. Determine the number of nights that she spent in each city.
A) 5 nights in Washington, 7 nights in Atlanta, and 10 nights in Dallas
B) 1 night in Washington, 19 nights in Atlanta, and 2 nights in Dallas
C) 4 nights in Washington, 10 nights in Atlanta, and 8 nights in Dallas
D) 6 nights in Washington, 4 nights in Atlanta, and 12 nights in Dallas
Danielle stayed in three different cities (Washington, D.C., Atlanta, Georgia, and Dallas, Texas) for a total of 22 nights. She spent twice as many nights in Dallas as she did in Washington. The total
Cost for 22 nights (excluding tax) was $3,100. Determine the number of nights that she spent in each city.
A) 5 nights in Washington, 7 nights in Atlanta, and 10 nights in Dallas
B) 1 night in Washington, 19 nights in Atlanta, and 2 nights in Dallas
C) 4 nights in Washington, 10 nights in Atlanta, and 8 nights in Dallas
D) 6 nights in Washington, 4 nights in Atlanta, and 12 nights in Dallas

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
33
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
34
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
True or false? A system of linear equations in three variables may have exactly one solution.
Provide the missing information.
True or false? A system of linear equations in three variables may have exactly one solution.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
35
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
True or false? A system of linear equations in three variables may have no solution.
Provide the missing information.
True or false? A system of linear equations in three variables may have no solution.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
36
Solve the problem.
Andre borrowed $40,000 to buy a truck for his business. He borrowed from his parents who charge him 3% simple interest. He borrowed from a credit union that charges 4% simple interest, and he
Borrowed from a bank that charges 6% simple interest. He borrowed four times as much from his
Parents as from the bank, and the amount of interest he paid at the end of 1 yr was $1,560. How
Much did he borrow from each source?
A) He borrowed $4,000 from his parents, $35,000 from the credit union, and $1,000 from the bank.
B) He borrowed 1,500 from his parents, 32,500 from the credit union, and 6,000 from the bank.
C) He borrowed 6,000 from his parents, 32,500 from the credit union, and 1,500 from the bank.
D) He borrowed $8,000 from his parents, $30,000 from the credit union, and $2,000 from the bank.
Andre borrowed $40,000 to buy a truck for his business. He borrowed from his parents who charge him 3% simple interest. He borrowed from a credit union that charges 4% simple interest, and he
Borrowed from a bank that charges 6% simple interest. He borrowed four times as much from his
Parents as from the bank, and the amount of interest he paid at the end of 1 yr was $1,560. How
Much did he borrow from each source?
A) He borrowed $4,000 from his parents, $35,000 from the credit union, and $1,000 from the bank.
B) He borrowed 1,500 from his parents, 32,500 from the credit union, and 6,000 from the bank.
C) He borrowed 6,000 from his parents, 32,500 from the credit union, and 1,500 from the bank.
D) He borrowed $8,000 from his parents, $30,000 from the credit union, and $2,000 from the bank.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
37
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
В)
C)
D)
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
38
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
В)
C)
D)
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
39
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
40
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
В)
C)
D)
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
41
Choose the one alternative that best completes the statement or answers the question.
For the given augmented matrix, determine the number of solutions to the corresponding system of
equations.
A) No solution
B) One solution
C) Infinitely many solutions
For the given augmented matrix, determine the number of solutions to the corresponding system of
equations.
A) No solution
B) One solution
C) Infinitely many solutions

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
42
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
If a system of linear equations has infinitely many solutions, then the equations are said to be
.
Provide the missing information.
If a system of linear equations has infinitely many solutions, then the equations are said to be
.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
43
The solution set to a system of dependent equations is given. Write the specific solution corresponding to
the given value of z.
is any real number
A)
В)
C)
D)
the given value of z.
is any real number
A)
В)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
44
Determine the solution set for the system represented by the augmented matrix.
A)
В)
C)
D){ }
A)
В)
C)
D){ }

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
45
Write the word or phrase that best completes each statement or answers the question.
Solve the problem.
Assume that traffic flows freely through intersections A, B, and C. The values , and all other
numbers in the figure represent flow rates in vehicles per hour.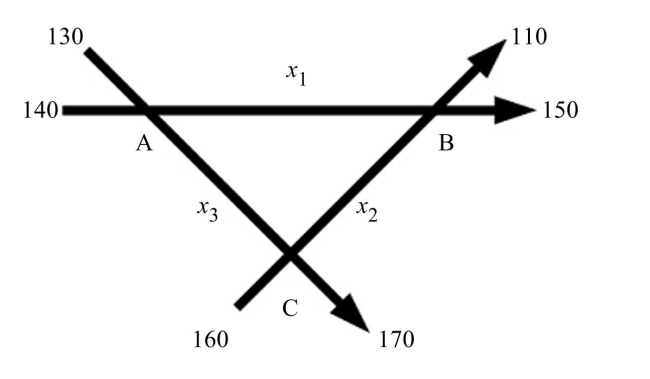 a. Write an equation representing equal flow into and out of intersection A.
a. Write an equation representing equal flow into and out of intersection A.
b. Write an equation representing equal flow into and out of intersection B.
c. Write an equation representing equal flow into and out of intersection C.
d. Write the system of equations from parts (a)-(c) in standard form.
e. Write the reduced row-echelon form of the augmented matrix representing the system of equations from
part (d).
f. If the flow rate between intersections A and C is 120 vehicles per hour, determine the flow rates and g. If the flow rate between intersections A and C is between 150 and 240 vehicles per hour, inclusive,
g. If the flow rate between intersections A and C is between 150 and 240 vehicles per hour, inclusive,
determine the flow rates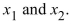
Solve the problem.
Assume that traffic flows freely through intersections A, B, and C. The values , and all other
numbers in the figure represent flow rates in vehicles per hour.
 a. Write an equation representing equal flow into and out of intersection A.
a. Write an equation representing equal flow into and out of intersection A.b. Write an equation representing equal flow into and out of intersection B.
c. Write an equation representing equal flow into and out of intersection C.
d. Write the system of equations from parts (a)-(c) in standard form.
e. Write the reduced row-echelon form of the augmented matrix representing the system of equations from
part (d).
f. If the flow rate between intersections A and C is 120 vehicles per hour, determine the flow rates and
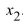 g. If the flow rate between intersections A and C is between 150 and 240 vehicles per hour, inclusive,
g. If the flow rate between intersections A and C is between 150 and 240 vehicles per hour, inclusive,determine the flow rates
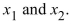

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
46
The solution set to a system of dependent equations is given. Write three ordered triples that are
solutions to the system. Answers may vary.
and are any real numbers
A)
B)
C)
D)
solutions to the system. Answers may vary.
and are any real numbers
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
47
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
If a system of linear equations has no solution, then the system is said to be .
Provide the missing information.
If a system of linear equations has no solution, then the system is said to be .

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
48
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
B) is any real number
C)
D){}
A)
B) is any real number
C)
D){}

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
49
Choose the one alternative that best completes the statement or answers the question.
For the given augmented matrix, determine the number of solutions to the corresponding system of
equations.
A) One solution
B) Infinitely many solutions
C) No solution
For the given augmented matrix, determine the number of solutions to the corresponding system of
equations.
A) One solution
B) Infinitely many solutions
C) No solution

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
50
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
B)
C) is any real number
D) { }
A)
B)
C) is any real number
D) { }

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
51
Solve the system using Gaussian elimination or Gauss-Jordan elimination.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
52
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
В)
C) { }
D)
A)
В)
C) { }
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
53
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
B) is any real number
C){}
D)
A)
B) is any real number
C){}
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
54
Write the word or phrase that best completes each statement or answers the question.
Solve the problem.

Solve the problem.



Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
55
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
True or false? A system of linear equations in three variables may have infinitely many solutions.
Provide the missing information.
True or false? A system of linear equations in three variables may have infinitely many solutions.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
56
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
If the of a matrix is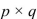 , then p represents the number of and q
, then p represents the number of and q
represents the number of .
Provide the missing information.
If the of a matrix is
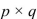 , then p represents the number of and q
, then p represents the number of and qrepresents the number of .

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
57
Write the word or phrase that best completes each statement or answers the question.
Solve the problem.
-An accountant checks the reported earnings for a concert venue for three different performers against the number of tickets sold.
Let , and represent the cost for children tickets, student tickets, and general admission tickets, respectively. Set up an augmented matrix for the system and solve for , and . Explain what the accountant knows about the reported earnings.
A){ } ; The system of equations reduces to a contradiction. There are no values for , and that can simultaneously meet the conditions of this problem.
B) ; There were children's tickets, student tickets, and general admission tickets sold.
C) ; There were children's tickets, student tickets, and general admission tickets sold.
D) ; There were children's tickets, student tickets, and general admission tickets sold.
Solve the problem.
-An accountant checks the reported earnings for a concert venue for three different performers against the number of tickets sold.
Let , and represent the cost for children tickets, student tickets, and general admission tickets, respectively. Set up an augmented matrix for the system and solve for , and . Explain what the accountant knows about the reported earnings.
A){ } ; The system of equations reduces to a contradiction. There are no values for , and that can simultaneously meet the conditions of this problem.
B) ; There were children's tickets, student tickets, and general admission tickets sold.
C) ; There were children's tickets, student tickets, and general admission tickets sold.
D) ; There were children's tickets, student tickets, and general admission tickets sold.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
58
Determine the solution set for the system represented by the augmented matrix.
A) is any real number
B){ }
C)
D) is any real number
A) is any real number
B){ }
C)
D) is any real number

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
59
Solve the system using Gaussian elimination or Gauss-Jordan elimination.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
60
Solve the system using Gaussian elimination or Gauss-Jordan elimination.
A)
B){ }
C)
D)
A)
B){ }
C)
D)

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
61
Give the order of the matrix. Classify the matrix as a square matrix, row matrix, column matrix, or none
of these.

of these.


Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
62
Find A + B.
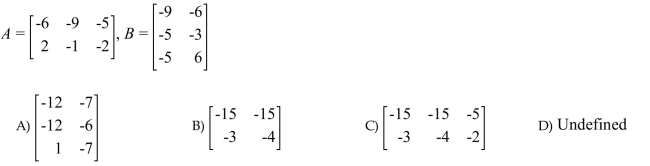
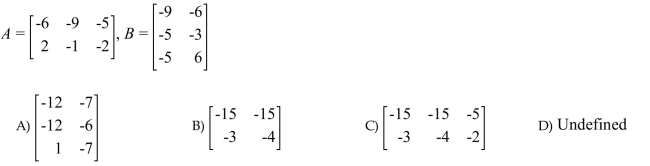

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
63
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
A matrix with the same number of rows and columns is called a matrix.
Provide the missing information.
A matrix with the same number of rows and columns is called a matrix.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
64
For what values of x, y, and z will A = B?



Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
65
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
An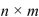 matrix whose elements are all zero is called a matrix.
matrix whose elements are all zero is called a matrix.
Provide the missing information.
An
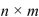 matrix whose elements are all zero is called a matrix.
matrix whose elements are all zero is called a matrix.
Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
66
Determine the value of the given element of the matrix.



Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
67
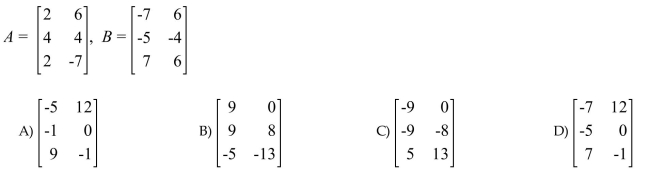
Find B - A.


Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
68
Give the order of the matrix. Classify the matrix as a square matrix, row matrix, column matrix, or none
of these.

of these.


Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
69
Find C - A + B
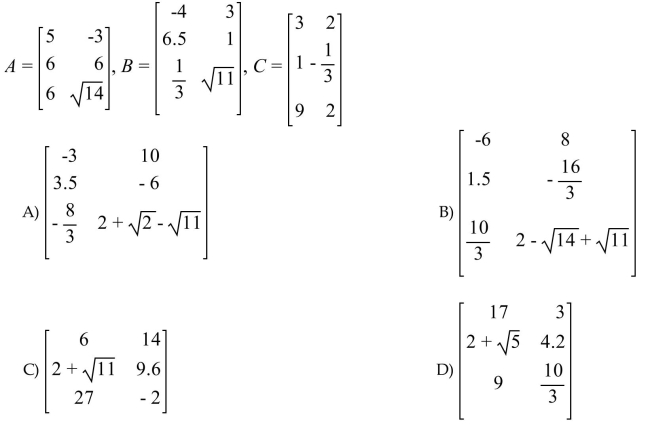
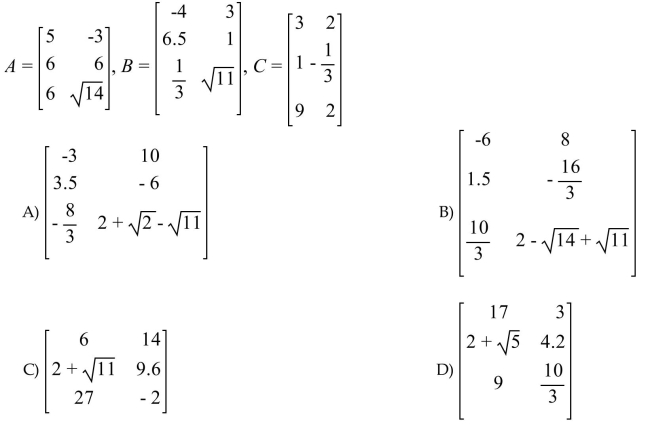

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
70
Choose the one alternative that best completes the statement or answers the question.
Give the order of the matrix.

Give the order of the matrix.


Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
71
Give the order of the matrix. Classify the matrix as a square matrix, row matrix, column matrix, or none
of these.

of these.


Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
72
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
True or false: If a row matrix A and a column matrix B have the same number of elements, then the
product AB is well defined.
Provide the missing information.
True or false: If a row matrix A and a column matrix B have the same number of elements, then the
product AB is well defined.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
73
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
To multiply two matrices A and B, the number of of A must equal the number of
of B.
Provide the missing information.
To multiply two matrices A and B, the number of of A must equal the number of
of B.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
74
Find A + B.
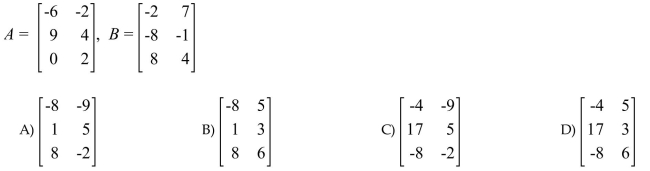


Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
75
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
True or false: Matrix multiplication is a commutative operation.
Provide the missing information.
True or false: Matrix multiplication is a commutative operation.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
76
Classify the matrix as a square matrix, row matrix, column matrix, or none of these.
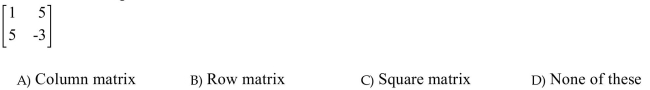
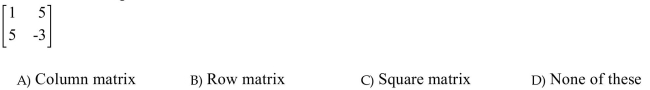

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
77
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
What are the requirements for two matrices to be equal?
Provide the missing information.
What are the requirements for two matrices to be equal?

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
78
Write the word or phrase that best completes each statement or answers the question.
Provide the missing information.
If A is a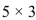 3 matrix and B is a
3 matrix and B is a 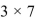 trix, then the product AB will be a matrix of order
trix, then the product AB will be a matrix of order
. The product BA (is/is not) defined.
Provide the missing information.
If A is a
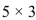 3 matrix and B is a
3 matrix and B is a 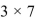 trix, then the product AB will be a matrix of order
trix, then the product AB will be a matrix of order. The product BA (is/is not) defined.

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
79
Give the order of the matrix. Classify the matrix as a square matrix, row matrix, column matrix, or none
of these.

of these.


Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck
80
Find the additive inverse of A.
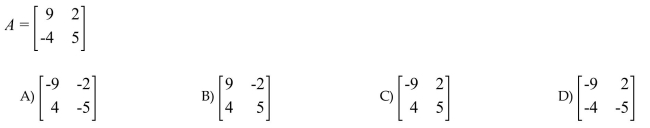
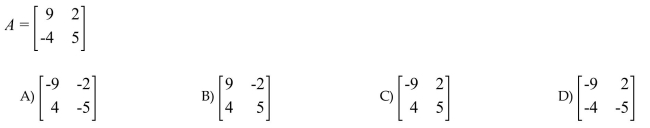

Unlock Deck
Unlock for access to all 154 flashcards in this deck.
Unlock Deck
k this deck



