Deck 12: Statistical Control Charts, Nonparametric Tests, and Hypothesis Testing
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/177
Play
Full screen (f)
Deck 12: Statistical Control Charts, Nonparametric Tests, and Hypothesis Testing
1
Examine the given run chart or control chart and determine whether the process is within_
statistical control. If it is not, identify which of the three out-of-statistical-control criteria apply.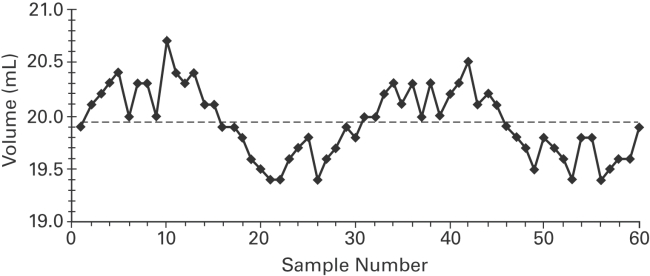
statistical control. If it is not, identify which of the three out-of-statistical-control criteria apply.

The process appears to be out of statistical control. There is a cyclical pattern.
2
Are control charts based on actual behavior or on desired behavior? Give an example to
illustrate the difference between the two types of behavior.
illustrate the difference between the two types of behavior.
Control charts are based on actual behavior. The control limits are based on the actual behavior of the process. They
are not related to the desired specifications indicated by the manufacturer. For example, it is possible to
manufacture rulers that are in statistical control but are consistently 14 inches long, indicating that the desired
behavior is not occurring.
are not related to the desired specifications indicated by the manufacturer. For example, it is possible to
manufacture rulers that are in statistical control but are consistently 14 inches long, indicating that the desired
behavior is not occurring.
3
Construct an R chart and determine whether the process variation is within statistical control.
Control Chart Constants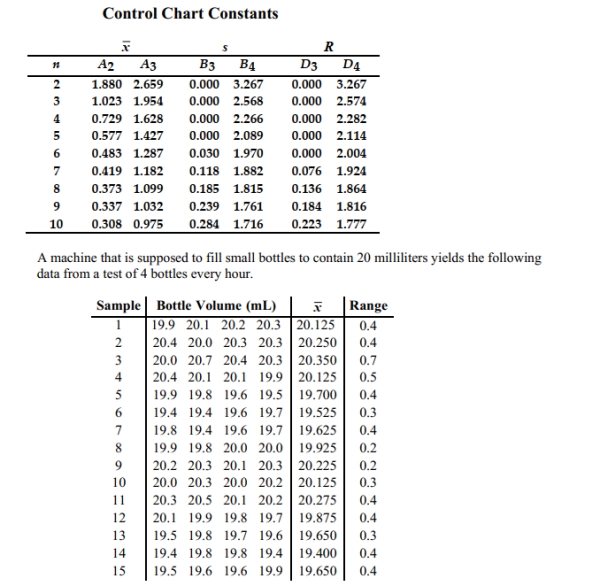
Control Chart Constants

The process appears to be within statistical control. 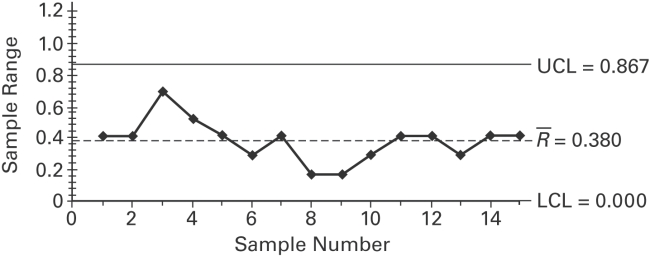

4
Sketch a control chart that indicates that a process is not statistically stable due to the run of 8
rule.
rule.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
5
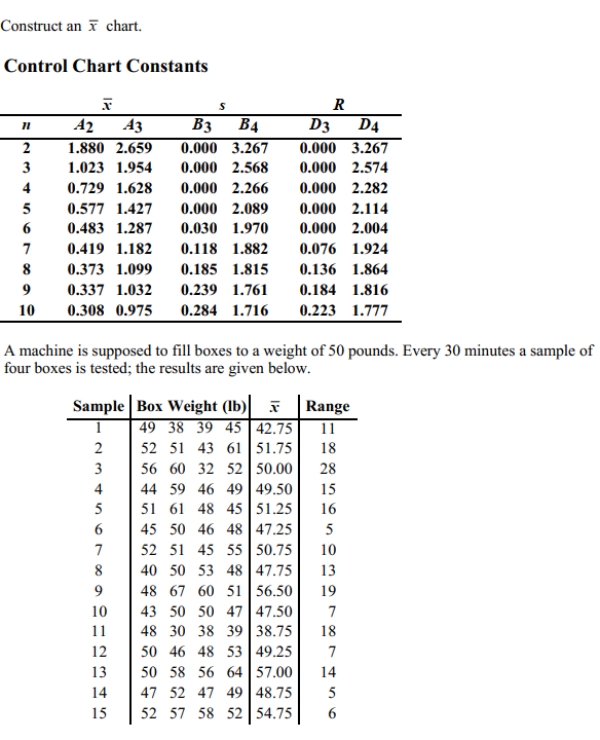

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
6
Construct an R chart and determine whether the process variation is within statistical control.
Control Chart Constants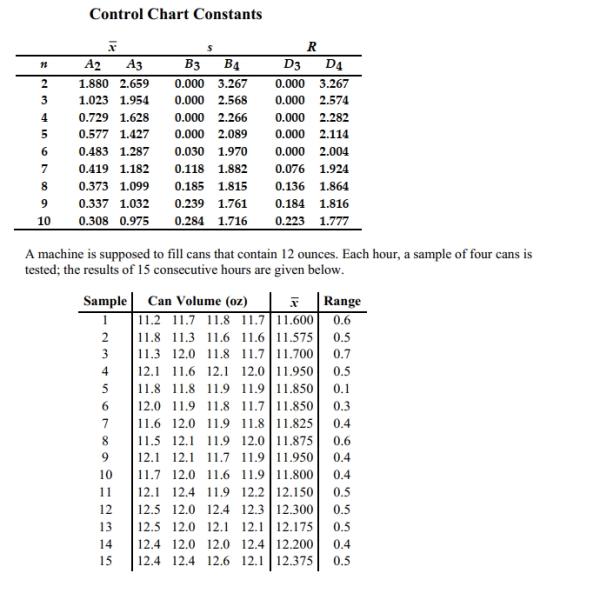
Control Chart Constants


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
7
Describe the three criteria used to determine if a control chart indicates a process which is not_
statistically stable.
statistically stable.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
8
Describe a run chart and give an example. Refer to the values on each of the axes as you
describe the run chart.
describe the run chart.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
9
A control chart for R is shown below. Determine whether the process variation is within_
statistical control. If it is not, identify which of the three out-of-control criteria lead to rejection
of statistically stable variation.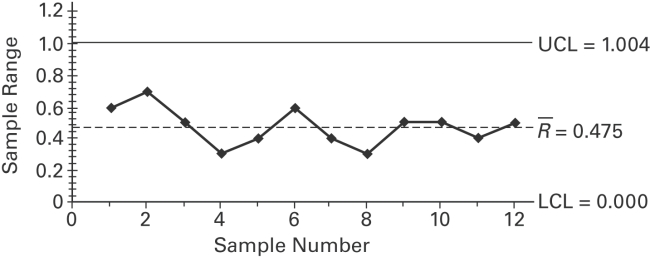
statistical control. If it is not, identify which of the three out-of-control criteria lead to rejection
of statistically stable variation.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
10

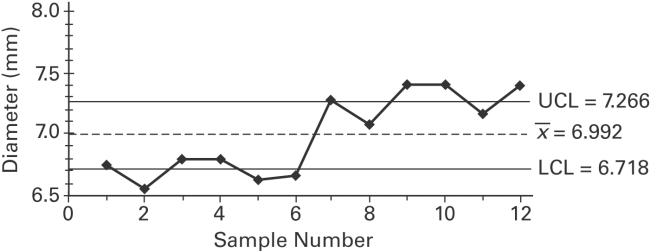

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
11
Examine the given run chart or control chart and determine whether the process is within_
statistical control. If it is not, identify which of the three out-of-statistical-control criteria apply.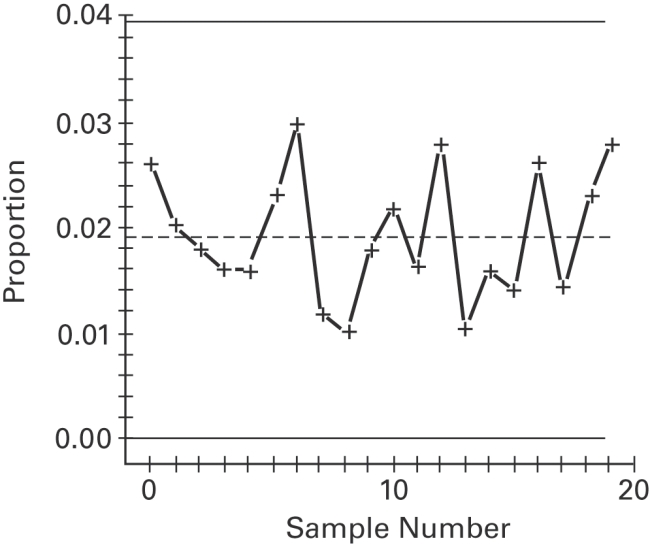
statistical control. If it is not, identify which of the three out-of-statistical-control criteria apply.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
12
Provide an appropriate response. Describe what process data are. Why are process data
important to businesses? What is a common goal of businesses using quality control?
important to businesses? What is a common goal of businesses using quality control?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
13
A control chart for 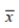 is shown below. Determine whether the process mean is within_
is shown below. Determine whether the process mean is within_
statistical control. If it is not, identify which of the three out-of-control criteria lead to
rejection of a statistically stable mean.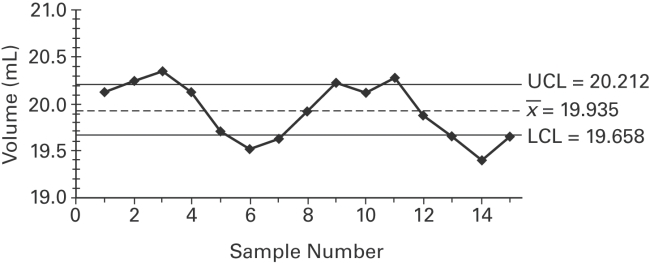
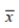 is shown below. Determine whether the process mean is within_
is shown below. Determine whether the process mean is within_statistical control. If it is not, identify which of the three out-of-control criteria lead to
rejection of a statistically stable mean.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
14
Define statistically stable (or "within statistical control"). Show examples of run charts which
illustrate processes which are not statistically controlled. Discuss the pattern which indicates
the process is not statistically controlled for each example.
illustrate processes which are not statistically controlled. Discuss the pattern which indicates
the process is not statistically controlled for each example.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
15
Use the given process data to construct a control chart for p. A manufacturer monitors the level_
of defects in the television sets that it produces. Each week, 200 television sets are randomly
selected and tested and the number of defects is recorded. The results for 12 consecutive weeks
are shown below.
of defects in the television sets that it produces. Each week, 200 television sets are randomly
selected and tested and the number of defects is recorded. The results for 12 consecutive weeks
are shown below.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
16
Construct a run chart for individual values corresponding to the given data. A machine is
supposed to fill boxes to a weight of 50 pounds. Every 30 minutes a sample of four boxes is
tested; the results are given below.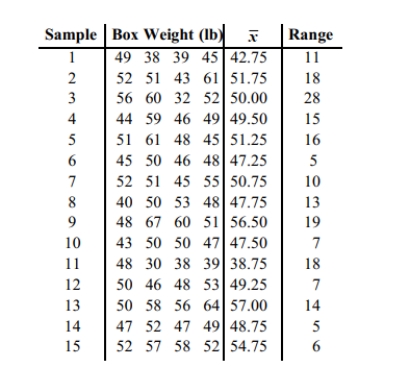
supposed to fill boxes to a weight of 50 pounds. Every 30 minutes a sample of four boxes is
tested; the results are given below.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
17
Examine the given run chart or control chart and determine whether the process is within
statistical control. If it is not, identity which of the three out-of-statistical-control criteria
apply. A run chart for individual values W is shown below. Does there appear to be a pattern
suggesting that the process is not within statistical control? If so, describe the pattern.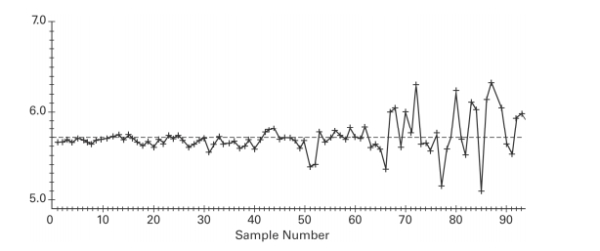
statistical control. If it is not, identity which of the three out-of-statistical-control criteria
apply. A run chart for individual values W is shown below. Does there appear to be a pattern
suggesting that the process is not within statistical control? If so, describe the pattern.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
18
A common goal of quality control is to reduce variation in a product or service. List and_
describe the two types of variability. Give an example of each.
describe the two types of variability. Give an example of each.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
19
Describe an R chart and give an example. What does it attempt to monitor?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
20
Use the given process data to construct a control chart for p. If the weight of cereal in a_
particular packet is less than 14 ounces, the packet is considered nonconforming. Each week,
the manufacturer randomly selects 1,000 cereal packets and determines the number that are
nonconforming. The results for 12 consecutive weeks are shown below.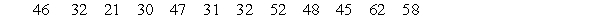
particular packet is less than 14 ounces, the packet is considered nonconforming. Each week,
the manufacturer randomly selects 1,000 cereal packets and determines the number that are
nonconforming. The results for 12 consecutive weeks are shown below.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
21
Construct an R chart and determine whether the process variation is within statistical control._ 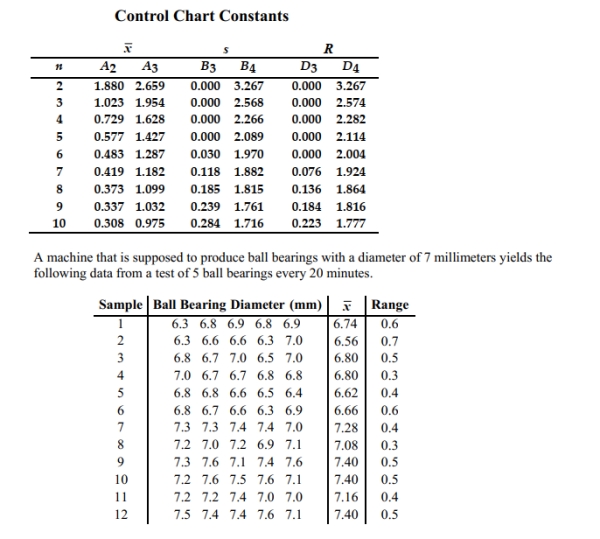


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
22
Examine the given run chart or control chart and determine whether the process is within_
statistical control. If it is not, identify which of the three out-of-statistical-control criteria apply.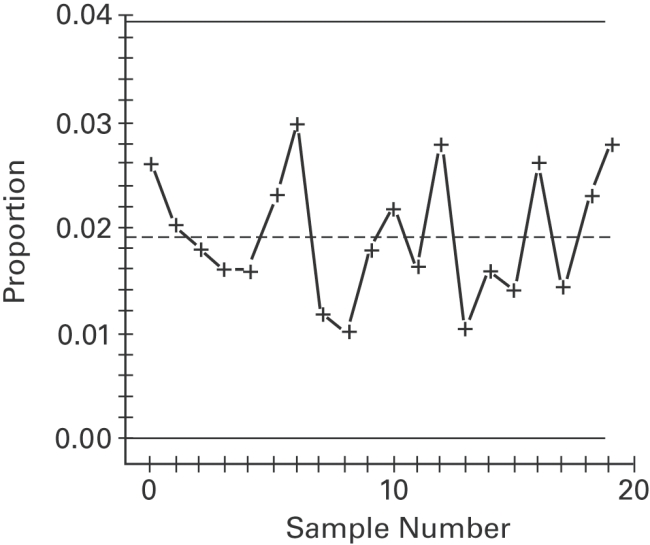
statistical control. If it is not, identify which of the three out-of-statistical-control criteria apply.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
23
Use the given process data to construct a control chart for p. A manufacturer monitors the level_
of defects in the television sets that it produces. Each week, 200 television sets are randomly
selected and tested and the number of defects is recorded. The results for 12 consecutive weeks
are shown below.
of defects in the television sets that it produces. Each week, 200 television sets are randomly
selected and tested and the number of defects is recorded. The results for 12 consecutive weeks
are shown below.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
24
Describe the three criteria used to determine if a control chart indicates a process which is not_
statistically stable.
statistically stable.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
25
Examine the given run chart or control chart and determine whether the process is within
statistical control. If it is not, identify which of the three out-of-statistical-control criteria
apply.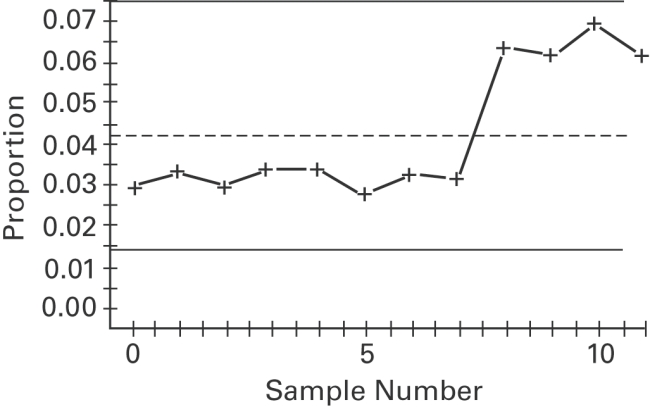
statistical control. If it is not, identify which of the three out-of-statistical-control criteria
apply.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
26
Use the given process data to construct a control chart for p. A drugstore considers a wait of
more than 5 minutes to be a defect. Each week 100 customers are randomly selected and
timed at the checkout line. The numbers of defects for 20 consecutive weeks are given below.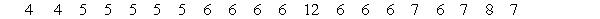
more than 5 minutes to be a defect. Each week 100 customers are randomly selected and
timed at the checkout line. The numbers of defects for 20 consecutive weeks are given below.
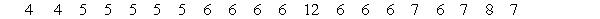

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
27
Construct an 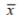 chart and determine whether the process variation is within statistical control.
chart and determine whether the process variation is within statistical control.
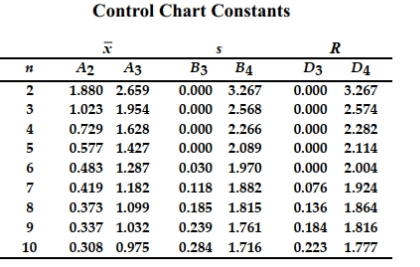
A machine that is supposed to produce ball bearings with a diameter of 7 millimeters yields the following data from a test of 5 ball bearings every 20 minutes.
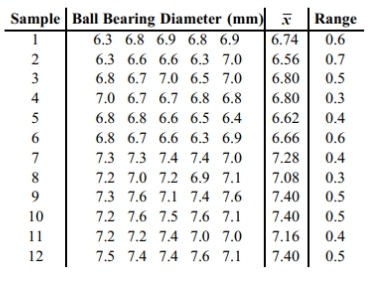
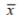 chart and determine whether the process variation is within statistical control.
chart and determine whether the process variation is within statistical control.
A machine that is supposed to produce ball bearings with a diameter of 7 millimeters yields the following data from a test of 5 ball bearings every 20 minutes.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
28
A control chart for attributes is to be constructed.
limits, a process which has been having a 5% rate of nonconforming items, or a process which
has been having a 10% of nonconforming items? Assume that both processes have the same
sample sizes. For a given sample size, would it be easier to detect a shift from 5% to 10% or a
shift from 10% to 15%? Explain your reasoning.
limits, a process which has been having a 5% rate of nonconforming items, or a process which
has been having a 10% of nonconforming items? Assume that both processes have the same
sample sizes. For a given sample size, would it be easier to detect a shift from 5% to 10% or a
shift from 10% to 15%? Explain your reasoning.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
29
A control chart for R is shown below. Determine whether the process variation is within_
statistical control. If it is not, identify which of the three out-of-control criteria lead to
rejection of statistically stable variation.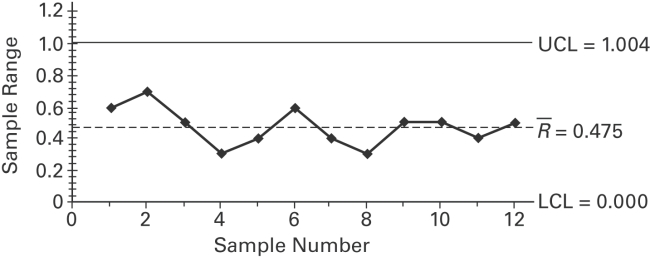
statistical control. If it is not, identify which of the three out-of-control criteria lead to
rejection of statistically stable variation.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
30
A control chart for 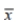 is shown below._
is shown below._
statistical control. If it is not, identify which of the three out-of-control criteria lead to rejection
of a statistically stable mean.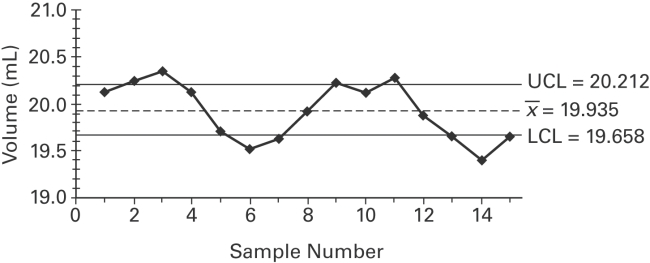
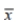 is shown below._
is shown below._statistical control. If it is not, identify which of the three out-of-control criteria lead to rejection
of a statistically stable mean.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
31
Sketch a run chart that that indicates that a process is not statistically stable due to increasing
variation.
variation.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
32
Construct a run chart for individual values corresponding to the given data. A machine that is_
supposed to produce ball bearings with a diameter of 7 millimeters yields the following data
from a test of 5 ball bearings every 20 minutes.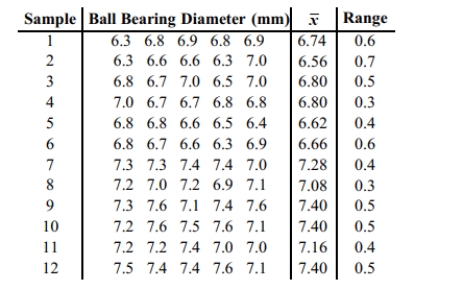
supposed to produce ball bearings with a diameter of 7 millimeters yields the following data
from a test of 5 ball bearings every 20 minutes.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
33
Provide an appropriate response. A common goal of quality control is to reduce variation in a
product or service. List and describe the two types of variability. Give an example of each.
product or service. List and describe the two types of variability. Give an example of each.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
34

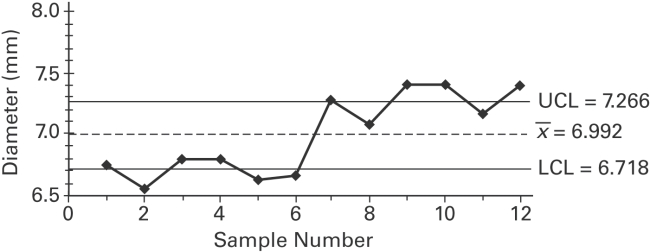

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
35
Describe a p chart and give an example.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
36
A run chart for individual values is shown below. Does there appear to be a pattern suggesting
that the process is not within statistical control? If so, describe the pattern.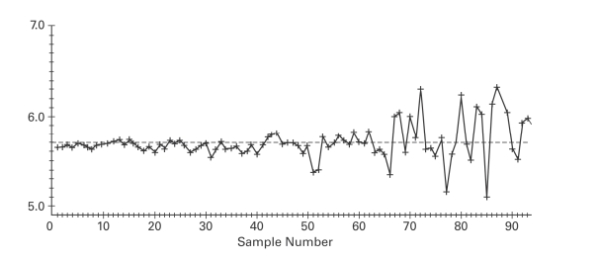
that the process is not within statistical control? If so, describe the pattern.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
37
Define statistically stable (or "within statistical control"). Show examples of run charts which
illustrate processes which are not statistically controlled. Discuss the pattern which indicates
the process is not statistically controlled for each example.
illustrate processes which are not statistically controlled. Discuss the pattern which indicates
the process is not statistically controlled for each example.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
38
A control chart for 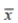 is shown below._
is shown below._
statistical control. If it is not, identify which of the three out-of-control criteria lead to
rejection of a statistically stable mean.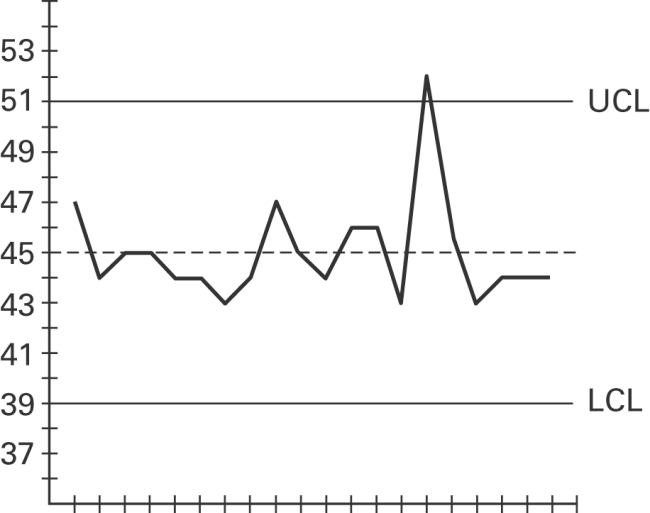
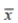 is shown below._
is shown below._statistical control. If it is not, identify which of the three out-of-control criteria lead to
rejection of a statistically stable mean.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
39
Examine the given run chart or control chart and determine whether the process is
within statistical control. If it is not, identify which of the three out-of-statistical-control
criteria apply.
within statistical control. If it is not, identify which of the three out-of-statistical-control
criteria apply.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
40
Consider process data consisting of the amounts of potato chips (in ounces)in randomly selected bags of chips. The process is to be monitored with  control charts based on samples
control charts based on samples
Of 50 bags randomly selected each day for 15 consecutive days of production. What does
X
Denote?
A)The mean of 50 bags.
B)The mean of all 15 sample means.
C)The mean of the ranges for the 15 days.
D)The mean of the standard deviations for the 15 days.
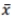 control charts based on samples
control charts based on samplesOf 50 bags randomly selected each day for 15 consecutive days of production. What does
X
Denote?
A)The mean of 50 bags.
B)The mean of all 15 sample means.
C)The mean of the ranges for the 15 days.
D)The mean of the standard deviations for the 15 days.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
41
__________________ variation results from causes that can be identified._
A)Random
B)Identifiable
C)Assignable
D)Definable
A)Random
B)Identifiable
C)Assignable
D)Definable

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
42
Use the given process data to construct a control chart for p. A drugstore considers a wait of more than 5 minutes to be a defect. Each week 100 customers are randomly selected and
Timed at the checkout line. The numbers of defects for 20 consecutive weeks are given below. What is the value of the upper control limit?
What is the value of the upper control limit?
A)1
B)0.0783
C)0.0622
D)6.2
Timed at the checkout line. The numbers of defects for 20 consecutive weeks are given below.
 What is the value of the upper control limit?
What is the value of the upper control limit?A)1
B)0.0783
C)0.0622
D)6.2

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
43
________________ measurement of a characteristic or good or services that result from some combination of
Equipment, people, materials, methods, and conditions.
A)Process
B)Service
C)Sequential
D)Material
Equipment, people, materials, methods, and conditions.
A)Process
B)Service
C)Sequential
D)Material

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
44
R charts are used to monitor_____________._
A)means
B)proportions
C)variation
D)correlation
A)means
B)proportions
C)variation
D)correlation

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
45
List the advantages and disadvantages of nonparametric tests._

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
46
Which graph using individual data values instead of a process characteristic? 


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
47
Examine the given run chart or control chart and determine whether the process is within_ statistical control. 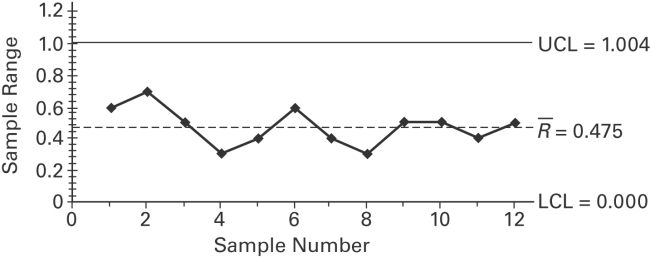
A)The process is within statistical control.
B)The process is not within statistical control.

A)The process is within statistical control.
B)The process is not within statistical control.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
48
Control charts are used to monitor changing characteristics of data over ____________._
A)time
B)space
C)distance
D)speed
A)time
B)space
C)distance
D)speed

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
49
Examine the given run chart or control chart and determine whether the process is within statistical control. 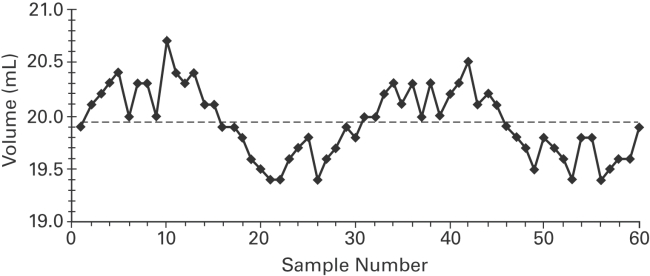
A)The process is within statistical control.
B)The process is not within statistical control.

A)The process is within statistical control.
B)The process is not within statistical control.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
50
Examine the given run chart or control chart and determine whether the process is within_ statistical control. 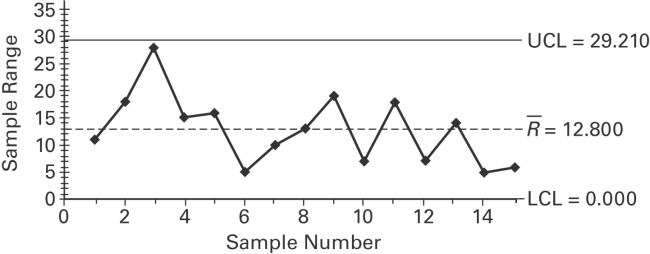
A)The process is within statistical control.
B)The process is not within statistical control.

A)The process is within statistical control.
B)The process is not within statistical control.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
51
Examine the given run chart or control chart and determine whether the process is within statistical control. 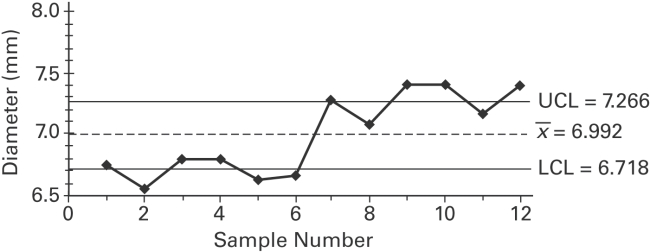
A)The process is within statistical control.
B)The process is not within statistical control.

A)The process is within statistical control.
B)The process is not within statistical control.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
52
Which type of chart would be best for monitoring the proportion of items that are defective? 


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
53
The centerline for a control chart for R consists of _______________._
A)the mean of the sample ranges
B)the mean of the sample data
C)the median
D)the standard deviation
A)the mean of the sample ranges
B)the mean of the sample data
C)the median
D)the standard deviation

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
54
Use the given process data to construct a control chart for p. A candy manufacturer considers a cracked piece of candy to be a defect. Each day 100 candies are randomly selected and
Checked for cracks. The numbers of defects for 20 consecutive days are given below.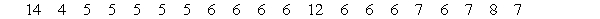 What is the value of the center line?
What is the value of the center line?
A)6.6
B)10
C)0.066
D)1.32
Checked for cracks. The numbers of defects for 20 consecutive days are given below.
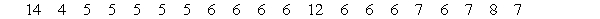 What is the value of the center line?
What is the value of the center line?A)6.6
B)10
C)0.066
D)1.32

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
55
A manufacturer of lightbulbs picks 10 lightbulbs at random each day for quality control. The mean and range (in hours)of the lifetimes of the 10 lightbulbs are calculated. The results for
12 consecutive days are shown in the table below. Construct an R chart to determine whether
The process is in statistical control.
A)
B)
12 consecutive days are shown in the table below. Construct an R chart to determine whether
The process is in statistical control.
A)
B)

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
56
A _________________ is a sequential plot of individual data values over time. One axis is used_ for the data values, and the other axis is used for the time sequence.
A)run chart
B)process chart
C)time-process chart
D)flow chart
A)run chart
B)process chart
C)time-process chart
D)flow chart

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
57
A machine that is supposed to fill small bottles to contain 20 milliliters yields the following data from a test of 4 bottles every hour. What would be the centerline for a control chart for
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
58
Use the Wilcoxon signed -ranks test to test the claim that the matched pairs have differences_
that come from a population with a median equal to zero. Eleven runners are timed at the 100-
meter dash and are timed again one month later after following a new training program. The
times (in seconds)are shown in the table. Use Wilcoxon's signed-ranks test and a significance
level of 0.05 to test the claim that the training has no effect on the times.
that come from a population with a median equal to zero. Eleven runners are timed at the 100-
meter dash and are timed again one month later after following a new training program. The
times (in seconds)are shown in the table. Use Wilcoxon's signed-ranks test and a significance
level of 0.05 to test the claim that the training has no effect on the times.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
59
Which of the following is not one of the criteria for determining if a control chart indicates that_ a process is not statistically stable?
A)Exceptional value
B)Upward shift
C)Downward trend
D)Decreasing variation
A)Exceptional value
B)Upward shift
C)Downward trend
D)Decreasing variation

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
60
Define rank. Explain how to find the rank for data which repeats (for example, the data set: 4,_
5, 5, 5, 7, 8, 12, 12, 15, 18).
5, 5, 5, 7, 8, 12, 12, 15, 18).

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
61
Describe the sign test. What types of hypotheses is it used to test? What is the underlying
concept?
concept?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
62
Use the runs test to determine whether the given sequence is random. Use a significance level_
of 0.05. A true-false test had the following answer sequence. Test the null hypothesis that the sequence was random.
Test the null hypothesis that the sequence was random.
of 0.05. A true-false test had the following answer sequence.
 Test the null hypothesis that the sequence was random.
Test the null hypothesis that the sequence was random.
Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
63
Provide the appropriate response. Describe the Wilcoxon signed-ranks test. What types of_
hypotheses is it used to test? What assumptions are made for this test?
hypotheses is it used to test? What assumptions are made for this test?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
64
A standard aptitude test is given to several randomly selected programmers, and the scores are
given below for the mathematics and verbal portions of the test. Use the sign test to test the
claim that programmers do better on the mathematics portion of the test. Use a 0.05 level of
significance.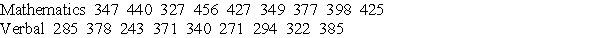
given below for the mathematics and verbal portions of the test. Use the sign test to test the
claim that programmers do better on the mathematics portion of the test. Use a 0.05 level of
significance.
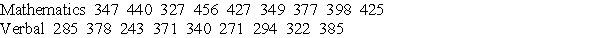

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
65
Use a Kruskal-Wallis test to test the claim that the samples come from populations with equal_
medians. Listed below are grade averages for randomly selected students with three different
categories of high-school background. At the 0.05 level of significance, test the claim that the
three groups have the same median grade average.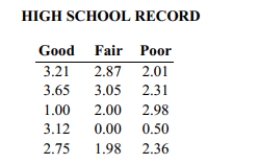
medians. Listed below are grade averages for randomly selected students with three different
categories of high-school background. At the 0.05 level of significance, test the claim that the
three groups have the same median grade average.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
66
Use the rank correlation coefficient to test for a correlation between the two variables. A_
placement test is required for students desiring to take a finite mathematics course at a
university. The instructor of the course studies the relationship between students' placement
test score and final course score. A random sample of eight students yields the following data.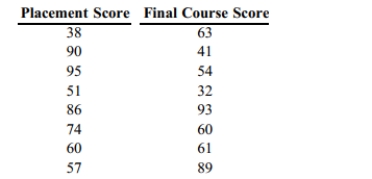 Compute the rank correlation coefficient, rs, of the data and test the claim of correlation
Compute the rank correlation coefficient, rs, of the data and test the claim of correlation
between placement score and final course score. Use a significance level of 0.05.
placement test is required for students desiring to take a finite mathematics course at a
university. The instructor of the course studies the relationship between students' placement
test score and final course score. A random sample of eight students yields the following data.
 Compute the rank correlation coefficient, rs, of the data and test the claim of correlation
Compute the rank correlation coefficient, rs, of the data and test the claim of correlationbetween placement score and final course score. Use a significance level of 0.05.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
67
Use the sign test to test the indicated claim. Fourteen people rated two brands of soda on a scale_
of 1 to 5.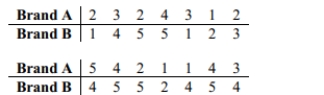 At the 5 percent level, test the null hypothesis that the two brands of soda are equally popular.
At the 5 percent level, test the null hypothesis that the two brands of soda are equally popular.
of 1 to 5.
 At the 5 percent level, test the null hypothesis that the two brands of soda are equally popular.
At the 5 percent level, test the null hypothesis that the two brands of soda are equally popular.
Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
68
Use the rank correlation coefficient to test for a correlation between the two variables.
Given that the rank correlation coefficient, rs, for 37 pairs of data is 0.324, test the claim of
correlation between the two variables. Use a significance level of 0.01.
Given that the rank correlation coefficient, rs, for 37 pairs of data is 0.324, test the claim of
correlation between the two variables. Use a significance level of 0.01.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
69
Use a 0.05 level of significance to test the claim that the sequence of computer -generated
numbers is random. Test for randomness above and below the mean.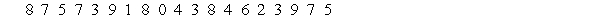
numbers is random. Test for randomness above and below the mean.
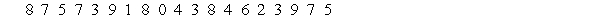

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
70
Use the runs test to determine whether the given sequence is random. Use a significance level_
of 0.05. The sequence of numbers below represents the maximum temperature (in degrees
Fahrenheit)in July in one U.S. town for 30 consecutive years. Test the sequence for
randomness above and below the median.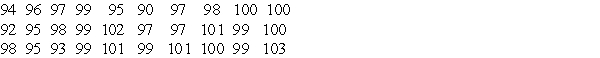
of 0.05. The sequence of numbers below represents the maximum temperature (in degrees
Fahrenheit)in July in one U.S. town for 30 consecutive years. Test the sequence for
randomness above and below the median.
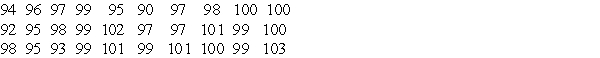

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
71
Use the Wilcoxon rank-sum test to test the claim that the two independent samples come from
populations with equal medians. Use the Wilcoxon rank-sum approach to test the claim that
the sample student grade averages at two colleges come from populations with the same
median. The sample data is listed below. Use a 0.05 level of significance, and assume that the
samples were randomly selected.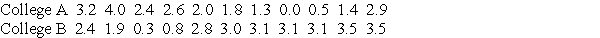
populations with equal medians. Use the Wilcoxon rank-sum approach to test the claim that
the sample student grade averages at two colleges come from populations with the same
median. The sample data is listed below. Use a 0.05 level of significance, and assume that the
samples were randomly selected.
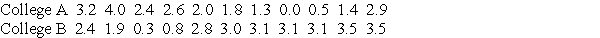

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
72
When applying the runs test for randomness above and below the median for 12 dollar/Euro
exchange rate highs, the test statistic is G = 2. What does that value tell us about the data?
exchange rate highs, the test statistic is G = 2. What does that value tell us about the data?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
73
Describe the Wilcoxon rank-sum test. What type of hypotheses is it used to test? What
assumptions are made for this test? What is the underlying concept?
assumptions are made for this test? What is the underlying concept?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
74
Explain what an efficiency rating is. You may use an example to explain this concept. Do
comparable parametric or nonparametric tests have higher efficiency ratings?
comparable parametric or nonparametric tests have higher efficiency ratings?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
75
Solve the problem. Critical values for the runs test for randomness can be calculated by listing_
all possible sequences. Using the elements B, B, B, R, R, R list the 20 different possible
sequences. Find the number of runs for each sequence. Are you able to find 5% cutoff values
for G? What do you conclude?
all possible sequences. Using the elements B, B, B, R, R, R list the 20 different possible
sequences. Find the number of runs for each sequence. Are you able to find 5% cutoff values
for G? What do you conclude?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
76
Use the Wilcoxon rank-sum test to test the claim that the two independent samples come from_
populations with equal medians. SAT scores for students selected randomly from two different
schools are shown below. Use a significance level of 0.05 to test the claim that the scores for
the two schools are from populations with the same median.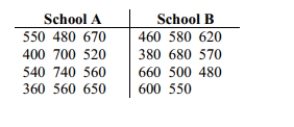
populations with equal medians. SAT scores for students selected randomly from two different
schools are shown below. Use a significance level of 0.05 to test the claim that the scores for
the two schools are from populations with the same median.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
77
Describe the rank correlation test. What types of hypotheses is it used to test? How does the
rank correlation coefficient rs differ from the Pearson correlation coefficient r?
rank correlation coefficient rs differ from the Pearson correlation coefficient r?

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
78
Use the sign test to test the indicated claim. The heights of 16 randomly selected women are
given below. Use a significance level of 0.05 to test the claim that the population median is
equal to 64.0 inches.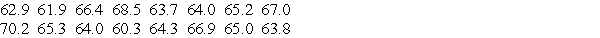
given below. Use a significance level of 0.05 to test the claim that the population median is
equal to 64.0 inches.
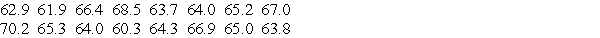

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
79
Give at least two examples of nonparametric tests and their comparable parametric tests.

Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck
80
Use a Kruskal-Wallis test to test the claim that the samples come from populations with equal_
medians. Listed below are grade averages for randomly selected students with three different
categories of high-school background. At the 0.05 level of significance, test the claim that the
three groups have the same median grade average.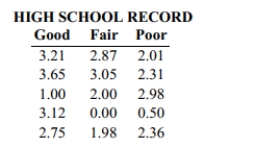
medians. Listed below are grade averages for randomly selected students with three different
categories of high-school background. At the 0.05 level of significance, test the claim that the
three groups have the same median grade average.


Unlock Deck
Unlock for access to all 177 flashcards in this deck.
Unlock Deck
k this deck



