Deck 23: Understanding Time Value of Money Formulas and Concepts
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/142
Play
Full screen (f)
Deck 23: Understanding Time Value of Money Formulas and Concepts
1
Compounding is the conversion of future cash flow amounts to their present value.
False
2
The formula to compute the present value of a dollar is
True
3
The future value grows more quickly when interest is compounded monthly than when interest is compounded annually.
True
4
One type of compensation provided by the time value of money is compensation for expected consumption.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
5
The formula to compute the future value of a single sum is

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
6
To determine an unstated interest rate, divide the future amount by the present value then divide by the number of periods.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
7
An ordinary annuity is if the cash flows occur on the first day of each period.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
8
The formula for the future value of an ordinary annuity of any amount is:

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
9
The interest that accrues on both the principal and the past unpaid accrued interest is called compound interest.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
10
The future value of an ordinary annuity is determined immediately after the last cash flow in the series occurs.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
11
The present value factors for any discount rate increase as the number of periods increases.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
12
One type of compensation provided by the time value of money is compensation for risk.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
13
The present value of a future amount depends on two variables: the interest rate and the number of periods.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
14
Discounting is the conversion of future cash flow amounts to their present value.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
15
An annuity is the same amount at the same time every period

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
16
An annuity due is an annuity for which the cash flows occur on the first day of each period.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
17
The formula to compute the future value of a single sum is:

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
18
The future value of an amount depends on two variables: the interest rate and the number of payments

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
19
The present value of an amount decreases as the discount rate increases.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
20
The future value of an ordinary annuity is higher if the discount rate is higher.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
21
Interest calculated on the original principal regardless of the number of time periods that have passed or the amount of interest that has been paid or accrued in the past is
A) compound interest.
B) simple interest.
C) present value of future cash flows.
D) future value of a single sum.
A) compound interest.
B) simple interest.
C) present value of future cash flows.
D) future value of a single sum.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
22
The method of converting a future dollar amount into its present dollar value by removing the time value of money is called
A) discounting
B) compounding
C) amortizing
D) interpolation
A) discounting
B) compounding
C) amortizing
D) interpolation

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
23
Compound interest is
A) calculated by multiplying the principal times the rate times the period of time.
B) interest on the original principal plus any past unpaid accrued interest to date.
C) interest on the original principal paid or received.
D) interest on any past unpaid interest accrued to date.
A) calculated by multiplying the principal times the rate times the period of time.
B) interest on the original principal plus any past unpaid accrued interest to date.
C) interest on the original principal paid or received.
D) interest on any past unpaid interest accrued to date.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
24
To calculate the present value of an annuity due the formula is:

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
25
The present value of an annuity is the present value of a series of equal cash flows that occur in the future.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
26
The future value of an annuity due is lower if the discount rate is higher.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
27
The formula to calculate a present value of a deferred annuity is:
PVdeferred = C × Converted Factor for Present Value of Deferred Annuity of 1)
PVdeferred = C × Converted Factor for Present Value of Deferred Annuity of 1)

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
28
Simple interest on a $25,000, 8%, 18-month note is
A) $22,000.
B) $23,000.
C) $3,000.
D) $2,000.
A) $22,000.
B) $23,000.
C) $3,000.
D) $2,000.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
29
The future value of an annuity due is determined one period after the first cash flow in the series.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
30
Interest compounded monthly on a $10,000 principal amount at 18% for two years is
A) $1,800.
B) $3,600.
C) $3,924.
D) $4,295.
A) $1,800.
B) $3,600.
C) $3,924.
D) $4,295.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
31
To calculate the present value of four annual installments of $1,000 at an 8% interest rate beginning on January 1, 2016 and payments due on December 31 of each year, one would use the present value of an ordinary annuity table.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
32
To compare the value of amounts received at different times in the future, dollar amounts
A) may be restated to their present value through discounting or restated to their future value by compounding.
B) must be converted to a single sum.
C) must be restated to their future value by adding the compound interest to date.
D) must be restated to their present value by removing the interest from the amount to be received in the future.
A) may be restated to their present value through discounting or restated to their future value by compounding.
B) must be converted to a single sum.
C) must be restated to their future value by adding the compound interest to date.
D) must be restated to their present value by removing the interest from the amount to be received in the future.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
33
The present value of a deferred annuity is determined on today's date, because the annuity payments begin some period after today's date.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
34
FASB's Statement of Financial Accounting Concepts No. 7 specifies when fair value should be based on present value.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
35
FASB's Statement of Financial Accounting Concepts No. 7 provides general principles governing the use of present value and the objectives of present value accounting measurements.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
36
Interest compounded quarterly on a $100,000 principal amount at 12% for one year is
A) $11,151.
B) $12,000.
C) $12,551.
D) $12,683.
A) $11,151.
B) $12,000.
C) $12,551.
D) $12,683.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
37
Simple interest on a $1,250,000, 9%, 15-month note is
A) $ 90,000.
B) $112,500.
C) $140,625.
D) $168,750.
A) $ 90,000.
B) $112,500.
C) $140,625.
D) $168,750.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
38
The present value of an annuity due is determined on the date of the last cash flow in the series.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
39
The formula to calculate the present value of an ordinary annuity is:

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
40
The amount of future cash flows is an accounting measurement that is considered relevant for decisions made by financial statement users.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
41
The future value of $50,000 deposited today and compounded quarterly at an 8% annual interest rate for seven years is
A) $57,434.
B) $87,051.
C) $85,691.
D) $78,000.
A) $57,434.
B) $87,051.
C) $85,691.
D) $78,000.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
42
Tessa won the lottery for $2,500,000 but due to a change in state laws she will not be able to collect it for three years. Ralph is willing to give her a lump sum today in return for the payment in three years. If current interest rates are 14% per year, how much will Tessa receive today?
A) $1,687,430
B) $5,804,080
C) $2,500,000
D) $3,703,860
A) $1,687,430
B) $5,804,080
C) $2,500,000
D) $3,703,860

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
43
If $100,000 is invested on December 31, 2016 to earn compound interest semiannually, and if the future value on December 31, 2022, is $225,219 what is the semiannual interest rate on the investment?
A) 7%
B) 6%
C) 5%
D) 8%
A) 7%
B) 6%
C) 5%
D) 8%

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
44
The future value of $7,000 deposited today and compounded quarterly at a 16% annual interest rate for five years is
A) $14,724.
B) $14,702.
C) $8,517.
D) $15,338.
A) $14,724.
B) $14,702.
C) $8,517.
D) $15,338.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
45
The future value of $7,000 deposited today and compounded semiannually at an 9% annual interest rate for four years is
A) $9,955.
B) $9,520.
C) $8,100.
D) $7,920.
A) $9,955.
B) $9,520.
C) $8,100.
D) $7,920.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
46
What is the formula for the future value of a single amount at compound interest? 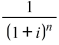
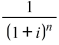

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
47
Table factors for present values
A) decrease as the interest rate decreases
B) decrease as the number of periods increases
C) increase as the interest rate increases
D) increase as the number of periods increases
A) decrease as the interest rate decreases
B) decrease as the number of periods increases
C) increase as the interest rate increases
D) increase as the number of periods increases

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
48
All of the following are conditions for an ordinary annuity due except
A) periodic cash flows must be equal in amount.
B) the time periods between the cash flows are the same length.
C) the future value is equal to the present value.
D) interest is compounded at the end of each time period.
A) periodic cash flows must be equal in amount.
B) the time periods between the cash flows are the same length.
C) the future value is equal to the present value.
D) interest is compounded at the end of each time period.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
49
The present value of $500,000 received at the end of five years discounted at 10% is
A) $805,255.
B) $310,461.
C) $306,957.
D) none of these
A) $805,255.
B) $310,461.
C) $306,957.
D) none of these

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
50
An annuity is a series of
A) equal payments with interest compounded annually.
B) payments made at regular intervals in the future with interest compounded yearly.
C) payments made at points in the future earning simple interest on a regular basis.
D) equal payments made at regular intervals in the future with interest compounded at the end of each time period.
A) equal payments with interest compounded annually.
B) payments made at regular intervals in the future with interest compounded yearly.
C) payments made at points in the future earning simple interest on a regular basis.
D) equal payments made at regular intervals in the future with interest compounded at the end of each time period.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
51
Marco needs $175,000 six years from today. How much should Marco deposit today into an investment account that provides a 12% annual return in order to accomplish his goals?
A) $89,523
B) $88,660
C) $85,487
D) $62,500
A) $89,523
B) $88,660
C) $85,487
D) $62,500

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
52
Margaret will receive an insurance settlement of $3,000,000 in five years. Randall is willing to give her a lump sum today in return for the payment in five years. If current interest rates are 12% per year, how much will Margaret receive today?
A) $960,637
B) $1,702,281
C) $1,116,790
D) $1,800,000
A) $960,637
B) $1,702,281
C) $1,116,790
D) $1,800,000

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
53
Bruno deposited $7,500 into an investment account and seven years later, the balance in the account was $10,910. What is the rate of return on this investment if interest is compounded annually?
A) 45.5%
B) 6.5%
C) 6.0%
D) 5.5%
A) 45.5%
B) 6.5%
C) 6.0%
D) 5.5%

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
54
Each of the following compound interest factors has the same number of periods n) at the same interest rate i). Which one is the table factor for the present value of a single sum?
A) 1.500730
B) 7.153291
C) 0.666342
D) 4.766540
A) 1.500730
B) 7.153291
C) 0.666342
D) 4.766540

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
55
On April 1, 2016, Meyers Company purchased a bulldozer. Payment, totaling $70,000, is not due until April 1, 2018. Assuming interest at a 12% annual rate, Meyers should debit Machinery on April 1, 2016, in the amount of
A) $70,000.
B) $62,500.
C) $61,600.
D) $55,804.
A) $70,000.
B) $62,500.
C) $61,600.
D) $55,804.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
56
Mildred desires to have $7,049 on deposit five years from today. If she has $4,000 to deposit, what rate of interest, compounded annually, must be obtained to accumulate the desired $7,049 in five years?
A) 12%
B) 10%
C) 9%
D) 8%
A) 12%
B) 10%
C) 9%
D) 8%

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
57
All of the following are conditions for an ordinary annuity except
A) periodic cash flows must be equal in amount
B) the time periods between the cash flows are the same length
C) the interest rate is constant for each time period
D) interest is compounded in the middle of each time period
A) periodic cash flows must be equal in amount
B) the time periods between the cash flows are the same length
C) the interest rate is constant for each time period
D) interest is compounded in the middle of each time period

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
58
What is the formula for the present value of a single sum at compound interest? 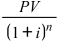
A)
B) FV × 1 + i)n
C)
D)
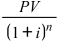
A)
B) FV × 1 + i)n
C)
D)

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
59
Maxine has $1,000 to invest today. How much will her money be worth in 15 years if she earns 9% compounded semiannually on her money?
A) $3,745
B) $13,268
C) $3,642
D) $1,935
A) $3,745
B) $13,268
C) $3,642
D) $1,935

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
60
All of the following are conditions for an annuity due except
A) periodic cash flows must be equal in amount.
B) the time periods between the cash flows are the same length.
C) the interest rate is constant for each time period.
D) interest is compounded at the end of each time period.
A) periodic cash flows must be equal in amount.
B) the time periods between the cash flows are the same length.
C) the interest rate is constant for each time period.
D) interest is compounded at the end of each time period.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
61
What is the formula for the future value of an ordinary annuity ? 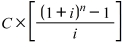
A)
B)
C)
D)
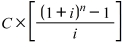
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
62
Currently on January 1, 2017), Nolan wants to have $45,000 available on December 31 2022 to purchase a luxury car. To be able to have this amount available, Nolan will make equal quarterly deposits for the next six years in an investment account earning a 16% annual return compounded quarterly. Nolan will make these deposits at the end of March, June, September, and December. What is the amount to be deposited quarterly for the next six years that will provide for a $45,000 balance at the end of 2022?
A) $1,875
B) $1,253
C) $1,151
D) $210
A) $1,875
B) $1,253
C) $1,151
D) $210

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
63
What is the formula for the present value of an ordinary annuity of 1? 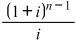
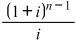

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
64
Jacob Sawyer will deposit $3,000 into a special account each year beginning December 31, 2016, with the last deposit being made on December 31, 2019. Jacob wants to know how much will be in his account on December 31, 2019, immediately after the final deposit, if the account earns 10% compounded annually. To solve the problem, Jacob must find the future value of
A) a single sum.
B) a deferred annuity.
C) an ordinary annuity.
D) an annuity due.
A) a single sum.
B) a deferred annuity.
C) an ordinary annuity.
D) an annuity due.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
65
At the beginning of 2017, Laura Company issued 10-year bonds with a face value of $4,000,000 due on December 31, 2022. The company will accumulate a fund to retire these bonds at maturity. It will make ten annual deposits to the fund beginning on December 31, 2017. How much must the company deposit each year, assuming that it will earn 12% interest compounded annually?
A) $363,636.36
B) $227,936.65
C) $226,008.92
D) $203,514.87
A) $363,636.36
B) $227,936.65
C) $226,008.92
D) $203,514.87

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
66
In order to measure the carrying value of investments in bonds, which of the following time value of money concepts is used?
A) the present value of an ordinary annuity
B) the future value of a single sum
C) the future value of an ordinary annuity
D) all of these
A) the present value of an ordinary annuity
B) the future value of a single sum
C) the future value of an ordinary annuity
D) all of these

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
67
The future amount of an annuity due is determined
A) one period after the last cash flow in the series.
B) one period before the last cash flow in the series.
C) at the same time as the last cash flow in the series.
D) one period after the next cash flow in the series.
A) one period after the last cash flow in the series.
B) one period before the last cash flow in the series.
C) at the same time as the last cash flow in the series.
D) one period after the next cash flow in the series.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
68
Savannah has just won the state lottery. She will receive ten equal annual payments of $15,000, beginning one year from today. Assuming an 8% interest rate compounded annually, the present value of those receipts today is
A) $80,913.
B) $100,651.
C) $108,703.
D) $102,000.
A) $80,913.
B) $100,651.
C) $108,703.
D) $102,000.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
69
In the present value of an annuity table, the factors.
A) increase as the interest rates increase.
B) decrease as the periods increase.
C) increase as the periods decrease.
D) decrease as the interest rates increase.
A) increase as the interest rates increase.
B) decrease as the periods increase.
C) increase as the periods decrease.
D) decrease as the interest rates increase.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
70
Georgia deposits $4,000 every three months for five years. The first deposit is made on March 31, 2016, and the last deposit is made on December 31, 2020. The fund earns 16% and interest is compounded quarterly. How much money will Georgia have on December 31, 2020, immediately after her last deposit? Factors for future value of an annuity of $1 are
A) $123,876
B) $119,112
C) $110,034
D) $107,508
A) $123,876
B) $119,112
C) $110,034
D) $107,508

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
71
Using the table approach, the future amount of an annuity due may be calculated by finding the table factor for the future amount of an ordinary annuity of
A) n + 1 and then subtract 1.
B) n + 1 and then add 1.
C) n - 1 and then add 1.
D) n - 1 and then subtract 1.
A) n + 1 and then subtract 1.
B) n + 1 and then add 1.
C) n - 1 and then add 1.
D) n - 1 and then subtract 1.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
72
Anne wants to accumulate $25,000 by December 31, 2019. To accumulate that sum, she will make twelve equal quarterly deposits of $1,616.66 at the end of March, June, September, and December, beginning on March 31, 2016, into a fund that earns interest compounded quarterly. What annual rate of interest must the fund provide to yield the desired sum?
A) 4.5%
B) 6.5%
C) 18%
D) 26%
A) 4.5%
B) 6.5%
C) 18%
D) 26%

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
73
Stephen Michaels wants to know how much he must deposit today at 12% interest to provide three equal annual withdrawals of $10,000, beginning one year from now. This is an example of the present value of
A) an ordinary annuity.
B) an annuity due.
C) a single sum.
D) a deferred annuity.
A) an ordinary annuity.
B) an annuity due.
C) a single sum.
D) a deferred annuity.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
74
On January 2, 2016, Christopher inherited a trust fund that he could use for college tuition. Christopher hopes to make five equal withdrawals of $40,000 each year for the next five years from the fund that will earn 10% compounded annually. The first withdrawal will be made on January 2, 2017. How much does he need to have invested in the fund on January 2, 2016, to be able to withdraw the needed amounts each year?
A) $151,631
B) $200,000
C) $244,204
D) $268,624
A) $151,631
B) $200,000
C) $244,204
D) $268,624

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
75
Jeff desires to accumulate $13,603.83 by December 1, 2018. To accumulate that sum, he will make six equal semiannual deposits of $2,000, beginning on June 1, 2016, into a fund that earns interest compounded semiannually. What annual rate of interest must the fund provide to yield the desired sum?
A) 5%
B) 6%
C) 10%
D) 12%
A) 5%
B) 6%
C) 10%
D) 12%

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
76
Jessie's Dry Cleaner began making $2,000 equal, annual deposits in a fund starting on January 2, 2016. The fund earns 10% compounded annually, and the last deposit is made on January 2, 2020. How much will be in the fund on January 2, 2021, one year after the final deposit?
A) $15,000
B) $13,431
C) $12,105
D) $10,641
A) $15,000
B) $13,431
C) $12,105
D) $10,641

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
77
Currently in August, 2017), Abby wants to have $20,000 available in August 2021 to make a college tuition payment. To be able to have this amount available, Abby will make equal annual deposits in an investment account earning 12% annually in August 2017,2018,2019,2020,and 2021. What is the annual amount to be deposited?
A) $5,548
B) $4,000
C) $3,148
D) $2,270
A) $5,548
B) $4,000
C) $3,148
D) $2,270

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
78
Jackie's parents loaned her $80,000 to fund her college education. Her parents are not charging interest. They desire to be paid one lump sum of $80,000 when Jackie can accumulate that amount. Jackie established a savings plan that earns 8% compounded annually. Her new job promises to pay an annual holiday bonus that will enable her to make equal annual, year-end deposits of $6,400. Approximately how many years will it take Jackie to accumulate the $80,000?
A) 8 years
B) 8.5 years
C) 9 years
D) 12.5 years
A) 8 years
B) 8.5 years
C) 9 years
D) 12.5 years

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
79
You deposit in a fund 10 annual payments of $2,500 each beginning January 1, 2016, with the last deposit being made on January 1, 2025. How much will be in the fund on December 31, 2025, one year after the final payment, if the fund earns interest at 4% compounded annually?
A) $21,088
B) $28,957
C) $30,015
D) $31,216
A) $21,088
B) $28,957
C) $30,015
D) $31,216

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck
80
You would like to deposit a sum of money today that would enable you to withdraw $2,000 a year for ten years. If the interest paid on the amount deposited is 10% compounded annually and if the first withdrawal is made one year from today, the formula you would use to determine the amount of the initial deposit is the
A) present value of a deferred annuity.
B) present value of an annuity due.
C) present value of an ordinary annuity.
D) future value of an ordinary annuity.
A) present value of a deferred annuity.
B) present value of an annuity due.
C) present value of an ordinary annuity.
D) future value of an ordinary annuity.

Unlock Deck
Unlock for access to all 142 flashcards in this deck.
Unlock Deck
k this deck



