Deck 11: Multifactor Analysis of Variance
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/62
Play
Full screen (f)
Deck 11: Multifactor Analysis of Variance
1
In the three-factor effects model, assume that there are 4 levels for factor A, 2 levels for factor B, 3 levels for factor C, and 4 observations for each combination of levels of the three factors. Then, the three-factor interaction sum of squares (SSABC) has df = __________.
6
2
An experiment in which there are p factors, each at two levels, is referred to as a
 factorial experiment.
factorial experiment. 3
In a three-factor experiment, if the levels of factor A are identified with the rows of a two-way table and the levels of B with the columns of the table, then the defining characteristic of a Latin square design is that every level of factor C appears exactly __________ in each row and exactly __________ in each column.
once, once
4
When several factors are to be studied simultaneously, an experiment in which there is at least one observation for every possible combination of levels is referred to as __________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
5
Consider a 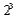 experiment with 2 blocks. The price paid for this blocking is that __________ of the factor effects cannot be estimated.
experiment with 2 blocks. The price paid for this blocking is that __________ of the factor effects cannot be estimated.
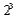 experiment with 2 blocks. The price paid for this blocking is that __________ of the factor effects cannot be estimated.
experiment with 2 blocks. The price paid for this blocking is that __________ of the factor effects cannot be estimated.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
6
The three-factor fixed effects model, with the same number of observations for each combination of levels, I, J, and K of the three factors A, B, and C, respectively, is represented by 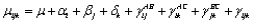 The parameters
The parameters  are called __________, and
are called __________, and 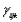 is called a __________, whereas
is called a __________, whereas 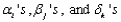 are the __________ parameters.
are the __________ parameters.
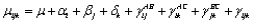 The parameters
The parameters  are called __________, and
are called __________, and 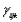 is called a __________, whereas
is called a __________, whereas 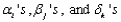 are the __________ parameters.
are the __________ parameters.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
7
The parameters for the fixed effects model with interaction are 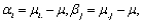 and
and 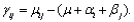 Thus the model is
Thus the model is 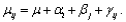 The
The 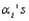 are called the __________ for factor A, whereas the
are called the __________ for factor A, whereas the 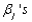 are the __________ for factor B. The
are the __________ for factor B. The 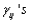 are referred to as the __________ parameters.
are referred to as the __________ parameters.
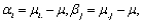 and
and 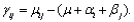 Thus the model is
Thus the model is 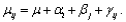 The
The 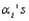 are called the __________ for factor A, whereas the
are called the __________ for factor A, whereas the 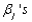 are the __________ for factor B. The
are the __________ for factor B. The 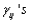 are referred to as the __________ parameters.
are referred to as the __________ parameters.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
8
In two-factor ANOVA, when factor A consists of I levels and factor B consists of J levels, there are __________ different combinations (pairs) of levels of the two factors, each called a __________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
9
In the fixed effects model with interaction, assume that there are I levels of factor A, J levels of Factor J, and K observations (replications) for each of the IJ combinations of levels of the two factors. Then SSE (the error sum of squares) has df = __________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
10
In the three-factor fixed effects model, assume that there are 5 levels of factor A, 4 levels of factor B, 3 levels of factor C, and 2 observations for each combination of levels of the three factors. Then the error sum of squares (SSE) has df = __________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
11
A 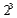 experiment has __________ factors, and each factor has __________ levels.
experiment has __________ factors, and each factor has __________ levels.
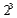 experiment has __________ factors, and each factor has __________ levels.
experiment has __________ factors, and each factor has __________ levels.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
12
In the fixed effects model with interaction, assume that there are I levels of factor A, J levels of factor B, and K observations (replications) for each of the IJ combinations of levels of the two factors. Then SSAB (the interaction sum of squares) has df =__________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
13
In the three-factor fixed effects model, assume that there are 4 levels for each of the three factors A, B, and C, and 3 observations for each combination of levels of the three factors. Then, the two-factor interaction sum of squares for factors B and C (SSBC) has df = __________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
14
In a two-factor experiment where factor A consists of I levels, factor B consists of J levels, and there is only one observation on each of the IJ treatments, SSE has __________ degrees of freedom.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
15
In the fixed effects model with interaction, assume that there are I levels of factor A, J levels of factor B, and K observations (replications) for each of the IJ combinations of levels of the two factors. Then SST (the total sum of squares) has df = __________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
16
In a two-factor experiment where factor A consists of I levels, factor B consists of J levels, and there is only one observation on each of the IJ treatments, SST has __________ degrees of freedom.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
17
A three-factor experiment, with I levels of factor A, J levels of factor B, and C levels of factor C, in which fewer than IJK observations are made is called an __________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
18
In a two-factor experiment where factor A consists of 5 levels, factor B consists of 4 levels, and there is only one observation on each of the 20 treatments, the critical value for testing the null hypothesis that the different levels of factor B have no effect on true average response at significance level .05 is denoted by __________, and is equal to __________.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
19
Assume the existence of I parameters 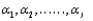 and J parameters
and J parameters 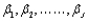 such that
such that 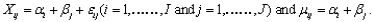 The model specified by the above equations is called an __________ model because each mean response
The model specified by the above equations is called an __________ model because each mean response 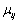 is the __________ of an effect due to factor A at level
is the __________ of an effect due to factor A at level 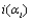 and an effect due to factor B at level
and an effect due to factor B at level 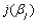 .
.
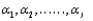 and J parameters
and J parameters 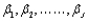 such that
such that 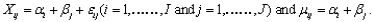 The model specified by the above equations is called an __________ model because each mean response
The model specified by the above equations is called an __________ model because each mean response 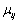 is the __________ of an effect due to factor A at level
is the __________ of an effect due to factor A at level 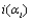 and an effect due to factor B at level
and an effect due to factor B at level 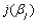 .
.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
20
In two-factor ANOVA, additivity means that the difference in true average responses for any two levels of one of the factors is the same for each level of the other factor. When additivity does not hold, we say that there is __________ between the different levels of the factors.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
21
In a two-factor experiment where factor A consists of 4 levels, factor B consists of 3 levels, and there is only one observation on each of the 12 treatments, which of the following statements are not true?
A) SST has 12 degrees of freedom
B) SSA has 3 degrees of freedom
C) SSB has 2 degrees of freedom
D) SSE has 6 degrees of freedom
E) None of the above statements are correct.
A) SST has 12 degrees of freedom
B) SSA has 3 degrees of freedom
C) SSB has 2 degrees of freedom
D) SSE has 6 degrees of freedom
E) None of the above statements are correct.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
22
To select a half-replicate of a 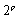 factorial experiment
factorial experiment 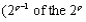 possible treatment conditions), the number of defining effects that must be selected is __________.
possible treatment conditions), the number of defining effects that must be selected is __________.
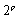 factorial experiment
factorial experiment 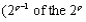 possible treatment conditions), the number of defining effects that must be selected is __________.
possible treatment conditions), the number of defining effects that must be selected is __________.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
23
The primary interest of designing a randomized block experiment is:
A) to reduce the variation among blocks
B) to increase the between-treatments variation to more easily detect differences among the treatment means
C) to reduce the within-treatments variation to more easily detect differences among the treatment means
D) to increase the total sum of squares
E) All of the above statements are true.
A) to reduce the variation among blocks
B) to increase the between-treatments variation to more easily detect differences among the treatment means
C) to reduce the within-treatments variation to more easily detect differences among the treatment means
D) to increase the total sum of squares
E) All of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
24
Which of the following statements are not true?
A) In a two-factor experiment there are I levels of one factor and J levels of the other factor. When there is more than one observation for at least one (i,j) pair of the IJ combinations of levels of the two factors, a valid estimator of the random error variance
Cannot be obtained without assuming additivity.
B) In a two-factor experiment, additivity means that the difference in true average responses for any two levels of one of the factors is the same for each level of the other factor.
C) The parameters for the fixed effects model with interaction are
The effect of factor A at level
The effect of factor B at level j, and
The interaction of factor A at level i and factor B at level j. Thus, the model is
This model is additive if and only if
D) All of the above statements are true.
E) None of the above statements are true.
A) In a two-factor experiment there are I levels of one factor and J levels of the other factor. When there is more than one observation for at least one (i,j) pair of the IJ combinations of levels of the two factors, a valid estimator of the random error variance
Cannot be obtained without assuming additivity.
B) In a two-factor experiment, additivity means that the difference in true average responses for any two levels of one of the factors is the same for each level of the other factor.
C) The parameters for the fixed effects model with interaction are
The effect of factor A at level
The effect of factor B at level j, and
The interaction of factor A at level i and factor B at level j. Thus, the model is
This model is additive if and only if
D) All of the above statements are true.
E) None of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
25
The following equation SST = SSA + SSB +SSC + SSAB + SSAC + SSBC + SSABC + SSE applies to which ANOVA model?
A) One-factor ANOVA
B) Two-factor ANOVA with interaction
C) Three-factor ANOVA with interactions
D) Randomized block design
E) Latin square design
A) One-factor ANOVA
B) Two-factor ANOVA with interaction
C) Three-factor ANOVA with interactions
D) Randomized block design
E) Latin square design

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
26
To select a quarter-replicate of a 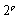 factorial experiment
factorial experiment  possible treatment conditions), the number of defining effects that must be selected is __________.
possible treatment conditions), the number of defining effects that must be selected is __________.
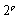 factorial experiment
factorial experiment  possible treatment conditions), the number of defining effects that must be selected is __________.
possible treatment conditions), the number of defining effects that must be selected is __________.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
27
In the three-factor fixed effects model, assume that there are 4 levels of factor A, 2 levels of factor B, 4 levels of factor C, and 3 observations for each combination of levels of the three factors. Then, the number of degrees of freedom for the three-factor interaction sum of squares (SSABC) is
A) 32
B) 10
C) 9
D) 12
E) 13
A) 32
B) 10
C) 9
D) 12
E) 13

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
28
The following equation SST = SSA + SSB +SSAB +SSE applies to which ANOVA model?
A) One-factor ANOVA
B) Two-factor ANOVA with interaction
C) Three-factor ANOVA
D) Randomized block design
E) All of the above
A) One-factor ANOVA
B) Two-factor ANOVA with interaction
C) Three-factor ANOVA
D) Randomized block design
E) All of the above

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
29
In a two-factor ANOVA problem, there are 4 levels of factor A, 5 levels of factor B, and 2 observations (replications) for each combination of levels of the two factors. Then, the number of treatments in this experiment is
A) 40
B) 11
C) 10
D) 20
E) 8
A) 40
B) 11
C) 10
D) 20
E) 8

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
30
Which of the following statements are not true regarding the model where
A)
Is the true grand mean; that is, the mean response averaged over all levels of both factors A and B.
B)
Is the effect of Factor A at level i.
C)
Is the effect of Factor B at level j.
D)
Are assumed independent and normally distributed with mean 0 and variance 1.
E) All of the above statements are true.
A)
Is the true grand mean; that is, the mean response averaged over all levels of both factors A and B.
B)
Is the effect of Factor A at level i.
C)
Is the effect of Factor B at level j.
D)
Are assumed independent and normally distributed with mean 0 and variance 1.
E) All of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
31
In the fixed effects model with interaction, assume that there are 4 levels of factor A, 3 levels of factor B, and 3 observations for each of the 12 combinations of levels of the two factors. Then, the number of degrees of freedom for the error sum of squares (SSE) is
A) 36
B) 35
C) 24
D) 10
E) 9
A) 36
B) 35
C) 24
D) 10
E) 9

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
32
Which of the following statements are true regarding a two-factor experiment?
A) In some experiments, the levels of either factor may have been chosen from a large population of possible levels, so that the effects contributed by the factor are random rather than fixed.
B) If both factors contribute random effects, the model is referred to as a random effects model.
C) If one factor is fixed, and the other contributes random effects, a mixed effects model results.
D) If both factors are fixed, the model is referred to as a fixed effects model.
E) All of the above statements are true.
A) In some experiments, the levels of either factor may have been chosen from a large population of possible levels, so that the effects contributed by the factor are random rather than fixed.
B) If both factors contribute random effects, the model is referred to as a random effects model.
C) If one factor is fixed, and the other contributes random effects, a mixed effects model results.
D) If both factors are fixed, the model is referred to as a fixed effects model.
E) All of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
33
Consider a 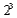 experiment with four blocks. In this case, __________ factor effects are confounded with the blocks.
experiment with four blocks. In this case, __________ factor effects are confounded with the blocks.
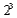 experiment with four blocks. In this case, __________ factor effects are confounded with the blocks.
experiment with four blocks. In this case, __________ factor effects are confounded with the blocks.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
34
In the fixed effects model with interaction, assume that there are 5 levels of factor A, 4 levels of factor B, and 3 observations (replications) for each of the 20 combinations of levels of the two factors. Then the number of degrees of freedom of the interaction sum of squares (SSAB) is
A) 60
B) 20
C) 15
D) 12
E) 59
A) 60
B) 20
C) 15
D) 12
E) 59

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
35
In the randomized block design for ANOVA where the single factor of primary interest has I levels, and b blocks are created to control for extraneous variability in experimental units or subjects, the number of degrees of freedom for SSE (error sum of squares) is given by
A) Ib-1
B) (I-1) (b-1)
C) I-1
D) b-1
E) I+b-1
A) Ib-1
B) (I-1) (b-1)
C) I-1
D) b-1
E) I+b-1

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
36
Which of the following statements are not true?
A) The model specified by
Is called an additive model.
B) The model
Are assumed independent, normally distributed with mean 0 and common variance
Is an additive model in which the parameters are uniquely determined.
C) In two-way ANOVA, when the model is additive, additivity means that the difference in mean responses for two levels of one of the factors is the same for all levels of the other factor.
D) All of the above statements are true.
E) None of the above statements are true.
A) The model specified by
Is called an additive model.
B) The model
Are assumed independent, normally distributed with mean 0 and common variance
Is an additive model in which the parameters are uniquely determined.
C) In two-way ANOVA, when the model is additive, additivity means that the difference in mean responses for two levels of one of the factors is the same for all levels of the other factor.
D) All of the above statements are true.
E) None of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
37
A two-factor experiment where factor A consists of I levels, factor B consists of J levels, and there is only one observation on each of the IJ treatments, can be represented by the model . Which of the following is the correct form in testing the null hypothesis that the different levels of factor A have no effect on true average response?
A)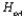 :
:
B) 11edff9d_a02c_75df_98d9_5f65b54a33be_TB3498_11
C) 11edff9d_a02c_75df_98d9_5f65b54a33be_TB3498_11
D)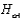
E) None of the above answers are correct.
A)
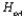 :
: B) 11edff9d_a02c_75df_98d9_5f65b54a33be_TB3498_11
C) 11edff9d_a02c_75df_98d9_5f65b54a33be_TB3498_11
D)
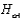
E) None of the above answers are correct.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
38
In the three-factor fixed effects model, assume that there are 3 levels for each of the three factors A, B, and C, and 2 observations for each combination of levels of the three factors. Then the number of degrees of freedom for the error sum of squares (SSE) is
A) 54
B) 27
C) 11
D) 18
E) 16
A) 54
B) 27
C) 11
D) 18
E) 16

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
39
In the fixed effects model with interaction, assume that there are 3 levels of factor A, 2 levels of factor B, and 3 observations for each of the six combinations of levels of the two factors. Then the critical value for testing the null hypothesis of no interaction between the levels of the two factors at the .05 significance level is
A) 3.49
B) 3.89
C) 3.00
D) 3.55
E) 3.11
A) 3.49
B) 3.89
C) 3.00
D) 3.55
E) 3.11

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
40
The three-factor fixed effects model, with the same number of observations for each combination of levels I, J, and K of the three factors A, B, and C, respectively, is represented by 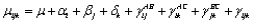 . Which of the following statements are true?
. Which of the following statements are true?
A) The restrictions necessary to obtain uniquely defined parameters are that the sum over any subscript of any parameter on the right-hand side of the above equation equals 0.
B) The parameters
Are called the main effects of the factors A, B, and C, respectively.
C) The parameters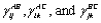
Are called two-factor interactions.
D) The parameter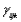
Is called a three-factor interaction.
E) All of the above statements are true.
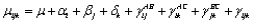 . Which of the following statements are true?
. Which of the following statements are true?A) The restrictions necessary to obtain uniquely defined parameters are that the sum over any subscript of any parameter on the right-hand side of the above equation equals 0.
B) The parameters
Are called the main effects of the factors A, B, and C, respectively.
C) The parameters
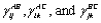
Are called two-factor interactions.
D) The parameter
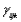
Is called a three-factor interaction.
E) All of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
41
The accompanying data was obtained in an experiment to investigate whether compressive strength of concrete cylinders depends of the type of capping material used or variability in different batches. Each number is a cell total

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
42
The strength of concrete used in commercial construction tends to vary from one batch to another. Consequently, small test cylinders of concrete sampled from a batch are "cured" for periods up to about 28 days in temperature- and moisture-controlled environments before strength measurements are made. Concrete is then "bought and sold on the basis of strength test cylinders". The accompanying data resulted from an experiment carried out to compare three different curing methods with respect to compressive strength (MPa). Analyze this data. 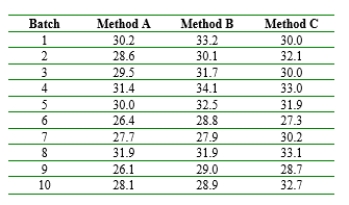


Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
43
A four-factor ANOVA experiment was carried out to investigate the effects of fabric (A), type of exposure (B), level of exposure (C), and fabric direction (D) on extent of color change in exposed fabric as measured by a spectrocolorimeter. Two observations were made for each of the three fabrics, two types, three levels, and two directions, resulting in MSA = 2207.329, MSB = 47.255, MSC = 491.783, MSD = .044, MSAB = 15.303, MSAC = 275.446, MSAD = .470, MSBC = 2.141, MSBD = .280, MSE = .977, and MST = 93.621 ("Accelerated Weathering of Marine Fabrics," J. Testing and Eval.,. 1992: 139-143). Assuming fixed effects for all factors, carry out an analysis of variance using 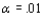 for all tests and summarize your conclusions.
for all tests and summarize your conclusions.
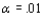 for all tests and summarize your conclusions.
for all tests and summarize your conclusions.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
44
In an experiment to see whether the amount of coverage of light-blue interior paint depends either on the brand of paint or on the brand of roller used, 1 gallon of each of four brands of paint was applied using each of three brands of roller, resulting in the following data (number of square feet covered). 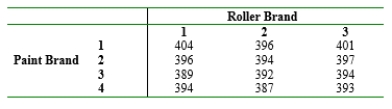
a. Construct the ANOVA table.
b. State and test hypotheses appropriate for deciding whether paint has any effect on coverage. Use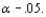
c. Repeat part (b) for brand of roller.
d. Use Tukey's method to identify significant differences among brands. Is there one brand that seems clearly preferable to the others?

a. Construct the ANOVA table.
b. State and test hypotheses appropriate for deciding whether paint has any effect on coverage. Use
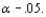
c. Repeat part (b) for brand of roller.
d. Use Tukey's method to identify significant differences among brands. Is there one brand that seems clearly preferable to the others?

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
45
Which of the following statements are not true?
A) If the two three-factor interactions BCD and CDE are chosen for confounding, then their generalized interaction is BE.
B) If the two three-factor interactions ABC and CDE are chosen for confounding, then their generalized interaction is ABCDE.
C) When the number p of factors is large, a single replicate of a
Experiment can be expensive and time consuming.
D) All of the above statements are true.
E) None of the above statements are true.
A) If the two three-factor interactions BCD and CDE are chosen for confounding, then their generalized interaction is BE.
B) If the two three-factor interactions ABC and CDE are chosen for confounding, then their generalized interaction is ABCDE.
C) When the number p of factors is large, a single replicate of a
Experiment can be expensive and time consuming.
D) All of the above statements are true.
E) None of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
46
The accompanying data resulted from a  experiment with three replications per combination of treatments designed to study the effects of concentration of detergent (A), concentration of sodium carbonate (B), and concentration of sodium carboxymethyl cellulose (C0 on cleaning ability of a solution in washing tests (a larger number indicates better cleaning ability than a smaller number).
experiment with three replications per combination of treatments designed to study the effects of concentration of detergent (A), concentration of sodium carbonate (B), and concentration of sodium carboxymethyl cellulose (C0 on cleaning ability of a solution in washing tests (a larger number indicates better cleaning ability than a smaller number). 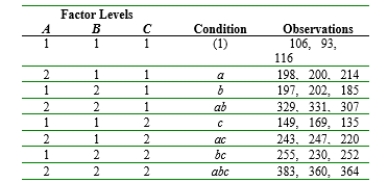
a. After obtaining cell totals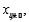
compute estimates of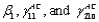
b. Use the cell totals along with Yate's method to compute the effect contrasts and sums of squares. Then construct an ANOVA table and test all appropriate hypotheses using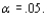
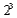 experiment with three replications per combination of treatments designed to study the effects of concentration of detergent (A), concentration of sodium carbonate (B), and concentration of sodium carboxymethyl cellulose (C0 on cleaning ability of a solution in washing tests (a larger number indicates better cleaning ability than a smaller number).
experiment with three replications per combination of treatments designed to study the effects of concentration of detergent (A), concentration of sodium carbonate (B), and concentration of sodium carboxymethyl cellulose (C0 on cleaning ability of a solution in washing tests (a larger number indicates better cleaning ability than a smaller number). 
a. After obtaining cell totals
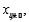
compute estimates of
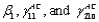
b. Use the cell totals along with Yate's method to compute the effect contrasts and sums of squares. Then construct an ANOVA table and test all appropriate hypotheses using
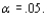

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
47
Which of the following statements are not true?
A) An experiment in which there are p factors, each at two levels, is referred to as a
Factorial experiment.
B) A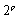
Factorial experiment provides a simple setting for introducing the important concepts of confounding and fractional replications.
C) A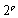
Experiment, with four factors A, B, C, and D, has 16 different experimental conditions.
D) All of the above statements are true.
E) None of the above statements are true.
A) An experiment in which there are p factors, each at two levels, is referred to as a
Factorial experiment.
B) A
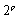
Factorial experiment provides a simple setting for introducing the important concepts of confounding and fractional replications.
C) A
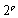
Experiment, with four factors A, B, C, and D, has 16 different experimental conditions.
D) All of the above statements are true.
E) None of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
48
The current (in 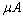 ) necessary to produce a certain level of brightness of a television tube was measured for two different types of glass and three different types of phosphor, resulting in the accompanying data:
) necessary to produce a certain level of brightness of a television tube was measured for two different types of glass and three different types of phosphor, resulting in the accompanying data:  Assuming that both factors are fixed, test
Assuming that both factors are fixed, test 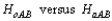 at level .01. Then if
at level .01. Then if 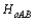 cannot be rejected, test the two sets of main effect hypotheses.
cannot be rejected, test the two sets of main effect hypotheses.
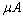 ) necessary to produce a certain level of brightness of a television tube was measured for two different types of glass and three different types of phosphor, resulting in the accompanying data:
) necessary to produce a certain level of brightness of a television tube was measured for two different types of glass and three different types of phosphor, resulting in the accompanying data:  Assuming that both factors are fixed, test
Assuming that both factors are fixed, test 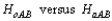 at level .01. Then if
at level .01. Then if 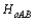 cannot be rejected, test the two sets of main effect hypotheses.
cannot be rejected, test the two sets of main effect hypotheses.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
49
Which of the following statements are true?
A) Blocking is always effective in reducing variation associated with extraneous sources.
B) It is often not possible to carry out all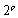
Experimental conditions of a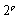
Factorial experiment in a homogeneous experimental environment.
C) When the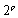
Experimental conditions are placed in
Homogeneous blocks (r<p), the price paid for this blocking is that
Of the factor effects cannot be estimated.
D) All of the above statements are true.
E) None of the above statements are true.
A) Blocking is always effective in reducing variation associated with extraneous sources.
B) It is often not possible to carry out all
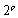
Experimental conditions of a
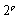
Factorial experiment in a homogeneous experimental environment.
C) When the
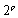
Experimental conditions are placed in
Homogeneous blocks (r<p), the price paid for this blocking is that
Of the factor effects cannot be estimated.
D) All of the above statements are true.
E) None of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
50
The following equation SST = SSA + SSB + SSC + SSE applies to which ANOVA model?
A) One-factor ANOVA
B) Two-factor ANOVA with interaction
C) Three-factor ANOVA with interactions
D) Latin square design
E) Randomized block design
A) One-factor ANOVA
B) Two-factor ANOVA with interaction
C) Three-factor ANOVA with interactions
D) Latin square design
E) Randomized block design

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
51
Which of the following statements are true?
A) For experimental situations with more than three factors, there are often no replications, so sums of squares associated with nonconfounded higher-order interactions are usually pooled to obtain an error sum of squares that can be used in the denominators of the various F statistics.
B) One replicate of a
Factorial experiment involves an observation for each of the 64 different experimental conditions.
C) If an experimenter decides to include only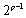
Of the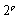
Possible conditions in the experiment; this is usually called a half-replicate.
D) The first step in selecting half-replicate is to select a defining effect as the nonestimable effect.
E) All of the above statements are true.
A) For experimental situations with more than three factors, there are often no replications, so sums of squares associated with nonconfounded higher-order interactions are usually pooled to obtain an error sum of squares that can be used in the denominators of the various F statistics.
B) One replicate of a
Factorial experiment involves an observation for each of the 64 different experimental conditions.
C) If an experimenter decides to include only
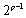
Of the
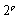
Possible conditions in the experiment; this is usually called a half-replicate.
D) The first step in selecting half-replicate is to select a defining effect as the nonestimable effect.
E) All of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
52
A data from an experiment to assess the effects of vibration (A), temperature cycling (B), altitude cycling (C), and temperature for altitude cycling and firing (D) on thrust duration are shown below. Use the Yates method to obtain sums of squares and the ANOVA table. Then assume that three- and four-factor interactions are absent, pool the corresponding sums of squares to obtain an estimate of 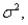 and test all appropriated hypotheses at level .05.
and test all appropriated hypotheses at level .05. 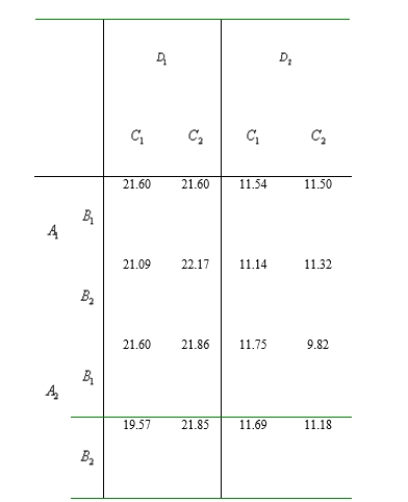
 and test all appropriated hypotheses at level .05.
and test all appropriated hypotheses at level .05. 

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
53
The accompanying data table gives observations on total acidity of coal samples of three different types, with determinations made using three different concentrations of ethanolic NaOH.
Type of Coal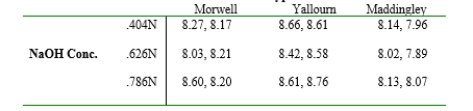 Additionally,
Additionally, 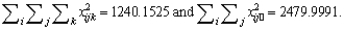
a. Assuming both effects to be fixed, construct an ANOVA table, test for the presence of
ANOVA table, test for the presence of interaction, and then test for the presence of main effects for each factor (all using level .01).
b. Use Tukey's procedure to identify significant differences among the types of coal.
Type of Coal
 Additionally,
Additionally, 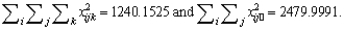
a. Assuming both effects to be fixed, construct an ANOVA table, test for the presence of
ANOVA table, test for the presence of interaction, and then test for the presence of main effects for each factor (all using level .01).
b. Use Tukey's procedure to identify significant differences among the types of coal.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
54
The number of miles useful tread wear (in 1000's) was determined for tires of five different makes of subcompact car (factor A, with I = 5) in combination with each of four different brands of radial tires (factor B, with J = 4), resulting in IJ = 20 observations. The values SSA = 30, SSB = 45, and SSE = 60 were then computed. Assume that an additive model is appropriate.
a. Test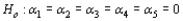
(no differences in true average tire lifetime due to makes of cars) versus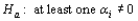
using a level .05 test.
b.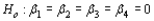
(no differences in "true" average tire lifetime due to brands of tires) versus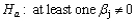
using a level .05 test.
a. Test
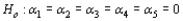
(no differences in true average tire lifetime due to makes of cars) versus
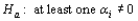
using a level .05 test.
b.
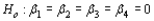
(no differences in "true" average tire lifetime due to brands of tires) versus
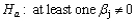
using a level .05 test.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
55
Because of potential variability in aging due to different castings and segments on the castings, a Latin square design with N = 7 was used to investigate the effect of heat treatment on aging. With A = castings, B = segments, C = heat treatments, summary statistics include 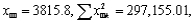 and
and 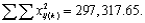 Obtain the ANOVA table and test at level .05 the hypothesis that heat treatment has no effect on aging.
Obtain the ANOVA table and test at level .05 the hypothesis that heat treatment has no effect on aging.
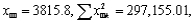 and
and 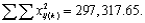 Obtain the ANOVA table and test at level .05 the hypothesis that heat treatment has no effect on aging.
Obtain the ANOVA table and test at level .05 the hypothesis that heat treatment has no effect on aging.
Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
56
A particular county in Indiana employs three assessors who are responsible for determining the value of residential property in the county. To see whether these assessors differ systemically in their assessments, five houses are selected, and each assessor is asked to determine the market value of each house. With factor A denoting assessors (I = 3) and factor B denoting houses (J=5), suppose SSA = 12, SSB = 110, and SSE = 26.
a. Test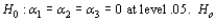
states that there are no systemic differences among assessors).
b. Explain why a randomized block experiment with only 5 houses was used rather than a one-way ANOVA experiment involving a total of 15 different houses with each assessor asked to assess 5 different houses (a different group of 5 for each assessor).
a. Test
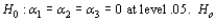
states that there are no systemic differences among assessors).
b. Explain why a randomized block experiment with only 5 houses was used rather than a one-way ANOVA experiment involving a total of 15 different houses with each assessor asked to assess 5 different houses (a different group of 5 for each assessor).

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
57
The output of a continuous extruding machine that coats steel pipe with plastic was studied as a function of the thermostat temperature profile (A, at three levels), type of plastic (B, at three levels), and the speed of the rotating screw that forces the plastic through a tube-forming die (C, at three levels). There were two replications (L = 2) at each combination of levels of the factors, yielding a total of 54 observations on output. The sums of squares were SSA = 14,144.44, SSB = 5511.27, SSC = 244,696.39, SSAB = 1069.62, SSAC = 62.67, SSBC = 331.67, SSE = 3127.50, and SST = 270,024.33.
a. Construct the ANOVA table.
b. Use appropriate F tests to show that none of the F ratios for two- or three-factor interactions is
at level .05.
c. Which main effects appear significant?
d. With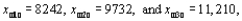
use Tukey's procedure to identify significant
differences among the levels of factor C.
a. Construct the ANOVA table.
b. Use appropriate F tests to show that none of the F ratios for two- or three-factor interactions is
at level .05.
c. Which main effects appear significant?
d. With
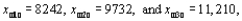
use Tukey's procedure to identify significant
differences among the levels of factor C.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
58
The following summary quantities were computed from an experiment involving four levels of nitrogen (A), two times of planting (B), and two levels of potassium (C). Only one observation (N content, in percentage, of corn grain) was made for each of the 16 combinations of levels.
SSA = .22625 SSB = .000025 SSC = .0036 SSAB = .004325
SSAC = .00065 SSBC = .000625 SST = .2384.
a. Construct the ANOVA table.
b. Assume that there are no three-way interaction effects, so that MSABC is a valid estimate of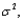
and test at level .05 for interaction and main effects.
c. The nitrogen averages are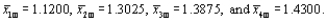
Use Tukey's method to examine differences in percentage N among the nitrogen levels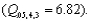
SSA = .22625 SSB = .000025 SSC = .0036 SSAB = .004325
SSAC = .00065 SSBC = .000625 SST = .2384.
a. Construct the ANOVA table.
b. Assume that there are no three-way interaction effects, so that MSABC is a valid estimate of
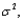
and test at level .05 for interaction and main effects.
c. The nitrogen averages are
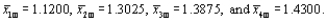
Use Tukey's method to examine differences in percentage N among the nitrogen levels
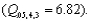

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
59
Which of the following statements are not true?
A) Tukey's multiple comparison procedure can be used in two-factor ANOVA but not in three-factor (or more) ANOVA.
B) When several factors are to be studied simultaneously, an experiment in which there is at least one observation for every possible combination of levels is referred to as complete layout.
C) A three-factor experiment, with I levels of factor A, J levels of factor B, and K levels of factor C, in which fewer than IJK observations are made is called an incomplete layout.
D) There are some incomplete layouts in which the pattern of combinations of factors is such that the analysis is straightforward. One such three-factor design is called a Latin square.
E) All of the above statements are true.
A) Tukey's multiple comparison procedure can be used in two-factor ANOVA but not in three-factor (or more) ANOVA.
B) When several factors are to be studied simultaneously, an experiment in which there is at least one observation for every possible combination of levels is referred to as complete layout.
C) A three-factor experiment, with I levels of factor A, J levels of factor B, and K levels of factor C, in which fewer than IJK observations are made is called an incomplete layout.
D) There are some incomplete layouts in which the pattern of combinations of factors is such that the analysis is straightforward. One such three-factor design is called a Latin square.
E) All of the above statements are true.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
60
In an experiment to assess the effects of curing time (factor A) and type of mix (factor B) on the compressive strength of hardened cement cubes, three different curing times were used in combination with four different mixes, with three observations obtained for each of the 12 curing time-mix combinations. The resulting sums of squares were computed to be SSA = 30,763.0, SSB = 34,185.6, SSE = 97,436.8, and SST = 205,966.6.
a. Construct an ANOVA table.
b. Test at level .05 the null hypothesis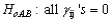
(no interaction of factors) against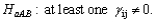
c. Test at level .05 the null hypothesis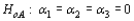
(factor A main effects are absent) against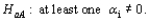
d. Test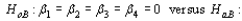
at least one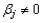
using a level .05 test.
e. The values of the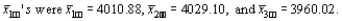
Use Tukey's procedure to investigate significant differences among the three curing times.
a. Construct an ANOVA table.
b. Test at level .05 the null hypothesis
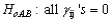
(no interaction of factors) against
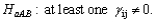
c. Test at level .05 the null hypothesis
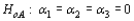
(factor A main effects are absent) against
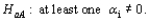
d. Test
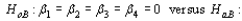
at least one
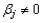
using a level .05 test.
e. The values of the
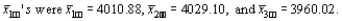
Use Tukey's procedure to investigate significant differences among the three curing times.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
61
Answer the following questions:
a. In a![Answer the following questions: a. In a experiment, suppose two blocks are to be used, and it is decided to confound the ABCD interaction with the block effect. Which treatments should be carried out in the first block [containing the treatment (1)], and which treatments are allocated to the second block? b. In an experiment to investigate niacin retention in vegetables as a function of cooking temperature (A), sieve size (B), type of processing (C), and cooking time (D), each factor was held at two levels. Two blocks were used, with the allocation of blocks as given in part (a) to confound only the ABCD interaction with blocks. Use Yate's procedure to obtain the ANOVA table for the accompanying data. c. Assume that all three-way interaction effects are absent, so that the associated sums of squares Can be combined to yield an estimate of and carry out all appropriate test at level .05.](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3eb3_fb81_9431_e3da1a6f22ff_TB3498_11.jpg)
experiment, suppose two blocks are to be used, and it is decided to confound the ABCD interaction with the block effect. Which treatments should be carried out in the first block [containing the treatment (1)], and which treatments are allocated to the second block?
b. In an experiment to investigate niacin retention in vegetables as a function of cooking temperature (A), sieve size (B), type of processing (C), and cooking time (D), each factor was held at two levels. Two blocks were used, with the allocation of blocks as given in part (a) to confound only the ABCD interaction with blocks. Use Yate's procedure to obtain the ANOVA table for the accompanying data.![Answer the following questions: a. In a experiment, suppose two blocks are to be used, and it is decided to confound the ABCD interaction with the block effect. Which treatments should be carried out in the first block [containing the treatment (1)], and which treatments are allocated to the second block? b. In an experiment to investigate niacin retention in vegetables as a function of cooking temperature (A), sieve size (B), type of processing (C), and cooking time (D), each factor was held at two levels. Two blocks were used, with the allocation of blocks as given in part (a) to confound only the ABCD interaction with blocks. Use Yate's procedure to obtain the ANOVA table for the accompanying data. c. Assume that all three-way interaction effects are absent, so that the associated sums of squares Can be combined to yield an estimate of and carry out all appropriate test at level .05.](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3eb3_fb82_9431_45cf96f48acd_TB3498_00.jpg)
c. Assume that all three-way interaction effects are absent, so that the associated sums of squares
Can be combined to yield an estimate of![Answer the following questions: a. In a experiment, suppose two blocks are to be used, and it is decided to confound the ABCD interaction with the block effect. Which treatments should be carried out in the first block [containing the treatment (1)], and which treatments are allocated to the second block? b. In an experiment to investigate niacin retention in vegetables as a function of cooking temperature (A), sieve size (B), type of processing (C), and cooking time (D), each factor was held at two levels. Two blocks were used, with the allocation of blocks as given in part (a) to confound only the ABCD interaction with blocks. Use Yate's procedure to obtain the ANOVA table for the accompanying data. c. Assume that all three-way interaction effects are absent, so that the associated sums of squares Can be combined to yield an estimate of and carry out all appropriate test at level .05.](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3eb3_fb83_9431_b96d36c8793b_TB3498_11.jpg)
and carry out all appropriate test at level .05.
a. In a
![Answer the following questions: a. In a experiment, suppose two blocks are to be used, and it is decided to confound the ABCD interaction with the block effect. Which treatments should be carried out in the first block [containing the treatment (1)], and which treatments are allocated to the second block? b. In an experiment to investigate niacin retention in vegetables as a function of cooking temperature (A), sieve size (B), type of processing (C), and cooking time (D), each factor was held at two levels. Two blocks were used, with the allocation of blocks as given in part (a) to confound only the ABCD interaction with blocks. Use Yate's procedure to obtain the ANOVA table for the accompanying data. c. Assume that all three-way interaction effects are absent, so that the associated sums of squares Can be combined to yield an estimate of and carry out all appropriate test at level .05.](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3eb3_fb81_9431_e3da1a6f22ff_TB3498_11.jpg)
experiment, suppose two blocks are to be used, and it is decided to confound the ABCD interaction with the block effect. Which treatments should be carried out in the first block [containing the treatment (1)], and which treatments are allocated to the second block?
b. In an experiment to investigate niacin retention in vegetables as a function of cooking temperature (A), sieve size (B), type of processing (C), and cooking time (D), each factor was held at two levels. Two blocks were used, with the allocation of blocks as given in part (a) to confound only the ABCD interaction with blocks. Use Yate's procedure to obtain the ANOVA table for the accompanying data.
![Answer the following questions: a. In a experiment, suppose two blocks are to be used, and it is decided to confound the ABCD interaction with the block effect. Which treatments should be carried out in the first block [containing the treatment (1)], and which treatments are allocated to the second block? b. In an experiment to investigate niacin retention in vegetables as a function of cooking temperature (A), sieve size (B), type of processing (C), and cooking time (D), each factor was held at two levels. Two blocks were used, with the allocation of blocks as given in part (a) to confound only the ABCD interaction with blocks. Use Yate's procedure to obtain the ANOVA table for the accompanying data. c. Assume that all three-way interaction effects are absent, so that the associated sums of squares Can be combined to yield an estimate of and carry out all appropriate test at level .05.](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3eb3_fb82_9431_45cf96f48acd_TB3498_00.jpg)
c. Assume that all three-way interaction effects are absent, so that the associated sums of squares
Can be combined to yield an estimate of
![Answer the following questions: a. In a experiment, suppose two blocks are to be used, and it is decided to confound the ABCD interaction with the block effect. Which treatments should be carried out in the first block [containing the treatment (1)], and which treatments are allocated to the second block? b. In an experiment to investigate niacin retention in vegetables as a function of cooking temperature (A), sieve size (B), type of processing (C), and cooking time (D), each factor was held at two levels. Two blocks were used, with the allocation of blocks as given in part (a) to confound only the ABCD interaction with blocks. Use Yate's procedure to obtain the ANOVA table for the accompanying data. c. Assume that all three-way interaction effects are absent, so that the associated sums of squares Can be combined to yield an estimate of and carry out all appropriate test at level .05.](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3eb3_fb83_9431_b96d36c8793b_TB3498_11.jpg)
and carry out all appropriate test at level .05.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck
62
In an experiment involving four factors (A,B,C, and D) and four blocks show that at least one main effect or two-factor interaction effect must be confounded with the block effect.

Unlock Deck
Unlock for access to all 62 flashcards in this deck.
Unlock Deck
k this deck



