Deck 8: Introduction to Probability
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/178
Play
Full screen (f)
Deck 8: Introduction to Probability
1
An urn contains three red R balls numbered 1, 2 and 3, four white W balls numbered 4, 5, 6 and 7, and three black B balls numbered 8, 9 and 10 A ball is drawn from the urn. What is the probability that it is red and odd-numbered O?
A)Pr(R and O) =
B)Pr(R and O) =
C)Pr(R and O) =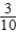
D)Pr(R and O) =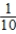
E)Pr(R and O) =
A)Pr(R and O) =

B)Pr(R and O) =

C)Pr(R and O) =
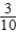
D)Pr(R and O) =
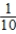
E)Pr(R and O) =

Pr(R and O) = 

2
An ordinary die is tossed. What is the probability of getting a 6 or a 5?
A)Pr(3 or 4) =
B)Pr(3 or 4) =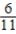
C)Pr(3 or 4) =
D)Pr(3 or 4) =
E)Pr(3 or 4) = 1
A)Pr(3 or 4) =

B)Pr(3 or 4) =
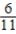
C)Pr(3 or 4) =

D)Pr(3 or 4) =

E)Pr(3 or 4) = 1
Pr(3 or 4) = 

3
In a sales promotion, a clothing store gives its customers a chance to draw a ticket from a box that contains a discount on their next purchase. The box contains 9000 tickets giving a 10% discount, 900 giving a 30% discount, 450 giving a 50% discount, and 4 giving a 100% discount. What is the probability that a given customer will randomly draw a ticket giving a 50% discount?
A)Pr(50%) =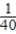
B)Pr(50%) =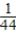
C)Pr(50%) =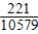
D)Pr(50%) =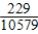
E)Pr(50%) =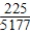
A)Pr(50%) =
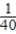
B)Pr(50%) =
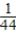
C)Pr(50%) =
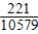
D)Pr(50%) =
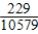
E)Pr(50%) =
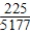
Pr(50%) = 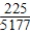
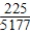
4
One ball is drawn at random from a bag containing 17 red R balls and 5 white W balls. What is the probability that the ball is green G?
A)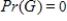
B)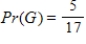
C)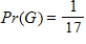
D)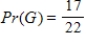
E)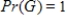
A)
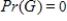
B)
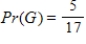
C)
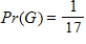
D)
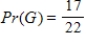
E)
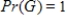

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
5
Fifty-seven percent of marijuana use among youth occurs in the inner cities. If an instance of such marijuana use is chosen at random, what is the probability, rounded to two decimal places, that the use occurs in an inner city?
A)Pr(Inner city) = 0.64
B)Pr(Inner city) = 0.57
C)Pr(Inner city) = 0.36
D)Pr(Inner city) = 0.43
E)Pr(Inner city) = 0.5
A)Pr(Inner city) = 0.64
B)Pr(Inner city) = 0.57
C)Pr(Inner city) = 0.36
D)Pr(Inner city) = 0.43
E)Pr(Inner city) = 0.5

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
6
An urn contains three red R balls numbered 1, 2 and 3, four white W balls numbered 4, 5, 6 and 7, and three black B balls numbered 8, 9 and 10. A ball is drawn from the urn. What is the probability that it is red?
A)P(R) =
B)P(R) =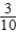
C)P(R) =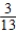
D)P(R) =
E)P(R) =
A)P(R) =

B)P(R) =
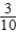
C)P(R) =
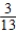
D)P(R) =

E)P(R) =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
7
An urn contains two red R balls numbered 1 and 2, five white W balls numbered 3, 4, 5, 6 and 7, and three black B balls numbered 8, 9 and 10. A ball is drawn from the urn. What is the probability that it is red or odd-numbered O?
A)Pr(R or O) =
B)Pr(R or O) =
C)Pr(R or O) =
D)Pr(R or O) =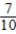
E)Pr(R or O) =
A)Pr(R or O) =

B)Pr(R or O) =

C)Pr(R or O) =

D)Pr(R or O) =
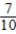
E)Pr(R or O) =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
8
Suppose a die is tossed 1,000 times and a 1 comes up 667 times. Find the empirical probability for a 1 to occur. On the basis of a comparison of the empirical probability and the theoretical probability, do you think the die is fair or biased?
A)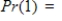
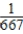
The die is biased since Pr(1) should be about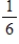
)
B)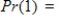
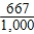
The die is biased since Pr(1) should be about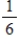
)
C)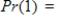
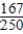
The die is biased since Pr(1) should be about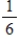
)
D)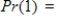
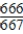
The die is fair since Pr(1) should be about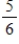
)
E)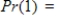
1
The die is fair since Pr(1) should be about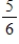
)
A)
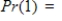
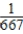
The die is biased since Pr(1) should be about
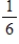
)
B)
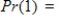
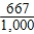
The die is biased since Pr(1) should be about
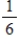
)
C)
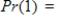
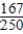
The die is biased since Pr(1) should be about
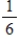
)
D)
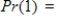
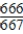
The die is fair since Pr(1) should be about
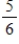
)
E)
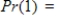
1
The die is fair since Pr(1) should be about
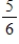
)

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
9
The following table gives the average number of voters in each of three political parties during the last 12 years, along with the average number that voted in presidential elections during this period. Use these data to find the probability, rounded to two decimal places, that a person selected at random from the registered Democratic voters will vote in the next election. 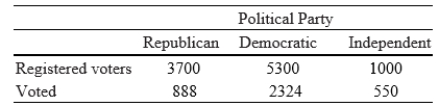
A)Pr(Democratic will vote) = 0.55
B)Pr(Democratic will vote) = 0.44
C)Pr(Democratic will vote) = 0.24
D)Pr(Democratic will vote) = 0.56
E)Pr(Democratic will vote) = 0.45

A)Pr(Democratic will vote) = 0.55
B)Pr(Democratic will vote) = 0.44
C)Pr(Democratic will vote) = 0.24
D)Pr(Democratic will vote) = 0.56
E)Pr(Democratic will vote) = 0.45

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
10
One ball is drawn at random from a bag containing 17 red R balls and 2 white W balls. What is the probability that the ball is red?
A)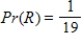
B)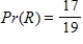
C)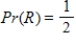
D)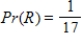
E)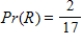
A)
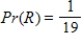
B)
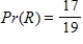
C)
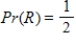
D)
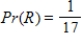
E)
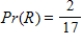

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
11
An urn contains five red balls numbered 1, 2, 3, 4 and 5, four white balls numbered 6, 7, 8 and 9, and three black balls numbered 10, 11 and 12. A ball is drawn from the urn. What is the probability that it is an odd-numbered ball O?
A)Pr(O) =
B)Pr(O) =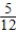
C)Pr(O) =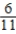
D)Pr(O) =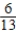
E)Pr(O) =
A)Pr(O) =

B)Pr(O) =
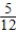
C)Pr(O) =
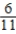
D)Pr(O) =
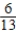
E)Pr(O) =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
12
An urn contains five red R balls numbered 1, 2, 3, 4 and 5, four white W balls numbered 6, 7, 8 and 9, and three black B balls numbered 10, 11 and 12. A ball is drawn from the urn. What is the probability that it is not black?
A)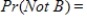

B)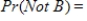

C)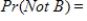
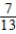
D)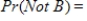
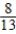
E)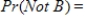

A)
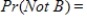

B)
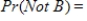

C)
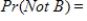
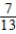
D)
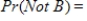
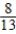
E)
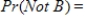


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
13
Suppose a fair coin is tossed 6 times. What is the probability of flipping exactly 2 heads?
A)Pr(2 heads) =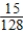
B)Pr(2 heads) =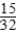
C)Pr(2 heads) =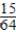
D)Pr(2 heads) =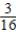
E)Pr(2 heads) =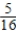
A)Pr(2 heads) =
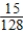
B)Pr(2 heads) =
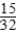
C)Pr(2 heads) =
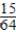
D)Pr(2 heads) =
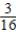
E)Pr(2 heads) =
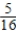

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
14
From a deck of 52 ordinary playing cards, one card is drawn. What is the probability that it is a spade(S)?
A)Pr(S) =
B)Pr(S) =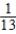
C)Pr(S) =
D)Pr(S) =
E)Pr(S) =
A)Pr(S) =

B)Pr(S) =
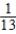
C)Pr(S) =

D)Pr(S) =

E)Pr(S) =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
15
A dry cleaning firm has 6 employees: 4 women and 2 men. 2 of the women and 1 of the men are 42 years old or older. The remainder are over 20 years of age and under 42. If a person is chosen at random from this firm, what is the probability that the person is under 42 years of age?
A)Pr(under 42) =
B)Pr(under 42) =
C)Pr(under 42) =
D)Pr(under 42) =
E)Pr(under 42) =
A)Pr(under 42) =

B)Pr(under 42) =

C)Pr(under 42) =

D)Pr(under 42) =

E)Pr(under 42) =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
16
If the odds that a particular horse will win a race are 15 : 9, what is the probability that the horse will win the race?
A)
B)
C)
D)
E)
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
17
If you draw one card at random from a deck of 10 cards numbered 1 through 10, inclusive, what is the probability that the number you draw is divisible by 9?
A)Pr(Divisible By 9) = 0
B)Pr(Divisible By 9) =
C)Pr(Divisible By 9) =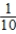
D)Pr(Divisible By 9) =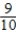
E)Pr(Divisible By 9) =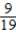
A)Pr(Divisible By 9) = 0
B)Pr(Divisible By 9) =

C)Pr(Divisible By 9) =
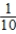
D)Pr(Divisible By 9) =
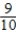
E)Pr(Divisible By 9) =

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
18
If a pair of dice, one green and one red, is rolled, what is the probability that the sum of the two dice is a number between 5 and 9, inclusive?
A)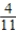
B)
C)
D)
E)
A)
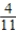
B)

C)

D)

E)


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
19
If the probability that an event will occur is 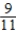 , what are the odds in favor of the event occurring?
, what are the odds in favor of the event occurring?
A)9 : 2
B)2 : 9
C)11 : 2
D)2 : 11
E)9 : 11
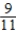 , what are the odds in favor of the event occurring?
, what are the odds in favor of the event occurring? A)9 : 2
B)2 : 9
C)11 : 2
D)2 : 11
E)9 : 11

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
20
One ball is drawn at random from a bag containing 6 red R balls and 10 white W balls. What is the probability that the ball is red or white?
A)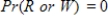
B)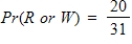
C)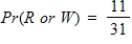
D)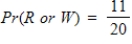
E)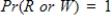
A)
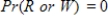
B)
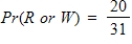
C)
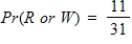
D)
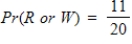
E)
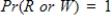

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
21
A ball is drawn from a bag containing 6 red balls numbered 1-6 and 14 white balls numbered 7-20. What is the probability that the ball is not red R?
A)Pr(R') =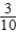
B)Pr(R') =
C)Pr(R') =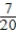
D)Pr(R') =
E)Pr(R') =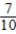
A)Pr(R') =
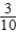
B)Pr(R') =

C)Pr(R') =
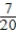
D)Pr(R') =

E)Pr(R') =
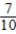

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
22
A bag contains 6 red balls numbered 1-6 and 14 white balls numbered 7-20. If a ball is drawn, what is the probability that the ball is red R or even-numbered E?
A)Pr(R∪E) =
B)Pr(R∪E) =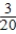
C)Pr(R∪E) =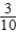
D)Pr(R∪E) =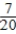
E)Pr(R∪E) =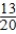
A)Pr(R∪E) =

B)Pr(R∪E) =
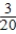
C)Pr(R∪E) =
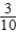
D)Pr(R∪E) =
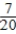
E)Pr(R∪E) =
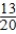

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
23
Five percent of the population of the United States has type AB blood. What is the probability, rounded to two decimal places, that a U.S. resident chosen at random will have type AB blood?
A)Pr(Not AB) = 0.05
B)Pr(Not AB) = 0.95
C)Pr(Not AB) = 0.50
D)Pr(Not AB) = 0.55
E)Pr(Not AB) = 0.16
A)Pr(Not AB) = 0.05
B)Pr(Not AB) = 0.95
C)Pr(Not AB) = 0.50
D)Pr(Not AB) = 0.55
E)Pr(Not AB) = 0.16

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
24
A ball is drawn from a bag containing 16 red balls numbered 1-16 and 12 white balls numbered 17-28. What is the probability that the ball is white W and odd-numbered O?
A)Pr(W∩O) =
B)Pr(W∩O) =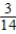
C)Pr(W∩O) =
D)Pr(W∩O) =
E)Pr(W∩O) =
A)Pr(W∩O) =

B)Pr(W∩O) =
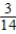
C)Pr(W∩O) =

D)Pr(W∩O) =

E)Pr(W∩O) =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
25
A bag contains 7 white, 9 black, and 10 green balls. What is the probability that a ball drawn at random from the bag is white W or green G?
A)Pr(W∪G) =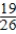
B)Pr(W∪G) =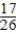
C)Pr(W∪G) =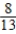
D)Pr(W∪G) =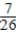
E)Pr(W∪G) =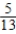
A)Pr(W∪G) =
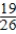
B)Pr(W∪G) =
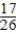
C)Pr(W∪G) =
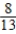
D)Pr(W∪G) =
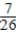
E)Pr(W∪G) =
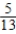

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
26
A ball is drawn from a bag containing 15 red balls numbered 1-15 and 6 white balls numbered 16-21. What is the probability that the ball is red R and even-numbered E?
A)Pr(R∩E) =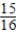
B)Pr(R∩E) =
C)Pr(R∩E) =
D)Pr(R∩E) =
E)Pr(R∩E) =
A)Pr(R∩E) =
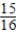
B)Pr(R∩E) =

C)Pr(R∩E) =

D)Pr(R∩E) =

E)Pr(R∩E) =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
27
A ball is drawn from a bag containing 3 red balls numbered 1-3 and 13 white balls numbered 4-16. What is the probability that the number on the ball is odd O?
A)Pr(O) =
B)Pr(O) =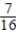
C)Pr(O) =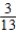
D)Pr(O) =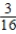
E)Pr(O) =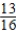
A)Pr(O) =

B)Pr(O) =
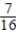
C)Pr(O) =
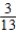
D)Pr(O) =
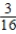
E)Pr(O) =
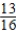

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
28
A company selling substandard drugs to developing countries sold 2,500,000 capsules with 150,000 of them empty. What is the probability that a person who takes a randomly chosen capsule from this company will get an empty capsule?
A)Pr(Empty) =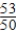
B)Pr(Empty) =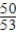
C)Pr(Empty) =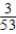
D)Pr(Empty) =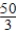
E)Pr(Empty) =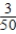
A)Pr(Empty) =
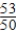
B)Pr(Empty) =
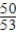
C)Pr(Empty) =
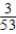
D)Pr(Empty) =
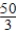
E)Pr(Empty) =
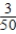

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
29
According to a recent medical study, 196 of 200 mammograms turn out to be normal. What is the probability that the mammogram of a woman chosen at random will show an abnormality?
A)Pr(abnormality) =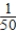
B)Pr(abnormality) = 196
C)Pr(abnormality) = 4
D)Pr(abnormality) =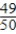
E)Pr(abnormality) =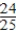
A)Pr(abnormality) =
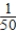
B)Pr(abnormality) = 196
C)Pr(abnormality) = 4
D)Pr(abnormality) =
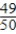
E)Pr(abnormality) =
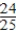

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
30
A computer store offers used computers free to local middle schools. Of the 55 machines available, 15 have defective memories, 12 have defective keys, and the remainder have no defects. If a teacher picks one at random, what is the probability that she will select a defective computer?
A)Pr(Defective) =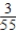
B)Pr(Defective) =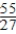
C)Pr(Defective) =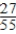
D)Pr(Defective) =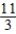
E)Pr(Defective) =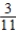
A)Pr(Defective) =
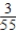
B)Pr(Defective) =
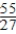
C)Pr(Defective) =
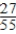
D)Pr(Defective) =
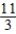
E)Pr(Defective) =
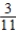

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
31
Suppose a country has 1,000,000 registered voters, 370,000 of whom are Democrats and 430,000 of whom are Republicans. If a registered voter is chosen at random, what is the probability that he or she is neither a Democrat nor a Republican?
A)Pr(neither Democrat nor Republican) = 0.8000
B)Pr(neither Democrat nor Republican) = 0.2000
C)Pr(neither Democrat nor Republican) = 0.9400
D)Pr(neither Democrat nor Republican) = 0.6300
E)Pr(neither Democrat nor Republican) = 0.4300
A)Pr(neither Democrat nor Republican) = 0.8000
B)Pr(neither Democrat nor Republican) = 0.2000
C)Pr(neither Democrat nor Republican) = 0.9400
D)Pr(neither Democrat nor Republican) = 0.6300
E)Pr(neither Democrat nor Republican) = 0.4300

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
32
A ball is drawn from a bag containing 8 red balls numbered 1-8 and 7 white balls numbered 9-15. What is the probability that the ball is neither red R nor even-numbered E?
A)Pr(R'∩E') =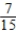
B)Pr(R'∩E') =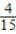
C)Pr(R'∩E') =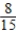
D)Pr(R'∩E') =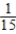
E)Pr(R'∩E') =
A)Pr(R'∩E') =
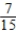
B)Pr(R'∩E') =
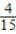
C)Pr(R'∩E') =
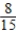
D)Pr(R'∩E') =
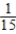
E)Pr(R'∩E') =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
33
An ordinary die is tossed. What is the probability of getting an even number or a number divisible by 6?
A)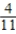
B)
C)
D)
E)
A)
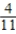
B)

C)

D)

E)


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
34
A ball is drawn from a bag containing 14 red balls numbered 1-14 and 11 white balls numbered 15-25. What is the probability that the ball is red R or even-numbered E?
A)Pr(R∪E) =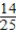
B)Pr(R∪E) =
C)Pr(R∪E) =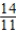
D)Pr(R∪E) =
E)Pr(R∪E) =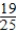
A)Pr(R∪E) =
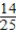
B)Pr(R∪E) =

C)Pr(R∪E) =
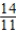
D)Pr(R∪E) =

E)Pr(R∪E) =
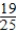

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
35
A ball is drawn from a bag containing 9 red balls numbered 1-9 and 7 white balls numbered 10-16. What is the probability that the ball is white W or odd-numbered O?
A)Pr(W∪O) =
B)Pr(W∪O) =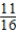
C)Pr(W∪O) =
D)Pr(W∪O) =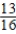
E)Pr(W∪O) =
A)Pr(W∪O) =

B)Pr(W∪O) =
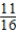
C)Pr(W∪O) =

D)Pr(W∪O) =
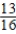
E)Pr(W∪O) =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
36
A card is drawn from an ordinary deck of 52 playing cards. What is the probability that it will be a jack (J), queen (Q), or king (K)?
A)Pr(J or Q or K) =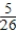
B)Pr(J or Q or K) =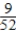
C)Pr(Jor Q or K) =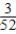
D)Pr(J or Q or K) =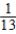
E)Pr(J or Q or K) =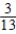
A)Pr(J or Q or K) =
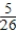
B)Pr(J or Q or K) =
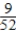
C)Pr(Jor Q or K) =
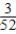
D)Pr(J or Q or K) =
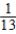
E)Pr(J or Q or K) =
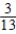

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
37
If the probability of rain today is 23%, what are the odds against rain today?
A)23 : 77
B)77 : 23
C)26 : 81
D)25 : 81
E)none of these choices
A)23 : 77
B)77 : 23
C)26 : 81
D)25 : 81
E)none of these choices

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
38
Suppose a die is biased (unfair) so that each odd-numbered face has probability  of resulting, and each even face has probability
of resulting, and each even face has probability 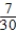 of resulting. Find the probability of getting a number greater than 1.
of resulting. Find the probability of getting a number greater than 1.
A)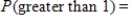
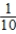
B)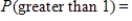
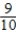
C)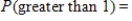
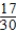
D)
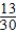
E)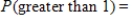
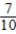
 of resulting, and each even face has probability
of resulting, and each even face has probability 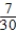 of resulting. Find the probability of getting a number greater than 1.
of resulting. Find the probability of getting a number greater than 1. A)
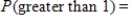
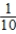
B)
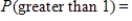
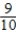
C)
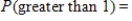
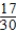
D)

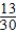
E)
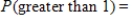
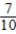

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
39
If the probability that event E will occur is  , what is the probability that E will not occur?
, what is the probability that E will not occur?
A)Pr(E') =
B)Pr(E') =
C)Pr(E') =
D)Pr(E') =
E)Pr(E') =
 , what is the probability that E will not occur?
, what is the probability that E will not occur? A)Pr(E') =

B)Pr(E') =

C)Pr(E') =

D)Pr(E') =

E)Pr(E') =


Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
40
A frustrated store manager is asked to make seven different yes-no decisions that have no relation to each other. Because he is impatient to leave work, he flips a coin for each decision. If the correct decision in each case was no, what is the probability that all of his decisions were correct?
A)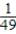
B)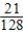
C)
D)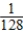
E)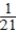
A)
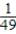
B)
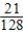
C)

D)
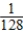
E)
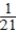

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
41
A fair die is rolled. Find the probability that the result is a 4, given that the result is even.
A)
B)
C)
D)
E)0
A)

B)

C)

D)

E)0

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
42
The following table gives the numbers of AIDS deaths in a single year for people over age 13 in various categories. Use the table to find the probability, rounded to four decimal places, that a person who died of AIDS in this year was Hispanic. 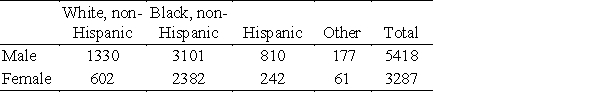
A)Pr(Hispanic) = 0.7155
B)Pr(Hispanic) = 0.4771
C)Pr(Hispanic) = 0.2736
D)Pr(Hispanic) = 0.0652
E)Pr(Hispanic) = 0.1209

A)Pr(Hispanic) = 0.7155
B)Pr(Hispanic) = 0.4771
C)Pr(Hispanic) = 0.2736
D)Pr(Hispanic) = 0.0652
E)Pr(Hispanic) = 0.1209

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
43
The probability that a wife W watches a certain television show is 0.31, that her husband H watches it is 0.21, and that both watch the show is 0.05. What is the probability, to two decimal places, that either the husband or the wife watches this show?
A)Pr(H∪W) = 0.21
B)Pr(H∪W) = 0.05
C)Pr(H∪W) = 0.57
D)Pr(H∪W) = 0.47
E)Pr(H∪W) = 0.52
A)Pr(H∪W) = 0.21
B)Pr(H∪W) = 0.05
C)Pr(H∪W) = 0.57
D)Pr(H∪W) = 0.47
E)Pr(H∪W) = 0.52

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
44
A bag contains 7 red balls numbered 1-7 and 15 white balls numbered 8-22. One ball is drawn from the bag. What is the probability that the ball is red R, given that the ball is even-numbered E?
A)Pr(R | E) =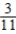
B)Pr(R | E) =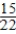
C)Pr(R | E) =
D)Pr(R | E) =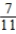
E)Pr(R | E) =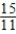
A)Pr(R | E) =
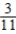
B)Pr(R | E) =
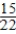
C)Pr(R | E) =

D)Pr(R | E) =
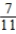
E)Pr(R | E) =

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
45
A bag contains 11 red balls and 13 white balls. Two balls are drawn without replacement. What is the probability that the second ball is white W, given that the first ball is red R?
A)Pr(W | R) =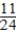
B)Pr(W | R) =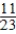
C)Pr(W | R) =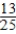
D)Pr(W | R) =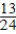
E)Pr(W | R) =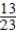
A)Pr(W | R) =
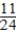
B)Pr(W | R) =
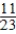
C)Pr(W | R) =
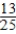
D)Pr(W | R) =
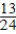
E)Pr(W | R) =
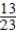

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
46
A mathematics class consists of 17 engineering majors E, 13 science majors S, and 9 liberal arts majors LA. Twelve of the engineering students, 3 of the science majors, and 3 of the liberal arts majors are female. What is the probability that a student selected at random is an engineering major or is a female F?
A)Pr(E∪F) =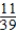
B)Pr(E∪F) =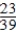
C)Pr(E∪F) =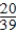
D)Pr(E∪F) =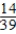
E)Pr(E∪F) =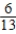
A)Pr(E∪F) =
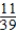
B)Pr(E∪F) =
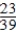
C)Pr(E∪F) =
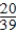
D)Pr(E∪F) =
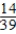
E)Pr(E∪F) =

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
47
The following table gives the numbers of AIDS deaths in a single year for people over age 13 in various categories. Use the table to find the probability, rounded to four decimal places, that a person who died of AIDS in this year was female or Hispanic. 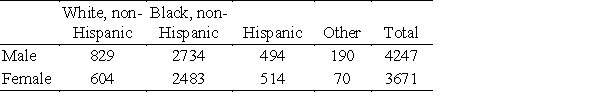
A)Pr(female∪Hispanic) = 0.3589
B)Pr(female∪Hispanic) = 0.4876
C)Pr(female∪Hispanic) = 0.8089
D)Pr(female∪Hispanic) = 0.5260
E)Pr(female∪Hispanic) = 0.5683

A)Pr(female∪Hispanic) = 0.3589
B)Pr(female∪Hispanic) = 0.4876
C)Pr(female∪Hispanic) = 0.8089
D)Pr(female∪Hispanic) = 0.5260
E)Pr(female∪Hispanic) = 0.5683

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
48
A box contains 3 red balls, 5 white balls, and 3 black balls. Two balls are drawn at random from the box with replacement of the first before the second is drawn.What is the probability of getting a red R ball on the first draw and a white W ball on the second?
A)Pr(R first ∩ W second) =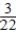
B)Pr(R first ∩ W second) =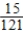
C)Pr(R first ∩ W second) =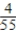
D)Pr(R first ∩ W second) =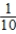
E)Pr(R first ∩ W second) =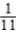
A)Pr(R first ∩ W second) =
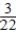
B)Pr(R first ∩ W second) =
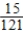
C)Pr(R first ∩ W second) =
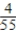
D)Pr(R first ∩ W second) =
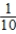
E)Pr(R first ∩ W second) =
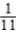

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
49
The following table gives the numbers of AIDS deaths in a single year for people over age 13 in various categories. Use the table to find the probability, rounded to four decimal places, that a person who died of AIDS in this year was female. 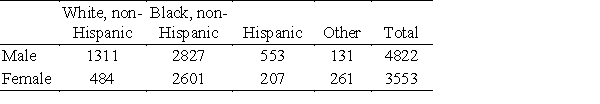
A)Pr(female) = 0.5758
B)Pr(female) = 0.4242
C)Pr(female) = 0.5601
D)Pr(female) = 0.3664
E)Pr(female) = 0.6179

A)Pr(female) = 0.5758
B)Pr(female) = 0.4242
C)Pr(female) = 0.5601
D)Pr(female) = 0.3664
E)Pr(female) = 0.6179

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
50
A fair die is thrown twice. What is the probability that a 3 will result the first time and a 2 the second time?
A)Pr(3 first ∩ 2 second) =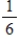
B)Pr(3 first ∩ 2 second) =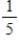
C)Pr(3 first ∩ 2 second) =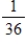
D)Pr(3 first ∩ 2 second) =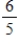
E)Pr(3 first ∩ 2 second) =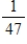
A)Pr(3 first ∩ 2 second) =
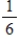
B)Pr(3 first ∩ 2 second) =
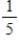
C)Pr(3 first ∩ 2 second) =
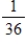
D)Pr(3 first ∩ 2 second) =
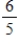
E)Pr(3 first ∩ 2 second) =
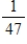

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
51
Suppose that a person groups 18 objects into three groups in such a way that
6 objects are in group A.
6 objects are in group B.
7 objects are in group C.
3 objects are in both group A and group B.
5 objects are in both group B and group C.
4 objects are in both group A and group C.
2 objects are in all three groups.
What is the probability that an object chosen at random has been placed in group A or group B?
A)
B)
C)
D)
E)0
6 objects are in group A.
6 objects are in group B.
7 objects are in group C.
3 objects are in both group A and group B.
5 objects are in both group B and group C.
4 objects are in both group A and group C.
2 objects are in all three groups.
What is the probability that an object chosen at random has been placed in group A or group B?
A)

B)

C)

D)

E)0

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
52
A bag contains 9 red balls and 15 white balls. Two balls are drawn without replacement. What is the probability that the second ball is red R, given that the first ball is white W?
A)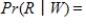

B)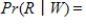
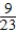
C)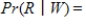
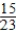
D)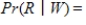

E)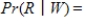
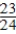
A)
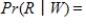

B)
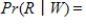
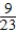
C)
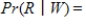
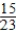
D)
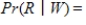

E)
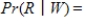
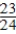

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
53
A mathematics class consists of 14 engineering majors E, 8 science majors S, and 15 liberal arts majors LA. What is the probability that a student selected at random will be an engineering or science major?
A)Pr(E∪S) =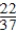
B)Pr(E∪S) =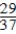
C)Pr(E∪S) =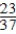
D)Pr(E∪S) =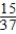
E)Pr(E∪S) =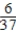
A)Pr(E∪S) =
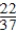
B)Pr(E∪S) =
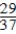
C)Pr(E∪S) =
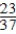
D)Pr(E∪S) =
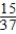
E)Pr(E∪S) =

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
54
A bag contains 4 red balls and 11 white balls. Two balls are drawn from the bag. What is the probability that the second ball is white W, given that the first ball is red and is replaced before the second is drawn?
A)Pr(W) =
B)Pr(W) =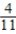
C)Pr(W) =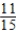
D)Pr(W) =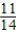
E)Pr(W) =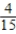
A)Pr(W) =

B)Pr(W) =
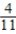
C)Pr(W) =
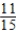
D)Pr(W) =
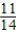
E)Pr(W) =
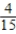

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
55
The following table gives the percent of employees of the Ace Company in each of three salary brackets, categorized by the sex of the employees. An employee is selected at random. What is the probability that the person is male or makes less than $30,000? 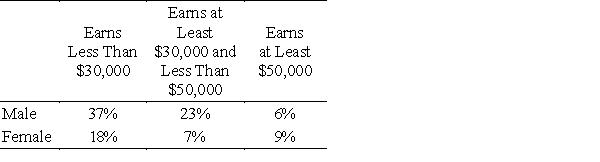
A)Pr(male∪$30,000) = 0.84
B)Pr(male∪$30,000) = 0.55
C)Pr(male∪$30,000) = 0.29
D)Pr(male∪$30,000) = 0.78
E)Pr(male∪$30,000) = 0.47
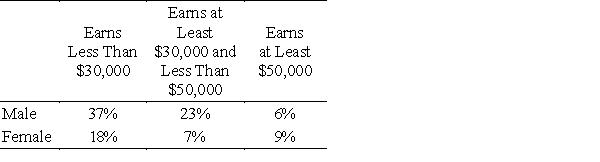
A)Pr(male∪$30,000) = 0.84
B)Pr(male∪$30,000) = 0.55
C)Pr(male∪$30,000) = 0.29
D)Pr(male∪$30,000) = 0.78
E)Pr(male∪$30,000) = 0.47

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
56
The following table gives the numbers of AIDS deaths in a single year for people over age 13 in various categories. Use the table to find the probability, rounded to four decimal places, that a person who died of AIDS in this year was male or black (non-Hispanic). 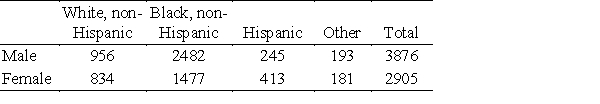
A)Pr(male∪black) = 0.4486
B)Pr(male∪black) = 0.5983
C)Pr(male∪black) = 0.7894
D)Pr(male∪black) = 0.6946
E)Pr(male∪black) = 0.6325

A)Pr(male∪black) = 0.4486
B)Pr(male∪black) = 0.5983
C)Pr(male∪black) = 0.7894
D)Pr(male∪black) = 0.6946
E)Pr(male∪black) = 0.6325

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
57
A mathematics class consists of 9 engineering majors E, 14 science majors S, and 8 liberal arts majors LA. What is the probability that a student selected at random will be a science or liberal arts major?
A)Pr(S∪LA) =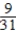
B)Pr(S∪LA) =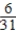
C)Pr(S∪LA) =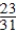
D)Pr(S∪LA) =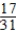
E)Pr(S∪LA) =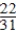
A)Pr(S∪LA) =
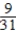
B)Pr(S∪LA) =
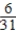
C)Pr(S∪LA) =
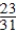
D)Pr(S∪LA) =
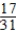
E)Pr(S∪LA) =
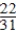

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
58
The following table gives the percent of employees of the Ace Company in each of three salary brackets, categorized by the sex of the employees. An employee is selected at random. What is the probability that the person selected is female and makes less than $30,000? 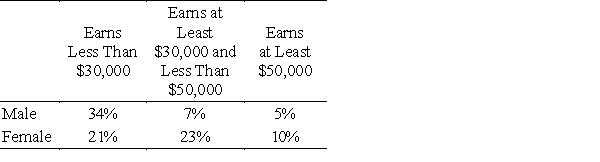
A)Pr(female∩less than $30,000) = 0.34
B)Pr(female∩less than $30,000) = 0.21
C)Pr(female∩less than $30,000) = 0.54
D)Pr(female∩less than $30,000) = 0.55
E)Pr(female∩less than $30,000) = 0.33
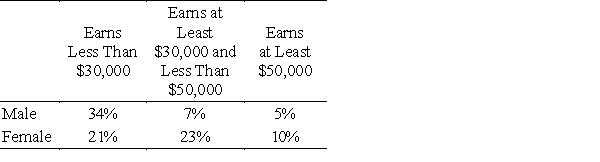
A)Pr(female∩less than $30,000) = 0.34
B)Pr(female∩less than $30,000) = 0.21
C)Pr(female∩less than $30,000) = 0.54
D)Pr(female∩less than $30,000) = 0.55
E)Pr(female∩less than $30,000) = 0.33

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
59
The following table gives the percent of employees of the Ace Company in each of three salary brackets, categorized by the sex of the employees. An employee is selected at random. What is the probability that the person selected makes at least $50,000? 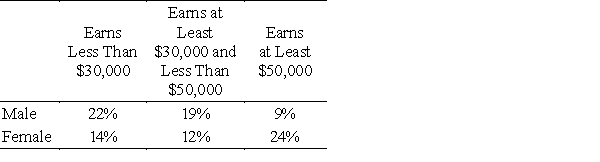
A)Pr(at least $50,000) = 0.31
B)Pr(at least $50,000) = 0.36
C)Pr(at least $50,000) = 0.50
D)Pr(at least $50,000) = 0.33
E)Pr(at least $50,000) = 0.57
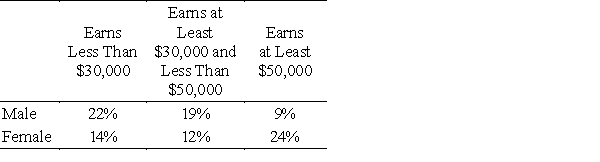
A)Pr(at least $50,000) = 0.31
B)Pr(at least $50,000) = 0.36
C)Pr(at least $50,000) = 0.50
D)Pr(at least $50,000) = 0.33
E)Pr(at least $50,000) = 0.57

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
60
Of 100 students, 26 can speak French F, 33 can speak German G, and 16 can speak both French and German. If a student is picked at random, what is the probability that he or she can speak French or German?
A)Pr(F∪G) =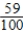
B)Pr(F∪G) =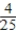
C)Pr(F∪G) =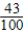
D)Pr(F∪G) =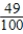
E)Pr(F∪G) =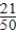
A)Pr(F∪G) =
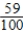
B)Pr(F∪G) =
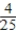
C)Pr(F∪G) =
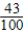
D)Pr(F∪G) =
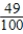
E)Pr(F∪G) =
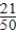

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
61
A red ball and 19 white balls are in a box. If two balls are drawn, without replacement, what is the probability of getting a red R ball on the first draw and a white W ball on the second?
A)Pr(R first ∩ W second) =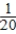
B)Pr(R first ∩ W second) =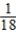
C)Pr(R first ∩ W second) =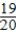
D)Pr(R first ∩ W second) =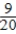
E)Pr(R first ∩ W second) = 1
A)Pr(R first ∩ W second) =
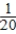
B)Pr(R first ∩ W second) =
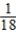
C)Pr(R first ∩ W second) =
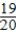
D)Pr(R first ∩ W second) =
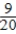
E)Pr(R first ∩ W second) = 1

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
62
The following table gives the results of a 2005 survey of 1000 people regarding the funding of universal health care by employers (employer mandate).  What is the probability that a person selected at random from this group will favor universal health care F with an employer mandate, given that the person is a Democrat D?
What is the probability that a person selected at random from this group will favor universal health care F with an employer mandate, given that the person is a Democrat D?
A)Pr(F | D) =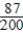
B)Pr(F | D) =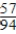
C)Pr(F | D) =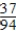
D)Pr(F | D) =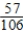
E)Pr(F | D) =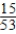
 What is the probability that a person selected at random from this group will favor universal health care F with an employer mandate, given that the person is a Democrat D?
What is the probability that a person selected at random from this group will favor universal health care F with an employer mandate, given that the person is a Democrat D?
A)Pr(F | D) =
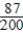
B)Pr(F | D) =
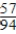
C)Pr(F | D) =
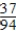
D)Pr(F | D) =
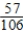
E)Pr(F | D) =

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
63
On June 16, 1997, two amateur golfers playing together hit back-to-back holes in one (Source: The Island Packet, June 19, 1997). Suppose the probability of an amateur golfer getting a hole-in-one is 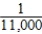 . If the golfers' shots are independent of each other, what is the probability that two amateur golfers will get back-to-back holes in one?
. If the golfers' shots are independent of each other, what is the probability that two amateur golfers will get back-to-back holes in one?
A)Pr(hole-in-one ∩ hole-in-one) =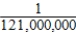
B)Pr(hole-in-one ∩ hole-in-one) =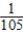
C)Pr(hole-in-one ∩ hole-in-one) =
D)Pr(hole-in-one ∩ hole-in-one) =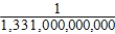
E)Pr(hole-in-one ∩ hole-in-one) =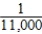
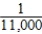 . If the golfers' shots are independent of each other, what is the probability that two amateur golfers will get back-to-back holes in one?
. If the golfers' shots are independent of each other, what is the probability that two amateur golfers will get back-to-back holes in one? A)Pr(hole-in-one ∩ hole-in-one) =
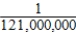
B)Pr(hole-in-one ∩ hole-in-one) =
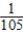
C)Pr(hole-in-one ∩ hole-in-one) =

D)Pr(hole-in-one ∩ hole-in-one) =
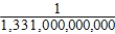
E)Pr(hole-in-one ∩ hole-in-one) =
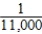

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
64
Forty-eight percent of the U.S. population is female, and 23.3% of the female population have a college degree. If a U.S. resident is chosen at random, what is the probability, to three decimal places, that the person is a female with a college degree?
A)Pr(female ∩ college degree) = 0.233
B)Pr(female ∩ college degree) = 0.713
C)Pr(female ∩ college degree) = 0.023
D)Pr(female ∩ college degree) = 0.112
E)Pr(female ∩ college degree) = 0.048
A)Pr(female ∩ college degree) = 0.233
B)Pr(female ∩ college degree) = 0.713
C)Pr(female ∩ college degree) = 0.023
D)Pr(female ∩ college degree) = 0.112
E)Pr(female ∩ college degree) = 0.048

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
65
An unprepared student must take a 7-question, multiple-choice test that has 5 possible answers per question. If the student can eliminate 1 of the possible answers on the first 5 questions, and if she guesses on every question, what is the probability that she will answer every question incorrectly?
A)Pr(all incorrect) =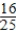
B)Pr(all incorrect) =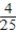
C)Pr(all incorrect) =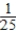
D)Pr(all incorrect) =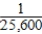
E)Pr(all incorrect) =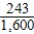
A)Pr(all incorrect) =
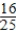
B)Pr(all incorrect) =
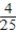
C)Pr(all incorrect) =
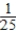
D)Pr(all incorrect) =
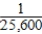
E)Pr(all incorrect) =
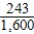

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
66
A bag contains 3 nickels, 5 dimes, and 4 quarters. If you draw 3 coins at random from the bag, without replacement, what is the probability that you will get a nickel N, a quarter Q, and a nickel N, in that order?
A)Pr(N first ∩ Q second ∩ N third) =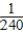
B)Pr(N first ∩ Q second ∩ N third) =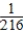
C)Pr(N first ∩ Q second ∩ N third) =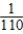
D)Pr(N first ∩ Q second ∩ N third) =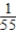
E)Pr(N first ∩ Q second ∩ N third) =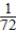
A)Pr(N first ∩ Q second ∩ N third) =
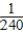
B)Pr(N first ∩ Q second ∩ N third) =
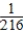
C)Pr(N first ∩ Q second ∩ N third) =
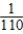
D)Pr(N first ∩ Q second ∩ N third) =
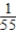
E)Pr(N first ∩ Q second ∩ N third) =
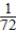

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
67
Two balls are drawn, without replacement, from a bag containing 18 blue balls numbered 1-18 and 4 green balls numbered 19-22. What is the probability that the first ball is blue and even-numbered and the second ball is even-numbered?
A)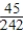
B)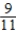
C)0
D)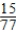
E)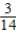
A)
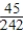
B)
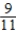
C)0
D)
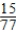
E)
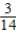

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
68
Suppose the following table summarizes the opinions of various groups on the issue of increased military spending. 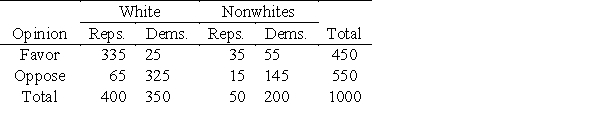
Given that a randomly selected individual is nonwhite NW, find the probability that he or she opposes increased military spending O.
A)Pr(O | NW) =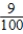
B)Pr(O | NW) =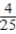
C)Pr(O | NW) =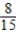
D)Pr(O | NW) =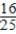
E)Pr(O | NW) =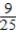

Given that a randomly selected individual is nonwhite NW, find the probability that he or she opposes increased military spending O.
A)Pr(O | NW) =
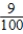
B)Pr(O | NW) =
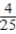
C)Pr(O | NW) =
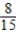
D)Pr(O | NW) =
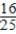
E)Pr(O | NW) =
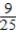

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
69
If 5% of all light bulbs a company manufactures are defective, the probability of any one bulb being defective is 0.05. What is the probability that four bulbs drawn independently from the company's stock will be defective? Round your answer to eight decimal places.
A)0.06250000
B)0.00000031
C)0.00012500
D)0.00000625
E)0.00000000
A)0.06250000
B)0.00000031
C)0.00012500
D)0.00000625
E)0.00000000

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
70
Assuming there are 365 different birthdays, find the probability that five people chosen at random will have different birthdays. Round your answer to five decimal places.
A)0.97736
B)0.84986
C)0.87286
D)0.48643
E)0.97286
A)0.97736
B)0.84986
C)0.87286
D)0.48643
E)0.97286

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
71
From a deck of 52 playing cards, two cards are drawn, one after the other without replacement. What is the probability that the first will be a king and the second will be a jack of the same suit?
A)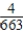
B)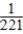
C)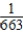
D)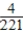
E)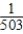
A)
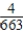
B)
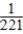
C)
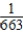
D)
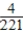
E)
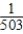

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
72
A red ball and 10 white balls are in a box. If two balls are drawn, without replacement, what is the probability of getting 2 white W balls?
A)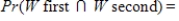

B)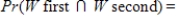
1
C)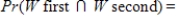
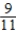
D)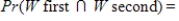
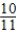
E)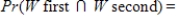
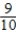
A)
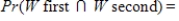

B)
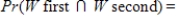
1
C)
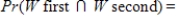
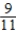
D)
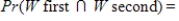
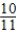
E)
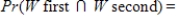
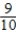

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
73
Each computer component that the Peggos Company produces is tested twice before it is shipped. There is a 0.7 probability that a defective component will be so identified by the first test and a 0.8 probability that it will be identified as being defective by the second test. What is the probability that a defective component will not be identified as defective before it is shipped?
A)Pr(not identified) = 0.560
B)Pr(not identified) = 0.100
C)Pr(not identified) = 0.060
D)Pr(not identified) = 0.500
E)Pr(not identified) = 0.240
A)Pr(not identified) = 0.560
B)Pr(not identified) = 0.100
C)Pr(not identified) = 0.060
D)Pr(not identified) = 0.500
E)Pr(not identified) = 0.240

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
74
A box contains 7 red balls, 6 white balls, and 3 black balls. Two balls are drawn at random from the box without replacement of the first before the second is drawn. What is the probability of getting a red R ball on the first draw and a white W ball on the second?
A)Pr(R first ∩ W second) =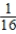
B)Pr(R first ∩ W second) =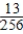
C)Pr(R first ∩ W second) =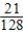
D)Pr(R first ∩ W second) =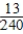
E)Pr(R first ∩ W second) =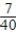
A)Pr(R first ∩ W second) =
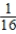
B)Pr(R first ∩ W second) =
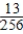
C)Pr(R first ∩ W second) =
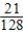
D)Pr(R first ∩ W second) =
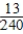
E)Pr(R first ∩ W second) =
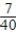

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
75
Thirty-seven percent of the cars owned by a car rental firm have some defect. What is the probability that if 3 cars are selected at random, at least one would have a defect? Round your answer to four decimal places.
A)0.0507
B)0.2500
C)0.7500
D)0.6031
E)0.8425
A)0.0507
B)0.2500
C)0.7500
D)0.6031
E)0.8425

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
76
An unprepared student must take a 7-question, multiple-choice test that has 5 possible answers per question. If the student can eliminate two of the possible answers on the first five questions, and if she guesses on every question, what is the probability that she will answer at least one question correctly?
A)Pr(at least 1 correct) =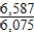
B)Pr(at least 1 correct) =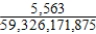
C)Pr(at least 1 correct) =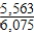
D)Pr(at least 1 correct) =
E)Pr(at least 1 correct) =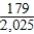
A)Pr(at least 1 correct) =
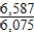
B)Pr(at least 1 correct) =
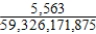
C)Pr(at least 1 correct) =
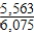
D)Pr(at least 1 correct) =

E)Pr(at least 1 correct) =
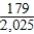

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
77
A company estimates that 50% of the country has seen its commercial and that if a person sees its commercial, there is a 45% probability that the person will buy its product. What is the probability that a person chosen at random in the country will have seen the commercial and bought the product? Round your answer to four decimal places.
A)0.0111
B)0.0500
C)0.4500
D)0.5000
E)0.2250
A)0.0111
B)0.0500
C)0.4500
D)0.5000
E)0.2250

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
78
A red ball and 17 white balls are in a box. If two balls are drawn, without replacement, what is the probability of getting 2 red R balls?
A)Pr(R first ∩ R second) = 1
B)Pr(R first ∩ R second) =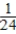
C)Pr(R first ∩ R second) =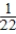
D)Pr(R first ∩ R second) =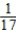
E)Pr(R first ∩ R second) = 0
A)Pr(R first ∩ R second) = 1
B)Pr(R first ∩ R second) =
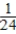
C)Pr(R first ∩ R second) =
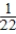
D)Pr(R first ∩ R second) =
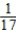
E)Pr(R first ∩ R second) = 0

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
79
From a deck of 52 playing cards, two cards are drawn, one after the other without replacement. What is the probability that the first will be an ace nd the second will be an ace?
A)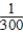
B)
C)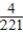
D)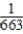
E)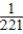
A)
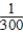
B)

C)
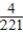
D)
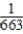
E)
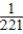

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck
80
An unprepared student must take a 7-question, multiple-choice test that has 5 possible answers per question. If the student can eliminate 2 of the possible answers on the first 4 questions, and if she guesses on every question, what is the probability that she will answer every question correctly?
A)Pr(all correct) =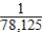
B)Pr(all correct) =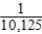
C)Pr(all correct) =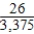
D)Pr(all correct) =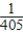
E)Pr(all correct) =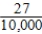
A)Pr(all correct) =
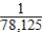
B)Pr(all correct) =
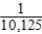
C)Pr(all correct) =
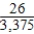
D)Pr(all correct) =
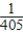
E)Pr(all correct) =
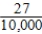

Unlock Deck
Unlock for access to all 178 flashcards in this deck.
Unlock Deck
k this deck



