Chat with AI
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Edition 3ISBN: 978-9352863501Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Edition 3ISBN: 978-9352863501 Exercise 6
This exercise fills in the details of the derivation of the asymptotic distribution of
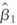given in Appendix 4.3.
a. Use Equation (17.19) to derive the expression
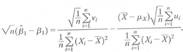
where
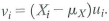
b. Use the central limit theorem, the law of large numbers, and Slutsky s theorem to show that the final term in the equation converges in probability to zero.
c. Use the Cauchy-Schwarz inequality and the third least squaies assumption in Key Concept 17.1 to prove that var( v i ) . Does the term
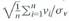satisfy the central limit theorem
d. Apply the central limit theorem and Slutsky's theorem to obtain the result in Equation (17.12).
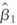given in Appendix 4.3.a. Use Equation (17.19) to derive the expression
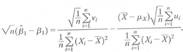
where
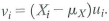
b. Use the central limit theorem, the law of large numbers, and Slutsky s theorem to show that the final term in the equation converges in probability to zero.
c. Use the Cauchy-Schwarz inequality and the third least squaies assumption in Key Concept 17.1 to prove that var( v i ) . Does the term
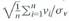satisfy the central limit theorem d. Apply the central limit theorem and Slutsky's theorem to obtain the result in Equation (17.12).
Explanation
Verified
Verified
a) The given equation is
Multiply by t...
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255
