
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Edition 3ISBN: 978-9352863501
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Edition 3ISBN: 978-9352863501 Exercise 3
One version of the expectations theory of the term structure of interest rates holds that a long-term rate equals the average of the expected values of short-term interest rates into the future, plus a term premium that is I (0). Specifically, let Rk t denote a k -period interest rate, let R l t denote a one-period interest rate, and let e t denote an I (0) term premium. Then
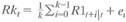 , where
, where
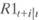 , is the forecast made at date t of the value of R 1 at date t + i. Suppose that R 1 t follows a random walk so that
, is the forecast made at date t of the value of R 1 at date t + i. Suppose that R 1 t follows a random walk so that
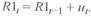
a. Show that
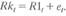
b. Show that Rk t and R 1 t are cointegrated. What is the cointegrating coefficient
c. Now suppose that R 1 t = 0.5 R 1 t 1 + u t. How does your answer to (b) change
d. Now suppose that R 1 t = 0.5 R 1 t 1 + u t. How does your answer to (b) change
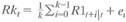 , where
, where 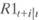 , is the forecast made at date t of the value of R 1 at date t + i. Suppose that R 1 t follows a random walk so that
, is the forecast made at date t of the value of R 1 at date t + i. Suppose that R 1 t follows a random walk so that 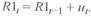
a. Show that
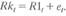
b. Show that Rk t and R 1 t are cointegrated. What is the cointegrating coefficient
c. Now suppose that R 1 t = 0.5 R 1 t 1 + u t. How does your answer to (b) change
d. Now suppose that R 1 t = 0.5 R 1 t 1 + u t. How does your answer to (b) change
Explanation
a) The given random walk model for short...
Introduction to Econometrics 3rd Edition by James Stock, Mark Watson
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255



