
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060X
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060XStep 1 of 4
The number of students per computer in U.S. public schools from the 1983-1984 school year through the 2003-2004 school year can be modeled as follows.
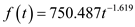
Here 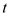 is the number of years after the beginning the 1980-1981 school year.
is the number of years after the beginning the 1980-1981 school year.
(a)
The other function (the number of students per computer) that models the above data is given as follows.
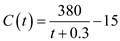
Here 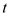 is the number of years after the beginning the 1980-1981 school year.
is the number of years after the beginning the 1980-1981 school year.
To find the basic function that can be transformed to find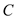 :
:
From the definition of the function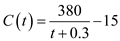 , observe that the basic function to obtain
, observe that the basic function to obtain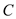 is
is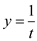 .
.
Stretch the graph of the function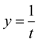 by 380, shift this horizontally by 0.3 units to the left, then shift vertically 15 units down to get the graph of
by 380, shift this horizontally by 0.3 units to the left, then shift vertically 15 units down to get the graph of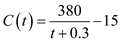 .
.
Step 2 of 4
Step 3 of 4
Step 4 of 4
Why don’t you like this exercise?
Other


