Chat with AI
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060XCollege Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060X
Verified
Step 1 of 3
Consider the function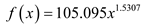
where x the number of years after 1990 and the function
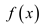gives the amount invested in mutual funds.
(a) Put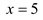
into the equation
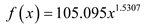to obtain the amount invested in mutual funds in the year 1995 because the number of years from 1990 to 1995 is 5.
Hence the amount invested in mutual funds in the year 1995 is
.
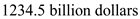Put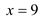
into the equation
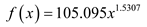to obtain the amount invested in mutual funds in the year 1999 because the number of years from 1990 to 1999 is 9.
Hence the amount invested in mutual funds in the year 1999 is
.
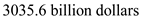The rate change of the function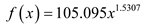
between the years 1995 and 1999 is given by the following equation.
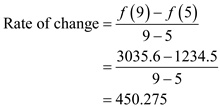
The rate of change is positive it means the function is an increasing function during the years 1995 to 1999.
Step 2 of 3
Step 3 of 3
Why don’t you like this exercise?
Other
