Chat with AI
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060XCollege Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060X Exercise 5
Step-by-step solution
Verified
Verified
Step 1 of 2
Consider the quadratic function:
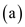
Change the function 
to the form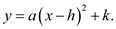 
Factor out 3 from 
Add and subtract 1 to express first three terms in parentheses as a perfect square.
The equation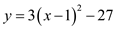 
is in the form of 
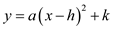with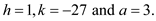 
The vertex coordinates of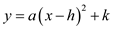 
is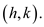 
Therefore, the vertex coordinates of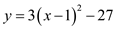 
is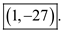 
Step 2 of 2
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255
