
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060X
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060X Exercise 37
Step-by-step solution
Step 1 of 2
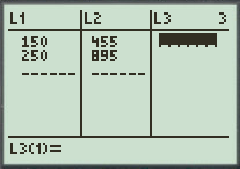
Consider that a company has determined that its profit for a product can be described by a linear function. The profit from the production and sale of 150 units is $455, and the profit from 250 units is $895.
(a) Let us write the equation of the profit function for this product.
By using x as the number of units and y as the profit, enter the data in the lists of a graphing utility.
The figure below shows a partial list of the data points.
The equation of the profit function, found using linear regression with a graphing calculator.
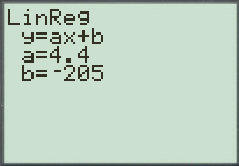
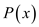 as a function of the number of units x is
as a function of the number of units x is 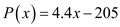 Thus, the equation of profit function is
Thus, the equation of profit function is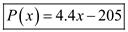 .
. Step 2 of 2
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255



