
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060X
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Edition 3ISBN: 032157060X Exercise 21
Step-by-step solution
Step 1 of 2
Consider the following system of linear equations:
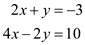
Let us solve this system by elimination.
We convert one of the equations into an equivalent equation of a form such that addition of the two equations will eliminate one of the variables.
Notice that the coefficient of y in the first equation, 1, is a factor of the coefficient of y in the second equation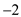 .
.
If we multiply both sides of the first equation by 2 and add the two equations, this will eliminate the y-variable.
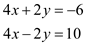
Now add these two equations.
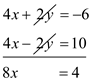
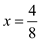 Divide both sides by 8.
Divide both sides by 8.
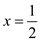
Step 2 of 2
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
Why don’t you like this exercise?
Other Minimum 8 character and maximum 255 character
Character 255



