Consider the following system of linear equations:

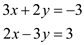
Let us solve this system by elimination.

We convert both the equations into equivalent equations of the form such that addition of the two equations will eliminate one of the variables.

First we multiply both sides of the first equation by 3 and second equation by 2.

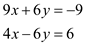
Now add these two equations.

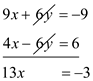
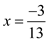Divide both sides by 13.