Deck 21: Game Theory
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/64
Play
Full screen (f)
Deck 21: Game Theory
1
A two-person game encompasses two players.
True
2
A point of equilibrium is a value that is simultaneously the minimum of a row and the maximum of a column.
True
3
In a zero-sum game, one player's gains represent another's exact losses.
True
4
In a ________ game each player adopts a single strategy as an optimal strategy.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
5
The value of the game is the defensive player's gain and the offensive player's loss in a zero-sum game.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
6
In a strategy it is assumed that the payoff table is known to all players.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
7
In a game situation, it is assumed that the payoff table is known to all players.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
8
The payoffs for a mixed strategy game assumes that the game is played many times.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
9
In a mixed strategy game, players switch decision in response to the other player.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
10
A ________ is a plan of action followed by a player.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
11
With the minimax criterion, each player seeks to minimize maximum possible losses.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
12
In a ________ game one player's gains represent another player's exact losses.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
13
In a pure strategy game, each player adopts a single strategy as an optimal strategy.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
14
The best strategy for each player is his or her optimal strategy.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
15
Game theory addresses decision situations with two or more decision makers in competition.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
16
The value of the game is the offensive player's gain and the defensive player's loss in a zero-sum game.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
17
Game theory addresses decision situations with two or fewer decision makers in competition.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
18
The payoffs for a mixed strategy game assumes that the game is played exactly one time.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
19
A point of equilibrium is a value that is simultaneously the maximum of a row and the minimum of a column.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
20
A strategy is a plan of action followed by a player.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
21
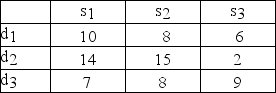
What decision should be made by the optimistic decision maker?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
22
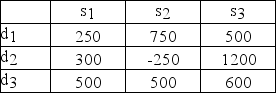
What decision should be made under minimax regret?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
23
The ________ method uses probabilities to determine the best strategy for each player.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
24
In the expected ________ method, a plan of strategies is determined by each player so that expected gain of one equals the expected loss of the other.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
25
In game theory, each player seeks to minimize maximum possible losses with the ________ criterion.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
26
In a(n) ________ game, players switch decisions in response to the decision of the other player and eventually return to the initial decisions, resulting in a closed loop.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
27
A(n) ________ occurs when the optimal strategy for each player results in the same payoff.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
28
In a pure strategy game the optimal strategy for each player results in the same payoff, called an ________ point.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
29
The local operations manager for the IRS must decide whether to hire one, two, or three temporary workers. He estimates that net revenues will vary with how well taxpayers comply with the new tax code. 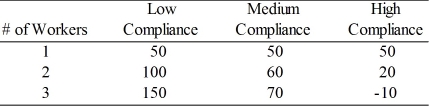
If he uses the maximin criterion, how many new workers will he hire?

If he uses the maximin criterion, how many new workers will he hire?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
30
A pure strategy game can be solved according to the ________ criterion.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
31
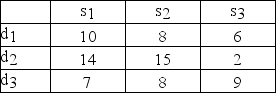
What decision should be made under minimax regret?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
32
A ________ game occurs when each player selects an optimal strategy that does not result in an equilibrium point when the minimax criterion is used.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
33
If the probabilities of s1, s2, and s3 are 0.2, 0.4, and 0.4, respectively, then what decision should be made under expected value?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
34
Given the following payoff table:
 What is the value of the game?
What is the value of the game?
 What is the value of the game?
What is the value of the game?
Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
35
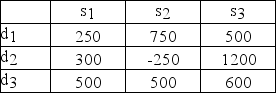
What decision should be made by the conservative decision maker?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
36
A strategy is ________ and can be eliminated if all its payoffs are worse than the corresponding payoffs for another strategy.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
37
The local operations manager for the IRS must decide whether to hire one, two, or three temporary workers. He estimates that net revenues will vary with how well taxpayers comply with the new tax code. 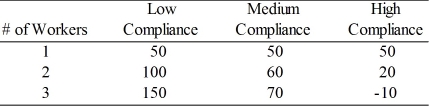
If he uses the minimax regret criterion, how many new workers will he hire?

If he uses the minimax regret criterion, how many new workers will he hire?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
38
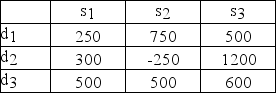
If the probabilities of d1, d2, and d3 are 0.2, 0.5, and 0.3, respectively, then what decision should be made under expected value?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
39
The ________ for a mixed strategy game assumes that the game is played many times.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
40
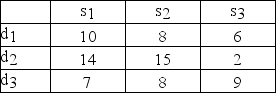
What decision should be made by the conservative decision maker?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
41
The ________ criterion results in the maximum of the minimum payoffs.
A) minimax
B) maximax
C) maximin
D) minimin
A) minimax
B) maximax
C) maximin
D) minimin

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
42
A ________ is a plan of action followed by a player.
A) game situation
B) strategy
C) value of the game
D) best strategy
E) pure strategy
A) game situation
B) strategy
C) value of the game
D) best strategy
E) pure strategy

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
43
In order to attract listeners, a radio station gives away money if listeners correctly identify the amount of money found by a "treasure hunter". A contestant would guess the amount on the air, and the disc jockey would then play a tape in which the "treasure hunter" said how much he had found. If the caller guessed correctly, they won the amount of money found by the treasure hunter. If they guessed incorrectly, they received nothing. Suppose that the treasure hunter either found $50 or $100. The payoff matrix represents this game: 
If the station plans on running the game every day for 10 days, how much will they pay out?

If the station plans on running the game every day for 10 days, how much will they pay out?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
44
Two companies are about to make a decision regarding an investment in a new promotional campaign. Company A will either advertise in all media or advertise in newspapers only. Company B will either run a sweepstake or run a big sale. The payoff matrix is shown below.
Determine the proportion of time that each company should employ each strategy.
Determine the proportion of time that each company should employ each strategy.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
45
Mary Schultz is the incumbent Senator from Ohio and John Henderson is her opponent in the upcoming election. Since Mr. Henderson is seeking to unseat Ms. Schultz, he is on the offensive, while Ms. Schultz hopes to minimize his gains or her losses in the election. The following payoff table shows the various percentage point gains/losses in the polls for the two candidates based on their selection of certain political strategies. 
Determine the optimal strategy and the optimal payoff for both players.

Determine the optimal strategy and the optimal payoff for both players.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
46
Two companies are about to make a decision regarding an investment in a new promotional campaign. Company A will either advertise in all media or advertise in newspapers only. Company B will either run a sweepstake or run a big sale. The payoff matrix is shown below.
Determine the value of the game.
Determine the value of the game.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
47
The local operations manager for the IRS must decide whether to hire one, two, or three temporary workers. He estimates that net revenues will vary with how well taxpayers comply with the new tax code. 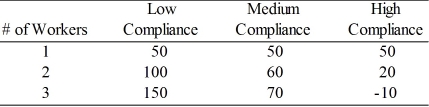
If he thinks the chances of low, medium, and high compliance are 20%, 30%, and 50%, respectively, what are the expected net revenues for the number of workers he will decide to hire?

If he thinks the chances of low, medium, and high compliance are 20%, 30%, and 50%, respectively, what are the expected net revenues for the number of workers he will decide to hire?

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
48
The expected value and expected opportunity loss criteria result in
A) the same decision.
B) different decisions.
C) similar decisions.
D) all of the above
A) the same decision.
B) different decisions.
C) similar decisions.
D) all of the above

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
49
With the ________ criterion each player seeks to minimize maximum possible losses.
A) minimax
B) maximax
C) minimin
D) maximin
A) minimax
B) maximax
C) minimin
D) maximin

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
50
The maximin approach to decision making refers to
A) minimizing the maximum return.
B) maximizing the minimum return.
C) maximizing the maximum return.
D) minimizing the minimum return.
A) minimizing the maximum return.
B) maximizing the minimum return.
C) maximizing the maximum return.
D) minimizing the minimum return.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
51
Regret is the difference between the payoff from the
A) best decision and all other decision payoffs.
B) worst decision and all other decision payoffs.
C) best decision and the worst decision payoffs.
D) none of the above
A) best decision and all other decision payoffs.
B) worst decision and all other decision payoffs.
C) best decision and the worst decision payoffs.
D) none of the above

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
52
Construct the payoff matrix for the game, "rock, paper, scissors" for 2 players, A and B. Assume that "paper covers rock", "rock breaks scissors," and "scissors cut paper." If player A wins, assign +1. If player A loses, assign -1.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
53
Given the following payoff/loss table for a mixed strategy game between two players: 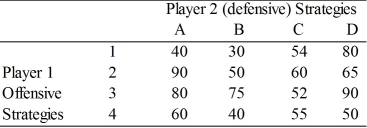
Identify dominated strategy(s), if it (they) exist(s).

Identify dominated strategy(s), if it (they) exist(s).

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
54
Game theory assumes that players behave
A) logically.
B) emotionally.
C) rationally.
D) A, B, and C
E) A and B
A) logically.
B) emotionally.
C) rationally.
D) A, B, and C
E) A and B

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
55
In a zero sum game one player's gains represent another's exact
A) gains.
B) losses.
C) gains and losses.
D) gains or losses.
A) gains.
B) losses.
C) gains and losses.
D) gains or losses.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
56
Mary Schultz is the incumbent senator from Ohio and John Henderson is her opponent in the upcoming election. Since Mr. Henderson is seeking to unseat Ms. Schultz, he is on the offensive, while Ms. Schultz hopes to minimize his gains or her losses in the election. The following payoff table shows the various percentage point gains/losses in the polls for the two candidates based on their selection of certain political strategies. 
Use the expected gain and loss method and determine the probability that each strategy will be used by each player.

Use the expected gain and loss method and determine the probability that each strategy will be used by each player.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
57
A tabular presentation that shows the outcome for each decision alternative under the various possible states of nature is called a
A) decision tree.
B) payoff table.
C) feasible region.
D) payback matrix.
A) decision tree.
B) payoff table.
C) feasible region.
D) payback matrix.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
58
The ________ is the offensive player's gain and the defensive player's loss in a zero-sum game.
A) game situation
B) value of the game
C) strategy
D) best strategy
E) pure strategy
A) game situation
B) value of the game
C) strategy
D) best strategy
E) pure strategy

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
59
Mary Schultz is the incumbent senator from Ohio and John Henderson is her opponent in the upcoming election. Since Mr. Henderson is seeking to unseat Ms. Schultz, he is on the offensive, while Ms. Schultz hopes to minimize his gains or her losses in the election. The following payoff table shows the various percentage point gains/losses in the polls for the two candidates based on their selection of certain political strategies. 
Use the expected gain and loss method and determine the equilibrium point.

Use the expected gain and loss method and determine the equilibrium point.

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
60
Determining the worst payoff for each alternative and choosing the alternative with the best of the worst payoffs is called a ________ criterion.
A) maximin
B) minimin
C) maximax
D) minimax
A) maximin
B) minimin
C) maximax
D) minimax

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
61
Two companies are about to make a decision regarding an investment in a new promotional campaign. Company A will either advertise in all media or advertise in newspapers only. Company B will either run a sweepstake or run a big sale. The payoff matrix is shown below.
Determine the proportion of time that Company B should employ each strategy.
A) B1: 12.5%, B2: 87.5%
B) B1: 25%, B2: 75%
C) B1: 37.5%, B2: 62.5%
D) B1: 50%, B2: 50%
E) B1: 62.5%, B2:37.5%
Determine the proportion of time that Company B should employ each strategy.
A) B1: 12.5%, B2: 87.5%
B) B1: 25%, B2: 75%
C) B1: 37.5%, B2: 62.5%
D) B1: 50%, B2: 50%
E) B1: 62.5%, B2:37.5%

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
62
A(n) ________ game occurs when each player selects an optimal strategy that does not result in an equilibrium point when the minimax criterion is used.
A) optimal strategy
B) pure strategy
C) mixed strategy
D) best strategy
A) optimal strategy
B) pure strategy
C) mixed strategy
D) best strategy

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
63
Two companies are about to make a decision regarding an investment in a new promotional campaign. Company A will either advertise in all media or advertise in newspapers only. Company B will either run a sweepstake or run a big sale. The payoff matrix is shown below.
Determine the proportion of time that Company A should employ each strategy.
A) A1: 12.5%, A2: 87.5%
B) A1: 25%, A2: 75%
C) A1: 37.5%, A2: 62.5%
D) A1: 50%, A2: 50%
E) A1: 62.5%, A2: 37.5%
Determine the proportion of time that Company A should employ each strategy.
A) A1: 12.5%, A2: 87.5%
B) A1: 25%, A2: 75%
C) A1: 37.5%, A2: 62.5%
D) A1: 50%, A2: 50%
E) A1: 62.5%, A2: 37.5%

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck
64
In order to attract listeners, a radio station gives away money if listeners correctly identify the amount of money found by a "treasure hunter". A contestant would guess the amount on the air, and the disc jockey would then play a tape in which the "treasure hunter" said how much he had found. If the caller guessed correctly, they won the amount of money found by the treasure hunter. If they guessed incorrectly, they received nothing. Suppose that the treasure hunter either found $50 or $100. The payoff matrix represents this game: If the station plans on running the game every day for 10 days, how much will they pay out?
A) $250
B) $333
C) $500
D) $750
E) $667
A) $250
B) $333
C) $500
D) $750
E) $667

Unlock Deck
Unlock for access to all 64 flashcards in this deck.
Unlock Deck
k this deck



