Deck 47: Matrices and Systems of Equations

Question 1

Select the order for the following matrix.​ $$\left[ \begin{array} { l l l l } 5 & - 4 & 3 & 3\end{array} \right]$$ ​

A)2 × 2
B)4 × 4
C)3 × 1
D)4 × 1
E)1 × 4

Answer 1

1 × 4

Question 2

Select the augmented matrix for the system of linear equations.​ $$\left\{ \begin{aligned}x + 14 y - 12 z & = 12 \\5 x - 4 y + 5 z & = 0 \\12 x + y & = 9\end{aligned} \right.$$ ​

A)​ $$\left[ \begin{array} { c c c c c } 1 & 14 & 12 & \vdots & 12 \\5 & - 4 & 5 & \vdots & 0 \\12 & 1 & 0 & \vdots & 9\end{array} \right]$$
B) $\left[\begin{array}{cccl}1 & 14 & -12 & :12 \\5 & -4 & 5 & :0\\12 & 1 & 0 & :9\end{array}\right]$
C)​ $\left[\begin{array}{cccl}1 & 14 & -12 & :12 \\5 & 4 & 5 & :0\\12 & 1 & 0 & :9\end{array}\right]$
D)​ $$\left[ \begin{array} { c c c c c } 1 & 14 & 12 & \vdots & 12 \\5 & 4 & 5 & \vdots & 0 \\12 & 1 & 0 & \vdots & 9\end{array} \right]$$
E)​ $\left[\begin{array}{cccl}1 & 14 & -12 & :12 \\5 & -4 & 5 & :0\\12 & 1 & 9 & :0\end{array}\right]$

Answer 2

$\left[\begin{array}{cccl}1 & 14 & -12 & :12 \\5 & -4 & 5 & :0\\12 & 1 & 0 & :9\end{array}\right]$

Question 3

Fill in the blank(s)using elementary row operations to form a row-equivalent matrix. ​​ $$\left[ \begin{array} { c c c } 6 & 9 & 4 \\5 & - 4 & 7\end{array} \right]$$ ​ $$\left[ \begin{array} { c c c } 2 & \cdots & \frac { 4 } { 3 } \\5 & - 4 & 7\end{array} \right]$$ ​

A)​ $$\left[ \begin{array} { c c c } 2 & 9 & \frac { 4 } { 3 } \\5 & - 4 & 7\end{array} \right]$$
B)​ $$\left[ \begin{array} { c c c } 2 & 2 & \frac { 4 } { 3 } \\5 & - 4 & 7\end{array} \right]$$
C)​ $$\left[ \begin{array} { l l l } 2 & - 3 & \frac { 4 } { 3 } \\5 & - 4 & 7\end{array} \right]$$
D)​ $$\left[ \begin{array} { c c c } 2 & 5 & \frac { 4 } { 3 } \\5 & - 4 & 7\end{array} \right]$$
E)​ $$\left[ \begin{array} { c c c } 2 & 3 & \frac { 4 } { 3 } \\5 & - 4 & 7\end{array} \right]$$

Answer 3

​ $$\left[ \begin{array} { c c c } 2 & 3 & \frac { 4 } { 3 } \\5 & - 4 & 7\end{array} \right]$$

Question 4

Fill in the blank(s)using elementary row operations to form a row-equivalent matrix.​ $$\left[ \begin{array} { c c c } 1 & 1 & 1 \\5 & - 2 & 4\end{array} \right]$$ $$\left[ \begin{array} { c c c } 1 & 1 & 1 \\0 & \cdots & - 1\end{array} \right]$$

A)​ $$\left[ \begin{array} { c c c } 1 & 1 & 1 \\0 & 3 & - 1\end{array} \right]$$
B)​ $$\left[ \begin{array} { c c c } 1 & 1 & 1 \\0 & 0 & - 1\end{array} \right]$$
C)​ $$\left[ \begin{array} { c c c } 1 & 1 & 1 \\0 & - 9 & - 1\end{array} \right]$$
D) $$\left[ \begin{array} { c c c } 1 & 1 & 1 \\0 & - 7 & - 1\end{array} \right]$$
E)​ $$\left[ \begin{array} { c c c } 1 & 1 & 1 \\0 & 7 & - 1\end{array} \right]$$

Question 5

Select the system of linear equations represented by the following augmented matrix.​ $$\left[ \begin{array} { r r r } 9 & - 6 & \vdots & 0 \\7 & 4 & \vdots & - 3\end{array} \right]$$ ​

A)​ $$\left\{ \begin{array} { l } 9 x - 6 y = 0 \\7 x + 4 y = 3\end{array} \right.$$
B)​ $$\left\{ \begin{array} { l } 9 x - 6 y = 0 \\7 x - 4 y = 3\end{array} \right.$$
C)​ $$\left\{ \begin{array} { l } 9 x - 6 y = 0 \\7 x + 4 y = 0\end{array} \right.$$
D)​ $$\left\{ \begin{array} { l } 9 x - 6 y = 0 \\7 x + 4 y = - 3\end{array} \right.$$
E)​ $$\left\{ \begin{array} { l } 9 x + 6 y = 0 \\7 x + 4 y = - 3\end{array} \right.$$

Question 6

Find the system of linear equations represented by the augmented matrix.Then use back substitution to solve.(Use variables x,y,z,and w if applicable. )​ $\left[\begin{array}{lll}1 & -4 & \vdots 6\\0 & 1 & \vdots -5\\\end{array} \right]$ ​

A)​ $$\left\{ \begin{aligned}x + 4 y & = 6 \\y & = - 5\end{aligned} \right.$$ (-14,-5)
​
B)​ $$\left\{ \begin{aligned}x - 4 y & = 6 \\y & = - 5\end{aligned} \right.$$ (-14,-5)
​
C)​ $$\left\{ \begin{aligned}x - 4 y & = 6 \\y & = - 5\end{aligned} \right.$$ ​(-14,5)
D)​ $$\left\{ \begin{aligned}x + 4 y & = 6 \\y & = - 5\end{aligned} \right.$$ ​(-5,-14)
E)​ $$\left\{ \begin{aligned}x - 4 y & = 6 \\y & = - 5\end{aligned} \right.$$ ​(-5,-14)

Question 7

Identify the elementary row operation being performed to obtain the new row-equivalent matrix. ​
Original Matrix New Row-Equivalent Matrix $$\left[ \begin{array} { r r r } 4 & - 1 & - 5 \\- 5 & 4 & 9\end{array} \right]$$ $$\left[ \begin{array} { r r r } 4 & - 1 & - 5 \\11 & 0 & - 11\end{array} \right]$$ ​

A)Add 4 times Row 2 to Row 1.
B)Add 5 times Row 2 to Row 1.
C)Add 9 times Row 1 to Row 2.
D)Add 4 times Row 1 to Row 2.
E)Add 5 times Row 1 to Row 2.

Question 8

Select the augmented matrix for the system of linear equations.​ $$\left\{ \begin{aligned}8 x - 4 y + z & = 14 \\16 x - 9 z & = 12\end{aligned} \right.$$ ​

A)​ $$\left[ \begin{array} { c c c c c } 8 & - 4 & 1 & \vdots & 14 \\16 & - 9 & 12 & \vdots & 0\end{array} \right]$$
B)​ $$\left[ \begin{array} { c c c c c } 8 & - 4 & 1 & \vdots & 14 \\16 & - 9 & 0 & \vdots & 12\end{array} \right]$$
C)​ $$\left[ \begin{array} { c c c c c } 8 & 4 & 1 & \vdots & 14 \\16 & 0 & - 9 & \vdots & 12\end{array} \right]$$
D)​ $$\left[ \begin{array} { c c c c c } 8 & - 4 & 0 & \vdots & 14 \\16 & 0 & 9 & \vdots & 12\end{array} \right]$$
E)​ $$\left[ \begin{array} { c c c l } 8 & - 4 & 1 & :14 \\16 & 0 & - 9 & :12\end{array} \right]$$

Question 9

Select the system of linear equations represented by the following augmented matrix.(Variables x,y,z,and w are used whenever applicable. )​ $$\left[ \begin{array} { c c c l } 1 & 3 & :8 \\3 & -4 & :5\end{array} \right]$$ ​

A)​ $$\left\{ \begin{array} { r } x - 3 y = 8 \\3 x - 4 y = 5\end{array} \right.$$
B)​ $$\left\{ \begin{array} { r } x + y = 8 \\3 x - 4 y = 5\end{array} \right.$$
C)​ $$\left\{ \begin{array} { r } x + 3 y = 8 \\4 x + 3 y = 5\end{array} \right.$$
D)​ $$\left\{ \begin{array} { r } x + 3 y = 8 \\3 x - 4 y = 5\end{array} \right.$$
E)​ $$\left\{ \begin{array} { r } x + 3 y = 8 \\3 x + 4 y = 5\end{array} \right.$$

Question 10

Select the order for the following matrix.​ $$\left[ \begin{array} { c c c c } - 3 & 5 & 10 & 0 \\0 & 0 & 5 & 5 \\4 & 4 & 8 & 5\end{array} \right]$$ ​

A)4 × 3
B)4 × 2
C)4 × 4
D)3 × 3
E)3 × 4

Question 11

Select the order for the following matrix.​ $$\left[ \begin{array} { r r r } - 9 & 8 & 7 \\0 & - 5 & 3\end{array} \right]$$ ​

A)2 × 3
B)3 × 1
C)3 × 2
D)3 × 3
E)2 × 2

Question 12

Find the system of linear equations represented by the augmented matrix.Then use back substitution to solve.(Use variables x,y,z,and w if applicable. )​ $$\left[ \begin{array} { c c c c } 1 & 7 & \vdots & 0 \\0 & 1 & \vdots & - 1\end{array} \right]$$ ​

A)​ $$\left\{ \begin{array} { r } x + 7 y = 0 \\y = 0\end{array} \right.$$ ​​(7,0)
B)​ $$\left\{ \begin{aligned}x + 7 y & = 0 \\x & = - 1\end{aligned} \right.$$ ​​(-1,7)
C)​ $$\left\{ \begin{aligned}x + 7 y & = 0 \\y & = - 1\end{aligned} \right.$$ ​​(7,-1)
D)​ $$\left\{ \begin{array} { r } x + 7 y = 0 \\y = 1\end{array} \right.$$ ​​(7,1)
E)​ $$\left\{ \begin{aligned}x + 7 y & = 0 \\y & = - 1\end{aligned} \right.$$ ​​​(7,1)

Question 13

Identify the elementary row operation being performed to obtain the new row-equivalent matrix. ​
Original Matrix New Row-Equivalent Matrix $$\left[ \begin{array} { c c c } - 4 & 6 & 1 \\4 & - 1 & - 9\end{array} \right]$$ $$\left[ \begin{array} { c c c } 20 & 0 & - 53 \\4 & - 1 & - 9\end{array} \right]$$ ​

A)Add 6 times Row 2 to Row 1.
B)Add 3 times Row 1 to Row 2.
C)Add 6 times Row 1 to Row 2.
D)Add 4 times Row 2 to Row 1.
E)Add 3 times Row 2 to Row 1.

Question 14

Determine whether the following matrix is in row-echelon form.If it is,determine if it is also in reduced row-echelon form. ​​ $$\left[ \begin{array} { l l l l } 1 & 0 & 1 & 0 \\0 & 1 & 0 & 3 \\0 & 0 & 1 & 0\end{array} \right]$$ ​

A)Row-echelon form
B)Not in row-echelon form
C)Reduced row-echelon form

Question 15

Select the system of linear equations represented by the following augmented matrix.​ $\left[\begin{array}{l}{\begin{array}{lllr}2 & 0 & 4 & \vdots-13\end{array}} \\\begin{array}{lllr}0 & 1&-2 & \vdots 6\end{array} \\\begin{array}{lllr}5 & 3 & 0 & \vdots 2\end{array} \\\end{array}\right]$ ​

A)​ $$\left\{ \begin{array} { r r } 2 x - 4 z = & 13 \\y - 2 z = & - 6 \\4 x + y = & 3\end{array} \right.$$
B)​ $\left\{\begin{aligned}2 x-4 z= & -13 \\y+2 z= & 6 \\4 x+y= & 3\end{aligned}\right.$
C)​ $$\left\{ \begin{array} { r r } x + y - 2 z = & - 13 \\x + y + 2 z = & 6 \\4 x + y + z = & 3\end{array} \right.$$
D) $$\left\{ \begin{aligned}x - 2 z = & - 13 \\x - 2 z = & 6 \\4 x + y = & 3\end{aligned} \right.$$
E)​ $$\left\{ \begin{array} { r r } 2 x + 4 z = & - 13 \\y - 2 z = & 6 \\5 x + 3 y = & 2\end{array} \right.$$

Question 16

Find the system of linear equations represented by the augmented matrix.Then use back substitution to solve.(Use variables x,y,z and if applicable. )​ $\left[\begin{array}{l}{\begin{array}{cccc}1 & -1 & 6 & \vdots8\end{array}} \\\begin{array}{llll}0 & 1 & -1 & \vdots6\end{array} \\\begin{array}{llll}0 & 0 & -1 & \vdots-6\end{array} \\\end{array}\right]$ ​

A)​ $$\left\{ \begin{aligned}x - y + 6 z & = 8 \\y + z & = 6 \\z & = - 6\end{aligned} \right.$$ ​​(56,12,-6)
B)​ $$\left\{ \begin{aligned}x - y + 6 z & = 8 \\y - z & = - 6 \\z & = 6\end{aligned} \right.$$ ​ ​(44,0,-6)
C)​ $$\left\{ \begin{array} { r } x - y + 6 z = 8 \\y + z = 6 \\z = 6\end{array} \right.$$ ​​(-40,12,6)
D)​ $$\left\{ \begin{aligned}x - y + 6 z & = 8 \\y - z & = 6 \\z & = - 6\end{aligned} \right.$$ ​ ​(44,0,-6)
E)​ $$\left\{ \begin{aligned}x - y + 6 z & = 8 \\y + z & = - 6 \\z & = 6\end{aligned} \right.$$ ​​(-40,12,6)

Question 17

Select the augmented matrix for the system of linear equations.​ $$\left\{ \begin{aligned}6 x - 5 y & = - 7 \\- x + 5 y & = 15\end{aligned} \right.$$ ​

A)​ $$\left[ \begin{array} { c c : c } 5 & - 6 & - 7 \\5 & - 1 & 15\end{array} \right]$$
B) $\left[\begin{array}{cc:c}6 & 5 & -7 \\-1 & 5 & 15\end{array}\right]$
C) $\left[\begin{array}{cc:c}6 & 5 & 7 \\1 & 5 & 15\end{array}\right]$
D) $\left[\begin{array}{cccc}6 & -5 & \vdots & -7 \\-1 & 5 & \vdots & 15\end{array}\right]$
E) $\left[\begin{array}{cc:c}5 & 6 & 7 \\5 & -1 & 15\end{array}\right]$

Question 18

Perform the sequence of row operations on the following matrix. Add -3 times R₁ to R₂.​ $$\left[ \begin{array} { c c c } 1 & 3 & 4 \\3 & - 1 & - 5 \\4 & 1 & - 1\end{array} \right]$$ ​

A)​ $$\left[ \begin{array} { c c c } 1 & 3 & 4 \\0 & 4 & - 17 \\4 & 1 & - 1\end{array} \right]$$
B) $$\left[ \begin{array} { c c c } 1 & 3 & 4 \\0 & - 10 & - 17 \\4 & 1 & - 1\end{array} \right]$$ ​
C) $$\left[ \begin{array} { c c c } 1 & 3 & 4 \\0 & - 10 & 3 \\4 & 1 & - 1\end{array} \right]$$ ​
D) $$\left[ \begin{array} { c c c } - 1 & 3 & 4 \\0 & - 10 & - 17 \\4 & - 1 & - 1\end{array} \right]$$ ​
E) $$\left[ \begin{array} { c c c } 1 & 3 & 4 \\0 & 1 & - 17 \\4 & 1 & - 1\end{array} \right]$$ ​

Question 19

Perform the sequence of row operations on the following matrix. Add R₃ to R₄.​ $$\left[ \begin{array} { c c } 7 & 1 \\0 & 2 \\- 3 & 4 \\4 & 1\end{array} \right]$$ ​

A)​ $$\left[ \begin{array} { l l } 7 & 1 \\0 & 2 \\7 & 5 \\4 & 1\end{array} \right]$$
B)​ $$\left[ \begin{array} { c c } 7 & 1 \\0 & 2 \\- 3 & 4 \\11 & 7\end{array} \right]$$
C)​ $$\left[ \begin{array} { l l } 7 & 1 \\0 & 2 \\1 & 5 \\4 & 1\end{array} \right]$$
D) $$\left[ \begin{array} { c c } 7 & 1 \\0 & 2 \\- 3 & 4 \\7 & 11\end{array} \right]$$
E)​ $$\left[ \begin{array} { c c } 7 & 1 \\0 & 2 \\- 3 & 4 \\1 & 5\end{array} \right]$$

Question 20

Fill in the blank(s)using elementary row operations to form a row-equivalent matrix.​ $$\left[ \begin{array} { c c c c } 1 & 6 & 5 & - 1 \\0 & 1 & - 2 & 2 \\0 & 0 & 1 & 6\end{array} \right]$$ ​​ $$\left[ \begin{array} { c c c c } 1 & 6 & \cdots & \cdots \\0 & 1 & - 2 & 2 \\0 & 0 & 1 & 6\end{array} \right]$$ ​

A)​ $$\left[ \begin{array} { c c c c } 1 & 0 & - 1 & - 13 \\0 & 1 & - 2 & 2 \\0 & 0 & 1 & 6\end{array} \right]$$
B)​ $$\left[ \begin{array} { c c c c } 1 & 6 & - 17 & - 13 \\0 & 1 & - 2 & 2 \\0 & 0 & 1 & 6\end{array} \right]$$
C)​ $$\left[ \begin{array} { c c c c } 1 & 6 & 17 & - 7 \\0 & 1 & - 2 & 2 \\0 & 0 & 1 & 6\end{array} \right]$$
D) $$\left[ \begin{array} { c c c c } 1 & 6 & 17 & - 13 \\0 & 1 & - 2 & 2 \\0 & 0 & 1 & 6\end{array} \right]$$
E)​ $$\left[ \begin{array} { c c c c } 1 & 0 & - 1 & - 7 \\0 & 1 & - 2 & 2 \\0 & 0 & 1 & 6\end{array} \right]$$

Question 21

An augmented matrix that represents a system of linear equations (in variables x,y,z and w if applicable)has been reduced using Gauss-Jordan elimination.Find the solution represented by the augmented matrix.​ $\left[\begin{array}{lll}1 & 0 & \vdots 5\\0 & 1 & \vdots -6\\\end{array} \right]$ ​

A)(-6,7)
B)(-6,5)
C)(-5,6)
D)(5,-6)
E)(6,5)

Question 22

Determine whether the matrix is in row-echelon form.If it is,determine if it is also in reduced row-echelon form. $$\left[ \begin{array} { r r r r } 1 & 8 & - 8 & 4 \\0 & 1 & 0 & 6 \\0 & 0 & 1 & - 9\end{array} \right]$$

A)row-echelon form and reduced row-echelon form
B)row-echelon form
C)neither

Question 23

Determine the order of the matrix.​ $$\left[ \begin{array} { l l l } 4 & 3 & - 8\end{array} \right]$$ ​

A)1 × 1
B)3
C)3 × 3
D)3 × 1
E)1 × 3

Question 24

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination.​ $$\left\{ \begin{aligned}x - 3 z & = - 7 \\3 x + y - 2 z & = 2 \\2 x + 2 y + z & = 4\end{aligned} \right.$$ ​

A)(-5,-5,4)
B)​(5,-5,-4)
C)​(-5,-5,-4)
D)​(5,5,4)
E)​(5,-5,4)

Question 25

Write the augmented matrix for the system of linear equations. $$\left\{ \begin{aligned}x - 3 y - 2 z & = - 2 \\4 y + 4 z & = 4 \\x + 4 z & = - 2\end{aligned} \right.$$

A) $$\left[ \begin{array} { c c c c c } 1 & - 3 & - 2 & \vdots & - 2 \\0 & 4 & 4 & \vdots & 4 \\1 & 4 & 0 & \vdots & - 2\end{array} \right]$$ ​
B) $$\left[ \begin{array} { c c c c c } 1 & - 3 & - 2 & \vdots & - 2 \\4 & 44 & 0 & \vdots & 4 \\1 & 4 & 0 & \vdots & - 2\end{array} \right]$$
C) $$\left[ \begin{array} { c c c c c } 1 & - 3 & - 2 & \vdots & - 2 \\1 & 4 & 4 & \vdots & 4 \\1 & 1 & 4 & \vdots & - 2\end{array} \right]$$
D) $$\left[ \begin{array} { c c c c c } 1 & - 3 & - 2 & \vdots & - 2 \\& 4 & 4 & \vdots & 4 \\1 & & 4 & \vdots & - 2\end{array} \right]$$
E) $$\left[ \begin{array} { c c c c c } 1 & - 3 & - 2 & \vdots & - 2 \\0 & 4 & 4 & \vdots & 4 \\1 & 0 & 4 & \vdots & - 2\end{array} \right]$$ ​

Question 26

The currents in an electrical network are given by the solution of the system​ $$\left\{ \begin{aligned}I _ { 1 } - I _ { 2 } + I _ { 3 } & = 5 \\3 I _ { 1 } + 4 I _ { 2 } & = 19 \\I _ { 2 } + 3 I _ { 3 } & = 28\end{aligned} \right.$$ ​ where I₁,I₂ and I₃ are measured in amperes.Solve the system of equations using matrices.
​

A)I₁ = 5,I₂ = 4,I₃ = 8
B)I₁ = 4,I₂ = 4,I₃ = 8 ​
C)I₁ = 3,I₂ = 4,I₃ = 8 ​
D)I₁ = 2,I₂ = 4,I₃ = 8 ​
E)I₁ = 1,I₂ = 4,I₃ = 8

Question 27

An augmented matrix that represents a system of linear equations (in variables x,y,z and w if applicable)has been reduced using Gauss-Jordan elimination.Find the solution represented by the augmented matrix.​ $\left[\begin{array}{l}{\begin{array}{cccc}1 & 0 & 0 & \vdots8\end{array}} \\\begin{array}{llll}0 & 1 & 0 & \vdots-6\end{array} \\\begin{array}{llll}0 & 0 & 1 & \vdots0\end{array} \\\end{array}\right]$ ​

A)​(0,8,-6)
B)(8,0,-6)
C)(8,-6,0)
D)(-8,-6,0)
E)(8,6,0)

Question 28

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination.​ $$\left\{ \begin{array} { r l c } - x + y - z & = - 20 \\2 x - y + z & = 29 \\3 x + 2 y + z & = 29\end{array} \right.$$ ​

A)​(-9,-3,8)
B)​(-9,-3,-8)
C)(9,-3,8)
D)​(9,-3,-8)
E)​(9,3,8)

Question 29

Write the matrix in reduced row-echelon form. $$\left[ \begin{array} { r r r r } 5 & - 7 & - 3 & 6 \\- 6 & - 6 & 2 & - 44 \\2 & - 4 & - 2 & - 4\end{array} \right]$$

A) $$\left[ \begin{array} { l l l l } 1 & 0 & 0 & 6 \\0 & 1 & 0 & 3 \\0 & 0 & 1 & 5\end{array} \right]$$
B) $$\left[ \begin{array} { l l l l } 1 & 0 & 0 & 1 \\0 & 1 & 0 & 1 \\0 & 0 & 1 & 1\end{array} \right]$$ ​
C) $$\left[ \begin{array} { l l l l } 1 & 0 & 0 & 7 \\0 & 1 & 0 & 2 \\0 & 0 & 1 & 5\end{array} \right]$$ ​
D) $$\left[ \begin{array} { l l l l } 1 & 0 & 0 & 0 \\0 & 1 & 0 & 0 \\0 & 0 & 1 & 0\end{array} \right]$$
E) $$\left[ \begin{array} { l l l l } 1 & 0 & 0 & 7 \\0 & 1 & 0 & 5 \\0 & 0 & 1 & 2\end{array} \right]$$ ​

Question 30

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination.​ $$\left\{ \begin{array} { l } 2 x + 6 y = 10 \\2 x + 3 y = 7\end{array} \right.$$ ​

A)​​(10,1)
B)​(2,1)
C)​​(2,-6)
D)​(-2,1)
E)​​(2,-1)

Question 31

Fill in the blank using elementary row operations to form a row-equivalent matrix. $$\left[ \begin{array} { r r r } 2 & 1 & - 2 \\- 10 & - 1 & 9\end{array} \right]$$ $$\left[ \begin{array} { l l l } 2 & 1 & - 2 \\0 & \square & - 1\end{array} \right]$$

A) $$\left[ \begin{array} { c c c } 2 & 1 & - 2 \\0 & - 6 & - 1\end{array} \right]$$
B) $$\left[ \begin{array} { l l l } 2 & 1 & - 2 \\0 & 4 & - 1\end{array} \right]$$
C) $$\left[ \begin{array} { r r r } 2 & 1 & - 2 \\0 & - 4 & - 1\end{array} \right]$$
D) $$\left[ \begin{array} { l l l } 2 & 1 & - 2 \\0 & 1 & - 1\end{array} \right]$$ ​
E) $$\left[ \begin{array} { l l l } 2 & 1 & - 2 \\0 & 0 & - 1\end{array} \right]$$ ​

Question 32

An augmented matrix that represents a system of linear equations (in variables x,y,z and w if applicable)has been reduced using Gauss-Jordan elimination.Find the solution represented by the augmented matrix.​ $\left[\begin{array}{l}{\begin{array}{cccc}1 & 0 & 0 & \vdots-3\end{array}} \\\begin{array}{llll}0 & 1 & 0 & \vdots-9\end{array} \\\begin{array}{llll}0 & 0 & 1 & \vdots3\end{array} \\\end{array}\right]$ ​

A)​(-3,9,3)
B)(3,-9,3)
C)(-3,-9,3)
D)(-3,-9,-3)
E)(-3,3,-9)

Question 33

Use matrices to find the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination.​ $$\left\{ \begin{aligned}5 x - 5 y & = 5 \\- 2 x - 3 y & = 18\end{aligned} \right.$$ ​

A)​(3,4)
B)(-3,4)
C)(5,18)
D)(-3,-4)
E)(3,-4)

Question 34

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination. ​​ $$\left\{ \begin{array} { r l r } 3 x + 2 y - z + w & = 0 \\x - y + 4 z - w & = 28 \\- 2 x + y + 2 z - w & = 3 \\x + y + z + w & = 8\end{array} \right.$$ ​

A)​(-3,-2,6,1)
B)(-3,2,-6,-1)
C)(3,-2,6,1)
D)(3,-2,6,-1)
E)(3,-2,-6,1)

Question 35

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination.​ $$\left\{ \begin{aligned}x - 4 y + 3 z - 2 w & = 10 \\3 x - 2 y + z - 4 w & = - 22 \\- 4 x + 3 y - 2 z + w & = - 2 \\- 2 x + y - 4 z + 3 w & = - 10\end{aligned} \right.$$ ​

A)​(-2,-1,8,6)
B)(-2,0,8,6)
C)(-2,0,8,-6)
D)​(-2,-1,-8,-6)
E)(-2,0,-8,6)

Question 36

Determine the order of the matrix.​ $$\left[ \begin{array} { l l l } 8 & 5 & 4 \\7 & 8 & 1\end{array} \right]$$ ​

A)3 × 3
B)2 × 2
C)3 × 1
D)2 × 3
E)3 × 2

Question 37

Identify the elementary row operation being performed to obtain the new row-equivalent matrix. Original Matrix
New Row-Equivalent Matrix $$\left[ \begin{array} { r r r } - 1 & - 8 & 5 \\- 8 & 9 & 9\end{array} \right]$$ $$\left[ \begin{array} { r r r } - 17 & 10 & 23 \\- 8 & 9 & 9\end{array} \right]$$

A)Add 2 times R₁ to R₂.
B)Add -2 times R₁ to R₂.
C)Add 2 times R₂ to R₁.
D)Add -2 times R₂ to R₁.
E)Add 2 times R₁ to R₁.

Question 38

Use the matrix capabilities of a graphing utility to reduce the augmented matrix corresponding to the system of equations,and solve the system.​ $$\left\{ \begin{aligned}x + 2 y + 2 z + 4 w & = 20 \\3 x + 6 y + 5 z + 12 w & = 53 \\x + 3 y - 3 z + 2 w & = - 18 \\6 x - y - z + w & = - 30\end{aligned} \right.$$ ​

A)​(-4,1,7,-2)
B)(-4,-1,7,2)
C)(-4,1,-7,2)
D)(-4,-1,-7,-2)
E)(-4,1,7,2)

Question 39

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination.​ $$\left\{ \begin{array} { l } x + 2 y = 6 \\2 x + y = 9\end{array} \right.$$ ​

A)​(1,4)
B)​(3,4)
C)(1,12)
D)(4,1)
E)(12,4)

Question 40

Write the system of linear equations represented by the augmented matrix.(Use variables x,y,z,and w. ) $\left[\begin{array}{l}{\begin{array}{lllll}-1 & 0 & 0 &3&\vdots2\end{array}} \\\begin{array}{lllll}-7 & 4 & 0 &0& \vdots8\end{array} \\\begin{array}{lllll}0 & 5 & 3 &-5& \vdots-8\end{array} \\\begin{array}{lllll}0 & 0 & -1 &-9& \vdots9\end{array} \\\end{array}\right]$

A) $$\left\{ \begin{array} { r l r } - x + 3 w & = 2 \\- 7 x + 4 y & = 8 \\5 y + 3 z - 5 w & = - 8 \\- y - 9 z & = 9\end{array} \right.$$ ​
B) $$\left\{ \begin{array} { r l r } - x + 3 z & = 2 \\- 7 x + 4 z & = 8 \\5 y + 3 z - 5 w & = - 8 \\- z - 9 w & = 9\end{array} \right.$$
C) $$\left\{ \begin{array} { r l r } - x + 3 y & = & 2 \\- 7 x + 4 y & = & 8 \\5 x + 3 y - 5 z & = - 8 \\- x - 9 y & = 9\end{array} \right.$$
D) $$\left\{ \begin{aligned}- x + 3 w & = 2 \\- 7 x + 4 y & = 8 \\5 y + 3 z - 5 w & = - 8 \\- z - 9 w & = 9\end{aligned} \right.$$
E) $$\left\{ \begin{array} { r l r } - x + 3 z & = 2 \\- 7 x + 4 y & = 8 \\5 y + 3 z - 5 w & = - 8 \\- z - 9 w & = 9\end{array} \right.$$

Question 41

Determine whether the matrix is in row-echelon form.If it is,determine if it is also in reduced row-echelon form. $$\left[ \begin{array} { r r r r } 1 & 9 & - 9 & - 6 \\0 & 1 & 0 & - 2 \\0 & 0 & 1 & 1\end{array} \right]$$

A)row-echelon form
B)row-echelon form and reduced row-echelon form
C)neither

Question 42

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination.​ $$\left\{ \begin{array} { c } 4 x + 9 y + z = - 17 \\6 x - 6 y - 8 z = 12 \\9 x + 8 y + z = - 43\end{array} \right.$$

A)x = 5,y = -1,z = -6
B)x = -5,y = -1,z = -6
C)x = -5,y = 1,z = -6
D)x = 1,y = 5,z = 6
E)no solution

Question 43

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination. $$\left\{ \begin{aligned}4 x - 9 y - 9 z & = - 74 \\6 x + 8 y - 2 z & = - 22 \\6 x - y + 9 z & = 4\end{aligned} \right.$$

A)x = 5,y = -2,z = 4
B)x = 2,y = 5,z = -4
C)x = -5,y = -2,z = 4
D)no solution
E)x = -5,y = 2,z = 4

Question 44

Use a system of equations to find the specified equation that passes through the points.Solve the system using matrices. Parabola: y = ax² + bx + c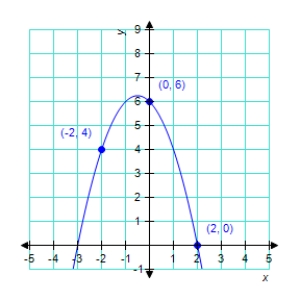

A)​ $y = 2 x ^ { 2 } - x + 6$
B)​ $y = - x ^ { 2 } + 6$
C)​ $y = - x ^ { 2 } + x + 6$
D)​ $y = + x ^ { 2 } + 6$
E)​ $y = - x ^ { 2 } - x + 6$

Question 45

Write the system of linear equations represented by the augmented matrix.(Use variables x,y,z,and w. ) $\left[\begin{array}{rrrrl}1 & 0 & 0 & 4 & :-5\\3 & -7 & 0 & 0 & :3\\0&-4&8&-1&:-6 \\0 & 0 & 3 & 7 &:7\end{array}\right]$

A) $$\left\{ \begin{array} { r l r } x + 4 y & = & - 5 \\3 x - 7 y & = & 3 \\- 4 x + 8 y - z & = & - 6 \\3 x + 7 y & = & 7\end{array} \right.$$ ​
B) $$\left\{ \begin{array} { r l r } x + 4 z & = - 5 \\3 x - 7 y & = 3 \\- 4 y + 8 z - w & = - 6 \\3 z z + 7 w & = 7\end{array} \right.$$ ​
C) $$\left\{ \begin{array} { r l r } x + 4 z & = - 5 \\3 x - 7 z & = 3 \\- 4 y + 8 z - w & = - 6 \\3 z z + 7 w & = 7\end{array} \right.$$ ​
D) $$\left\{ \begin{array} { r l r } x + 4 w & = - 5 \\3 x - 7 y & = 3 \\- 4 y + 8 z - w & = - 6 \\3 y + 7 z & = 7\end{array} \right.$$ ​
E) $$\left\{ \begin{aligned}x + 4 w & = - 5 \\3 x - 7 y & = 3 \\- 4 y + 8 z - w & = - 6 \\3 z + 7 w & = 7\end{aligned} \right.$$ ​

Question 46

Perform the sequence of row operations on the matrix.What did the operations accomplish?​ $$\left[ \begin{array} { c c c } 1 & 1 & - 7 \\4 & 5 & - 7 \\1 & 3 & 35\end{array} \right]$$ ​ Add -4 times R₁ to R₂,
Add -1 times R₁ to R₃,
Add -2 times R₂ to R₃,
Add -1 times R₂ to R₁.
​

A) $$\left[ \begin{array} { l l l } 1 & 0 & 0 \\0 & 1 & 0 \\0 & 0 & 1\end{array} \right]$$ The operations produce a row-equivalent matrix in reduced row-echelon form.
B) $$\left[ \begin{array} { r r r } 1 & 0 & - 28 \\0 & 1 & 21 \\0 & 0 & 0\end{array} \right]$$ The operations produce a row-equivalent matrix in reduced row-echelon form.
C) $$\left[ \begin{array} { r r r } 1 & 1 & - 28 \\0 & 1 & 21 \\0 & 0 & 0\end{array} \right]$$ The operations produce a row-equivalent matrix in reduced row-echelon form.
D) $$\left[ \begin{array} { c c c } 1 & 1 & - 7 \\0 & 1 & 21 \\0 & 2 & 42\end{array} \right]$$ The operations produce a row-equivalent matrix in reduced row-echelon form.
E) $$\left[ \begin{array} { r r r } 1 & 0 & - 28 \\0 & 0 & 21 \\0 & 0 & 0\end{array} \right]$$ The operations produce a row-equivalent matrix in reduced row-echelon form.

Question 47

Determine whether the two systems of linear equations yield the same solutions.If so,find the solutions using matrices. $$\left\{ \begin{aligned}x - y - 6 z & = - 33 \\y + 3 z & = 18 \\z & = 4\end{aligned} \right.$$ $$\left\{ \begin{array} { r l r } x - 7 y - 3 z & = - 57 \\y - 9 z & = - 30 \\z & = 4\end{array} \right.$$

A)x = -3,y = 6,z = 4
B)The systems yield different solutions.
C)x = 3,y = 4,z = -3
D)x = 3,y = -6,z = 4
E)x = 6,y = 4,z = -3

Question 48

Write the augmented matrix for the system of linear equations. $$\left\{ \begin{aligned}x + 7 y + 7 z & = - 6 \\3 y + 3 z & = 6 \\x + 7 z & = - 7\end{aligned} \right.$$

A) $$\left[ \begin{array} { r r r r r } 1 & 7 & 7 & \vdots & - 6 \\1 & 3 & 3 & \vdots & 6 \\1 & 1 & 7 & \vdots & - 7\end{array} \right]$$ ​
B) $$\left[ \begin{array} { r r r r r } 1 & 7 & 7 & \vdots & - 6 \\1 & 3 & 3 & \vdots & 6 \\1 & 0 & 7 & \vdots & - 7\end{array} \right]$$ ​
C) $$\left[ \begin{array} { r r r r r } 1 & 7 & 7 & \vdots & - 6 \\& 3 & 3 & \vdots & 6 \\1 & & 7 & \vdots & - 7\end{array} \right]$$ ​
D) $\left[\begin{array}{rrrrr}1 & 7 & 7 & \vdots & -6 \\3 & 33 & 0 & \vdots & 6 \\1 & 7 & 0 & \vdots & -7\end{array}\right]$ ​
E) $$\left[ \begin{array} { r r r r r } 1 & 7 & 7 & \vdots & - 6 \\0 & 3 & 3 & \vdots & 6 \\1 & 7 & 0 & \vdots & - 7\end{array} \right]$$

Question 49

Write the matrix in reduced row-echelon form. $$\left[ \begin{array} { r r r } 4 & 5 & 11 \\3 & 1 & - 11 \\3 & 5 & 17\end{array} \right]$$

A) $$\left[ \begin{array} { r r r } 1 & 0 & - 6 \\0 & 0 & 0 \\0 & 0 & 0\end{array} \right]$$
B) $$\left[ \begin{array} { l l l } 1 & 0 & 0 \\0 & 1 & 0 \\0 & 0 & 0\end{array} \right]$$ ​
C) $$\left[ \begin{array} { c c c } 1 & 0 & - 4 \\0 & 1 & 5 \\0 & 0 & 1\end{array} \right]$$ ​
D) $$\left[ \begin{array} { c c c } 1 & 0 & - 7 \\0 & 1 & - 6 \\0 & 0 & 0\end{array} \right]$$ ​
E) $$\left[ \begin{array} { r r r } 1 & 0 & - 6 \\0 & 1 & 7 \\0 & 0 & 0\end{array} \right]$$ ​

Question 50

Determine the order of the matrix. $$\left[ \begin{array} { r r r } 6 & - 7 & 7 \\9 & 6 & 7\end{array} \right]$$

A)2 × 3
B)3 × 3
C)3 × 1
D)3 × 2
E)2 × 2

Question 51

An augmented matrix that represents a system of linear equations (in variables x,y,and z)has been reduced using Gauss-Jordan elimination.Write the solution represented by the augmented matrix. $\left[\begin{array}{llll}1&0&0&:2\\0 & 1 & 0 & :3 \\0 & 0 & 1 & :4\end{array}\right]$

A)x = 0,y = 0,z = 0
B)x = 2x,y = 4y,z = 3z
C)x = 2,y = 3,z = 4
D)x = -2,y = -3,z = -4
E)x = 2,y = 0,z = 0

Question 52

Identify the elementary row operation being performed to obtain the new row-equivalent matrix. Original Matrix
New Row-Equivalent Matrix $$\left[ \begin{array} { r r r } - 9 & - 8 & 6 \\- 8 & - 5 & - 3\end{array} \right]$$ $$\left[ \begin{array} { r r r } 7 & 2 & 12 \\- 8 & - 5 & - 3\end{array} \right]$$

A)Add -2 times R₁ to R₂.
B)Add 2 times R₂ to R₁.
C)Add 2 times R₁ to R₂.
D)Add -2 times R₁ to R₁.
E)Add -2 times R₂ to R₁.

Question 53

Write the system of linear equations represented by the augmented matrix.Then use back-substitution to solve.(Use variables x,y,and z. ) $\left[\begin{array}{rrrrrr}1 & 2 & 5 & \vdots & 34 \\0 & 1 & 2 & \vdots & 14 \\0 & 0 & 1 & \vdots & 5\end{array}\right]$

A)x = 35,y = -3,z = 1
B)x = 4,y = 1,z = 5
C)x = 1,y = -4,z = -5
D)x = 1,y = 4,z = 5
E)x = -4,y = 5,z = -1

Question 54

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination. $$\left\{ \begin{aligned}7 x - 6 y & = - 40 \\- 5 x + 7 y & = 34\end{aligned} \right.$$

A)x = 4,y = -2
B)x = -4,y = 2
C)x = 2,y = -4
D)x = 2,y = 4
E)no solution

Question 55

Write the system of linear equations represented by the augmented matrix.Then use back-substitution to solve.(Use variables x,y,and z. ) $$\left[ \begin{array} { r r r r r } 1 & 9 & - 3 &\vdots& - 39 \\0 & 1 & 2 & \vdots & 0 \\0 & 0 & 1 & \vdots & 2\end{array} \right]$$

A)x = 4,y = 2,z = -3
B)x = 3,y = -4,z = 2
C)x = 3,y = 4,z = -2
D)x = -18,y = -1,z = 4
E)x = -4,y = 3,z = 2

Question 56

Fill in the blank using elementary row operations to form a row-equivalent matrix. $$\left[ \begin{array} { r r r } - 1 & 5 & - 3 \\- 2 & 1 & 4\end{array} \right]$$ $\left[\begin{array}{rrr}-1 & 5 & -3 \\0 & \square & 10\end{array}\right]$

A) $$\left[ \begin{array} { r r r } - 1 & 5 & - 3 \\0 & - 9 & 10\end{array} \right]$$ ​
B) $$\left[ \begin{array} { r r r } - 1 & 5 & - 3 \\0 & 0 & 10\end{array} \right]$$ ​
C) $$\left[ \begin{array} { r r r } - 1 & 5 & - 3 \\0 & 11 & 10\end{array} \right]$$ ​
D) $$\left[ \begin{array} { r r r } - 1 & 5 & - 3 \\0 & 5 & 10\end{array} \right]$$ ​
E) $$\left[ \begin{array} { r r r } - 1 & 5 & - 3 \\0 & 9 & 10\end{array} \right]$$ ​

Question 57

Use matrices to solve the system of equations (if possible).Use Gaussian elimination with back-substitution or Gauss-Jordan elimination. $$\left\{ \begin{aligned}2 x + 9 y & = - 35 \\- 9 x + 2 y & = 30\end{aligned} \right.$$

A)x = -3,y = -4
B)x = 4,y = 3
C)x = -4,y = -3
D)x = -3,y = 4
E)no solution

Question 58

Write the matrix in reduced row-echelon form. $$\left[ \begin{array} { r r r r } 9 & - 6 & - 3 & - 33 \\8 & - 1 & 1 & - 47 \\- 7 & - 7 & 6 & - 8\end{array} \right]$$

A) $$\left[ \begin{array} { l l l l } 1 & 0 & 0 & 0 \\0 & 1 & 0 & 0 \\0 & 0 & 1 & 0\end{array} \right]$$ ​
B) $$\left[ \begin{array} { l l l r } 1 & 0 & 0 & - 5 \\0 & 1 & 0 & - 6 \\0 & 0 & 1 & 1\end{array} \right]$$ ​
C) $$\left[ \begin{array} { r r r r } 1 & 0 & 0 & - 6 \\0 & 1 & 0 & 2 \\0 & 0 & 1 & - 6\end{array} \right]$$ ​
D) $$\left[ \begin{array} { r r r r } 1 & 0 & 0 & - 5 \\0 & 1 & 0 & 1 \\0 & 0 & 1 & - 6\end{array} \right]$$ ​
E) $$\left[ \begin{array} { l l l l } 1 & 0 & 0 & 1 \\0 & 1 & 0 & 1 \\0 & 0 & 1 & 1\end{array} \right]$$

Question 59

Determine whether the two systems of linear equations yield the same solutions.If so,find the solutions using matrices. $$\left\{ \begin{aligned}x + 2 y - 4 z & = - 8 \\y - 7 z & = 4 \\z & = - 1\end{aligned} \right.$$ $$\left\{ \begin{aligned}x - y - 5 z & = 21 \\y - 6 z & = 3 \\z & = - 1\end{aligned} \right.$$

A)x = 6,y = 3,z = -1
B)x = -6,y = -3,z = -1
C)x = 3,y = -1,z = -6
D)The systems yield different solutions.
E)x = -3,y = -1,z = -6

Question 60

The currents in an electrical network are given by the solutions of the system $$\left\{ \begin{array} { r l r } I _ { 1 } + I _ { 2 } - I _ { 3 } & = 0 \\2 I _ { 1 } + 3 I _ { 3 } & = 37 \\4 I _ { 2 } + I _ { 3 } & = 25\end{array} \right.$$ where I₁,I₂,and I₃ are measured in amperes.Solve the system of equations using matrices.

A)no solution
B)I₁ = 3,I₂ = 3,I₃ = 7
C)I₁ = 5,I₂ = 4,I₃ = 9
D)I₁ = 3,I₂ = 3,I₃ = 6
E)I₁ = 5,I₂ = 9,I₃ = 4

Question 61

The currents in an electrical network are given by the solutions of the system $$\left\{ \begin{aligned}I _ { 1 } + I _ { 2 } - I _ { 3 } & = 0 \\4 I _ { 1 } + 5 I _ { 3 } & = 23 \\6 I _ { 2 } + I _ { 3 } & = 9\end{aligned} \right.$$ where I₁,I₂,and I₃ are measured in amperes.Solve the system of equations using matrices.

A)I₁ = 2,I₂ = 3,I₃ = 1
B)I₁ = 2,I₂ = 1,I₃ = 3
C)I₁ = 4,I₂ = 4,I₃ = 9
D)I₁ = 4,I₂ = 4,I₃ = 8
E)no solution

Question 62

Solve the system using Gauss-Jordan elimination.​ $$\left\{ \begin{aligned}x + y + 2 z + t & = 62 \\x + 2 y + z + t & = 62 \\2 x + y + z + t & = 49 \\x + y + z + 2 t & = 62\end{aligned} \right.$$ ​

A) $( 2 , - 15,15 , - 15 )$ ​
B) $( - 2,15,15,15 )$ ​
C) $( 2 , - 15,15,15 )$ ​
D) $( 2,15,15,15 )$ ​
E) $( 2,15 , - 15,15 )$ ​

Question 63

Solve the system by Gauss - Jordan elimination.​ $$\left\{ \begin{aligned}\frac { 1 } { 3 } x + \frac { 3 } { 4 } y - \frac { 2 } { 3 } z & = - 4 \\x + \frac { 1 } { 2 } y + \frac { 1 } { 3 } z & = 30 \\\frac { 1 } { 6 } x - \frac { 1 } { 8 } y - z & = - 30\end{aligned} \right.$$ ​

A) $( - 12 , - 16 , - 30 )$ ​
B) $( - 12,16 , - 30 )$ ​
C) $( 12,16,30 )$ ​
D) $( 0,16 , - 30 )$ ​
E) $( 12 , - 16,30 )$ ​

Question 64

Solve the system using Gauss-Jordan elimination.​ $$\left\{ \begin{array} { l } w + x = 9 \\w + y = 0 \\x + z = 0\end{array} \right.$$

A) $$w = - 9 + z , x = - z , y = - 9 - z , z$$
B) $w = 9 + z , x = - z , y = - 9 - z , z$
C) $$w = 9 + z , x = - z , y = 9 - z , z$$ ​
D) $w = 9 + z , x = - z , y = 9 + z , z$ ​
E) $w = 9 - z , x = - z , y = - 9 - z , z$ ​

Question 65

Solve the system using Gaussian elimination.​ $$\left\{ \begin{array} { l } x + y = - 2 \\x + z = - 4 \\y + z = - 4\end{array} \right.$$ ​

A) $( 1,1,3 )$
B) $( - 1 , - 1 , - 3 )$
C) $( 1,1 , - 3 )$
D) $( 1 , - 1 , - 3 )$
E) $( - 1,1 , - 3 )$