Deck 8: Combinations of Functions Composite Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/58
Play
Full screen (f)
Deck 8: Combinations of Functions Composite Functions
1
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
5
Evaluate the indicated function for and .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
6
Find (f + g)(x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
7
Find (f / g)(x).What is the domain of f / g?
A) ;all real numbers x.
B) ;all real numbers x except x = 0
C) ;all real numbers x except x =
D) ;all real numbers x except x = 0
E) ;all real numbers x except x =
A) ;all real numbers x.
B) ;all real numbers x except x = 0
C) ;all real numbers x except x =
D) ;all real numbers x except x = 0
E) ;all real numbers x except x =

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
8
Find (f + g)(x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
9
Evaluate the indicated function for and .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
10
Evaluate the indicated function for and .
A)30
B)
C)
D)
E)
A)30
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
11
Evaluate the indicated function for and .
A)12
B)-10
C)7
D)14
E)10
A)12
B)-10
C)7
D)14
E)10

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
12
Evaluate the indicated function for and .
A)48
B)39
C)9
D)0
E)-39
A)48
B)39
C)9
D)0
E)-39

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
13
Find (f + g)(x).
A)2x
B)4x
C)-4x
D)-2x
E)2x + 8
A)2x
B)4x
C)-4x
D)-2x
E)2x + 8

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
14
Evaluate the indicated function for and .
A)92
B)90
C)-86
D)89
E)91
A)92
B)90
C)-86
D)89
E)91

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
15
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
16
Find (f - g)(x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
17
Find (f - g)(x).
A)2x - 6
B)6
C)2x - 3
D)2x + 6
E)2x
A)2x - 6
B)6
C)2x - 3
D)2x + 6
E)2x

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
18
Find (fg)(x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
19
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
20
Evaluate the indicated function for and .
A)28
B)38
C)-38
D)125
E)17
A)28
B)38
C)-38
D)125
E)17

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
21
The number N of bacteria in a refrigerated food is given by where T is the temperature of the food in degrees Celsius.When the food is removed from refrigeration,the temperature of the food is given by where t is the time in hours. Find the bacteria count after 0.5 hour.
A)About 565 bacteria
B)About 793 bacteria
C)About 653 bacteria
D)About 390 bacteria
E)About 705 bacteria
A)About 565 bacteria
B)About 793 bacteria
C)About 653 bacteria
D)About 390 bacteria
E)About 705 bacteria

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
22
Determine whether the statement is true or false.
If f(x)= x + 1 and g(x)= 5x,then .
A)False
B)True
If f(x)= x + 1 and g(x)= 5x,then .
A)False
B)True

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
23
Consider the functions and .
Find .
A)
B)
C)
D)
E)
Find .
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
24
Find and the domain of the composite function.
A) Domain of : all real numbers x except
B) Domain of : all real numbers x except
C) Domain of : all real numbers x except
D) Domain of : all real numbers x except
E) Domain of : all real numbers x except
A) Domain of : all real numbers x except
B) Domain of : all real numbers x except
C) Domain of : all real numbers x except
D) Domain of : all real numbers x except
E) Domain of : all real numbers x except

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
25
Find and the domain of the composite function.
A) Domain of : all real numbers x
B) Domain of : all real numbers x
C) Domain of : all real numbers x
D) Domain of : all real numbers x
E) Domain of : all real numbers x
A) Domain of : all real numbers x
B) Domain of : all real numbers x
C) Domain of : all real numbers x
D) Domain of : all real numbers x
E) Domain of : all real numbers x

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
26
A pebble is dropped into a calm pond,causing ripples in the form of concentric circles.The radius (in feet)of the outer ripple is ,where t is the time in seconds after the pebble strikes the water.The area of the circle is given by the function .Find and interpret .
A) ; represents the area of the circle at time t.
B) ; represents the area of the circle at time t.
C) ; represents the area of the circle at time t.
D) ; represents the area of the circle at time t.
E) ; represents the area of the circle at time t.
A) ; represents the area of the circle at time t.
B) ; represents the area of the circle at time t.
C) ; represents the area of the circle at time t.
D) ; represents the area of the circle at time t.
E) ; represents the area of the circle at time t.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
27
From 2003 through 2008,the sales (in thousands of dollars)for one of two restaurants owned by the same parent company can be modeled by where t = 3 represents 2003.During the same six-year period,the sales (in thousands of dollars)for the second restaurant can be modeled by
Write a function that represents the total sales of the two restaurants owned by the same parent company.
A)
B)
C)
D)
E)
Write a function that represents the total sales of the two restaurants owned by the same parent company.
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
28
The spread of a contaminant is increasing in a circular pattern on the surface of a lake.The radius of the contaminant can be modeled by ,where r is the radius in meters and t is the time in hours since contamination.
Find a function that gives the area A of the circular lake in terms of the time since the spread began.
A)
B)
C)
D)
E)
Find a function that gives the area A of the circular lake in terms of the time since the spread began.
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
29
Find and the domain of the composite function.
A) Domain of : all real numbers x except
B) Domain of : all real numbers x except
C) Domain of : all real numbers x except
D) Domain of : all real numbers x except
E) Domain of : all real numbers x except
A) Domain of : all real numbers x except
B) Domain of : all real numbers x except
C) Domain of : all real numbers x except
D) Domain of : all real numbers x except
E) Domain of : all real numbers x except

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
30
Find and the domain of the composite function.
A) Domain of : all real numbers x
B) Domain of : all real numbers x
C) Domain of : all real numbers x
D) Domain of : all real numbers x
E) Domain of : all real numbers x
A) Domain of : all real numbers x
B) Domain of : all real numbers x
C) Domain of : all real numbers x
D) Domain of : all real numbers x
E) Domain of : all real numbers x

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
31
The suggested retail price of a new hybrid car is p dollars.The dealership advertises a factory rebate of $2000 .
Select a function R in terms of p giving the cost of the hybrid car after receiving the rebate from the factory.
A)
B)
C)
D)
E)
Select a function R in terms of p giving the cost of the hybrid car after receiving the rebate from the factory.
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
32
The weekly cost C of producing units x in a manufacturing process is given by .The number of units x produced in t hours is given by .
Find the cost of the units produced in 6 hours.
A)11,855
B)11,850
C)11,846
D)13,350
E)11,854
Find the cost of the units produced in 6 hours.
A)11,855
B)11,850
C)11,846
D)13,350
E)11,854

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
33
Find and the domain of the composite function.
A) Domain of : all real numbers x
B) Domain of : all real numbers x
C) Domain of : all real numbers x
D) Domain of : all real numbers x
E) Domain of : all real numbers x
A) Domain of : all real numbers x
B) Domain of : all real numbers x
C) Domain of : all real numbers x
D) Domain of : all real numbers x
E) Domain of : all real numbers x

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
34
The number of people playing tennis T (in millions)in the United States from 2000 through 2007 can be approximated by the function and the U.S.population P (in millions)from 2000 through 2007 can be approximated by the function ,where t represents the year,with t = 0 corresponding to 2000.
Evaluate the function for t = 0 and 3.
A)h(0)= 0.1060,h(3)= 0.0783
B)h(0)= 0.3060,h(3)= 0.2783
C)h(0)= -0.2060,h(3)= -0.1783
D)h(0)= 0.1783,h(3)= 0.2060
E)h(0)= -0.1060,h(3)= -0.0783
Evaluate the function for t = 0 and 3.
A)h(0)= 0.1060,h(3)= 0.0783
B)h(0)= 0.3060,h(3)= 0.2783
C)h(0)= -0.2060,h(3)= -0.1783
D)h(0)= 0.1783,h(3)= 0.2060
E)h(0)= -0.1060,h(3)= -0.0783

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
35
The total numbers of Navy personnel N (in thousands)and Marines personnel M (in thousands)from 2000 through 2007 can be approximated by the models and where t represents the year,with t = 0 corresponding to 2000.
Find and interpret .
A) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
B) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
C) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
D) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
E) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
Find and interpret .
A) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
B) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
C) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
D) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.
E) ,which represents the difference between the number of Navy personnel and the number of Marines personnel.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
36
The number of people playing tennis T (in millions)in the United States from 2000 through 2007 can be approximated by the function and the U.S.population P (in millions)from 2000 through 2007 can be approximated by the function ,where t represents the year,with t = 0 corresponding to 2000.
Find .
A)
B)
C)
D)
E)
Find .
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
37
The total numbers of Navy personnel N (in thousands)and Marines personnel M (in thousands)from 2000 through 2007 can be approximated by the models and where t represents the year,with t = 0 corresponding to 2000.
Find and interpret .
A) ,which represents the total number of Navy and Marines personnel combined.
B) ,which represents the total number of Navy and Marines personnel combined.
C) ,which represents the total number of Navy and Marines personnel combined.
D) ,which represents the total number of Navy and Marines personnel combined.
E) ,which represents the total number of Navy and Marines personnel combined.
Find and interpret .
A) ,which represents the total number of Navy and Marines personnel combined.
B) ,which represents the total number of Navy and Marines personnel combined.
C) ,which represents the total number of Navy and Marines personnel combined.
D) ,which represents the total number of Navy and Marines personnel combined.
E) ,which represents the total number of Navy and Marines personnel combined.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
38
The research and development department of an automobile manufacturer has determined that when a driver is required to stop quickly to avoid an accident,the distance (in feet)the car travels during the driver's reaction time is given by ,where x is the speed of the car in miles per hour.The distance (in feet)traveled while the driver is braking is given by .Find the function that represents the total stopping distance T.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
39
Find and the domain of the composite function.
A) Domain of : all real numbers x
B) Domain of : all real numbers x
C) Domain of : all real numbers x
D) Domain of : all real numbers x
E) Domain of : all real numbers x
A) Domain of : all real numbers x
B) Domain of : all real numbers x
C) Domain of : all real numbers x
D) Domain of : all real numbers x
E) Domain of : all real numbers x

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
40
Find (f + g)(x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
41
Let 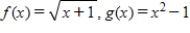 .Find the composite function.
.Find the composite function. 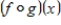
 .Find the composite function.
.Find the composite function. 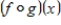

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
42
Let f (x)= 2x + 1,g(x)= 3x - 2.Find the function.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
43
Let f (x)= 2x - 1,g(x)= 3x - 2.Find the domain of the function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
44
Use the graphs of f and g to evaluate the function. 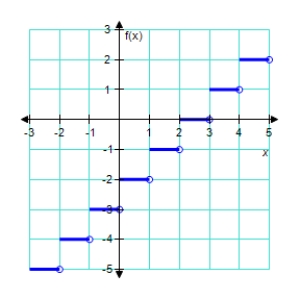
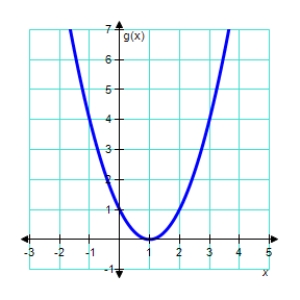
A)1
B)-2
C)4
D)-1
E)2


A)1
B)-2
C)4
D)-1
E)2

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
45
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
46
Find ( fg )(x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
47
Let f (x)= 3x,g (x)= x + 1.Find the composite function. 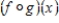
Please give the respnce as an expression (not an equation).
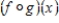
Please give the respnce as an expression (not an equation).

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
48
Evaluate the indicated function for and .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
49
Evaluate the indicated function for and . ( fg )(1)
A)15
B)-35
C)-23
D)-25
E)-33
A)15
B)-35
C)-23
D)-25
E)-33

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
50
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
51
Let .Find the composite function which expresses the given correspondence correctly.
A)
B)
C)
D)
E)none of the above
A)
B)
C)
D)
E)none of the above

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
52
Let f (x)= x2 - 1,g (x)= 3x - 2.Find the value of the function. 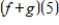
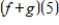

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
53
Find ( f / g )(x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
54
The monthly cost C of running the machinery in a factory for t hours is given by The number of hours t needed to produce x products is given by . Find the equation representing the cost C of manufacturing x products.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
55
Let f (x)= 2x + 1,g (x)= 3x - 2.Find the function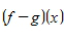
Please give the responce as a simplified expression (not an equation).
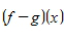
Please give the responce as a simplified expression (not an equation).

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
56
Find ( f − g )(x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
57
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
58
Find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck



